Tag: Eureka Math Geometry Module 2

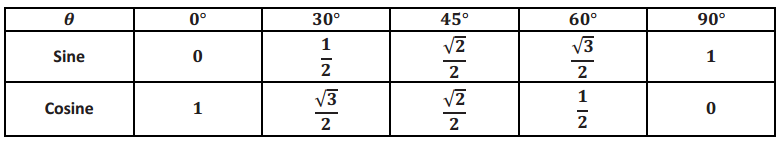
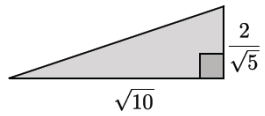
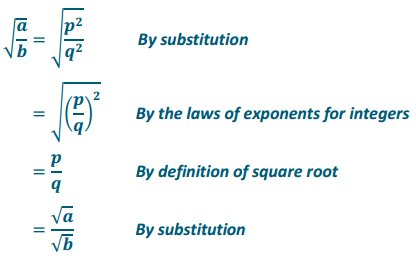
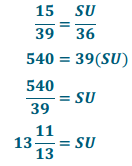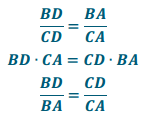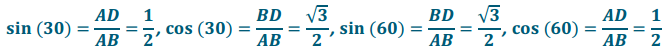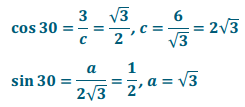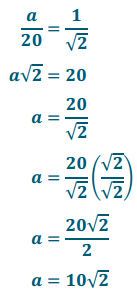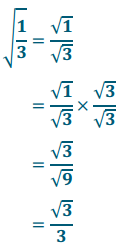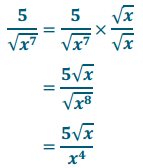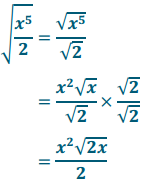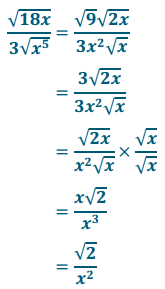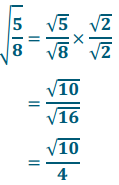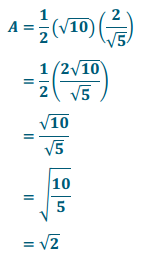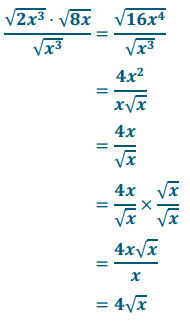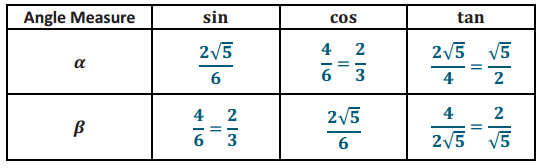Engage NY Eureka Math Geometry Module 2 Lesson 21 Answer Key

Eureka Math Geometry 2 Module 2 Lesson 21 Example Answer Key

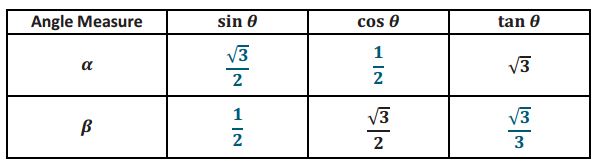
Example 1.
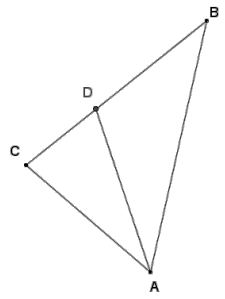
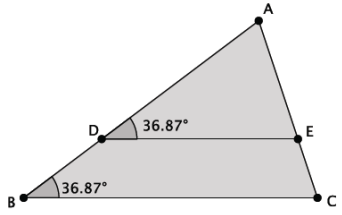
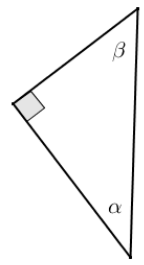
Recall that an altitude of a triangle is a perpendicular line segment from a vertex to the line determined by the opposite side. In ∆ ABC below, \(\overline{B D}\) is the altitude from vertex B to the line containing \(\overline{A C}\).

a. How many triangles do you see n the figure?
Answer:
There are three triangles in the figure.

b. Identify the three triangles by name.
Note that there are many ways to name the three triangles. Ensure that the names students give show corresponding angles.
Answer:
∆ ABC, ∆ ADB, and ∆ BDC.

We want to consider the altitude of a right triangle from the right angle to the hypotenuse. The altitude of a right triangle splits the triangle Into two right triangles, each of which shares a common acute angle with the original triangle. In ∆ ABC, the altitude \(\overline{B D}\) divides the right triangle into two sub-triangles, ∆ BDC and ∆ ADB.

c. Is ∆ ABC ~ ∆ BDC? Is ∆ ABC ~ ∆ ADB? Explain.
Answer:
∆ ABC and ∆ BDC are similar by the AA criterion. Each has a right angle, and each shares ∠C. ∆ ABC and ∆ ADB are similar because each has a right angle, and each shares ∠A, so, again, these triangles are similar by the AA criterion.

d. Is ∆ ABC ~ ∆ DBC? Explain.
Answer:
∆ ABC and ∆ DBC are not similar, because their corresponding angles, under the given correspondence of vertices, do not have equal measure.

e. Since ∆ ABC ~ ∆ BDC and ∆ ABC ~ ∆ ADB, can we conclude that ∆ BDC ~ ∆ ADB? Explain.
Answer:
Since similarity is transitive, ∆ ABC ~ ∆ BDC and ∆ ABC ~ ∆ ADB implies that ∆ ABC ~ ∆ BDC ~ ∆ ADB.

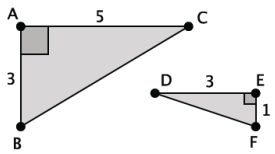
f. Identify the altitude drawn in ∆ EFG.
Answer:
\(\overline{G H}\) is the altitude from vertex G to the line
containing \(\overline{E F}\).

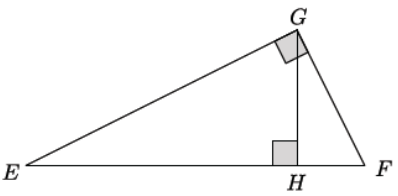
g. As before, the altitude divides the triangle into two sub-triangles, resulting in a total of three triangles including the given triangle. Identify them by name so that the corresponding angles match up.
Answer:
∆ EFG, ∆ GFH, and ∆ EGH

h. Does the altitude divide ∆ EFG into two similar sub-triangles as the altitude did with ∆ ABC?
Answer:
Yes.

Example 2
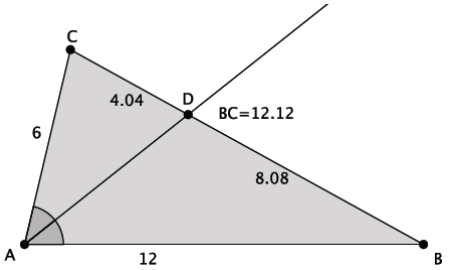
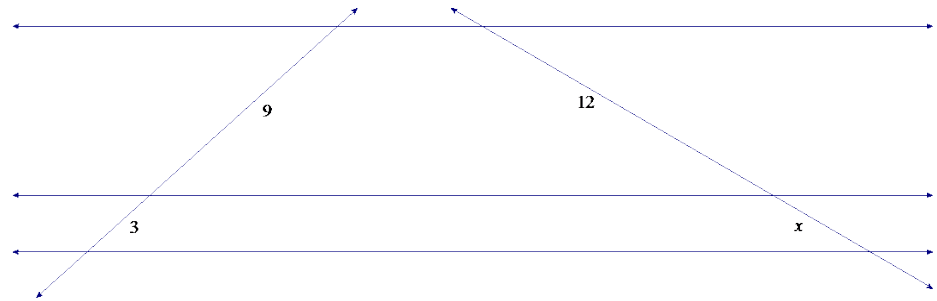
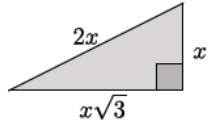
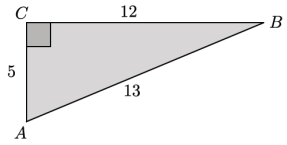
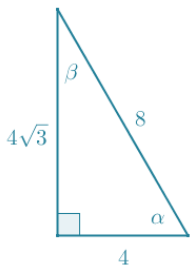
Consider the right triangle ∆ ABC below.

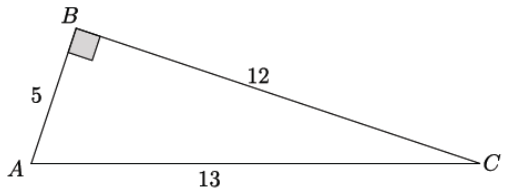
Draw the altitude \(\overline{B D}\) from vertex B to the line containing \(\overline{A C}\). Label \(\overline{A D}\) as x, \(\overline{D C}\) as y, and \(\overline{B D}\) as z.

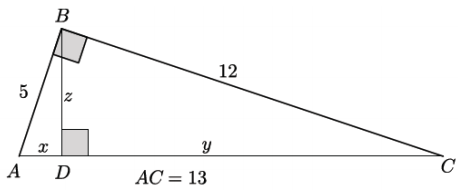
Find the values of x, y, and z.
Answer:
Provide students time to find the values of x, y, and z. Allow students to use any reasonable strategy to complete the task. The suggested time allotment for this part of the example is 5 minutes. Next, have students briefly share their solutions and explanations for finding the lengths x = 1\(\frac{12}{13}\), y = 11\(\frac{1}{13}\), and z = 4\(\frac{8}{13}\). For example, students may first use what they know about similar triangles and corresponding side lengths having equal ratios to determine x, then use the equation x + y = 13 to determine the value of y, and finally use the Pythagorean theorem to determine the length of z.

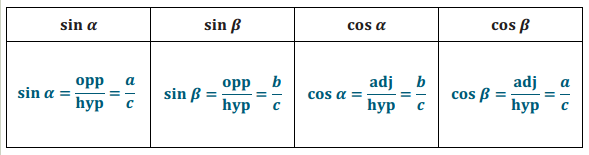
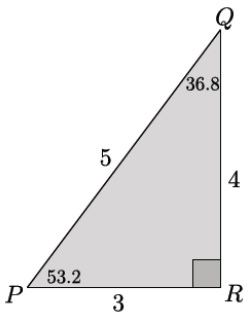
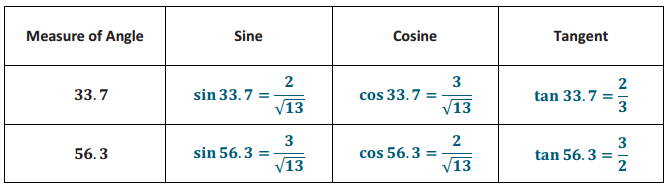
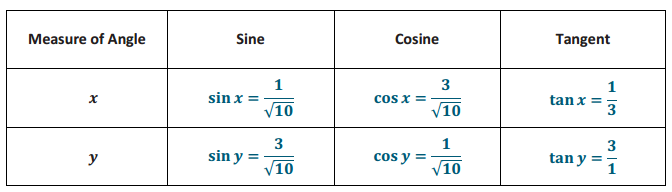
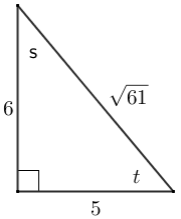
Now we will look at a different strategy for determining the lengths of X, y, and z. The strategy requires that we complete a table of ratios that compares different parts of each triangle.
Answer:
Students may struggle with the initial task of finding the values of x, y, and z. Encourage them by letting them know that they have all the necessary tools to find these values. When transitioning to the use of ratios to find values, explain any method that yields acceptable answers. We are simply looking to add another tool to the toolbox of strategies that apply in this situation.
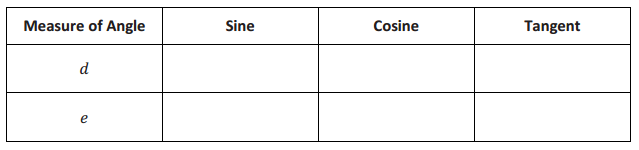
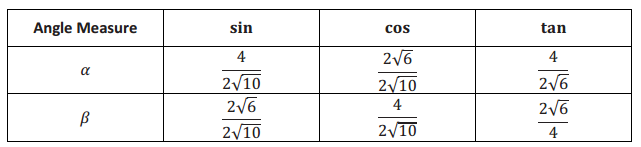
Provide students a moment to complete the ratios related to ∆ ABC.

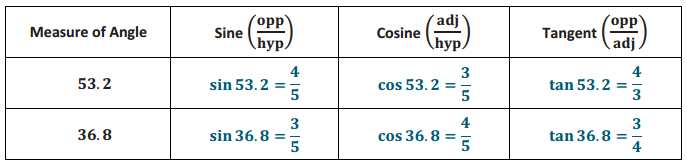
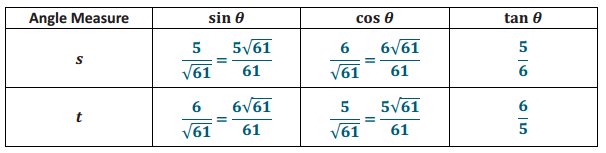
Make a table of ratios for each triangle that relates the sides listed in the column headers.

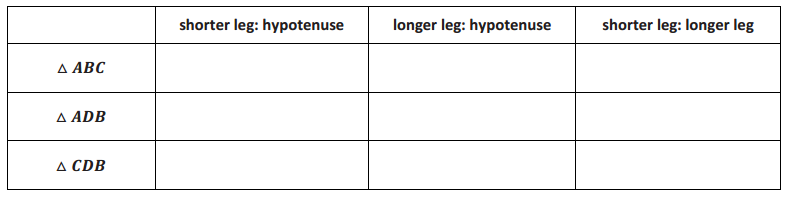
Answer:

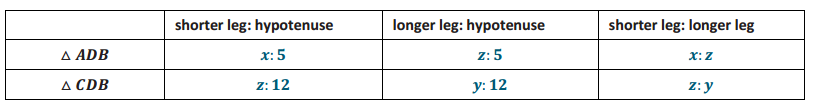
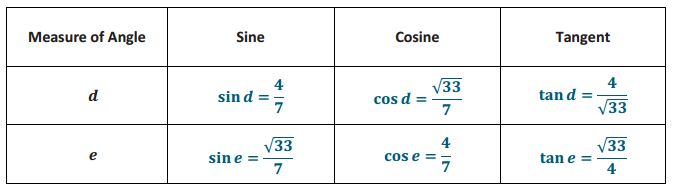
Our work in Example 1 showed us that ∆ ABC ~ ∆ ADB ~ ∆ CDB. Since the triangles are similar, the ratios of their corresponding sides are equal. For example, we can find the length of x by equating the values of shorter leg: hypotenuse ratios of ∆ ABC and ∆ ADB.
\(\frac{x}{5}=\frac{5}{13}\)
13x = 25
\(\frac{25}{13}=1 \frac{12}{13}\)

Why can we use these ratios to determine the length of x?
Answer:
We can use these ratios because the triangles are similar. Similar triangles have ratios of corresponding sides that are equal We also know that we can use ratios between figures or within figures. The ratios used were within-figure ratios.

Which ratios can we use to determine the length of y?
Answer:
To determine the value of y, we can equate the values longer leg: hypotenuse ratios for ∆ CDB and ∆ ABC.
\(\frac{y}{12}=\frac{12}{13}\)
13y = 144
\(\frac{144}{13}=11 \frac{1}{13}\)

Use ratios to determine the length of z.
Answer:
To determine the value of z, we can equate the values of longer leg: hypotenuse ratios for ∆ ADB and ∆ ABC:
\(\frac{z}{5}=\frac{12}{13}\)
13z = 60
z = \(\frac{60}{13}=4 \frac{8}{13}\)

To determine the value of z, we can equate the values of shorter leg: hypotenuse ratios for ∆ CDB and ∆ ABC:
\(\frac{z}{12}=\frac{5}{13}\)
13z = 60
z = \(\frac{60}{13}=4 \frac{8}{13}\)

To determine the value of z, we can equate the values of shorter leg: longer leg ratios for ∆ ADB and ∆ ABC:

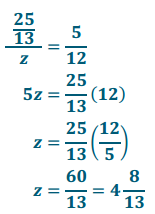
To determine the value of z, we can equate the values of shorter leg: longer leg ratios for ∆ CDB and ∆ ABC:

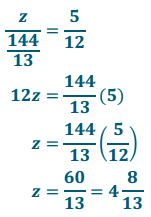
Since corresponding ratios within similar triangles are equal, we can solve for any unknown side length by equating the values of the corresponding ratios. In the coming lessons, we will learn about more useful ratios for determining unknown side lengths of right triangles.

Eureka Math Geometry 2 Module 2 Lesson 21 Opening Exercise Answer Key

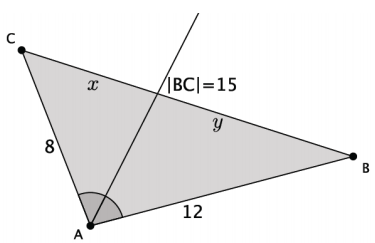
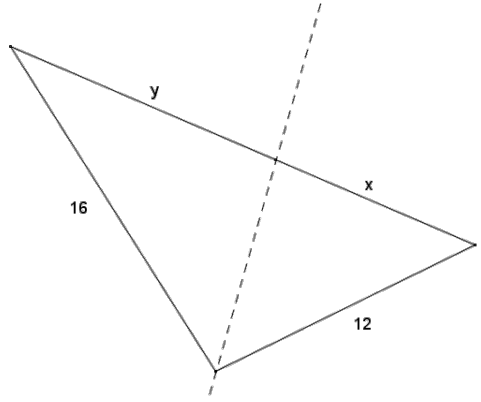
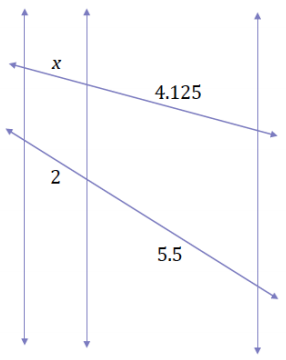
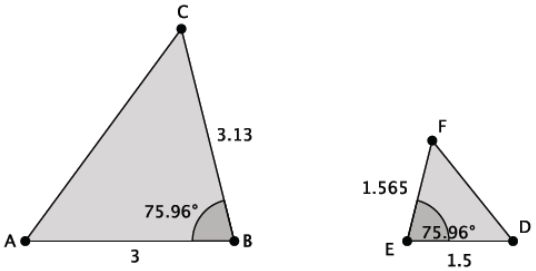
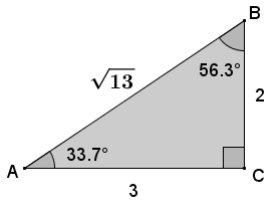
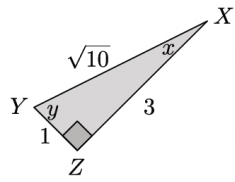
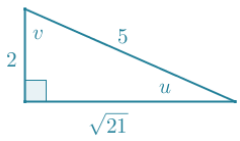
Use the diagram below to complete parts (a)-(c).

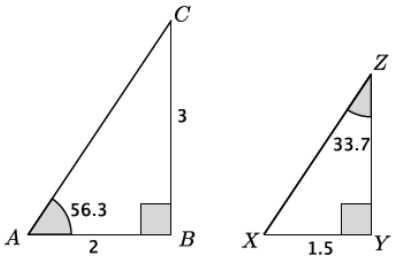
a. Are the triangles shown above similar? Explain.
Answer:
Yes, the triangles are similar by the AA criterion. Both triangles have a right angle, and m∠A = m∠X and m∠C = m∠Z.

b. Determine the unknown lengths of the triangles.
Answer:
Let x represent the length of \(\overline{Y Z}\).
\(\frac{3}{2}=\frac{x}{1.5}\)
2x = 4.5
x = 2.25

Let y be the length of the hypotenuse of ∆ ABC.
2² + 3² = y²
4 + 9 = y²
13 = y²
√13 = y

Let z be the length of the hypotenuse of ∆ XYZ.
1. 5² + 2.2² = z²
2.25 + 5.0625 = z²
7.3125 = z²
\(\sqrt{7.3125}\) = z

c. Explain how you found the lengths in part (a).
Answer:
Since the triangles are similar, I used the values of the ratios of the corresponding side lengths to determine the length of \(\overline{X Z}\). To determine the lengths of \(\overline{A C}\) and \(\overline{Y Z}\), I used the Pythagorean theorem.

Eureka Math Geometry 2 Module 2 Lesson 21 Problem Set Answer Key

Question 1.
a.

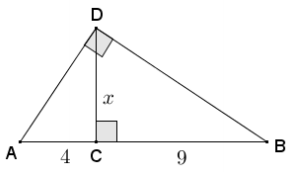
Answer:
∆ ACD ~ ∆ DCB by AA criterion, so corresponding sides are
proportional.
\(\frac{x}{4}=\frac{9}{x}\)
x² = 36
x = √6 = 6

b.

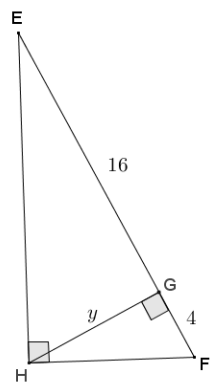
Answer:
∆ GHE ~ ∆ GFH by AA criterion, so corresponding sides are
proportional.
\(\frac{y}{4}=\frac{16}{y}\)
y² = 64
y = √64 = 8

c.

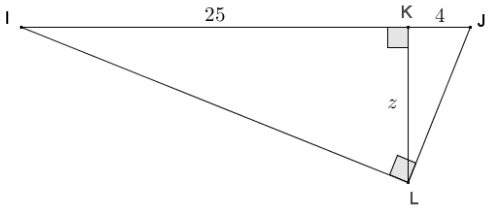
Answer:
∆ LKI ~ ∆ IKL by AA criterion, so corresponding sides are proportional:
\(\frac{z}{25}=\frac{4}{z}\)
z² = 100
y = √100 = 10

d. Describe the pattern that you see in your calculations for parts (a) through (c).
Answer:
For each of the given right triangles, the length of the altitude drawn to its hypotenuse is equal to the square root of the product of the lengths of the pieces of the hypotenuse that it cuts.

Question 2.
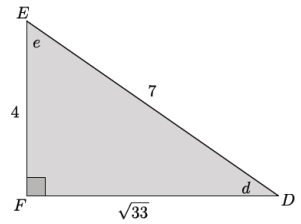
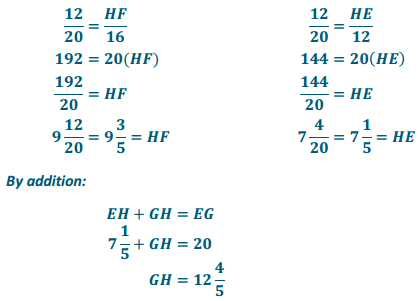
Given right triangle EFG with altitude \(\overline{F H}\) drawn to the hypotenuse, find the lengths of \(\overline{E H}\), \(\overline{F H}\), and \(\overline{G H}\).

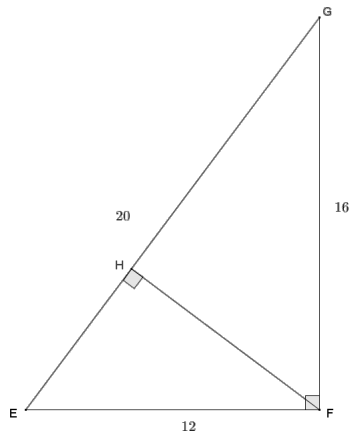
Answer:
The altitude drawn from F to H cuts triangle EFG into two similar sub-triangles, providing the following correspondence:
∆ EFG ~ ∆ EHF ~ ∆ FHG
Using the ratio shorter leg: hypotenuse for the similar triangles:

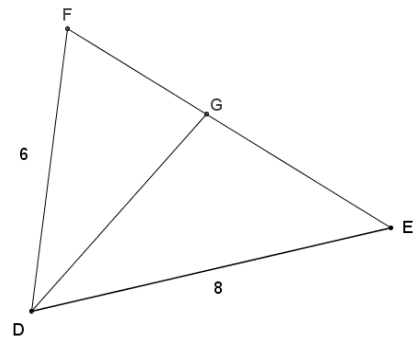
Question 3.
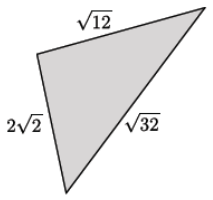
Given triangle IMJ with altitude \(\overline{J L}\), JL = 32, and IL = 24, find IJ, JM, LM, and IM.

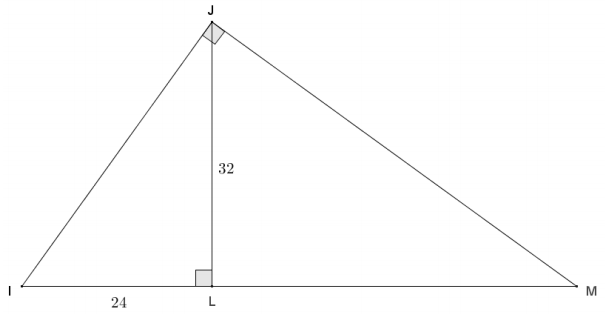
Answer:
Altitude \(\overline{J L}\) cuts ∆ IMJ into two similar sub-triangles such that ∆ IMJ ~ ∆ JML ~ ∆ IJL.
By the Pythagorean theorem:
24² + 32² = IJ²
576 + 1024 = IJ²
1600 = IJ²
√1600 = IJ
40 = IJ

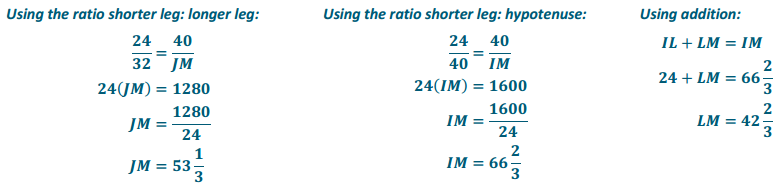
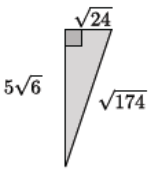
Question 4.
Given right triangle RST with altitude \(\overline{R U}\) to its hypotenuse, TU = 1\(\frac{24}{25}\), and RU = 6\(\frac{18}{25}\), find the lengths of the sides of ∆ RST.

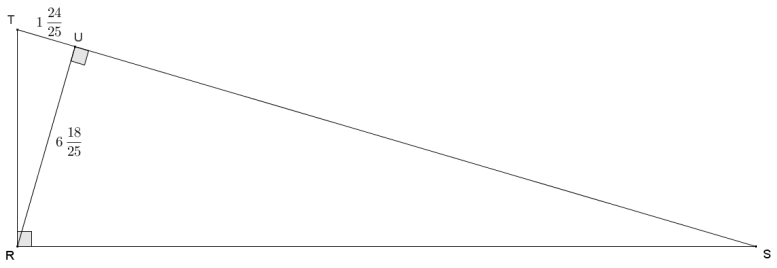
Answer:
Altitude \(\overline{R U}\) cuts ∆ RST Into similar sub-triangles: ∆ URT ~ ∆ USR.
Using the Pythagorean theorem:

Using the ratio shorter leg: hypotenuse:
\(\frac{\frac{49}{25}}{7}=\frac{7}{S T}\)
\(\frac{49}{25}\)ST = 49
ST = 25

Using the Pythagorean theorem:
RS² + RT² = ST²
RS² + 7² = 25²
RS² + 49 = 625
RS² = 576
RS = √576 = 24

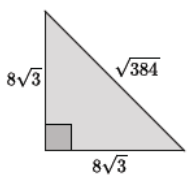
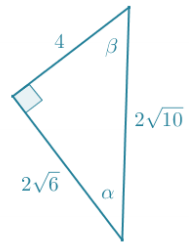
Question 5.
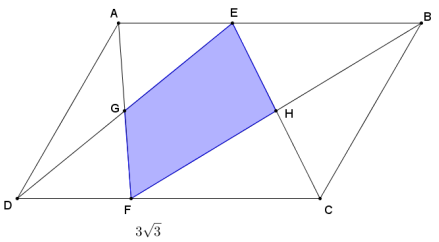
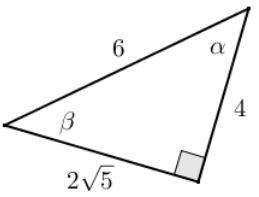
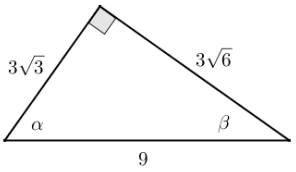
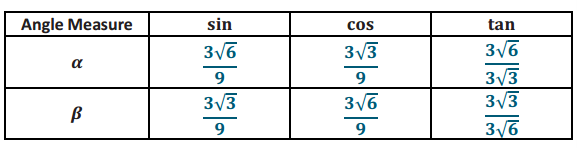
Given right triangle ABC with altitude \(\overline{C D}\), find AD, BD, AB, and DC.

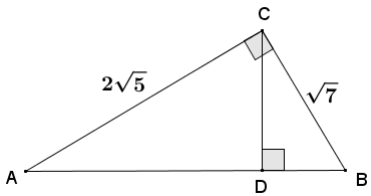
Answer:
Using the Pythagorean theorem:
(2√5)² + (√7)² = AB²
20 + 7 = AB²
27 = AB²
√27 = AB
3√3 = AB
An altitude from the right angle in a right triangle to the hypotenuse cuts the triangle into two similar right triangles: ∆ ABC ~ ∆ ACD ~ ∆ CBD.

Using the ratio shorter leg: hypotenuse:

Question 6.
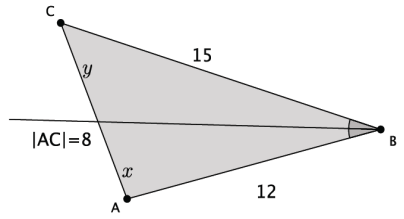
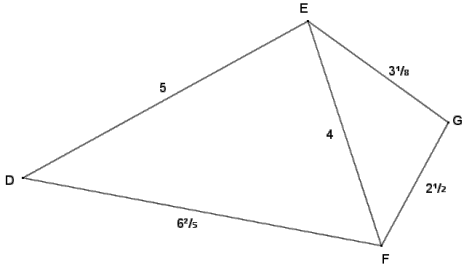
Right triangle DEC is inscribed in a circle with radius AC = 5. \(\overline{D C}\) is a diameter of the circle, \(\overline{E F}\) is an altitude of ∆ DEC, and DE = 6. Find the lengths x and y.

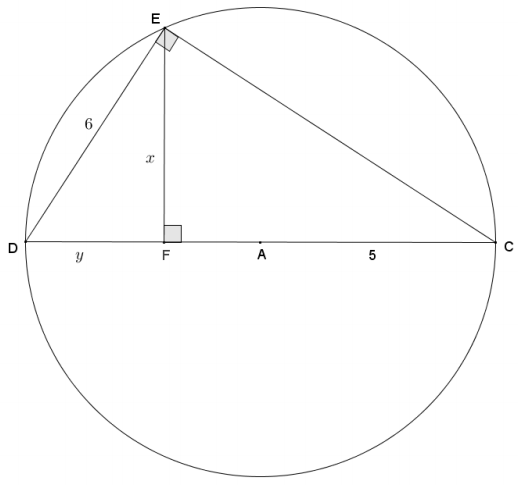
Answer:
The radius of the circle is 5, and DC = 2(5) = 10.

By the Pythagorean theorem:
6² + EC² = 10²
36 + EC² = 100
EC² = 64
EC = 8
(EC = – 8 is also a solution; however, since EC represents a distance, its value must be positive, so the solution EC = – B is disregarded.)

We showed that an altitude from the right angle of a right triangle to the hypotenuse cuts the triangle into two similar sub-triangles, so
∆ DEC ~ ∆ DFE ~ ∆ EFC.

Using the ratio shorter leg: hypotenuse for similar right triangles:
\(\frac{6}{10}=\frac{y}{6}\)                       \(\frac{6}{10}=\frac{x}{8}\)
36 = 10y                       48 = 10x
3.6 = y                          4.8 = x

Question 7.
In right triangle ABD, AB = 53, and altitude DC = 14. Find the lengths of \(\overline{B C}\) and \(\overline{A C}\).

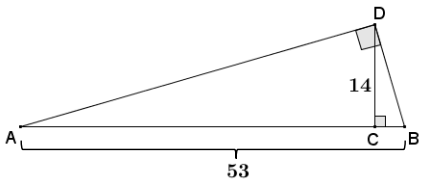
Answer:
Let length BC = x. Then, AC = 53 – x.
Using the pattern from Problem 1,
14² = x(53 – x)
196 = 53x – x²
x² – 53x + 196 = 0
(x – 49) (x – 4) = 0
x = 49 or x = 4.
Using the solutions from the equation and the given information, either BC = 4 and AC = 49, or BC = 49 and AC = 4.

Eureka Math Geometry 2 Module 2 Lesson 21 Exit Ticket Answer Key

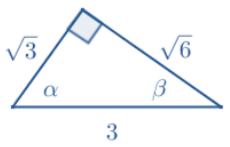
Given ∆ RST, with altitude \(\overline{S U}\) drawn to its hypotenuse, ST = 15, RS = 36, and RT = 39, answer the questions below.

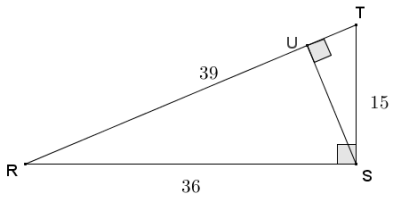
Question 1.
Complete the similarity statement relating the three triangles in the diagram.
∆ RST ~ ∆ _______ ~ ∆ ________
Answer:
∆ RST ~ ∆   RUS    ~ ∆   SUT  
Using the right angles and shared angles, the triangles are similar by AA criterion. The transitive property may also be used.

Question 2.
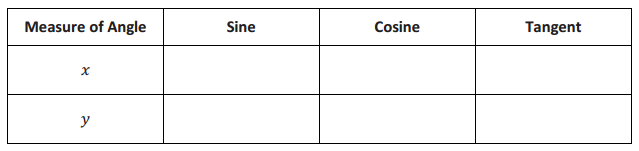
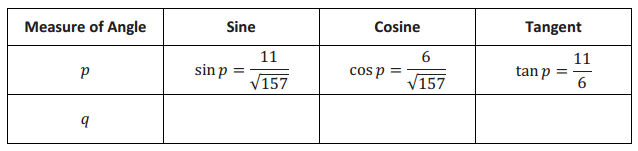
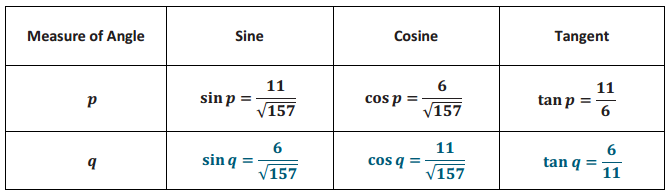
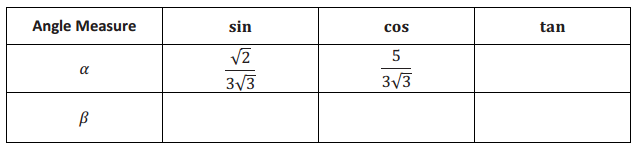
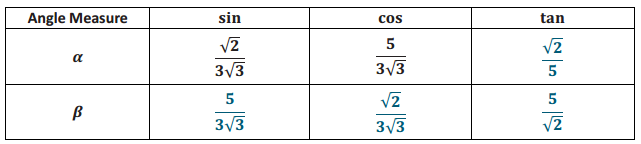
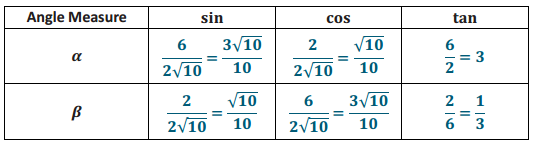
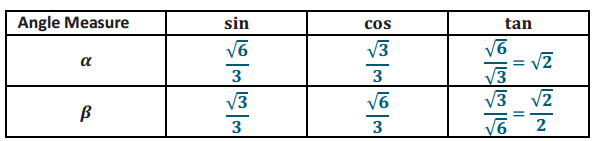
Complete the table of ratios specified below.

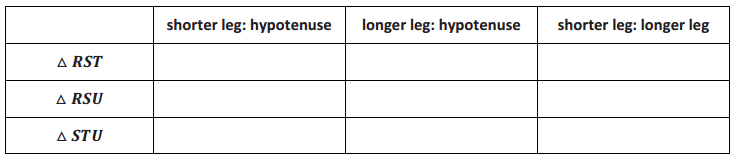
Answer:

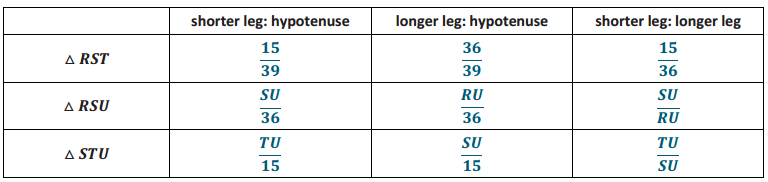
Question 3.
Use the values of the ratios you calculated to find the length of \(\overline{S U}\).
Answer:

Engage NY Eureka Math Geometry Module 2 Lesson 18 Answer Key

Eureka Math Geometry Module 2 Lesson 18 Opening Exercise Answer Key

a. What is an angle bisector?
Answer:
The bisector of an angle is a ray in the Interior of the angle such that the two adjacent angles formed by it have equal measures.

b. Describe the angle relationships formed when parallel lines are cut by a transversal.
Answer:
When parallel lines are cut by a transversal, the corresponding, alternate interior, and alternate exterior angles are all congruent; the same-side interior angles are supplementary.

c. What are the properties of an isosceles triangle?
Answer:
An isosceles triangle has at least two congruent sides, and the angles opposite to the congruent sides (i.e., the base angles) are also congruent.

Discussion

In the diagram below, the angle bisector of ∠A in ∆ ABC meets side \(\overline{B C}\) at point D. Does the angle bisector create any observable relationships with respect to the side lengths of the triangle?

Answer:
Acknowledge any relationships students may find, but highlight the relationship BD: CD = BA: CA. Then, continue the discussion below that proves this relationship.

Eureka Math Geometry Module 2 Lesson 18 Exercise Answer Key

Exercise 1.
The sides of a triangle are 8, 12, and 15. An angle bisector meets the side of length 15. Find the lengths x and y. Explain how you arrived at your answers.

Answer:
\(\frac{y}{x}=\frac{12}{8}\)                  x = 15 – 9
x= 15 – y                 x = 6
8y = 12x
8y = 12(15 – y)
8y = 180 – 12y
20y = 180
y = 9
The length x is 6, and the length y is 9.

Since I know that ∠A is bisected, i applied what I knew about the angle bisector theorem to determine the lengths x and y. Specifically, the angle bisector cuts the side that is opposite the bisected angle so that y: x = 12:8. I set up an equation using the values of the ratios, which could be solved once I rewrote one of the variables x or y. I rewrote x as 15 – y and then solved for y. Once I had a value for y, I could replace it in the equation x = 15 – y to determine the value of X.

Exercise 2.
The sides of a triangle are 8, 12, and 15. An angle bisector meets the side of length 12. Find the lengths x and y.

Answer:
y = 12- x                  y = 12 – 4\(\frac{4}{23}\)
\(\frac{x}{12-x}=\frac{8}{15}\)                  y = 7\(\frac{19}{23}\)
15x = 8(12 – x)
15x = 96 – 8x
23x = 96
x = \(\frac{96}{23}=4 \frac{4}{23}\)
The length of x is 4\(\frac{4}{23}\) and the length of y is 7\(\frac{19}{23}\).

Exercise 3.
The sides of a triangle are 8, 12, and 15. An angle bisector meets the side of length 8. Find the lengths x and y.

Answer:
\(\frac{y}{x}=\frac{15}{12}\)                  y = 8 – 3\(\frac{5}{9}\)
y = 8 – x                  y = 4\(\frac{4}{9}\)
15x = 12y
15x = 12(8-x)
15x = 96 – 12x
27x = 96
x =\(\frac{96}{27}\) = 3\(\frac{15}{27}\) = 3\(\frac{5}{9}\)
The length of x is 3\(\frac{5}{9}\) and the length of y is 4\(\frac{5}{9}\).

Exercise 4.
The angle bisector of an angle splits the opposite side of a triangle into lengths 5 and 6. The perimeter of the triangle is 33. Find the lengths of the other two sides.
Answer:
Let z be the scale factor of a similarity. By the angle bisector theorem, the side of the triangle adjacent to the segment of length 5 has length of 5z, and the side of the triangle adjacent to the segment of length 6 has length of 6z. The sum of the sides is equal to the perimeter.
5 + 6 + 5z + 6z = 33
11 + 11z = 33
11z = 22
z = 2
5(2) = 10, and 6(2) = 12 The lengths of the other two sides are 10 and 12.

Eureka Math Geometry Module 2 Lesson 18 Problem Set Answer Key

Question 1.
The sides of a triangle have lengths of 5, 8, and 6\(\frac{1}{2}\). An angle bisector meets the side of length 6\(\frac{1}{2}\). Find the lengths X and y.
Answer:
Using the angle bisector theorem, \(\frac{x}{y}=\frac{5}{8}\) and y = 6 \(\frac{1}{2}\) – x so

Question 2.
The sides of a triangle are 10\(\frac{1}{2}\), 16\(\frac{1}{2}\) and 9. An angle bisector meets the side of length 9. Find the lengths x and y.

Answer:
By the angle bisector theorem, \(\frac{x}{y}=\frac{16 \frac{1}{2}}{10 \frac{1}{2}}\), and y = 9 – x, so
\(\frac{x}{9-x}=\frac{16.5}{10.5}\)                     y = 9 – x
10.5x = 16.5(9 – x)                  y = 9 – 5.5
10.5x = 148.5 – 16.5x                  y = 3.5
27x = 148.5
x = 5.5

Question 3.
In the diagram of triangle DEF below, \(\overline{D G}\) is an angle bisector, DE = 8, DF = 6, and EF = 8\(\frac{1}{6}\). Find FG and EG.

Answer:

Question 4.
Given the diagram below and ∠BAD ≅ ∠DAC, show that BD: BA = CD: CA.

Answer:
Using the given information, Th is the angle bisector of angle A. By the angle bisector theorem, BD : CD = BA : CA, so

Question 5.
The perimeter of triangle LMN is 32 cm. \(\overline{N X}\) is the angle bisector of angle N, LX = 3 cm, and XM = 5 cm. Find LN and MN.
Answer:
Since \(\overline{N X}\) is an angle bisector of angle N, by the angle bisector theorem, XL: XM = LN: MN; thus, LN: MN = 3: 5.
Therefore, LN = 3x and MN = 5x for some positive number x. The perimeter of the triangle is 32 cm, so
XL + XM + MN + LN = 32
3 + 5 + 3x + 5x = 32
8 + 8x = 32
8x = 24
x = 3
3x = 3(3) = 9 and 5x = 5(3) = 15
LN = 9cm and MN = 15cm

Question 6.
Given CD = 3, DB = 4, BF = 4, FE = 5, AB = 6, and ∠CAD ≅∠DAB ≅ ∠BAF ≅ ∠FAE, find the perimeter of quadrilateral AEBC.

Answer:
\(\overline{A D}\) is the angle bisector of angle CAB, so by the angle bisector theorem, CD: BD = CA: BA.
\(\frac{3}{4}=\frac{C A}{6}\)
4 · CA = 18
CA = 4.5
\(\overline{A D}\) is the angle bisector of angle BAE, so by the angle bisector theorem, BF: EF = BA: EA.
\(\frac{4}{5}=\frac{6}{E A}\)
4 · EA = 30
EA = 7.5
The perimeter of quadrilateral AEBC:
AEBC = CD + DB + BF + FE + EA + AC
AEBC = 3 + 4 + 4 + 5 + 7.5 + 4.5
AEBC = 28

Question 7.
If \(\overline{A E}\) meets \(\overline{B C}\) at D such that CD: BD = CA: BA, show that ∠CAD ≅ ∠BAD. Explain how this proof relates to the angle bisector theorem.

Answer:

Question 8.
In the diagram below, \(\overline{E D}\) ≅ \(\overline{D B}\), \(\overline{B E}\) bisects ∠ABC, AD = 4, and DC = 8. Prove that ∆ ADB ~ ∆ CEB.

Answer:
Using given information, ED = DB, so it follows that EB = 2DB. Since \(\overline{B E}\) bisects ∠ABC, ∠ABD ≅ ∠CBD. Also, by the angle bisector theorem, \(\frac{C D}{A D}=\frac{B C}{B A}\), which means \(\frac{B C}{B A}=\frac{8}{4}=\frac{2}{1}\).

Since \(\frac{E B}{D B}=\frac{C B}{A B}=\frac{2}{1}\), and ∠ABD ≅ ∠CBD, ∆ ADB ~ ∆ CEB by the SAS criterion for showing similar triangles.

Eureka Math Geometry Module 2 Lesson 18 Exit Ticket Answer Key

Question 1.
The sides of a triangle have lengths of 12, 16, and 21. An angle bisector meets the side of length 21. Find the lengths x and y.

Answer:
By the angle bisector theorem, \(\), and y = 21 – x, so
\(\frac{21-x}{x}=\frac{16}{12}\)                     y = 21 – x
12(21 – x) = 16x                   y = 21 – 9
252 – 12x = 16x                   y = 12
252 = 28x
9 = x

Question 2.
The perimeter of ∆ UVW is 22\(\frac{1}{2}\). \(\overrightarrow{W Z}\) bisects ∠UWV, UZ = 2, and VZ = 2\(\frac{1}{2}\). Find UW and VW.

Answer:
By the angle bisector theorem, \(\frac{2}{2.5}=\frac{U W}{V W}\) so UW = 2x and VW = 2. 5x for some positive number x. The perimeter of the triangle is 22\(\frac{1}{2}\), s0
2 + 2.5 + 2x + 2.5x = 22.5
4.5 + 4.5x = 22.5
4.5x = 18
x = 4
UW = 2x = 2(4) = 8
VW = 2.5x = 2.5(4) = 10

Engage NY Eureka Math Geometry Module 2 Lesson 27 Answer Key

Eureka Math Geometry 2 Module 2 Lesson 27 Example Answer Key

If α and β are the measurements of complementary angles, then we are going to show that sin α = cos β.

In right triangle ABC, the measurement of acute angle ∠A is denoted by α, and the measurement of acute angle ∠B is denoted by β?.

Determine the following values in the table:

Answer:

What can you conclude from the results?
Answer:
Since the ratios for sin α and cos β are the same, sin α = cos β, and the ratios for cos α and sin β are the same; additionally, cos α = sin β. The sine of an angle is equal to the cosine of its complementary angle, and the cosine of an angle is equal to the sine of its complementary angle.

→ Therefore, we conclude for complementary angles α and β that sin α = cos β, or in other words, when 0 < 6 < 90 that sin(90 – θ) = cos θ, and sin θ = cos (90 – θ). Any two angles that are complementary can be realized as the acute angles in a right triangle. Hence, the co- prefix in cosine is a reference to the fact that the cosine of an angle is the sine of its complement.

Example 2.
What is happening to a and b as θ changes? What happens to sin θ and cos θ?

Answer:
→ There are values for sine and cosine commonly known for certain angle measurements. Two such angle measurements are when θ = 0° and θ = 90°.

→ To better understand sine and cosine values, imagine a right triangle whose hypotenuse has a fixed length c of 1 unit. We illustrate this by imagining the hypotenuse as the radius of a circle, as in the image.

→ What happens to the value of the sine ratio as θ approaches 0°? Consider what is happening to the opposite side, a.

With one end of the meter stick fixed at A, rotate it like the hands of a clock, and show how a decreases as θ decreases. Demonstrate the change in the triangle for each case.

As θ gets closer to 0°, a decreases. Since sin θ = the value of sin θ is also approaching 0.

→ Similarly, what happens to the value of the cosine ratio as θ approaches 0°? Consider what is happening to the adjacent side, b.
As θ gets closer to 0°, b increases and becomes closer to 1. Since cos θ = \(\frac{b}{1}\) the value of cos θ is approaching 1.

→ Now, consider what happens to the value of the sine ratio as θ approaches 90°. Consider what is happening to the opposite side, a.
As θ gets closer to 90°, a increases and becomes closer to 1. Since sin θ = \(\frac{a}{1}\) the value of sin θ is also approaching 1.

→ What happens to the value of the cosine ratio as θ approaches 90°? Consider what is happening to the adjacent side, b.
As θ gets closer to 90°, b decreases and becomes closer to 0. Since cos θ = \(\frac{b}{1}\) the value of cos θ is approaching 0.

→ Remember, because there are no right triangles with an acute angle of 0° or of 90°, in the above thought experiment, we are really defining sin 0 = 0 and cos 0 = 1.

→ Similarly, we define sin 90 = 1 and cos 90 = 0; notice that this falls in line with our conclusion that the sine of an angle is equal to the cosine of its complementary angle.

Example 3
There are certain special angles where it is possible to give the exact value of sine and cosine. These are the angles that measure 0°, 30°, 45°, 60°, and 90°; these angle measures are frequently seen.

You should memorize the sine and cosine of these angles with quick recall just as you did your arithmetic facts.
a. Learn the following sine and cosine values of the key angle measurements.

We focus on an easy way to remember the entries In the table. What do you notice about the table values?
Answer:
The entries for cosine are the same as the entries for sine but ¡n the reverse order.

This is easily explained because the pairs (0, 90), (30, 60), and (45, 45) are the measures of complementary angles.
So, for instance, sin 30 = cos 60.

The sequence 0, \(\frac{1}{2}, \frac{\sqrt{2}}{2}, \frac{\sqrt{3}}{2}\), 1 may be easier to remember as the sequence \(\frac{\sqrt{0}}{2}, \frac{\sqrt{1}}{2}, \frac{\sqrt{2}}{2}, \frac{\sqrt{3}}{2}, \frac{\sqrt{4}}{2}\).

b. ∆ ABC is equilateral, with side length 2; D is the midpoint of side \(\overline{A C}\). Label all side lengths and angle measurements for ∆ ABD. Use your figure to determine the sine and cosine of 30 and 60.

Answer:

c. Draw an isosceles right triangle with legs of length 1. What are the measures of the acute angles of the triangle? What is the length of the hypotenuse? Use your triangle to determine sine and cosine of the acute angles.

Answer:

Parts (b) and (c) demonstrate how the sine and cosine values of the mentioned special angles can be found. These triangles are common to trigonometry; we refer to the triangle in part (b) as a 30-60-90 triangle and the triangle in part (c) as a 45-45-90 triangle.

Eureka Math Geometry Module 2 Lesson 27 Exercise Answer Key

Exercise 1.
Consider the right triangle ABC so that ∠C is a right angle, and the degree measures of ∠A and ∠B are α and β, respectively.

a. Find α + β.
Answer:
90°

b. Use trigonometric ratios to describe \(\frac{B C}{A B}\) two different ways.
Answer:
sin ∠A = \(\frac{B C}{A B}\), cos ∠B = \(\frac{B C}{A B}\)

c. Use trigonometric ratios to describe \(\frac{A C}{A B}\) two different ways.
Answer:
sin ∠B = \(\frac{A C}{A B}\), cos∠A = \(\frac{A C}{A B}\)

d. What can you conclude about sin α and cos β?
Answer:
sin α = cos β

e. What can you conclude about cos α and sin β?
Answer:
cos α = sin β

Exercise 2.
Find values for θ that make each statement true.
a. sin θ = cos (25)
Answer:
θ = 65

b. sin 80 = cos θ
Answer:
θ = 10

c. sin θ = cos (θ + 10)
Answer:
θ = 40

d. sin(θ – 45) = cos(θ)
Answer:
θ = 67.5

Exercise 3.
For what angle measurement must sine and cosine have the same value? Explain how you know.
Answer:
Sine and cosine have the same value for θ = 45. The sine of an angle is equal to the cosine of its complement.
Since the complement of 45 is 45, sin 45 = cos 45.

Exercise 4.
Find the missing side length in the triangle.

Answer:

Exercise 5.
Find the missing side length in the triangle.

Answer:

Eureka Math Geometry Module 2 Lesson 27 Problem Set Answer Key

Question 1.
Find the value of θ that makes each statement tr
a. sin θ = cos(θ + 38)
Answer:
cos(90 – θ) = cos(θ + 3θ)
90 – θ = θ + 3θ
52 = 2θ
26 = θ

b. cos θ = sin(θ – 30)
Answer:
sin(90 – θ) = sin(θ – 30)
90 – θ = θ – 30
120 = 2θ
60 = θ

c. sin θ = cos(3θ + 20)
Answer:
cos(90 – θ) = cos(3θ + 20)
90 – θ = 3θ + 20
70 = 4θ
17.5 = θ

d. sin(\(\frac{\theta}{3}\) + 10) = cos θ
Answer:
sin (\(\frac{\theta}{3}\) + 10) = sin(90 – θ)
\(\frac{\theta}{3}\) + 10 = 90 – θ
\(\frac{4 \theta}{3}\) = 80
θ = 60

Question 2.
a. Make a prediction about how the sum sin 30 + cos 60 will relate to the sum sin 60 + cos 30.
Answer:
Answers will vary; however, some students may believe that the sums will be equal. This is explored in Problems 3 through 5.

b. Use the sine and cosine values of special angles to find the sum: sin 30 + cos 60.
Answer:
sin 30 = \(\frac{1}{2}\) and cos 60 = \(\frac{1}{2}\) Therefore, sin 30+ cos 60 = \(\frac{1}{2}\) + \(\frac{1}{2}\) = 1.
Alternative strategy:
cos 60° = sin (90 – 60)° = sin 30°
sin 300 + cos 60° = sin 30° + sin 30° = 2(sin 30°) = 2 (\(\frac{1}{2}\)) = 1

c. Find the sum: sin 60 + cos 30.
Answer:
sin 60 = \(\frac{\sqrt{3}}{2}\) and cos30 = \(\frac{\sqrt{3}}{2}\) Therefore, sin 60 + cos 30 = \(\frac{\sqrt{3}}{2}+\frac{\sqrt{3}}{2}\) = √3.

d. Was your prediction a valid prediction? Explain why or why not.
Answer:
Answers will vary.

Question 3.
Langdon thinks that the sum sin 30 + sin 30 is equal to sin 60. Do you agree with Langdon? Explain what this means about the sum of the sines of angles.
Answer:
I disagree. Explanations may vary. It was shown in the solution to Problem 3 that sin 30 + sin 30 = 1, and it is known that sin 60 = \(\frac{\sqrt{3}}{2}\) ≠ 1. This shows that the sum of the sines of angles is not equal to the sine of the sum of the angles.

Question 4.
A square has side lengths of 7√2. Use sine or cosine to find the length of the diagonal of the square. Confirm your answer using the Pythagorean theorem.

Answer:
The diagonal of a square cuts the square into two congruent 45 – 45 – 90 right triangles. Let d represent the length of the diagonal of the square:
cos 45 = \(\frac{\sqrt{2}}{2}\)
\(\frac{\sqrt{2}}{2}=\frac{7 \sqrt{2}}{d}\)
d√2 = 14√2
d = 14
Confirmation using Pythagorean theorem:
(7√2)² + (7√2)² = hyp²
98 + 98 = hyp²
196 = hyp²
√196 = hyp
14 = hyp

Question 5.
Given an equilateral triangle with sides of length 9, find the length of the altitude. Confirm your answer using the Pythagorean theorem.
Answer:

An altitude drawn within an equilateral triangle cuts the equilateral triangle into two congruent 30 – 60 – 90 right triangles. Let h represent the length of the altitude:
sin 60 = \(\frac{\sqrt{3}}{2}\)
\(\frac{\sqrt{3}}{2}=\frac{h}{9}\)
9√3 = 2h
\(\frac{9 \sqrt{3}}{2}\) = h
The altitude of the triangle has a length of \(\frac{9 \sqrt{3}}{2}\).
Confirmation using Pythagorean theorem:

Eureka Math Geometry Module 2 Lesson 27 Exit Ticket Answer Key

Question 1.
Find the values for θ that make each statement true.
a. sin θ = cos 32
Answer:
θ = 90 – 32
θ = 58

b. cos θ = sin(θ + 20)
Answer:
sin(90 – θ) = sin(θ + 20)
90 – θ = θ + 20
70 = 2θ
35 = θ

Question 2.
∆ LMN is a 30-60-90 right triangle. Find the unknown lengths x and y.

Answer:

Engage NY Eureka Math Geometry Module 2 Lesson 24 Answer Key

Eureka Math Geometry Module 2 Lesson 24 Exercise Answer Key

Exercise 1.
Find the length of the hypotenuse of a right triangle whose legs have lengths 50 and 100.
Answer:
c² = 50² + 100²
c² = 2500 + 10000
c² = 12500
√c² = √12500
c = \(\sqrt{2^{2} \cdot 5^{5}}\)
c = 2 · 5² √5
c = 50√5

Exercise 2.
Can you think of a simpler method for finding the length of the hypotenuse in Exercise 1? Explain.
Answer:
Accept any reasonable methods. Students may recall from Grade 8 that they can use what they know about similar triangles and scale factors to make their computations easier.

Exercise 3.
Find the length of the hypotenuse of a right triangle whose legs have lengths 75 and 225.
Answer:
A right triangle with leg lengths 75 and 225 has leg lengths that are 75 times longer than a triangle with leg lengths 1 and 3. A triangle with leg lengths 1 and 3 has a hypotenuse of length √10. Therefore, the length of the hypotenuse of a triangle with leg lengths 75 and 225 is 75√10.

Exploratory Challenge/Exercise 4-5

Exercise 4.
An equilateral triangle has sides of length 2 and angle measures of 60°, as shown below. The altitude from one vertex to the opposite side divides the triangle into two right triangles.
a. Are those triangles congruent? Explain.

Answer:
Yes, the two right triangles are congruent by ASA. Since the altitude is perpendicular to the base, then each of the right triangles has angles of measure 90° and 60°. By the triangle sum theorem, the third angle has a measure of 30°. Then, each of the right triangles has corresponding angle measures of 30° and 60°, and the included side length is 2.

b. What is the length of the shorter leg of each of the right triangles? Explain.
Answer:
Since the total length of the base of the equilateral triangle is 2, and the two right triangles formed are congruent, then the bases of each must be equal in length. Therefore, the length of the base of one right triangle is 1.

c. Use the Pythagorean theorem to determine the length of the altitude.
Answer:
Let h represent the length of the altitude.
1² + h² = 2²
h² = 2² – 1²
h² = 3
h = √3

d. Write the ratio that represents shorter leg: hypotenuse.
Answer:
1: 2

e. Write the ratio that represents longer leg: hypotenuse.
Answer:
√3: 2

f. Write the ratio that represents shorter leg: longer leg.
Answer:
1: √3

g. By the AA criterion, any triangles with measures 30 – 60 – 90 will be similar to this triangle. If a 30 – 60 – 90 triangle has a hypotenuse of length 16, what are the lengths of the legs?

Answer:
Consider providing the following picture for students:
Let a represent the length of the shorter leg.
\(\frac{a}{16}=\frac{1}{2}\)
a = 8

Let b represent the length of the longer leg.
\(\frac{b}{16}=\frac{\sqrt{3}}{2}\)
2b = 16√3
b = 8√3

The length a = 8, and the length b = 8√3.

Note: After finding the length of one of the legs, some students may have used the ratio shorter leg: longer leg to determine the length of the other leg.

Exercise 5.
An isosceles right triangle has leg lengths of 1, as shown.
a. What are the measures of the other two angles? Explain.

Answer:
Base angles of an isosceles triangle are equal; therefore, the other two angles have a measure of 45°.

b. Use the Pythagorean theorem to determine the length of the hypotenuse of the right triangle.
Answer:
Let c represent the length of the hypotenuse.
1² + 1² = c²
2 = c²
√2 = c

c. Is It necessary to write all three ratios: shorter leg: hypotenuse, longer leg: hypotenuse, and shorter leg: longer leg? Explain.
Answer:
No, it is not necessary to write all three ratios. The reason is that the shorter leg and the longer leg are the same length. Therefore, the ratios shorter leg: hypotenuse and longer leg: hypotenuse will be the same. Additionally, the shorter leg: longer leg ratio would be 1: 1, which is not useful since we are given that the right triangle is an isosceles right triangle.

d. Write the ratio that represents leg: hypotenuse.
Answer:
1: √2

e. By the AA criterion, any triangles with measures 45 – 45 – 90 will be similar to this triangle. If a 45 – 45 – 90 triangle has a hypotenuse of length 20, what are the lengths of the legs?
Answer:
Let a represent the length of the leg.

Eureka Math Geometry Module 2 Lesson 24 Problem Set Answer Key

Question 1.
In each row of the table below are the lengths of the legs and hypotenuses of different right triangles. Find the missing side lengths In each row, In simplest radical form.

Leg1	Leg2	Hypotenuse
15		25
15	36
3		7
100	200

Answer:

Leg1	Leg2	Hypotenuse
15	20	25
15	36	39
3	2√10	7
100	200	100√5

Answers provided in table
By the Pythagorean theorem:
leg₁² + leg₁² = hyp²
15² + y² = 25²
225 + y² = 625
y² = 400
y = 20 or y = – 20
The case where y = – 20 does not make sense since it represents a length of a side of a triangle and Is, therefore, disregarded.

Alternative strategy:
Divide each side length by the greatest common factor to get the side lengths of a similar right triangle. Find the missing side length for the similar triangle, and multiply by the GCF.

Leg: 15, Hypotenuse: 25, GCF(15, 25) = 5
Consider the triangle with Leg: 3 and Hypotenuse: 5. This Is a 3-4-5 right triangle. The missing leg length is 5 · 4 = 20.

Legs: 100 and 200: GCF(100, 200) = 100
Consider the right triangle with Legs: 1 and 2. Hypotenuse = √5. The missing hypotenuse length is 100√5.

Question 2.
Claude sailed his boat due south for 38 miles and then due west for 25 miles. Approximately how far is Claude from where he began?
Answer:

Claude’s path forms a right triangle since south and west are perpendicular to each other. His distance from where he began is a straight line segment represented by the hypotenuse of the triangle.
38² + 25² = hyp²
1444 + 625 = hyp2
2069 = hyp²
√2069 = hyp
Claude is approximately 45.5 miles from where he began.

Question 3.
Find the lengths of the legs in the triangle given the hypotenuse with length 100.

Answer:
By the Pythagorean theorem:
leg² + leg² = 100²
2 leg² 10000
leg² = 5000
leg = √5000
leg = √2500 √2
leg = 50√2

Alternative strategy:
The right triangle is an isosceles right triangle, so the leg lengths are equal. The hypotenuse of an isosceles right triangle can be calculated as follows:
hyp= leg · √2
100 = leg√2
\(\frac{100}{\sqrt{2}}\) = leg
\(\frac{100 \sqrt{2}}{2}\) = 50√2 = leg
The legs of the 45 – 45 – 90 right triangle with a hypotenuse of 100 are 50√2.

Question 4.
Find the length of the hypotenuse in the right triangle given that the legs have lengths of 100.

Answer:
By the Pythagorean theorem:
100² + 100² = hyp²
10000 + 10000 = hyp²
20000 = hyp²
√20000 = hyp
√10000 √2 = hyp²
100√2 = hyp

Alternative strategy:
The given right triangle is a 45 – 45 – 90 triangle. Therefore, the ratio of the length of its legs to the length of its hypotenuse is 1: √2
hyp = leg · √2
hyp = 100√2
The hypotenuse of the right triangle with legs of length 100 is 100√2.

Question 5.
Each row in the table below shows the side lengths of a different 30 – 60 – 90 right triangle. Complete the table with the missing side lengths in simplest radical form. Use the relationships of the values in the first three rows to complete the last row. How could the expressions in the last row be used?

Shorter Leg	Longer Leg	Hypotenuse
25		50
15
	3	2√3
x

Answer:

Shorter Leg	Longer Leg	Hypotenuse
25	25√3	50
15	15√3	30
√3	3	2√3
x	x√3	2x

The last row of the table shows that the sides of a 30 – 60 – 90 right triangle are multiples of 1, 2, and √3 by some constant x, with 2x being the longest and, therefore, the hypotenuse. The expressions could be used to find two unknown sides of a 30 – 60 – 90 triangle where only one of the sides is known.

Question 6.
In right triangle ABC with ∠C a right angle, an altitude of length h is dropped to side \(\overline{A B}\) that splits the side \(\overline{A B}\) into segments of length x and y. Use the Pythagorean theorem to show h² = xy.

Answer:
By the Pythagorean theorem, a² + b² = C².
Since c = x + y, we have
a² + b² = (x + y)²
a² + b² = x² + 2xy + y².
Also by the Pythagorean theorem,
a² = h² + y², and b² = h² + x², so
a² + b² = h² + y² + h² + x²
a² + b² = 2h² + x² + y².
Thus, by substitution,
x² + 2xy + y² = 2h² + x² + y²
2xy = 2h²
xy = h².

Question 7.
In triangle ABC, the altitude from ∠C splits side \(\overline{A B}\) into two segments of lengths x and y. If h denotes the length of the altitude and h² = xy, use the Pythagorean theorem and its converse to show that triangle ABC is a right triangle with ∠C a right angle.

Answer:
Let a, b, and c be the lengths of the sides of the triangle opposite and ∠C, respectively. By the Pythagorean theorem:
a² = h² + y² and b² = h² + x², so
a² + b² = h² + y² + h² + x²
a² + b² = x² + 2h² + y²
a² + b² = x² + 2xy + y²
a² + b² = (x + y)²
a² + b² = c².
So, by the converse of the Pythagorean theorem, ∆ ABC is a right triangle, a and b are the lengths of legs of the triangle, and c is the hypotenuse, which lies opposite the right angle. Therefore, ∠C is the right angle of the right triangle.

Eureka Math Geometry Module 2 Lesson 24 Exit Ticket Answer Key

A right triangle has a leg with a length of 18 and a hypotenuse with a length of 36. Bernie notices that the hypotenuse is twice the length of the given leg, which means it is a 30 – 60 – 90 triangle. If Bernie is right, what should the length of the remaining leg be? Explain your answer. Confirm your answer using the Pythagorean theorem.
Answer:
A right angle and two given sides of a triangle determine a unique triangle. All 30 – 60 – 90 triangles are similar by AA criterion, and the lengths of their sides are 1c, 2c, and c√3 for some positive number c. The given hypotenuse is twice the length of the given leg of the right triangle, so Bernie’s conclusion is accurate. The ratio of the length of the short leg to the length of the longer leg of any 30 – 60 – 90 triangle is 1: √3 The given leg has a length of 18, which is \(\frac{1}{2}\) of the hypotenuse and, therefore, must be the shorter leg of the triangle.

Engage NY Eureka Math Geometry Module 2 Lesson 19 Answer Key

Eureka Math Geometry Module 2 Lesson 19 Opening Exercise Answer Key

Show x: y = x’: y’ is equivalent to x: x’ = y: y’.

Answer:

Eureka Math Geometry Module 2 Lesson 19 Exercise Answer Key

Exercise 1.

Answer:
x = 4

Exercise 2.

Answer:
x = 1.5

Eureka Math Geometry Module 2 Lesson 19 Problem Set Answer Key

Question 1.
Given the diagram shown, \(\overline{A D}\|\overline{G J}\| \overline{L O} \| \overline{Q T}\) and \(\overline{A Q}\|\overline{B R}\| \overline{C S} \| \overline{D T}\) Use the additional information given in each part below to answer the questions:

a. If GL = 4, what is HM?
Answer:
GHML forms a parallelogram since opposite sides are parallel, and opposite sides of a parallelogram are equal in length; therefore, HM = GL = 4.

b. If GL = 4, LQ = 9, and XV = 5, what is YZ?
Answer:
Parallel lines cut transversals proportionally; therefore, it is true that = \(\frac{G L}{L Q}=\frac{X Y}{Y Z}\), and likewise YZ = \(\frac{L Q}{G L}\) (XV).
YZ = \(\frac{9}{4}\)(5)
YZ= 11\(\frac{1}{4}\)

c. Using information from part (b), If CI = 18, what is WX?
Answer:
By the same argument used in part (a), IN = GL = 4. Parallel lines cut transversals proportionally; therefore, it is true that \(\frac{C I}{I N}=\frac{W X}{X Y}\), and likewise WX = \(\frac{C I}{I N}\)(XY).
WX = \(\frac{18}{4}\)(5)
WX = 22\(\frac{1}{2}\)

Question 2.
Use your knowledge about families of parallel lines to find the coordinates of point P on the coordinate plane below.

Answer:
The given lines on the coordinate plane are parallel because they have the same slope m = 3. First, draw a horizontal transversal through points (8, -2) and (10, -2) and a second transversal through points (10,4) and (10, -4). The transversals intersect at (10, -2). Parallel lines cut transversals proportionally, so using horizontal and vertical distances, \(\frac{6}{2}=\frac{2}{x}\), where x represents the distance from point (10, -2) to P.
x = \(\frac{2}{6}\)(2)
x = \(\frac{4}{6}=\frac{2}{3}\)
Point P is \(\frac{2}{3}\) unit more than 10, or 10\(\frac{2}{3}\), so the coordinates of point Pare (1o\(\frac{2}{3}\), -2).

Question 3.
ACDB and FCDE are both trapezoids with bases \(\overline{A B}, \overline{F E}\), and \(\overline{C D}\). The perimeter of trapezoid ACDB is 24\(\frac{1}{2}\). If the ratio of AF: FC is 1: 3, AB = 7, and ED = 5\(\frac{5}{8}\), find AF, FC, and BE.

Answer:

Question 4.
Given the diagram and the ratio of a: b is 3: 2, answer each question below:

a. Write an equation for a_n in terms of b_n.
Answer:
a_n = \(\frac{3}{2}\)b_n

b. Write an equation for b_n in terms of a_n.
Answer:
b_n = \(\frac{2}{3}\)a_n

c. Use one of your equations to find b₁ in terms of a if a₁ = 1.2(a).
Answer:
b_n = \(\frac{2}{3}\)a_n
b₁ = \(\frac{2}{3}\left(\frac{12}{10} a\right)\)
b₁ = \(\frac{4}{5}\)a

d. What is the relationship between b₁ and b?
Answer:
Using the equation from parts (a) and (c), a = \(\frac{3}{2}\)b, so b₁ = \(\frac{4}{5}\left(\frac{3}{2} b\right)\); thus, b1 = \(\frac{6}{5}\)b.

e. What constant, c, relates b1 and b? Is this surprising? Why or why not?
Answer:
The constant relating b1 and b is the same constant relating a1 to a, c = \(\frac{12}{10}\) = 1.2.

f. Using the formula a_n = c · a_{n – 1}, find a₃ in terms of a.
Answer:

g. Using the formula b_n = c · b_n-1, find b₃ in terms of b.
Answer:
b₃ = \(\frac{216}{125}\)b

h. Use your answers from parts (f) and (g) to calculate the value of the ratio of a₃: b₃.
Answer:

Question 5.
julius wants to try to estimate the circumference of the earth based on measurements made near his home. He cannot find a location near his home where the sun Is straight overhead. Will he be able to calculate the circumference of the earth? If so, explain and draw a diagram to support your claim.
Answer:
Note to the teacher: This problem is very open-ended, requires critical thinking, and may not be suitable for all students. The problem may be scaffolded by providing a diagram with possible measurements that Julius made based on the description in the student solution below.

Possible solution: If Julius can find two locations such that those locations and their shadows lie in the same straight path, then the difference of the shadows’ angles can be used as part of the 360° in the earth’s circumference. The distance between those two locations corresponds with that difference of angles.

Eureka Math Geometry Module 2 Lesson 19 Exit Ticket Answer Key

Question 1.
Given the diagram shown, \(\overline{A G}\) || \(\overline{B H}\) || \(\overline{C I}\), AB = 6.5 cm, GH = 7.5 cm, and HI = 18 cm. Find BC.

Answer:

Question 2.
Martin the Martian lives on Planet Mart. Martin wants to know the circumference of Planet Mart, but it is too large to measure directly. He uses the same method as Eratosthenes by measuring the angle of the sun’s rays in two locations. The sun shines on a flagpole in Martinsburg, but there is no shadow. At the same time, the sun shines on a flagpole in Martville, and a shadow forms a 10° angle with the pole. The distance from Martville to Martinsburg is 294 miles. What is the circumference of Planet Mart?
Answer:
The distance from Martinsburg to Martville makes up only 10° of the total rotation about the planet. There are 360° in the complete circumference of the planet, and 36 · 10° = 360°, so 36 294 miles = 10584 miles.
The circumference of planet Mart is 10,584 miles.

Engage NY Eureka Math Geometry Module 2 Lesson 17 Answer Key

Eureka Math Geometry Module 2 Lesson 17 Opening Exercise Answer Key

a. Choose three lengths that represent the sides of a triangle. Draw the triangle with your chosen lengths using construction tools.
Answer:
Answers will vary. Sample response: 6 cm, 7 cm, and 8 cm.

b. Multiply each length in your original triangle by 2 to get three corresponding lengths of sides for a second triangle. Draw your second triangle using construction tools.
Answer:
Answers will be twice the lengths given in part (a). Sample response: 12 cm, 14 cm, and 16 cm.

c. Do your constructed triangles appear to be similar? Explain your answer.
Answer:
The triangles appear to be similar. Their corresponding sides are given as having lengths in the ratio 2: 1, and the corresponding angles appear to be equal in measure. (This can be verified using either a protractor or patty paper.)

d. Do you think that the triangles can be shown to be similar without knowing the angle measures?
Answer:
Answers will vary.

Eureka Math Geometry Module 2 Lesson 17 Exploratory Challenge/Exercise Answer Key

Exercise 1.
Examine the figure, and answer the questions to determine whether or not the triangles shown are similar.

a. What information is given about the triangles in Figure 1?
Answer:
We are given that ∠A is common to both ∆ ABC and ∆ AB’C’. We are also given information about some of the side lengths.

b. How can the information provided be used to determine whether ∆ ABC is similar to ∆ AB’C’?
Answer:
We know that similar triangles will have ratios of corresponding sides that are proportional; therefore, we can use the side lengths to check for proportionality.

c. Compare the corresponding side lengths of ∆ ABC and ∆ AB’C’. What do you notice?
Answer:
\(\frac{4}{13}=\frac{3}{9.75}\)
39 = 39
The cross-products are equal; therefore, the side lengths are proportional.

d. Based on your work in parts (a)-(c), draw a conclusion about the relationship between ∆ ABC and ∆ AB’C’. Explain your reasoning.
Answer:
The triangles are similar. By the triangle side splitter theorem, I know that when the sides of a triangle are cut proportionally, then \(\overline{B C}\) || \(\overline{B^{\prime} C^{\prime}}\). Then, I can conclude that the triangles are similar because they have two pairs of corresponding angles that are equal.

Exercise 2.
Examine the figure, and answer the questions to determine whether or not the triangles shown are similar.

a. What information is given about the triangles in Figure 2?
Answer:
We are given that ∠P is common to both ∆ PQR and ∆ PQ’R’. We are also given information about some of the side lengths.

b. How can the information provided be used to determine whether ∆ PQR is similar to ∆ PQ’R’?
Answer:
We know that similar triangles will have ratios of corresponding sides that are proportional; therefore, we can use the side lengths to check for proportionality.

c. Compare the corresponding side lengths of ∆ PQR and ∆ PQ’R’. What do you notice?
Answer:
\(\frac{3}{13} \neq \frac{2}{9}\)
27 ≠ 26
The side lengths are not proportional.

d. Based on your work in parts (a)-(c), draw a conclusion about the relationship between ∆ PQR and ∆ PQ’R’. Explain your reasoning.
Answer:
The triangles are not similar. The side lengths are not proportional, which is what I would expect in similar triangles. I know that the triangles have one common angle, but I cannot determine from the information given whether there is another pair of equal angles. Therefore, I conclude that the triangles are not similar.

Exercise 3.
Examine the figure, and answer the questions to determine whether or not the triangles shown are similar.

a. What information is given about the triangles in Figure 3?
Answer:
We are only given information related to the side lengths of the triangles.

b. How can the information provided be used to determine whether ∆ ABC is similar to ∆ A’B’C’?
Answer:
We know that similar triangles will have ratios of corresponding sides that are proportional; therefore, we can use the side lengths to check for proportionality.

c. Compare the corresponding side lengths of ∆ ABC and ∆ A’B’C’. What do you notice?
Answer:
\(\frac{1.41}{2.82}=\frac{3.5}{7}=\frac{2.7}{5.4}=\frac{1}{2}\)
The side lengths are proportional.

d. Based on your work in parts (a)-(c), make a conjecture about the relationship between ∆ ABC and ∆ A’B’C’. Explain your reasoning.
Answer:
I think that the triangles are similar. The side lengths are proportional, which is what I would expect in similar triangles.

Exercise 4.
Examine the figure, and answer the questions to determine whether or not the triangles shown are similar.

a. What information is given about the triangles in Figure 4?
Answer:
We are only given information related to the side lengths of the triangles.

b. How can the information provided be used to determine whether ∆ ABC is similar to ∆ A’B’C’?
Answer:
We know that similar triangles will have ratios of corresponding sides that are proportional; therefore, we can use the side lengths to check for proportionality.

c. Compare the corresponding side lengths of ∆ ABC and ∆ A’B’C’. What do you notice?
Answer:
\(\frac{2.1}{1.41} \neq \frac{8.29}{6.04} \neq \frac{7.28}{5.25}\)
The side lengths are not proportional.

d. Based on your work in parts (a)-(c), make a conjecture about the relationship between ∆ ABC and ∆ A’B’C’. Explain your reasoning.
Answer:
I think that the triangles are not similar. I would expect the side lengths to be proportional if the triangles were similar.

Exercise 5.
Are the triangles shown below similar? Explain. If the triangles are similar, write the similarity statement.

Answer:
\(\frac{1}{0.68} \neq \frac{6.4}{2.42} \neq \frac{5.83}{2.13}\)
There is no information about the angle measures, so we cannot use AA or SAS to conclude the triangles are similar. Since the side lengths are not proportional, we cannot use SSS to conclude the triangles are similar. Therefore, the triangles shown are not similar.

Exercise 6.
Are the triangles shown below similar? Explain. If the triangles are similar, write the similarity statement.

Answer:
\(\frac{3.13}{1.565}=\frac{3}{1.5}\)
Yes, the triangles shown are similar. ∆ ABC ~ ∆ DEF by SAS because m∠B = m∠E, and the adjacent sides are proportional.

Exercise 7.
Are the triangles shown below similar? Explain. If the triangles are similar, write the similarity statement.

Answer:
Yes, the triangles shown are similar. ∆ ABC ~ ∆ ADE by AA because m∠ADE = m∠ABC, and both triangles share LA.

Exercise 8.
Are the triangles shown below similar? Explain, lithe triangles are similar, write the similarity statement.

Answer:
\(\frac{5}{3} \neq \frac{3}{1}\)
There is no information about the angle measures other than the right angle, so we cannot use AA to conclude the triangles are similar. We only have information about two of the three side lengths for each triangle, so we cannot use SSS to conclude they are similar. If the triangles are similar, we would have to use the SAS criterion, and since the side lengths are not proportional, the triangles shown are not similar. (Note that students could also utilize the Pythagorean theorem to determine the lengths of the hypotenuses and then use SSS similarity criterion to answer the question.)

Exercise 9.
Are the triangles shown below similar? Explain. If the triangles are similar, write the similarity statement.

Answer:
\(\frac{3.6}{2.7}=\frac{7.28}{5.46}=\frac{10}{7.5}\)
Yes, the triangles are similar. ∆ PQR ~ ∆ XYZ by SSS.

Exercise 10.
Are the triangles shown below similar? Explain. If the triangles are similar, write the similarity statement.

Answer:
\(\frac{2.24}{4.48}=\frac{3.16}{6.32}\)
Yes, the triangles are similar. ∆ ABE ~ ∆ DCE by SAS because m∠AEB = m∠DEC (vertical angles are congruent), and the sides adjacent to those angles are proportional.

Eureka Math Geometry Module 2 Lesson 17 Problem Set Answer Key

Question 1.
For parts (a) through (d) below, state which of the three triangles, if any, are similar and why.

Answer:
Triangles B and C are similar because they share three pairs of corresponding sides that are in the same ratio.
\(\frac{4}{8}=\frac{6}{12}=\frac{8}{16}=\frac{1}{2}\)
Triangles A and B are not similar because the ratios of their corresponding sides are not in the same ratio.
\(\frac{6}{16} \neq \frac{4}{12}\)
Further, if triangle A is not similar to triangle B, then triangle A is not similar to triangle C

b.

Answer:
Triangles A and B are not similar because their corresponding sides are not oil in the same ratio. Two pairs of corresponding sides are proportional, but the third pair of corresponding sides is not.
\(\frac{3}{6} \neq \frac{5}{7.5}=\frac{6}{9}\)
Triangles B and C are not similar because their corresponding sides are not in the same ratio.
\(\frac{6}{6} \neq \frac{7}{7.5} \neq \frac{8}{9}\)
Triangles A and C are not similar because their corresponding sides are not in the same ratio.
\(\frac{3}{6} \neq \frac{5}{7} \neq \frac{6}{8}\)

c.

Answer:
Triangles B and D are the only similar triangles because they have the same angle measures. Using the angle sum of a triangle, both triangles B and D have angles of 75°, 60°, and 45°.

d.

Answer:
Triangles A and B are similar because they have two pairs of corresponding sides that are in the same ratio, and their included angles are equal measures. Triangle C cannot be shown to be similar because, even though it has two sides that are the same length as two sides of triangle A, the 70° angle in triangle C is not the included angle and, therefore, does not correspond to the 70° angle in triangle A.

Question 2.
For each given pair of triangles, determine If the triangles are similar or not, and provide your reasoning. If the triangles are similar, write a similarity statement relating the triangles.
a.

Answer:
The triangles are similar because, using the angle sum of a triangle, each triangle has angle measures of 50°, 60°, and 70°. Therefore, ∆ ABC ~ ∆ TSR.

b.

Answer:
The triangles are not similar, because the ratios of corresponding sides are not all in proportion.
\(\frac{A B}{D E}=\frac{B C}{E F}\) = 2; however, \(\frac{A C}{D F}=\frac{7}{4}\) ≠ 2.

c.

Answer:
∆ ABC ~ ∆ FDE by the SSS criterion for showing similar triangles because the ratio of all pairs of corresponding sides \(\frac{A C}{F E}=\frac{A B}{F D}=\frac{B C}{D E}\) = 2.

d.

Answer:
\(\frac{A B}{D E}=\frac{B C}{D F}=\frac{4}{5}\), and included angles B and D are both 36° and, therefore, congruent, so ∆ ABC ~ ∆ EDF by the SAS criterion for showing similar triangles.

Question 3.
For each pair of similar triangles below, determine the unknown lengths of the sides labeled with letters.
a.

Answer:
The ratios of corresponding sides must be equal, so \(\frac{5}{n}=\frac{3 \frac{3}{4}}{9 \frac{3}{8}}\), giving n = 12\(\frac{1}{2}\). Likewise, \(\frac{m}{7 \frac{1}{2}}=\frac{3 \frac{3}{4}}{9 \frac{3}{8}}\), giving m = 3

b.

Answer:
The ratios of corresponding sides must be equal, so = \(\frac{8}{6}=\frac{s}{6 \frac{3}{4}}\), giving s = 9.
Likewise, = \(\frac{8}{6}=\frac{7}{t}\), giving t = 5\(\frac{1}{4}\).

Question 4.
Given that \(\overline{A D}\) and \(\overline{B C}\) intersect at E and \(\overline{A D}\) || \(\overline{B C}\), show that ∆ ABE ~ ∆ DCE.

Answer:
∠AEB and ∠DEC are vertically opposite angles and,
therefore, congruent. ∠A ≅ ∠D by alt. int. ∠’s, \(\overline{A D}\) || \(\overline{B C}\). Therefore, ∆ ABE ~ ∆ DCE by the AA criterion for showing similar triangles.

Question 5.
Given BE = 11, EA = 11, BD = 7, and DC = 7, show that ∆ BED ~ t BAC.

Answer:
Both triangles share angle B, and by the reflexive property, ∠B ≅ ∠B. BA = BE + EA, so BA = 22, and BC = BD + DC, so BC = 14. The ratios of corresponding sides \(\frac{B E}{B A}=\frac{B D}{B C}=\frac{1}{2}\). Therefore, ∆ BED ~ ∆ BAC by the SAS criterion for triangle similarity.

Question 6.
Given the diagram below, X is on \(\overline{R S}\) and Y is on \(\overline{R T}\), XS = 2, XY = 6, ST = 9, and YT = 4.

a. Show that ∆ RXY ~ ∆ RST.
Answer:
The diagram shows ∠RST ≅ ∠RXY. Both triangle RXY and RST share angle R, and by the reflexive property, ∠R ≅ ∠R, so ∆ RXY ~ ∆ RST by the AA criterion show triangle similarity.

b. Find RX and RY.
Answer:
Since the triangles are similar, their corresponding sides must be in the same ratio.

Question 7.
One triangle has a 120° angle, and a second triangle has a 65° angle. Is it possible that the two triangles are similar? Explain why or why not.
Answer:
No The triangles cannot be similar because in the first triangle, the sum of the remaining angles is 60°, which mean that it is not possible for the triangle to have a 65° angle. For the triangles to be similar, both triangles would have to have angles measuring 120° and 65°, but this is impossible due to the angle sum of a triangle.

Question 8.
A right triangle has a leg that is 12 cm, and another right triangle has a leg that is 6 cm. Can you tell whether the two triangles are similar? If so, explain why. If not, what other information would be needed to show they are similar?
Answer:
The two triangles may or may not be similar. There is not enough information to make this claim. If the second leg of the first triangle is twice the length of the second leg of the first triangle, then the triangles are similar by SAS criterion for showing similar triangles.

Question 9.
Given the diagram below, JH = 7. 5, HK = 6, and KL = 9, is there a pair of similar triangles? If so, write a similarity statement, and explain why. If not, explain your reasoning.
Answer:
∆ LKJ ~ ∆ HKL by the SAS criterion for showing triangle similarity. Both triangles share ∠K, and by the reflexive property, ∠K ≅ ∠K. Furthermore, \(\frac{L K}{J K}=\frac{H K}{L K}=\frac{2}{3}\), giving two pairs of corresponding sides in the same ratio and included angles of the same size.

Eureka Math Geometry 2 Module 2 Lesson 17 Exit Ticket Answer Key

Question 1.
Given ∆ ABC and ∆ MLN in the diagram below, determine if the triangles are similar. If so, write a similarity statement, and state the criterion used to support your claim. B

Answer:
In comparing the ratios of sides between figures, I found that \(\frac{A B}{M L}=\frac{C B}{N L}\) because the cross-products of the proportion \(\frac{8}{3}=\frac{12 \frac{2}{3}}{4 \frac{3}{4}}\) are both 38. We are given that ∠L ≅ ∠B. Therefore, ∆ ABC ~ ∆ MLN by the SAS criterion for proving similar triangles.

Question 2.
Given ∆ DEF and ∆ EGF in the diagram below, determine if the triangles are similar. If so, write a similarity statement, and state the criterion used to support your claim.

By comparison, if the triangles are in fact similar, then the longest sides of each triangle will correspond, and likewise the shortest sides will correspond. The corresponding sides from each triangle are proportional since
\(\frac{5}{3 \frac{1}{8}}=\frac{4}{2 \frac{1}{2}}=\frac{6 \frac{2}{5}}{4}=\frac{8}{5}\), so \(\frac{D E}{E G}=\frac{E F}{G F}=\frac{D F}{E F}\)
Therefore, by the SSS criterion for showing triangle similarity, ∆ DEF ~ ∆ EGF.

Engage NY Eureka Math Geometry Module 2 Lesson 22 Answer Key

Eureka Math Geometry Module 2 Lesson 22 Exercise Answer Key

Simplify as much as possible.

Exercise 1.
√17² =
Answer:
√17² = 17

Exercise 2.
√5¹⁰ =
Answer:
\(\sqrt{5^{10}}=\sqrt{5^{2}} \times \sqrt{5^{2}} \times \sqrt{5^{2}} \times \sqrt{5^{2}} \times \sqrt{5^{2}}\)
= 5 × 5 × 5 × 5 × 5
= 5

Exercise 3.
√4x⁴ =
Answer:
\(\sqrt{4 x^{4}}=\sqrt{4} \times \sqrt{x^{2}} \times \sqrt{x^{2}}\)
= 2 × x × x
= 2|x|²

Exercise 4.
Complete parts (a) through (c). radical.
a. Compare the value of √36 to the value of √9 × √4.
Answer:
The value of the two expressions is equal. The square root of 36 is 6, and the product of the square roots of 9 and 4 is also 6.

b. Make a conjecture about the validity of the following statement: For nonnegative real numbers a and b, √ab = √a · √b. Explain.
Answer:
Answers will vary. Students should say that the statement √ab = √a · √b is valid because of the problem that was just completed: √36 = √9 × √4 = 6.

c. Does your conjecture hold true for a = – 4 and b = – 9?
Answer:
No. The conjecture is not true when the numbers are negative because we cannot take the square root of a negative number. \(\sqrt{(-4)(-9)}=\sqrt{36}\) = 6, but we cannot calculate \(\sqrt{-4} \times \sqrt{-9}\) in order to compare.

Exercise 5.
Complete parts (a) through (c).
a. Compare the value of \(\sqrt{\frac{100}{25}}\) to the value of \(\frac{\sqrt{100}}{\sqrt{25}}\).
Answer:
The value of the two expressions is equal. The fraction \(\frac{100}{25}\) simplifies to 4, and the square root of 4 is 2. The square root of 100 divided by the square root of 25 is equal to \(\frac{10}{5}\), which is equal to 2.

b. Make a conjecture about the validity of the following statement: For nonnegative real numbers a and b, when b ≠ 0, \(\sqrt{\frac{a}{b}}=\frac{\sqrt{a}}{\sqrt{b}}\) . Explain.
Answer:
Answers will vary. Students should say that the statement \(\sqrt{\frac{a}{b}}=\frac{\sqrt{a}}{\sqrt{b}}\) is valid because of the problem that was just completed: \(\sqrt{\frac{100}{25}}=\frac{\sqrt{100}}{\sqrt{25}}\) = 2.

c. Does your conjecture hold true for a = – 100 and b = – 25?
Answer:
No. The conjecture is not true when the numbers are negative because we cannot take the square root of a negative number. \(\sqrt{\frac{-100}{-25}}\) = √4 = 2, but we cannot calculate \(\frac{\sqrt{-100}}{\sqrt{-25}}\) in order to compare.

Simplify each expression as much as possible, and rationalize denominators when applicable.

Exercise 6.
√72 =
Answer:
√72 = √36 · √2
= 6√2

Exercise 7.
\(\sqrt{\frac{17}{25}}\) =
Answer:
\(\sqrt{\frac{17}{25}}=\frac{\sqrt{17}}{\sqrt{25}}\)
= \(\frac{\sqrt{17}}{5}\)

Exercise 8.
√32x =
Answer:
√32x = √16 √2x
= 4√2x

Exercise 9.
\(\sqrt{\frac{1}{3}}\)
Answer:

Exercise 10.
√54x² =
Answer:
√54x²= √9√6√x²
= 3|x|√6

Exercise 11.
\(\frac{\sqrt{36}}{\sqrt{18}}\) =
Answer:
\(\frac{\sqrt{36}}{\sqrt{18}}=\sqrt{\frac{36}{18}}\)
= √2

Exercise 12.
\(\sqrt{\frac{4}{x^{4}}}\) =
Answer:
\(\sqrt{\frac{4}{x^{4}}}=\frac{\sqrt{4}}{\sqrt{x^{4}}}\)
= \(\frac{2}{x^{2}}\)

Exercise 13.
\(\frac{4 x}{\sqrt{64 x^{2}}}\) =
Answer:
\(\frac{4 x}{\sqrt{64 x^{2}}}=\frac{4 x}{8 x}\)
= \(\frac{1}{2}\)

Exercise 14.
\(\frac{5}{\sqrt{x^{7}}}\) =
Answer:

Exercise 15.
\(\sqrt{\frac{x^{5}}{2}}\)
Answer:

Exercise 16.
\(\frac{\sqrt{18 x}}{3 \sqrt{x^{5}}}\) =
Answer:

Exercise 17.
The captain of a ship recorded the ship’s coordinates, then sailed north and then west, and then recorded the new coordinates. The coordinates were used to calculate the distance they traveled, √578 km. When the captain asked how far they traveled, the navigator said, “About 24 km.” Is the navigator correct? Under what conditions might he need to be more precise in his answer?
Answer:
Sample student responses:
The number √578 is close to the perfect square √576. The perfect square √576 = 24; therefore, the navigator is correct in his estimate of the distance traveled.

When the number √578 is simplified, the result is 17√2. The number 578 has factors of 289 and 2. Then:
√578 = √289 √2
= 17√2
= 24.04163…
≈ 24
Yes, the navigator is correct in his estimate of the distance traveled.

A more precise answer may be needed if the captain were looking for a particular location, such as the location of a shipwreck or buried treasure.

Eureka Math Geometry Module 2 Lesson 22 Problem Set Answer Key

Question 1.
√6 · √60 =
Answer:
√6 · √60 = √6 · √6 · √10
= 6√10

Question 2.
√108 =
Answer:
√108 = √9 · √4 · √3
= 3 · 2√3
= 6√3

Question 3.
Pablo found the length of the hypotenuse of a right triangle to be √45. Can the length be simplified? Explain.
Answer:
√45 = √9√5
= 3√5
Yes, the length can be simplified because the number 45 has a factor that is a perfect square.

Question 4.
√12x⁴ =
Answer:
√12x⁴ = √4√3√x⁴
= 2x²√3

Question 5.
Sarahi found the distance between two points on a coordinate plane to be Can this answer be simplified? Explain.
Answer:
The number 74 can be factored, but none of the factors are perfect squares, which are necessary to simplify. Therefore, √74 cannot be simplified.

Question 6.
√16x³ =
Answer:
√16x³ = √16√x²√x
= 4x√x

Question 7.
\(\frac{\sqrt{27}}{\sqrt{3}}\) =
Answer:
\(\frac{\sqrt{27}}{\sqrt{3}}=\sqrt{\frac{27}{3}}\)
= √9
= 3

Question 8.
Nazem and Joff rey are arguing about who got the right answer. Nazem says the answer is \(\frac{1}{\sqrt{3}}\) and Joffrey says the answer is \(\frac{\sqrt{3}}{3}\). Show and explain that their answers are equivalent.
Answer:
\(\frac{1}{\sqrt{3}}=\frac{1}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}}\)
= \(\frac{\sqrt{3}}{3}\)
If Nazem were to rationalize the denominator in his answer, he would see that it is equal to Joffrey’s answer.

Question 9.
\(\sqrt{\frac{5}{8}}\) =
Answer:

Question 10.
Determine the area of a square with side length 2√7 in.
Answer:
A = (2√7)²
= 2²(√7)²
= 4(7)
= 28
The area of the square is 28 in².

Question 11.
Determine the exact area of the shaded region shown below.

Answer:
Let r be the length of the radius.
By special triangles or the Pythagorean theorem, r = 5√2.
The area of the rectangle containing the shaded region is
A = 2(5√2) (5√2) = 2(25)(2) = 100.
The sum of the two quarter circles in the rectangular region is
A = \(\frac{1}{2}\)π(5√2)²
= \(\frac{1}{2}\) π(25)(2)
= 25π.
The area of the shaded region is 100 – 25π square units.

Question 12.
Determine the exact area of the shaded region shown to the right.

Answer:
The radius of each quarter circle is \(\frac{1}{2}\)(√20) = \(\frac{1}{2}\) (2√5) = √5.
The sum of the area of the four circular regions is A = π(√5)² = 5π.
The area of the square is A = (√20)² = 20.
The area of the shaded region is 20 – 5π square units.

Question 13.
Calculate the area of the triangle to the right.

Answer:

The area of the triangle is √2 square units.

Question 14.
\(\frac{\sqrt{2 x^{3}} \cdot \sqrt{8 x}}{\sqrt{x^{3}}}\) =
Answer:

Question 15.
Prove Rule 2 for square roots: \(\sqrt{\frac{a}{b}}=\frac{\sqrt{a}}{\sqrt{b}}\) (a ≥ 0, b > 0)
Let p be the nonnegative number so that p² = a, and let q be the nonnegative number so that q² = b. Then,
Answer:

Eureka Math Geometry Module 2 Lesson 22 Exit Ticket Answer Key

Question 1.
√243 =
Answer:
√243 = √81 · √3
= 9√3

Question 2.
\(\sqrt{\frac{7}{5}}\)
Answer:

Question 3.
Teja missed class today. Explain to her how to write the length of the hypotenuse in simplest radical form.

Answer:
Use the Pythagorean theorem to determine the length of the hypotenuse, C:
5² + 13² = c²
25 + 169 = c²
194 = c²
√194 = c
To simplify the square root, rewrite the radicand as a product of its factors. The goal is to find a factor that is a perfect square and can then be simplified. There are no perfect square factors of the radic and; therefore, the length of the hypotenuse in simplest radical form is √194.

Engage NY Eureka Math Geometry Module 2 Lesson 23 Answer Key

Eureka Math Geometry Module 2 Lesson 23 Example Answer Key

Example 1.
Explain how the expression 8. 3√2 + 7. 9√2 can be simplified using the distributive property.
Answer:
Each term of the expression has a common factor, √2. For that reason, the distributive property can be applied.
8. 3√2 + 7. 9√2 = (8.3 + 7. 9)√2 By the distributive property
= 16.2√2

Explain how the expression 11√7 – 6√7 + 3√2 can be simplified using the distributive property.
Answer:
The expression can be simplified because the first two terms contain the expression √7. Using the distributive property,
we get the following:
11√7 – 6√7 + 3√2 = (11 – 6)√7 + 3√2 By the distributive property
= 5√7 + 3√2

Example 2.
Explain how the expression 19√2 + 2√8 can be simplified using the distributive property.
Answer:
The expression can be simplified, but first the term 2√8 must be rewritten.
19√2 + 2√8 = 19√2 + 2√4 · √2 By Rule 1
= 19√2 + 2 · 2√2
= 19√2 + 4√2
= (19 + 4)√2 By the distributive property
= 23√2

Can the expression 19√2 + 2√8 be simplified using the distributive property?
Answer:
No, the expression cannot be simplified because neither term can be rewritten in a way that the distributive property could be applied.

Eureka Math Geometry Module 2 Lesson 23 Exercise Answer Key

Simplify each expression as much as possible.

Exercise 1.
√32 =
Answer:
√32 = √16√2
= 4√2

Exercise 2.
√45
Answer:
√45 = √9√5
= 3√5

Exercise 3.
√300 =
Answer:
√300 = √100√3
= 10√3

Exercise 4.
The triangle shown below has a perimeter of 6. 5√2 units. Make a conjecture about how this answer was reached.

Answer:
It appears that when all three sides of the triangle were added, the numbers that preceded the square roots were the only numbers that were added:
3 + 2 + 1.5 = 6.5. The √2 shown as part of each length remained √2.

Exercise 5.
The sides of a triangle are 4√3, √12, and √75. Make a conjecture about how to determine the perimeter of this
triangle.
Answers will vary. The goal is for students to realize that √12 and √75 can be rewritten so that each has a factor of √3, which then strongly resembles Exercise 4. By rewriting each side length as a multiple of √3, we get Some students may answer incorrectly by adding 3 + 12 + 75. Show that this is incorrect using a simpler example.
√9 + √16 ≠ √25

Exercise 6.
Circle the expressions that can be simplified using the distributive property. Be prepared to explain your choices.

Answer:
The expressions that can be simplified using the distributive property are noted in red.

Eureka Math Geometry Module 2 Lesson 23 Problem Set Answer Key

Question 1.
18√5 – 12√5 =
Answer:
18√5 – 12√5 = (18 – 12)√5
= 6√5

Question 2.
√24 + 4√54 =
Answer:
= √24 + 4√54 = √4 · √6 + 4 · √9 · √6
= 2√6 + 4 · 3√6
= (2 + 12)√6
= 14√6

Question 3.
2√7 + 4√63 =
Answer:
2√7 + 4√63 = 2√7 + 4√9√7
= 2√7 + 4(3)√7
= (2 + 12)√7
= 14√7

Question 4.
What is the perimeter of the triangle shown below?

Answer:
2√2 + √12 + √32 = 2√2 + √4 · √3 + √16 · √2
= 2√2 + 2√3 + 4√2
= (2 + 4)√2 + 2√3
= 6√2 + 2√3
The perimeter of the triangle is 6√2 + 2√3 units.

Question 5.
Determine the area and perimeter of the triangle shown. Simplify as much as possible.

The perimeter of the triangle is
√24 + 5√6 + √174 = √4√6 + 5√6 + √174
= 2√6 + 5√6 + √174
= (2 + 5)√6 + √174
= 7√6 + √174.
The area of the triangle is
\(\frac{\sqrt{24}(5 \sqrt{6})}{2}=\frac{2 \sqrt{6}(5 \sqrt{6})}{2}=\frac{60}{2}\) = 30.
The perimeter is 7√6 + √174 units, and the area Is 30 square units.

Question 6.
Determine the area and perimeter of the rectangle shown. Simplify as much as possible.

Answer:
The perimeter of the rectangle is
11√3 + 11√3 + √75 + √75 = 2(11√3) + 2(√25√3)
= 22√3 + 10√3
= (22 + 10)√3
= 32.
The area of the rectangle is
11√3(5√3) = 55(3)
= 165.
The perimeter is 32√3 units, and the area is 165 square units.

Question 7.
Determine the area and perimeter of the triangle shown. Simplify as much as possible.

Answer:
The perimeter of the triangle is
8√3 + 8√3 + √384 = (8 + 8)√3 + √384
= 16√3 + √384
= 16√3 + √64√6
= 16√3 + 8√6.
The area of the triangle is
\(\frac{(8 \sqrt{3})^{2}}{2}=\frac{8^{2}(\sqrt{3})^{2}}{2}\)
= \(\frac{64(3)}{2}\)
= 32(3)
= 96.
The perimeter of the triangle is 16√3 + 8√6 units, and the area of the triangle is 96 square units.

Question 8.
Determine the area and perimeter of the triangle shown. Simplify as much as possible.

Answer:
The perimeter of the triangle is
2x + x + x√3 = 3x + x√3.
The area of the triangle is
\(\frac{x(x \sqrt{3})}{2}=\frac{x^{2} \sqrt{3}}{2}\)
The perimeter is 3x + x√3 units, and the area is \(\frac{x^{2} \sqrt{3}}{2}\) square units.

Question 9.
The area of the rectangle shown in the diagram below is 160 square units. Determine the area and perimeter of the shaded triangle. Write your answers in simplest radical form, and then approximate to the nearest tenth.

Answer:
The length of the rectangle is 8x, and the width is 4x. Using the given area of the rectangle:
Area = length × width
160 = 8x · 4x
160 = 32x²
5 = x²
Area_rectangle = A₁ + A₂ + A₃ + A₄

160 = 30 + 40 + 20 + A₄
A₄ = 70
The area of the shaded triangle in the diagram is 70 square units.
The perimeter of the shaded triangle requires use of the Pythagorean theorem to find the hypotenuses of right triangles 1,2, and 3. Let h₁, h₂, and h₃ represent the lengths of the hypotenuses of triangles 1, 2, and 3, respectively.

Perimeter = C₁ + C₂ + C₃
Perimeter = 5√5 + 4√10 + 5√13
The perimeter of the shaded triangle is approximately 41.9 units.

Question 10.
Parallelogram ABCD has an area of 9 square units. DC = 3√3 and G and H are midpoints of \(\overline{D E}\) and \(\overline{C E}\), respectively. Find the area of the shaded region. Write your answer in simplest radical form.

Answer:
Using the area of a parallelogram:
Area(ABCD) = bh
9√3 = 3√3 · h
3 = h
The height of the parallelogram is 3.

The area of the shaded region is the sum of the areas of ∆ EGH and ∆ FGH.

The given points G and H are midpoints of \(\overline{D E}\) and \(\overline{C E}\); therefore, by the triangle side splitter theorem, [/latex] and \(\overline{G H}\) must be parallel to [/latex] and \(\overline{D C}\), and thus, also parallel to [/latex] and \(\overline{A B}\). Furthermore,
GH = \(\frac{1}{2}\)CD = \(\frac{1}{2}\)AB = \(\frac{3}{2}\)√3.

∆ EGH ~ ∆ EDC by AA ~ criterion with a scale factor of \(\frac{1}{2}\). The areas of scale drawings are related by the square of the scale factor; therefore, Area(∆ EGH) = \(\left(\frac{1}{2}\right)^{2}\) Area(∆ EDC).

Eureka Math Geometry Module 2 Lesson 23 Exit Ticket Answer Key

Question 1.
Simplify 5√11 – 17√11.
Answer:
5√11 – 17√11 = (5 – 17)√11
= – 12√11

Question 2.
Simplify √8 + 5√2.
Answer:
√8 + 5√2 = √4√2 + 5√2
= 2√2 + 5√2
= (2 + 5)√2
= 7√2

Question 3.
Write a radical addition or subtraction problem that cannot be simplified, and explain why it cannot be simplified.
Answer:
Answers will vary. Students should state that their expression cannot be simplified because one or both terms cannot be rewritten so that each has a common factor. Therefore, the distributive property cannot be applied.

Engage NY Eureka Math Geometry Module 2 Lesson 28 Answer Key

Eureka Math Geometry Module 2 Lesson 28 Example Answer Key

Example 1.
Find the value of a and b.

Answer:
→ Now that we can calculate the sine and cosine of a given angle using a calculator, we can use the decimal value of the ratio to determine the unknown side length of a triangle.

Consider the following triangle.
→ What can we do to find the length of side a?
We can find the sin 40 or cos 50.

→ Let’s begin by using the sin 40. We expect sin 40 = \(\frac{a}{26}\). Why?
By definition of sine; sin θ = \(\frac{\text { opp }}{\text { hyp }}\).

→ To calculate the length of a, we must determine the value of 26 sin 40 because a = 26 sin 40. We will round our answer to two decimal places.

→ Using the decimal approximation of sin 40 ≈ 0.642 8, we can write
26(0.6428) ≈ a
16.71 ≈ a

→ Now let’s use cos 50, which is approximately 0.6428. What do you expect the result to be? Explain.
I expect the result to be the same. Since the approximation of sin 40 is equal to the approximation of cos 50, the computation should be the same.

Note that students may say that sin 40 = cos 50. Ensure that students know that once decimal approximations are used in place of the functions, they are no longer looking at two quantities that are equal because the decimals are approximations. To this end, ask students to recall that in Exercise1 they were only taking the first four decimal digits of the number; that is, they are using approximations of those values. Therefore, they cannot explicitly claim that sin 40 = cos 50, rather that their approximations are extremely close in value to one another.

If necessary, show the computation below that verifies the claim made above.
cos 50 = \(\frac{a}{26}\)
26 cos 50 = a
26(0.6428) ≈ a
16.71 ≈ a

→ Now, calculate the length of side b.
Side b can be determined using sin 50 or cos 40.
26(0.7660) ≈ b
19.92 ≈ b

→ Could we have used another method to determine the length of side b?
Yes. Because this is a right triangle and two sides are known, we could use the Pythagorean theorem to determine the length of the third side.

The points below are to make clear that the calculator gives approximations of the ratios we desire when using trigonometric functions.

→ When we use a calculator to compute, what we get is a decimal approximation of the ratio \(\frac{a}{26}\). Our calculators are programmed to know which number a is needed, relative to 26, so that the value of the ratio \(\frac{a}{26}\) is equal to the value of sin 40. For example, sin 40 = \(\frac{a}{26}\) and sin 40 ≈ 0.6428. Our calculators give us the number a that, when divided by 26, is closest to the actual value of sin 40.

→ Here is a simpler example illustrating this fact. Consider a right triangle with an acute angle of 300 and hypotenuse length of 9 units. Then, sin 30 = \(\frac{a}{9}\). We know that sin 30 = \(\frac{1}{2}\) = 0.5. What our calculators do is find the number a so that \(\frac{a}{9}=\frac{1}{2}\) = 0.5, which is a = 4.5.

Example 2.
Johanna borrowed some tools from a friend so that she could precisely, but not exactly, measure the corner space in her backyard to plant some vegetables. She wants to build a fence to prevent her dog from digging up the seeds that she plants. Johanna returned the tools to her friend before making the most Important measurement: the one that would give the length of the fence!

Johanna decided that she could just use the Pythagorean theorem to find the length of the fence she would need. Is the Pythagorean theorem applicable in this situation? Explain.

Answer:
No The corner of her backyard is not a 90° angle; therefore, the Pythagorean theorem cannot be applied in this situation. The Pythagorean theorem will, however, provide an approximation since the given angle has a measure that is close to 90°.

→ What can we do to help Johanna figure out the length of fence she needs?
I Provide time for students to discuss this in pairs or small groups. Allow them to make sense of the problem and persevere in solving it. It may be necessary to guide their thinking using the prompts below.
→ If we dropped an altitude from the angle with measure 950, could that help? How?
→ Would we be able to use the Pythagorean theorem now? Explain.
→ If we denote the side opposite the 95° angle as x and y, as shown, can we use what we know about sine and cosine? Explain.

The missing side length is equal to x + y. The length x is equal to 100 cos 35, and the length y is equal to 74.875 cos 50. Therefore, the length of
x + y = 100 cos 35 + 74.875 cos 50 ≈ 81.92 + 48.12872 130.05.
Note: The Pythagorean theorem provides a reasonable approximation of 124.93.

Eureka Math Geometry Module 2 Lesson 28 Exercise Answer Key

Exercise 1.
a. The bus drops you off at the corner of H Street and 1^st Street, approximately 300 ft. from school. You plan to walk to your friend Janneth’s house after school to work on a project. Approximately how many feet will you have to walk from school to Janneth’s house? Round your answer to the nearest foot. (Hint: Use the ratios you developed in Lesson 25.)

Answer:
Let x represent the distance from school to Janneth’s house.
sin θ = \(\frac{\text { opp }}{\text { hyp }}\), then sin 41 = \(\frac{5.3}{8}\). Then, \(\frac{300}{x}\) = \(\frac{5.3}{8}\) and
x = 452.8301887…
The distance I will have to walk from school to Janneth’s house is approximately 453 ft.

b. In real life, it is unlikely that you would calculate the distance between school and Janneth’s house in this manner. Describe a similar situation in which you might actually want to determine the distance between two points using a trigonometric ratio.
Answer:
Accept any reasonable responses. Some may include needing to calculate the distance to determine if a vehicle has enough fuel to make the trip or the need to determine the length prior to attempting the walk because a friend is on crutches and cannot easily get from one location to the next when the distance is too long.

Exercise 2.
Use a calculator to find the sine and cosine of θ. Give your answer rounded to the ten-thousandth place.

Answer:

Exercise 3.
What do you notice about the numbers in the row sin θ compared with the numbers in the row cos θ?
Answer:
The numbers are the same but reversed in order.

Exercise 4.
Provide an explanation for what you noticed in Exercise 2.
Answer:
The pattern exists because the sine and cosine of complementary angles are equal.

Exercise 5.
A shipmate set a boat to sail exactly 27° NE from the dock. After traveling 120 miles, the shipmate realized he had misunderstood the instructions from the captain; he was supposed to set sail going directly east!

a. How many miles will the shipmate have to travel directly south before he is directly east of the dock? Round your answer to the nearest mile.
Answer:
Let S represent the distance they traveled directly south.
sin 27 = \(\frac{S}{120}\)
120 sin 27 = S
54.47885997… = S
He traveled approximately 54 mi. south.

b. How many extra miles does the shipmate travel by going the wrong direction compared to going directly east? Round your answer to the nearest mile.
Answer:
Solutions may vary. Some students may use the Pythagorean theorem while others may use the cosine function. Both are acceptable strategies. If students use different strategies, make sure to share them with the class, and discuss the benefits of each.

Let E represent the distance the boat is when it is directly east of the dock.
cos 27 = \(\frac{E}{120}\)
120 cos 27 = E
106.9207829… = E
107 ≈ E
The total distance traveled by the boat is 120 + 54 = 174. They ended up exactly 107 miles east of the dock. 174 – 107 = 67,so they traveled an extra 67 miles.

Exercise 6.
The measurements of the triangle shown below are rounded to the nearest hundredth. Calculate the missing side length to the nearest hundredth.

Answer:
Drop an altitude from the angle that measures 99°.

Then, the length of the missing side is x + y, which can be found by
4.04 cos 39 + 3.85 cos 42 ≈ 3.139669 + 2.861107 = 6.000776 ≈ 6.00.

Eureka Math Geometry Module 2 Lesson 28 Problem Set Answer Key

Question 1.
Given right triangle GHI, with right angle at H, GH = 12. 2, and m∠G = 28°, find the measures of the remaining sides and angle to the nearest tenth.

Answer:
cos 28 = \(\frac{12.2}{G I}\)
GI = \(\frac{12.2}{\cos 28}\)
GI ≈ 13.8

tan 28 = \(\frac{\text { IH }}{12.2}\)
IH = 12.2 tan 28
IH ≈ 6.5

28° + m∠I = 90°
m∠I = 62°

Question 2.
The Occupational Safety and Health Administration (OSHA) provides standards for safety at the workplace. A ladder is leaned against a vertical wall according to OSHA standards and forms an angle of approximately 75° with the floor.

a. If the ladder is 25 ft. long, what is the distance from the base of the ladder to the base of the wall?
Answer:
Let b represent the distance of the base of the ladder from the wall in feet.
b = 25(cos 75)
b ≈ 6.5
The base of the ladder is approximately 6 ft. 6 in. from the wall.

b. How high on the wall does the ladder make contact?
Answer:
Let h represent the height on the wall where the ladder makes contact in feet.
h = 25(sin75)
h ≈ 24.1
The ladder contacts the wall just over 24 ft. above the ground.

c. Describe how to safely set a ladder according to OSHA standards without using a protractor.
Answer:
Answers will vary. Possible description:
The horizontal distance of the base of the ladder to the point of contact of the ladder should be approximately \(\frac{1}{4}\) of the length of the ladder.

Question 3.
A regular pentagon with side lengths of 14 cm is inscribed in a circle. What is the radius of the circle?

Answer:
Draw radii from center C of the circle to two consecutive vertices of the pentagon, A and B, and draw an altitude from the center C to D on \(\overline{A B}\).

The interior angles of a regular pentagon have measure of 108°, and \(\overline{A C}\) and \(\overline{B C}\) bisect the interior angles at A and B.
AD = BD = 7 cm

Let x represent the lengths of \(\overline{A C}\) in centimeters.

Using cosine, cos 54 = \(\frac{7}{x}\) and thus:
x = \(\frac{7}{\cos 54}\)
x ≈ 11.9.
\(\overline{A C}\) is a radius of the circle and has a length of approximately 11.9 cm.

Question 4.
The circular frame of a Ferris wheel is suspended so that it sits 4 ft. above the ground and has a radius of 30 ft. A segment joins center C to point S on the circle. If \(\overline{C S}\) makes an angle of 48° with the horizon, what is the distance of point S to the ground?

Answer:
Note to teacher: There are two correct answers to this problem since the segment can make an angle of 48° above or below the horizon in four distinct locations, providing two different heights above the ground.

There are four locations at which the segment makes an angle of 48° with the horizon. In each case, \(\overline{C S}\) is the hypotenuse of a right triangle with acute angles with measures of 48° and 42°.

Let d represent the distance in feet from point S to the horizon (applies to each case):
sin 48 = \(\frac{d}{30}\)
30 (sin 48) = d
22.3 ≈ d

The center of the Ferris wheel is 34 ft. above the ground; therefore, the distance from points S₁ and S₄ to the ground in feet is
34 – 22.3 = 11.7.
Points S₂ and S₃ are approximately 22.3 ft. above the center of the Ferris wheel, so the distance from S₂ and S₃ to the ground in feet is
34 + 22.3 = 56.3.

When \(\overline{C S}\) forms a 48° angle with the horizon, point S is either approximately 11.7 ft. above the ground or approximately 56.3 ft. above the ground.

Question 5.
Tim is a contractor who is designing a wheelchair ramp for handicapped access to a business. According to the Americans with Disabilities Act (ADA), the maximum slope allowed for a public wheelchair ramp forms an angle of approximately 4. 76° to level ground. The length of a ramp’s surface cannot exceed 30 ft. without including a flat 5 ft. × 5 ft. platform (minimum dimensions) on which a person can rest, and such a platform must be included at the bottom and top of any ramp.

Tim designs a ramp that forms an angle of 4° to the level ground to reach the entrance of the building. The entrance of the building is 2 ft. 9 in. above the ground. Let x and y as shown in Tim’s initial design below be the indicated distances in feet.

a. Assuming that the ground in front of the building’s entrance is flat, use Tim’s measurements and the ADA requirements to complete and/or revise his wheelchair ramp design.

Answer:
Note to teacher: Student designs will vary; however, the length of the ramp’s surface is greater than 30 ft., which requires at least one resting platform along the ramp. Furthermore, Tim’s design does not include a platform at the top of the ramp as required by the guidelines, rendering his design incorrect.

Possible student solution:
2 ft. 9 in. = 2.75ft.
Using tangent, tan 4 = \(\frac{2.75}{x}\), and thus
x = \(\frac{2.75}{\tan 4}\)
x ≈ 39.33.

The ramp begins approximately 39 ft. 4 in. from the building; thus, the in length. The hypotenuse of the triangle represents the sloped surface the legs. Tim’s design will not meet the ADA guidelines because it does the ramp’s slope, nor does it include a platform at the top of the ramp. ground. The student’s design may or may not include a platform at the ramp’s surface is greater than 30 ft. of the ramp and must be longer than not include a flat resting section along (The bottom of the ramp is flat bottom.)

The vertical distance from the ground to the entrance is 2.75 ft. Using sine, sin 4 = \(\frac{2.75}{y}\), and thus,
y = \(\frac{2.75}{\sin 4}\)
y ≈ 39.42.
The total length of the ramp surface is approximately 39 ft. 5 in.; however, because of its length, it requires a resting platform somewhere in the first 30 ft. and another platform at the top.

b. What is the total distance from the start of the ramp to the entrance of the building in your design?
Answer:
If each platform is 5 ft. in length, then the total distance along the ramp from the ground to the building is approximately 49 ft. 5 in.

Question 6.
Tim is designing a roof truss in the shape of an isosceles triangle. The design shows the base angles of the truss to have measures of 18. 5°. If the horizontal base of the roof truss is 36 ft. across, what is the height of the truss?
Answer:

Let h represent the height of the truss in feet. Using tangent, tan 18.5 = \(\frac{h}{18}\), and thus,
h = 18(tan 18. 5)
h ≈ 6.
The height of the truss is approximately 6 ft.

Eureka Math Geometry Module 2 Lesson 28 Exit Ticket Answer Key

Question 1.
Given right triangle ABC with hypotenuse AB = 8.5 and m∠A = 55°, find AC and BC to the nearest hundredth.

Answer:
BC = 8. 5(sin 55)
BC ≈ 6.96

AC = 8.5 (cos 55)
AC ≈ 4.88

Question 2.
Given triangle DEF, m∠D = 22°, m∠F = 91°, DF = 16. 55, and EF = 6.74, find DE to the nearest hundredth.
Answer:
Draw altitude from F to \(\overline{D E}\) at point P. Cosines can be used on angles D and E to determine the lengths of \(\overline{D P}\) and \(\overline{P E}\), which together compose \(\overline{D E}\).
PE = 6. 74(cos 67)
PE ≈ 2. 6335

DP = 16. 55(cos 22)
DP ≈ 15. 3449

DE = DP+PE
DE ≈ 15. 3449 + 2. 6335
DE ≈ 17.98
Note to teacher: Answers of DE ≈ 17.97 result from rounding to the nearest hundredth too early in the problem.