The perimeter is the distance around a shape. A quadrilateral is a polygon that has four sides and four angles. To find the perimeter of a quadrilateral adds the measurements of four sides of it. We have given the most common types of quadrilaterals along with the perimeter of that quadrilateral. Check out the complete article and know how to find the perimeter of the quadrilateral. Some of the examples of a quadrilateral are Parallelogram, Rectangle, Square, Rhombus, and Trapezium, etc.
Also, Read:
- Sum of Angles of a Quadrilateral
- Different Types of Quadrilaterals
- Construct Different Types of Quadrilaterals
Perimeter of Different Types of Quadrilaterals
The sum of the four angles of the quadrilateral is equal to 360°. We have different types of quadrilateral. They are
- Parallelogram
- Rectangle
- Square
- Rhombus
- Trapezium
The perimeter of the Quadrilateral is the sum of the distance around the image. That means the perimeter of a quadrilateral is equal to the sum of the four sides of the image or object. That is,

(AB + BD + DC + CA) = Perimeter of the Quadrilateral.
1. Parallelogram
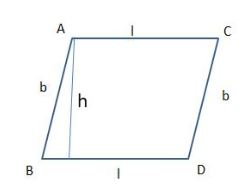
Here, the lengths of the two sides of the parallelogram are equal and the breadths of the two sides of the parallelogram are equal. Opposite sides are equal in a parallelogram.
Perimeter of the Parallelogram = (AB + BD + DC + CA)
= (b + l + b + l).
Perimeter of the Parallelogram = 2(l + b).
Here, ‘l’ represents the length and ‘b’ represents the breadth of the parallelogram.
Area of the parallelogram = Base * height.
2. Rectangle
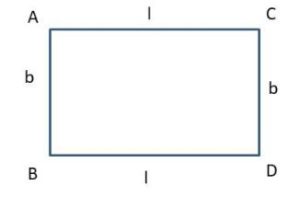
In a rectangle, both the lengths of the sides are equal and the breadths of the sides of a rectangle are equal. That means, opposite sides of the rectangle are equal.
Perimeter of the rectangle = (AB + BD + DC + CA) = (b + l + b + l) = 2(l + b).
Perimeter of the Rectangle = 2(l + b).
Area of the Rectangle = length * breadth = l *b.
Here, ‘l’ indicates the length of the rectangle and ‘b’ indicates the breadth of the rectangle.
3. Square
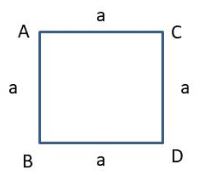
In this, a square is also enclosed with the four sides and the lengths of these four sides are equal.
Here, ‘a’ indicates the length of the sides of the square.
Perimeter of the square = (AB + BD + DC + CA) = (a + a + a + a) = 4a.
The perimeter of the square = 4a.
Area of the Square = Side * Side = a * a = a^2.
4. Rhombus
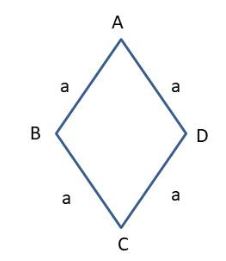
The lengths of the four sides of the rhombus are equal. Here, ‘a’ represents the length of the side.
Perimeter of the Rhombus = (AB + BC + CD + DA) = (a + a + a + a) = 4a.
The perimeter of the Rhombus = 4a.
Area of the Rhombus = (Base * height).
5. Trapezium
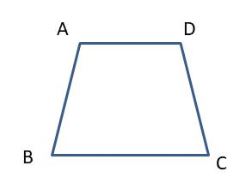
Two opposite sides of the trapezium are parallel. Perimeter of the Trapezium = (AB + BC + CD + DA).
AB + BC + CD + DA = (c + b + d + a)cm.
Area of the Trapezium = (a + b) / 2 * h.
Here, a, b, c, d are the sides of the trapezium. And ‘h’ indicates the height of the trapezium.
Perimeter of Quadrilateral Examples
1. Find the Perimeter of the Quadrilateral with the sides 2cm, 10cm, 5cm, and 20cm?
Solution:
The given information is the length of the four sides of the quadrilateral is = 2cm, 10cm, 5cm, 20cm.
The perimeter of the quadrilateral = sum of the length of the four sides of the quadrilateral.
Perimeter of the quadrilateral = (2 + 10 + 5 + 20)cm. = 37cm.
So, the perimeter of the quadrilateral is equal to 37cm.
2. The Perimeter of the quadrilateral is 40cm and the length of the three sides of the quadrilateral is 5cm, 10cm, and 5cm. Find the length of the four sides of the quadrilateral?
Solution:
The given details are the Perimeter of the quadrilateral = 40cm.
Length of the three sides of quadrilateral = 5cm, 10cm, and 5cm.
The perimeter of the quadrilateral = sum of the length of four sides of the quadrilateral.
40cm = (5 + 10 + 5 + x) cm.
40 = 20 + x.
x = 40 – 20 = 20cm.
Finally, the length of the fourth side of the quadrilateral is equal to 20cm.
3. A woman crosses a distance of 24m long while going round a quadrilateral field twice. What will be the cost of fencing the field at the rate of cost $1.20per m?
Solution:
The given information is Women crosses a distance of 24m long = perimeter of its boundary = 24 / 2 = 12m.
Cost of fencing the field for 1m = $1.20.
Then the cost of 12m of fencing the field = 12 * $1.20 = $14.4.
Therefore, the cost of the 12m fencing field is equal to $14.4.
4. One side of the square is 4cm. Find the perimeter of the square?
Solution:
As per the given information, the length of the side of the square = 4cm.
The perimeter of the square = 4a.
Here, a = 4.
By substituting the ‘a’ value in the formulae, we will get
Perimeter of the square = 4 * 4 = 16cm.
So, the perimeter of the square is equal to 16cm.
5. Length of the rectangle is 10cm and the breadth of the rectangle is 5cm. Calculate the Perimeter of the Rectangle?
Solution:
As per the given details, the Length of the rectangle (l) = 10cm.
Breadth of the rectangle (b) = 5cm.
Perimeter of the rectangle = 2(l + b).
By substituting the values in the formulae, we will get like
Perimeter of the rectangle = 2(10 + 5) = 2(15) = 30cm.
Therefore, the perimeter of the rectangle is equal to 30cm.
6. If the area of the rhombus is 20 square units and the height of the rhombus is 6 units, then calculate the base of the rhombus?
Solution:
From the given information, Area of the Rhombus = 20 Sq units.
Height of the Rhombus (h) = 6 units.
Area of the Rhombus = Base * height.
20 = Base * 6.
Base = 20 / 6 = 10 / 3 = 0.3 units.
The base of the Rhombus is equal to 0.3 units.
Frequently Asked Questions
1. What is Quadrilateral?
The quadrilateral is enclosed with four sides. It has four corners, four angles, and four sides. The total angle of the quadrilateral is equal to 360°.
2. What are the types of Quadrilateral?
The types of Quadrilateral are,
1. Square
2. Rectangle
3. Parallelogram
4. Trapezium
5. Rhombus
3. How we can find the perimeter of a quadrilateral?
The area of the quadrilateral is the sum of all sides of the quadrilateral. Yes, by adding all sides of the quadrilateral, we can find the perimeter of the quadrilateral.