Engage NY Eureka Math Algebra 1 Module 4 Lesson 4 Answer Key
Eureka Math Algebra 1 Module 4 Lesson 4 Example Answer Key
Example: Splitting the Linear Term
How might we find the factors of 6x2 + 5x – 6?
1. Consider the product (a)(c): (6)( – 6) = – 36.
2. Discuss the possibility that a and c are also multiplied when the leading coefficient is 1.
3. List all possible factor pairs of (a)(c): (1, – 36), ( – 1,36), (2, – 18), ( – 2,18), (3, – 12), ( – 3,12), (4, – 9), ( – 4,9), and ( – 6,6).
4. Find the pair that satisfies the requirements of the product – sum method (i.e., a pair of numbers whose product equals ac and whose sum is b): ( – 4) + 9 = 5.
5. Rewrite the expression with the same first and last term but with an expanded b term using that pair of factors as coefficients: 6x2 – 4x + 9x – 6.
6. We now have four terms that can be entered into a tabular model or factored by grouping.
7. Factoring by grouping: Take the four terms above and pair the first two and the last two; this makes two groups.
[6x2 – 4x] + [9x – 6] [Form two groups by pairing the first two and the last two.]
[2x(3x – 2)] + [3(3x – 2)] [Factor out the GCF from each pair.]
The common binomial factor is now visible as a common factor of each group. Now rewrite by carefully factoring out the common factor, 3x – 2, from each group: (3x – 2)(2x + 3).
Note that we can factor difficult quadratic expressions, such as 6x2 + 5x – 6, using a tabular model or by splitting the linear term algebraically. Try both ways to see which one works best for you.
Answer:
Have students try switching the – 4x and the + 9x in step 5. A common error when factoring out a negative number is to mix up the signs on the final result.
→ Does it work? Check your answer by multiplying the binomials.
Yes, it works. (3x – 2)(2x + 3) = 6x2 – 4x + 9x – 6 = 6x2 + 5x – 6
We can factor difficult quadratic expressions using the tabular model method or by splitting the linear term algebraically. Demonstrate the two methods below for factoring 6x2 + 5x – 6, and ask students to compare the two methods.
For each example, we start with the original trinomial, 6x2 + 5x – 6, and find the two numbers whose product is (a)(c) and whose sum is b. In this case, the numbers are ( + 9) and ( – 4). (Hint: Always keep the associated sign with the numbers.)
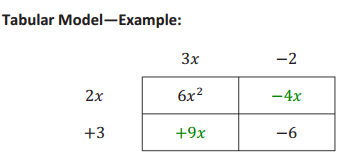
Fill in the table’s cells starting with 6x2 and – 6 in the left to right diagonal and the split linear term in the right to left diagonal. Working backward, you may have to try a few combinations since the upper left cell could have been formed from (2x)(3x) or (6x)(x), and the lower right could come from (±2)(±3) or (±1)(±6). Just look for combinations that also give you the linear terms in the other diagonal.
The final answer is (3x – 2)(2x + 3).
Splitting the Linear Term—Example:
Using the two numbers we found as coefficients on the linear term (sometimes called the middle term), split into two parts:
6x2 – 4x + 9x – 6.
Grouping by pairs (i.e., putting the first two together and the second two together) and factoring out the GCF from each, shows that one of the factors is visible as the common factor.
2x(3x – 2) + 3(3x – 2)
Do you see the common factor in the two groups?
(3x – 2)(2x + 3)
You can always check your answers by multiplying the factors:
→ What are the advantages of using the tabular model? What are the disadvantages?
Responses should vary and reflect personal choices. Some students may see the tabular model’s visual element as an advantage. Some may see it as more complicated than the algebraic method.
→ What are the advantages of using the second method, splitting the linear term? What are the disadvantages?
Responses should vary and reflect personal choices. Some students may prefer the algebraic method for its simplicity. Some may prefer the visual aspect of the tabular model.
→ How is using the tabular model similar to using the product – sum method?
Both look for two numbers that equal the product, (a)(c), and whose sum is b.
Eureka Math Algebra 1 Module 4 Lesson 4 Exercise Answer Key
Opening Exercise
Factor the following quadratic expressions.
a. 2x2 + 10x + 12
Answer:
2(x2 + 5x + 6) = 2(x + 2)(x + 3)
b. 6x2 + 5x – 6
Answer:
(3x – 2)(2x + 3)
Exercises
Factor the following expressions using your method of choice. After factoring each expression completely, check your answers using the distributive property. Remember to always look for a GCF prior to trying any other strategies.
Exercise 1.
2x2 – x – 10
Answer:
Find two numbers such that the product is – 20 and the sum is – 1: ( – 5)( + 4).
Now reverse the tabular model or split the linear term, grouping by pairs:
x(2x – 5) + 2(2x – 5) = (2x – 5)(x + 2).
Exercise 2.
6x2 + 7x – 20
Answer:
Find two numbers such that the product is – 120 and the sum is + 7: ( – 8)( + 15).
Now reverse the tabular model or split the linear term, grouping by pairs:
2x(3x – 4) + 5(3x – 4) = (3x – 4)(2x + 5).
Exercise 3.
– 4x2 + 4x – 1
Answer:
Find the two numbers such that the product is + 4 and the sum is + 4: ( + 2)( + 2).
Now reverse the tabular model or split the linear term, grouping by pairs:
– 4x2 + 2x + 2x – 1 = – 2x(2x – 1) + 1(2x – 1) = (2x – 1)( – 2x + 1).
Exercise 4.
The area of a particular triangle can be represented by x2 + \(\frac{3}{2}\) x – \(\frac{9}{2}\). What are its base and height in terms of x?
Answer:
Factoring out the \(\frac{1}{2}\) first gives: \(\frac{1}{2}\)(2x2 + 3x – 9). Now we are looking for a pair of numbers with product – 18 and sum + 3: ( – 3)( + 6).
Now split the linear term: \(\frac{1}{2}\) (2x2 – 3x + 6x – 9) = \(\frac{1}{2}\) (x(2x – 3) + 3(2x – 3)) = \(\frac{1}{2}\) (2x – 3)(x + 3).
So, the dimensions of the triangle would be 2x – 3 and x + 3 for the base and the height. (There is not enough information to tell which is which.)
Eureka Math Algebra 1 Module 4 Lesson 4 Problem Set Answer Key
Question 1.
Factor completely.
a. 9x2 – 25x
Answer:
x(9x – 25)
b. 9x2 – 25
Answer:
(3x + 5)(3x – 5)
c. 9x2 – 30x + 25
Answer:
(3x – 5)(3x – 5) or (3x – 5)2
d. 2x2 + 7x + 6
Answer:
(2x + 3)(x + 2)
e. 6x2 + 7x + 2
Answer:
(3x + 2)(2x + 1)
f. 8x2 + 20x + 8
Answer:
GCF is 4: 4(2x2 + 5x + 2) = 4(2x + 1)(x + 2)
g. 3x2 + 10x + 7
Answer:
(3x + 7)(x + 1)
h. x2 + \(\frac{11}{2}\) x + \(\frac{5}{2}\)
Answer:
\(\frac{1}{2}\) (2x2 + 11x + 5) = \(\frac{1}{2}\)(2x + 1)(x + 5)
i. 6x3 – 2x2 – 4x [Hint: Look for a GCF first.]
Answer:
2x(3x2 – 1x – 2) = 2x(3x + 2)(x – 1)
Question 2.
The area of the rectangle below is represented by the expression 18x2 + 12x + 2 square units. Write two expressions to represent the dimensions, if the length is known to be twice the width.

Answer:
If we factor out the 2 first (GCF), we can use that to double one of the dimensions after we finish factoring to give us 2(9x2 + 6x + 1) = 2(3x + 1)(3x + 1) = (6x + 2)(3x + 1). So, the length is (6x + 2), and the width is (3x + 1).
Question 3.
Two mathematicians are neighbors. Each owns a separate rectangular plot of land that shares a boundary and has the same dimensions. They agree that each has an area of 2x2 + 3x + 1 square units. One mathematician sells his plot to the other. The other wants to put a fence around the perimeter of his new combined plot of land. How many linear units of fencing does he need? Write your answer as an expression in x.
Note: This question has two correct approaches and two different correct solutions. Can you find them both?
Answer:
The dimensions of each original plot can be found by factoring the expression for area given in the prompt, 2x2 + 3x + 1, which gives us (2x + 1)(x + 1) as the dimensions. Selecting which boundary is common affects the solution because the length of the common side is not included when finding the perimeter of the combined plot. Those measures could be either (2x + 1) or (x + 1).
If the first: P = 2(2x + 1) + 4(x + 1) = 4x + 2 + 4x + 4 = 8x + 6.
If the second: P = 2(x + 1) + 4(2x + 1) = 2x + 2 + 8x + 4 = 10x + 6.
Eureka Math Algebra 1 Module 4 Lesson 4 Exit Ticket Answer Key
Question 1.
Explain the importance of recognizing common factors when factoring complicated quadratic expressions.
Answer:
Students should see the importance of factoring out a GCF before attempting to apply the reverse tabular model or splitting the linear term. In every case, the quadratic expressions are much easier to handle if the common factors are out of the way.
Question 2.
Factor: 8x2 + 6x + 1.
Answer:
(4x + 1)(2x + 1)