Prasanna

This article will assist the students in learning and understanding more about the geometry concept volume. Here, you will find different volume questions for different shapes such as cube, cone, cylinders, and sphere, etc. Indeed students can have the volume questions from easy ones to difficult ones.

Also, you can get an idea of volume in terms of square units and also in cubic units. Teachers and students of can use this article to find volume test questions for different shapes.

Also, Read: Units of Volume

Volume – Definition & Formulas

A volume is defined as the amount of space occupied by any three-dimensional object. An object can be a cube, a solid, a cuboid, and a sphere, etc. In volume different shapes have different volumes. In geometry, we have shapes and solids namely cube, cuboid, sphere, and cylinder, etc., all are defined in three dimensions.

Name of geometrical shape	Volume formula
Cube	V = a3, where a is the edge length of a cube
Cuboid	V = length x width x height
Cone	V = ⅓ πr²h, Where r is the radius and h is the height of a cone
Cylinder	V = πr²h, Where r is the radius and h is the height of a cylinder
Sphere	V = 4/3 πr3, Where r is the radius of a sphere
Volume of Frustum	πh/3 (R2+r2+Rr) Where ‘R’ and ‘r’ are the radii of base and top of frustum
Volume of Prism	Base Area x Height
Volume of Pyramid	⅓ (Area of base) (Height)
Volume of Hemisphere	⅔ (πr3), Where r is the radius

Let us practice some problems on volume in different shapes and solids to get a clear perception for students about the concept of volume.

Practice Questions on Volume

1. Find the volume of a cube, having the sides of length 6 cm.

Solution: 

Given, the length of the sides of the cube is 6 cm.
As we know, the volume of a cube = (length of the sides of a cube)³ = a³.
Now, volume = (6 cm)³ = 6 cm× 6 cm× 6 cm = 216 cm³.
Thus, the volume of a cube, V = 261 cm³.

---

2. Find the volume of a cube of a side 22 cm.

Solution: 

Given, the volume of a cube of a side is 22 cm.
The volume of a cube, V = a³.
Now, V = (22 cm)³ = 22 cm× 22 cm× 22 cm = 10648 cm³.
Hence, the volume of a cube is 10648 cm³.

---

3. Find the volume of a cuboid of dimensions 6 cm, 4.2 cm, 12 cm.

Solution: 

Given, Volume of cuboid dimensions is 6 cm, 4.2 cm, 12 cm.
The formula used for the volume of cuboid is V = length x width x height.
V = 6 cm× 4.2 cm× 12 cm = 302.4 cubic centimeters.
Therefore, the volume of a cuboid is 302.4 cu cm.

---

4. A cylinder has a diameter of 4.2 cm and is 6.4 cm in height. Calculate the volume of a cylinder and have to answer with one decimal place.

Solution: 

Given, dimensions are the diameter of 4.2 cm and height of 6.4 cm.
The volume of a cylinder is V = πr²h.
Now, V = 3.14× 2.1× 2.1× 6.4 = 88.62336 cm³.
Thus, the volume of a cylinder is 88.6 cm³.

---

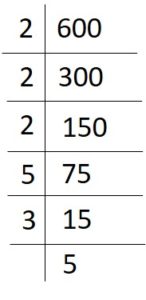
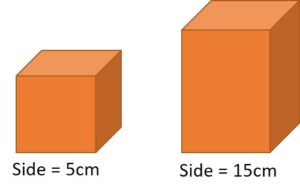
5. How many cubes of side 5 cm are needed to build a cube of side 15 cm?

Solution: 

Given
The side of a small cube is 5 cm.
The side of a large cube is 15 cm.
We required n no. of small cubes.
The volume of the cube, V = a³
n no. of cubes = Volume of a large cube / Volume of a small cube
Now, we find the volume of both the cubes
The volume of a small cube, V = (5 cm)³ = 125 cm³.
The volume of a large cube, V = (15 cm)³ = 3375 cm³.
n no. of cubes = 3375/125 = 125.
Hence, 125 cubes of side 5 cm are essential to build a cube of 15 cm.

---

6. Find the length of the edges of a cube, if the volume is equal to 216 m³.

Solution: 

Given, a cube of a volume is 216 m³.
Let, the length of the edges is a³.
By the formula, Volume of a cube, V = (length of the edges of a cube)³ = a³.
Now, substitute the given values
216 = a³
a = ³√216 = 6 m
Hence, the length of a cube is 6 m.

---

7. What is the volume of a gift box, if the dimensions of a cuboidal gift box are 20 inches, 45 inches, 15 inches?

Solution:

Given that, a gift box is in cuboidal shape.
The dimensions of a gift box are
The length of the gift box is 20 in
The width of the gift box is 45 in
The height of the gift box is 15 in
The volume of the gift box is
V =  length × width × height
V = 20 in× 45 in× 15 in = 13,500 in³.
Therefore, the volume of a cuboidal gift box is 13,500 cubic inches.

---

8. If the cuboid volume is 212 cm³, its length is 6 cm and height is 8 cm. Find the cuboid breadth?

Solution: 

Given that,
Cuboid volume is 212 cm³
Cuboid length is 6 cm
Cuboid height is 8 cm
The formula for the volume of cuboid is, V = length × breadth × height
Substitute the given values in the formula,
212 = 6 × breadth × 8
Cuboid breadth = Volume / (length × height)
Cuboid breadth = 212 / (6 × 8) = 212 / 48 = 4.416 cm.
Thus, the breadth of a cuboid is 4.416 cm.

---

Subtracting Literals Worksheet deals with the subtraction of literals using various ways and solve the questions on the concept. Different models of questions used in the Worksheet on Subtraction of Literals make these activity sheets engaging and interesting. Subtraction of Literals is a crucial topic that you will find in algebra concepts. Subtracting Literals Worksheet PDF approaches questions in a stepwise manner so that you won’t find any difficulty in understanding the questions. Download the Subtraction of Literals Worksheet in PDF Formats for free and score well in your exams.

Also, See:

• Worksheet on Multiplication of Literals
• Worksheet on Division of Literals

Subtracting Literals Practice Worksheet

I. Represent the following statements algebraically:

(i) 12 less than z
(ii)  x less than 54
(iii) 380 taken away from m
(iv) 67 less than x
(v) Decrease the sum of m and n by p
(vi) y less than 220
(vii) y less than the sum of z and 7

Solution:

(i) 12 less than z is represented as z-12
(ii)  x less than 54 is represented as 54-x
(iii) 380 taken away from m is represented as m-380
(iv) 67 less than x is represented as x-67
(v) Decrease the sum of m and n by p is represented as (m+n)-p
(vi) y less than 220 is represented as 220-y
(vii) y less than the sum of z and 7 is y-(z+7)

---

II. Write each of the following algebraically using signs and symbols:
(i) 357 less than literal y
(ii) Decrease m by o
(iii) Subtract h from 1000
(iv) 185 is diminished by w
(v) n less than 88
(vi) 43 less than p
(vii) 689 less than literal n
(viii) 42 less than v
(ix) 323 is diminished by k

Solution:

(i) 357 less than literal y is represented as y-357.
(ii) Decrease m by o is represented as m – o.
(iii) Subtract h from 1000 is represented as 1000 – h.
(iv) 185 is diminished by w is represented as 185 – w.
(v) n less than 88 is represented as 88 – n.
(vi) 43 less than p is represented as p-43.
(vii) 689 less than literal n is represented as n-689.
(viii) 42 less than v is represented as v-42.
(ix) 323 is diminished by k is represented as 323 – k.

---

III. Write each of the following statements using numbers, literals, and symbols:
(i) Subtract x from 770
(ii) r less than a sum of q and 20
(iii) 13 less than a sum of b and c
(iv) Decrease the sum of p and q by y
(v) Decrease the sum of k and 10 by l
(vi) x is diminished by 890
(vii) Decrease b by 7

Solution:

(i) Subtract x from 770 is represented as 770-x
(ii) r less than a sum of q and 20 is represented as (q+20)-r
(iii) 13 less than a sum of b and c is represented as (b+c)-13
(iv) Decrease the sum of p and q by y is represented as (p+q)-y
(v) Decrease the sum of k and 10 by l is represented as (K+10)-l
(vi) x is diminished by 890 is represented as x-890
(vii) Decrease b by 7 is represented as b-7

---

IV. Write the following statements algebraically:
(i) 19 less than literal k
(ii) Decrease m by 9
(iii) Subtract 18 from y
(iv) n took away from d
(v) 48 is diminished by v
(vi) 109 less than a sum of m and n
(vii) 500 less than literal z
(viii) Number 660 less than w

Solution:

(i) 19 less than literal k is represented as k-19
(ii) Decrease m by 9 is represented as m-9
(iii) Subtract 18 from y is represented as y-18
(iv) n took away from d is represented as d-n
(v) 48 is diminished by v is represented as 48-v
(vi) 109 less than a sum of m and n is represented as (m+n)-109
(vii) 500 less than literal z is represented as z-500
(viii) Number 660 less than w is represented as w-660

---

 

Powers of Literal Numbers is nothing but the repeated product of a number with itself written in exponential form. Practice using the Worksheet on Powers of Literal Numbers and know the different models of questions framed on the topic. Use the Powers of Literal Numbers Worksheet PDF as a cheat sheet to self-examine your preparation on the concept.

Math Students are advised to solve the questions from the Powers of Literal Numbers Worksheet with Answers to master the concept as well as to enhance their general math skills. You can also check the Solutions for the Problems on Literal Numbers Powers in case of any doubts and learn how to frame quality answers in your exams and thereby score well.

Free Printable Worksheet on Powers of Literal Numbers

I. Write each of the following in the exponential form:
(i) c × c × c × c × c
(ii) 5 × c × b × z × z × z
(iii) m × 12 × n × b × z
(iv) m× n × p × q × n × 55
(v) 230 × b × c × c × b
(vi) m × m × n × n × n × 280

Solution:

(i) Given c × c × c × c × c
Here c has written as 5 times.
It can be written as an exponent of 5.
c × c × c × c × c=c⁵
(ii) Given 5 × c × b × z × z × z
Here 5 is written ‘1’ time.
c has written ‘1’ time.
b has written ‘1’ time.
z has written 3 times.
5 × c × b × z × z × z=5cbz³
(iii) m × 12 × n × b × z
Here m is written ‘1’ time.
12 has written ‘1’ time.
n,b, and Z are written 1 time.
m × 12 × n × b × z=12mnbz

(iv) m× n × p × q × n × 55
Here m is written ‘1’ time.
n has written 2 times.
p,q,55 are written 1 time.
m× n × p × q × n × 55 =55pqmn²
(v) 230 × b × c × c × b
Here 230 is written ‘1’time.
b has written 2 times.
c has written 2 times.
230 × b × c × c × b= 230b²c²

(vi) m × m × n × n × n × 280
Here m is written 2 times.
n has written 3 times.
280 has written ‘1’ time.
m × m × n × n × n × 280=280m²n³

---

II. Convert each of the following exponential form to (expanded) product form:
(i) y³z²
(ii) p²q³r⁵
(iii) 5khb²
(iv) 18c³d⁴h⁵
(v) 25ab²cd³

Solution:

(i) y³z²
y³ is written in expanded form as y × y × y.
z² is written in expanded form as z × z.
y³z²= y × y × y × z × z.
(ii) p²q³r⁵
p² is written in expanded form as p × p.
q³ is written in expanded form as q × q × q.
r⁵ is written in expanded form as r × r × r × r × r.
p²q³r⁵= p × p × q × q × q × r × r × r × r × r.
(iii) 5khb²
b² is written in expanded form as b × b.
5,h,k is written only once.
5khb²= 5 × k ×h × b × b.
(iv) 18c³d⁴h⁵
c³ is written in expanded form as c × c × c.
d⁴ is written in expanded form as d × d × d × d.
h⁵ is written in expanded form as h × h × h × h × h.
18c³d⁴h⁵=18 × c × c × c × d × d × d × d × h × h × h × h × h.
(v) 25ab²cd³
25, a, c are written once.
b² is written in expanded form as b × b.
d³ is written in expanded form as d × d × d.
25ab²cd³=25 × a × b × b × c × d × d × d.

---

III. Write each of the following in product form:
(i) 3p²q⁴r
(ii) 73b⁴c²z³
(iii) a⁴b³c²
(iv) 7p²q³r⁴
(v) 17ac²dy³

Solution:

(i) Given 3p²q⁴r
p²=p × p
q⁴=q × q × q × q
3p²q⁴r is written in product form as 3 × p × p × q × q × q × q × r.

(ii) Given 73b⁴c²z³
b⁴= b × b × b × b
c²= c × c
z³= z × z × z
73b⁴c²z³ is written in product form as 73 × b × b × b × b × c × c × z × z × z.
(iii) Given a⁴b³c²
a⁴= a × a × a × a
b³= b × b × b
c² = c × c
a⁴b³c² is written in product form as a × a × a × a × b × b × b × c × c
(iv)Given 7p²q³r⁴
p²= p × p
q³= q × q × q
r⁴= r × r × r × r
7p²q³r⁴ is written in product form as 7 × p × p × q × q × q × r × r × r × r.
(v) Given 17ac²dy³
c²= c × c
y³= y × y × y
17ac²dy³=17 × a × c × c × d × y × y × y.

---

IV. Write each of the following products in index (exponential) form:
(i) p × p × p × p x a × a × b × b × b
(ii) 10 × a × a × b × b × b × c
(iii) c × c × c × c × ..… 8 times d × d × d × d × ..… 8 times.
(iv) a × a × a × ..… 15 times b × b × b × ..… 7 times c × c × c× ..… 20 times.
(v) 5 × a × a × a ×…6 times  b × b × b

Solution:

(i) Given p × p × p × p x a × a × b × b × b
Here P is written 4 times. It can be written as an exponent of 4.
p × p × p × p=p⁴
a was written 2 times. It can be written as an exponent of 2.
a × a=a²
b was written 3 times. It can be written as an exponent of 3.
b × b × b=b³
p × p × p × p x a × a × b × b × b in exponential form is p⁴a²b³.
(ii) Given 10 × a × a × b × b × b × c
Here a is written 2 times. It can be written as an exponent of 2.
a × a=a²
b was written 3 times. It can be written as an exponent of 3.
b × b × b=b³
10 × a × a × b × b × b × c=10a²b³c.
(iii) Given c × c × c × c × ..… 8 times d × d × d × d × ..… 8 times.
c was written 8 times. It can be written as an exponent of 8.
c × c × c × c × ..… 8 times=c⁸
d was written 8 times. It can be written as an exponent of 8.
d × d × d × d × ..… 8 times=d⁸
c × c × c × c × ..… 8 times d × d × d × d × ..… 8 times=c⁸d⁸.
(iv) Given a × a × a × ..… 10 times b × b × b × ..… 7 times c × c × c × ..… 14 times.
a was written 10 times. It can be written as an exponent of 10.
a × a × a × ..… 10 times=a¹⁰.
b was written 7 times. It can be written as an exponent of 7.
b × b × b × ..… 7 times=b⁷.
c was written 14 times. It can be written as an exponent of 14.
a × a × a × ..… 10 times b × b × b × ..… 7 times c × c × c × ..… 14 times=a¹⁰b⁷c14.
(v) Given 5 × a × a × a ×…6 times b × b × b
a was written 6 times. It can be written as an exponent of 6.
a × a × a ×…6 times=a⁶
b was written 3 times. It can be written as an exponent of 3.
b × b × b=b³
5 × a × a × a ×…6 times b × b × b=5a⁶b³

---

V. State whether true or false:
(i) m × m × m × m × m × m = m6
(ii) 5 × 2 × a × a × b × b = 52a²b²
(iii) 3 × 7 × b × b × c × c × c = 33b²c³
(iv) k × l × k × l × k × l = k³l³
(v) m × n × 10 × p × q × q× p = 10mnp²q²
(vi) a × a × a × b × b × b × c × c=a³b³c²
(vii) 6 × a × a × b × b × b × c × c=6a²b³c²

Solution:

(i) true
(ii) false
(iii) false
(iv) true
(v) true
(vi) true
(vii) true

---

 

Worksheet on Circle is a great resource for students to learn complete circle concepts in Math. Practice all the questions provided in the Circle Worksheet to score good marks in the exams. All questions including radius, diameter, Circumference, Area are given in this article.

Check out different ways to solve circle math problems in this article. The Circle Worksheet with Answers has questions on finding the circumference, area, identifying radius, chord, and diameter of a circle, etc. Download the Printable Worksheet on Circle in PDF Formats and practice the questions on a frequent basis and improve your proficiency in the concept.

Do Refer: Practice Test on Circle

Circle Worksheet with Answers

1. Explain the following terms of a circle?
(i) Radius
(ii) Centre
(iii) Chord
(iv) Diameter
(v) Interior of a Circle

Solution:

Radius – The radius is the line segment from the center of the circle to the circumference or surface of the circle.
Center – The Center of the circle is the middle point that is equidistant from all the points on the edge of the circle.
Chord – The chord on the circle that joins two points on the circumference.
Diameter – The diameter of the circle is defined as double the length of the radius of a circle.

---

2. The following figure shows a circle with center O and some line segments drawn in it. Classify the line segments as chord, radius, and diameter, etc.

(i) OM = ………………..
(ii) OL = ………………..
(iii) ON = ………………..
(iv) AB = ………………..
(v) CD = ………………..
(vi) EF = ………………..

Solution:

(i) OM = Radius
(ii) OL = Radius
(iii) ON = Radius.
(iv) AB = Diameter
(v) CD = Chord
(vi) EF = Chord

---

3. Find the area and the circumference of a circle whose radius is 30 cm. (Take the value of π = 3.14)

Solution:

Given that the radius is 30 cm.
Area =πr²
Area =  3.14  × (30)²
Area =  2826 cm²
Circumference, C = 2πr
Circumference = 2 × 3.14 × 30
Circumference = 188.4 cm

---

4. Find the area of a circle whose circumference is 15.7 cm?

Solution:

Given that the circumference is 15.7 cm.
To find the area of a circle, we must find the radius.
From the circumference, the radius can be calculated
2 π r = 15.7
(2)(3.14)r = 15.7
r = \(\frac { 15.7 }{ (2)(3.14) } \)
r = \(\frac { 15.7 }{ 6.28 } \)
r = 2.5
Therefore, the radius of the circle is 2.5 cm.
The area of a circle is πr² square units
Now, substitute the radius value in the area of a circle formula, we get
A = π(2.5)²
A = 3.14 x 6.25
A =  19.625 cm²

Therefore, the area of a circle is 19.625 cm².

---

5. Observe the circles given below and identify them.

(a) Radius = …………………………
(b) Chord = …………………………
(c) Diameter = …………………………

Solution:

(a) Radius = OC, OD, OM
(b) Chord = EF, CN
(c) Diameter = CD

---

6. Take a point and draw a circle of radii 5 cm, 2 cm, 7 cm, each having the same center M.

Solution:

Given that three circles having a radius of 5 cm, 2 cm, 7 cm.
All 3 circles must have the same center named as M.
So, the figure with three different radii is given below.

---

7. Take two points Q and R on a circle. Draw a circle with Q in the center and passes through R.

Solution:

Given that two points Q and R are present on a circle.
Q is the center of the circle and it passes through R.
So, the figure with three different radii is given below.

---

8. To cover a distance of 5 km a wheel rotates 2500 times. Find the radius of the wheel?

Solution:

Given that a distance of 5 km a wheel rotates 2500 times.
No. of rotations = 2500.
Total distance covered = 5 km, and we have to find out the radius of the circle.
Let ‘r’ be the radius of the wheel.
Circumference of the wheel = Distance covered in 1 rotation = 2πr.
In 2500 rotations, the distance covered = 5 km = 500000 cm.
Hence, in 1 rotation, the distance covered = 500000 cm/2500 = 200cm
But this is equal to the circumference. Hence, 2πr = 200 cm
r = \(\frac { 200 }{ 2π } \)
r = \(\frac { 100 }{ π } \)
Taking the approximate value of π as 22/7, we get
r = 100 x 7/22
r = 31.82 cm approx.

---

9. The difference between the circumference and the diameter of a circular bangle is 10 cm. Find the radius of the bangle. (Take π = 22/7)

Solution:

Let the radius of the bangle be ’r’.
According to the question:
Circumference – Diameter = 10 cm
We know that the Circumference of a circle = 2πr
Diameter of a circle = 2r
Therefore, 2πr – 2r =10 cm
2r(π-1) = 10 cm
2r((22/7) – 1) = 10 cm
r(\(\frac { 15 }{ 7 } \)) = 5 cm
r = 5 (\(\frac { 7 }{ 15 } \))
r = 2.333 cm

The radius of the bangle is 2.333 cm.

---

10. Observe the circles given below and identify

Label the centre as O.
Draw 2 radius OA and OB.
Draw the chord CB.

Solution:

Given that the circle and point the centre as O. OA = OB = 2.
The CB is a chord.
The below figure shows the exact details of the given information.

---

11. Draw a diameter, radius, chord in the given circle using the points. Also, find the length of diameter and radius.
(i) Radius = ……………… = ……………… cm
(ii) Diameter = ……………… = ……………… cm
(iii) Highlight the circumference by using green color.
(iii) Highlight the chord by using blue color.

Solution:

Given that the circle and point the centre as O.
Let us consider the radius 2 cm.
OL and OM are the radii of the circle. LM is the diameter of the circle.
The MN and NL are the is a chord of the circle.
(i) OL = OM = 2 cm
(ii) LM = 4 cm = 2 (OL)
The below figure shows the exact details of the given information.

---

12. Draw a circle of the radius with the radii
(i) 6 cm
(ii) 4 cm
having the same circle.

Solution:

Given that two circles with radii of 4 cm and 6 cm.
Both circles must have the same center.
Let the centre be X. Then, the first circle will have a radius of 4 cm. The second circle will have a radius of 6 cm.

---

13. Draw a circle of radius 5 cm.

Solution:

Take a Circle with centre O. Draw the radius of the circle 5 cm.
The radius of the circle is the line segment from the center to any point on the circumference.
Let the point be A.
OA = 5 cm.

---

14. Draw a circle of diameter 7.5 cm.

Solution:

Take a Circle with centre O. Draw the diameter of the circle 7.5 cm.
The diameter of the circle is defined as double the length of the radius of a circle.
Let the points of the diameter are A and B.
AB = 7.5 cm.

---

15. Draw a circle with centre M and radius 2.4 cm. Mark points A, B, C such that A lies in the interior of the circle, B lies on the circle and C lies in the exterior of the circle.

Solution:

Take a Circle with centre M. Draw the given points on the circle.
A lies inside the circle. B lies on the circle and C lies outside the circle.

---

16. Draw a circle whose diameter is 20 cm. Find its radius?

Solution:

Given that the diameter of the circle is 20 cm.
The diameter is double the length of the radius.
d = 2r where d is the diameter of the circle and r is the radius of the circle.
20 = 2r
r = 10 cm.
Therefore, the radius of the circle is 10 cm.

---

17. Find the radius if the diameter of the circle is:
(i) 6 cm
(ii) 16 cm
(iii) 14 m
(iv) 18 cm

Solution:

(i) Given that the diameter of the circle is 6 cm.
The diameter is double the length of the radius.
d = 2r where d is the diameter of the circle and r is the radius of the circle.
6 = 2r
r = 3 cm.
Therefore, the radius of the circle is 3 cm.

(ii) Given that the diameter of the circle is 16 cm.
The diameter is double the length of the radius.
d = 2r where d is the diameter of the circle and r is the radius of the circle.
16 = 2r
r = 8 cm.
Therefore, the radius of the circle is 8 cm.

(iii) Given that the diameter of the circle is 14 cm.
The diameter is double the length of the radius.
d = 2r where d is the diameter of the circle and r is the radius of the circle.
14 = 2r
r = 7 cm.
Therefore, the radius of the circle is 7 cm.

(iv) Given that the diameter of the circle is 18 cm.
The diameter is double the length of the radius.
d = 2r where d is the diameter of the circle and r is the radius of the circle.
18 = 2r
r = 9 cm.
Therefore, the radius of the circle is 9 cm.

---

18. Find the diameter if the radius of the circle is:
(i) 22 cm
(ii) 5 cm
(iii) 20 cm
(iv) 19 cm

Solution:

(i) Given that the radius of the circle is 22 cm.
The radius is half of the diameter of the circle.
d = 2r where d is the diameter of the circle and r is the radius of the circle.
d = 2r
d = 2 (22) cm.
d = 44 cm.
Therefore, the diameter of the circle is 44 cm.

(ii) Given that the radius of the circle is 5 cm.
The radius is half of the diameter of the circle.
d = 2r where d is the diameter of the circle and r is the radius of the circle.
d = 2r
d = 2 (5) cm.
d = 10 cm.
Therefore, the diameter of the circle is 10 cm.

(iii) Given that the radius of the circle is 20 cm.
The radius is half of the diameter of the circle.
d = 2r where d is the diameter of the circle and r is the radius of the circle.
d = 2r
d = 2 (20) cm.
d = 40 cm.
Therefore, the diameter of the circle is 40 cm.

(iv) Given that the radius of the circle is 19 cm.
The radius is half of the diameter of the circle.
d = 2r where d is the diameter of the circle and r is the radius of the circle.
d = 2r
d = 2 (19) cm.
d = 38 cm.
Therefore, the diameter of the circle is 38 cm.

---

19. With the same circle, draw three circles first with a radius of 3 cm. second with a radius of 5 cm, and third with a radius of 7 cm.

Solution:

Given that three circles having a radius of 3 cm, 5 cm, 7 cm.
All 3 circles must have the same center named as M.
So, the figure with three different radii is given below.

---

20. A circle has a radius 8 cm. Find the length of the longest chord of this circle?

Solution:

Given that a circle has a radius of 8 cm.
The longest chord of this circle is a diameter.
d = 2r where d is the diameter of the circle and r is the radius of the circle.
d = 2r
d = 2 (8) cm.
d = 16 cm.
Therefore, the diameter or the length of the longest chord of the given circle is 16 cm.

---

 

Worksheet on Addition and Subtraction of Polynomials will assist students to learn the concept of addition, subtraction of polynomials. Polynomials play a key role in laying the foundation for several topics related to algebra and students need to know about how to perform arithmetic operations on them.

Access the numeric questions available in the Adding and Subtracting Polynomials Worksheet that are well structured and practice using them on a regular basis. The Math Printable Worksheet for Adding and Subtracting Polynomials will develop logical and reasoning skills among you.

Read Similar Articles:

• Worksheet on Multiplying Monomial and Polynomial
• Worksheet on Multiplying Monomial and Binomial

Addition and Subtraction of Algebraic Expressions Worksheets with Answers

I. Simplify the following polynomial expressions:

(i) (10x²-5x+5)+(8x+10)
(ii) Take 3a + 9b – c from 7a + 12b – 4c

Solution:

(i) Given (10x²-5x+5)+(8x+10)
Arrange the like terms together and then add,
=10x²-5x+8x+5+10
=10x²+3x+15
Hence, By simplifying (10x²-5x+5)+(8x+10) we get 10x²+3x+15.
(ii) Take 3a + 9b – c from 7a + 12b – 4c
i.e. we have to Subtract 3a + 9b – c from 7a + 12b – 4c.
=7a+12b-4c-(3a+9b-c)
=7a+12b-4c-3a-9b+c
=7a-3a+12b-9b-4c+c
=4a+3b-3c
Hence, By simplifying 3a + 9b – c from 7a + 12b – 4c we get 4a+3b-3c.

---

II. The sum of two expressions is 5x² – 4xy + 2y². If one of them is 3x² + y², find the other?

Solution:

Given,
The sum of the two expressions is= 5x² – 4xy + 2y²
one of the expression is=3x² +y²Let the other expression be p(x).
3x² + y²+P(x)=5x² – 4xy + 2y²
p(x)=5x² – 4xy + 2y²-(3x² + y²)
p(x)=5x² – 4xy + 2y²-3x² – y²
=2x² -4xy+y²
Therefore, the other expression is 2x² -4xy+y².

---

III. Evaluate the following expressions
i) ( 15a² + 10a − 6a ) + (25a + 10)
ii) On adding 14a² +8𝑎⁴ with 3a²+ 2a⁴+4

Solution:

i) Given ( 15a² + 10a − 6a ) + (25a + 10)
=15a² + 10a − 6a+25a+10
=15a²+29a+10
Hence, By adding ( 15a² + 10a − 6a ) + (25a + 10) we get 15a²+29a+10.
ii) add 14a² +8𝑎⁴ ,3a²+ 2a⁴+4
=14a² +8𝑎⁴+3a²+ 2a⁴+4
=17a²+10𝑎⁴+4
Hence, By adding 14a² +8𝑎⁴ ,3a²+ 2a⁴+4 we get 17a²+10𝑎⁴+4.

---

IV. Subtract the following polynomial expression
(-150a²-54a+5)-(34a²+66a)

Solution:

Given, (-150a²-54a+5)-(34a²+66a)
=-150a²-54a+5-34a²-66a
=-184a²-120a+5
Hence, By subtracting 34a²+66a from -150a²-54a+5 we get -184a²-120a+5.

---

V. Solve the following

i) How much is 4x + 2y greater than x – 2y?
ii) How much should p + 2q – r be increased to get 5p+q?

Solution:

i) Subtract x-2y from 4x+2y.
=4x+2y-(x-2y)
=4x+2y-x+2y
=4x-x+4y
=3x+4y
Hence, 4x + 2y greater than x – 2y by 3x+4y.
ii) Let p + 2q – r be increased by x.
p+2q-r+x=5p+q
x=5p+q-(p+2q-r)
=5p+q-p-2q+r
=4p-q+r
Hence, p + 2q – r be increased to 4p-q+r to get 5p+q.

---

VI. From the sum of 5x + y – 8z and 2y – 4z, subtract 2x – y – 3z.

Solution:

Sum of 5x + y – 8z and 2y – 4z is
=5x + y – 8z+2y-4z
=5x+3y-12z
Subtract 2x – y – 3z from 5x+3y-12z
=5x+3y-12z-(2x – y – 3z)
=5x+3y-12z-2x+y+3z
=3x+4y-9z
Hence, By subtracting 2x – y – 3z from the sum of 5x + y – 8z and 2y – 4z we get 3x+4y-9z.

---

VII. Subtract 2x – 3y – z from the sum of 6x – 7y + 2z and 2x + y – 4z.

Solution:

Sum of 6x – 7y + 2z and 2x + y – 4z is=6x-7y+2z+2x+y-4z
=6x+2x-7y+y+2z-4z
=8x-6y-2z
Now, Subtract 2x – 3y – z from 8x-6y-2z.
=8x-6y-2z-(2x-3y-z)
=8x-2x-6y+3y-2z+z
=6x-3y-z
Hence, By subtracting 2x – 3y – z from the sum of 6x – 7y + 2z and 2x + y – 4z is 6x-3y-z.

---

VIII. By how much 8a² – 2b² be diminished to give 3a² + b²?

Solution:

Let the value be x.
x-(8a² – 2b²)=3a² + b²
x=3a² + b² +(8a² – 2b²)
x=11a²-b²
Therefore, 11a²-b² be diminished to give 3a² + b².

---

IX. Subtract m² – 5n + 7 from 4m² + 2n and add your result to m²+ n – 1.

Solution:

Subtract m² – 5n + 7 from 4m² + 2n
=4m² + 2n-(m² – 5n + 7)
=4m² + 2n-m² + 5n -7
=4m²-m²+2n+5n-7
=3m²+7n-7
Add 3m²+7n-7 to m²+ n – 1.
=3m²+7n-7+m²+ n – 1
=4m²+8n-8
Therefore, the sum of 3m²+7n-7 and m²+ n – 1 is 4m²+8n-8.

---

x. Subtract 2m²n – 3n² from 6m²n– 2mn²+ 8n² and subtract your result from the sum of the two expressions 3mn² + 5n² – 2m²n and m²n + 5m² – 2mn².

Solution:

Subtract 2m²n – 3n² from 6m²n– 2mn²+ 8n²
=6m²n– 2mn²+ 8n²-(2m²n-3n²)
=6m²n-2m²n-2mn²+8n²+3n²
=4m²n-2mn²+11n²
The sum of the two expressions 3mn² + 5n² – 2m²n and m²n + 5m² – 2mn² is
= 3mn² + 5n² – 2m²n + m²n + 5m² – 2mn²
=3mn²-2mn²+5n²-2m²n + m²n + 5m²
=mn²– m²n +5m² + 5n²
Subtract 4m²n-2mn²+11n²  from mn²– m²n +5m² + 5n²
=mn²– m²n +5m² + 5n²– (4m²n-2mn²+11n²)
=mn²+2mn²-m²n-4m²n+5m²+5n²-11n²
=3mn²-5m²n+5m²-6n²
Therefore, the result is 3mn²-5m²n+5m²-6n².

---

 

Worksheet on Multiplying Monomial and Polynomial will assist students in learning the multiplication of algebraic expressions involving Monomial and Polynomial. Learn the necessary math skill so that you will find it easy while solving complex algebraic expressions. Multiplication of Monomial and Polynomial Worksheet will offer step by step explanation for all the problems within so that you will get to know the algebraic methods used in solving the polynomial and monomial multiplication expressions.

Multiplying Monomial and Polynomial Worksheet with Answers will guide you in having an in-depth understanding of the concept as well get grip on the rules associated with it. Attain and Fluency by answering the questions from the Free Printable Math Multiplication of Monomial and Polynomial Worksheet on a frequent basis.

Do Check:

• Worksheet on Multiplying Binomials
• Worksheet on Dividing Monomials

Multiplication of a Monomial and Polynomial Worksheet

I. Multiply monomial by polynomial:
(i) 4x and (2x – 3y + 5z)
(ii) (-5m) and (3m – 4n + 2p)
(iii) 6xyz and (-7xy – 2yz – zx)
(iv) 7a³b²c² and (4a²b – 2a³c² – b³c)
(v) (-2x³y²z⁴) and (4x⁴y³ – 3x³y²z³ – 6xy²z²y)

Solution:

(i) Given 4x and (2x – 3y + 5z)
Multiply the monomial with every term of the polynomial
=4x(2x)-3y(4x)+5z(4x)
=8x²-12xy+20xz
Hence, By multiplying 4x and (2x – 3y + 5z) is 8x²-12xy+20xz.
(ii) Given (-5m) and (3m – 4n + 2p)
Multiply the monomial with every term of the polynomial
=(-5m)(3m) -4n(-5m)+ 2p(-5m)
=-15m²+20mn-10pm
Hence, By multiplying (-5m) and (3m – 4n + 2p) we get -15m²+20mn-10pm.
(iii) Given 6xyz and (-7xy – 2yz – zx)
Multiply the monomial with every term of the polynomial
=6xyz(-7xy)-2yz(6xyz)-zx(6xyz)
=-42x²y²z-12xy²z²-6x²yz²
Hence, By multiplying 6xyz and (-7xy – 2yz -zx) we get -42x²y²z-12xy²z²-6x²yz².
(iv) Given 7a³b²c² and (4a²b – 2a³c² – b³c)
Multiply the monomial with every term of the polynomial
=4a²b(7a³b²c²)-2a³c²(7a³b²c² )-b³c(7a³b²c²)
=28a⁵b³c²-14a⁶b²c⁴-7a³b⁵c³
Hence, By multiplying 7a³b²c² and (4a²b – 2a³c² – b³c)  we get 28a⁵b³c²-14a⁶b²c⁴-7a³b⁵c³  .
(v) Given (-2x³y²z⁴) and (4x⁴y³ – 3x³y²z³ – 6xy²z²)
Multiply the monomial with every term of the polynomial
=4x⁴y³((-2x³y²z⁴) -3x³y²z³(-2x³y²z⁴) -6xy²z²(-2x³y²z⁴)
=-8x⁷y⁵z⁴+6x⁶y⁴z⁷+12x⁴y⁴z⁶
Hence, By multiplying (-2x³y²z⁴)  and (4x⁴y³ – 3x³y²z³ – 6xy²z²)  we get -8x⁷y⁵z⁴+6x⁶y⁴z⁷+12x⁴y⁴z⁶.

---

II. Multiply polynomial by monomial:
(i) (m+ m⁴ + 1) and 5m
(ii) (ax² + bx³ + 5x) and x²
(iii) (2x + xz + z³) and 7z
(iv) (m – 2mn + 8n) and (–m⁷)
(v) (a + 2bc + ca) and (-a)

Solution:

(i) Given, (m+ m⁴ + 1) and 5m
Multiply the monomial with every term of the polynomial
=m(5m) + m⁴ (5m) + 1(5m)
=5m²+5m⁵+5m
Therefore, By multiplying (m+ m⁴ + 1) and 5m we get 5m²+5m⁵+5m.
(ii) Given, (ax² + bx³ + 5x) and x²
Multiply the monomial with every term of the polynomial
=ax²(x²) + bx³(x²) + 5x(x²)
=ax⁴+bx⁵+5x³
Therefore, By multiplying (ax² + bx³ + 5x) and x² we get ax⁴+bx⁵+5x³.
(iii) Given, (2x + xz + z³) and 7z
Multiply the monomial with every term of the polynomial
=2x(7z) + xz(7z) + z³ (7z)
=14xz + 7xz² + 7z⁴
Therefore, By multiplying (2x + xz + z³) and 7z is 14xz + 7xz² + 7z⁴.
(iv) Given, (m – 2mn + 8n) and (–m⁷)
Multiply the monomial with every term of the polynomial
=m(–m⁷) – 2mn(–m⁷) + 8n(–m⁷)
=-m⁸+2m⁸n-8m⁷n
Therefore, By multiplying (m – 2mn + 8n) and (–m⁷) we get -m⁸+2m⁸n-8m⁷n.
(v) Given,(a + 2bc + ca) and (-a)
Multiply the monomial with every term of the polynomial
=a(-a)+2bc(-a)+ca(-a)
=-a²-2abc-a²c
Therefore, By multiplying (a + 2bc + ca) and (-a) we get -a²-2abc-a²c.

---

III. Find the product of the following:
(i) 3ab(2ab + b²c + 5ca)
(ii) (-13m²)(5 + mx + ny)
(iii) 2m²n(mn + n – n²)
(iv) mn(m²+n²)
(v) -6a²bc(3ab + bc – 7ca)
(vi) (x+3y)(2x+6y)

Solution:

(i) Given, 3ab(2ab + b²c + 5ca)
Multiply the monomial with every term of the polynomial
=3ab(2ab)+b²c(3ab) + 3ab(5ca)
=6a2b2 + 3ab3c + 15a2bc
Hence, By multiplying 3ab(2ab + b²c + 5ca) we get 6a2b2 + 3ab3c + 15a2bc.
(ii) Given, (-13m²)(5 + mx + ny)
Multiply the monomial with every term of the polynomial
=(-13m²)5 + mx((-13m²) + ny(-13m²)
=-65m²-13m3x-13m2ny
Hence, By multiplying (-13m²)(5 + mx + ny) we get -65m²-13m3x-13m2ny.
(iii) Given, 2m²n(mn + n – n²)
Multiply the monomial with every term of the polynomial
=2m²n(mn) + n(2m²n)-n²(2m²n)
=2m3n2+2m2n2-2m2n3
Hence, By multiplying 2m²n(mn + n – n²) we get 2m3n2+2m2n2-2m2n3.
(iv) Given, mn(3m²+2n²)
Multiply the monomial with every term of the polynomial
=mn(3m²) + mn(2n²)
=3m3n + 2mn3
Hence, By multiplying mn(m²+n²) we get 3m3n + 2mn3.
(v) Given, -6a²bc(3ab + bc – 7ca)
Multiply the monomial with every term of the polynomial
=-6a²bc(3ab) + bc(-6a²bc) -7ca(-6a²bc)
=-18a3b2c-6a2b2c2+42a3bc2
Hence, By multiplying -6a²bc(3ab + bc – 7ca) we get -18a3b2c-6a2b2c2+42a3bc2.
(vi) Given, (x+3y)(2x+6y)
By using the distributive property, multiply the polynomials,
=x(2x+6y) + 3y(2x+6y)
=2×2+6xy+6yx+18y2
=2×2+12xy+18y2
Hence, By multiplying (x+3y)(2x+6y) we get 2×2+12xy+18y2.

---

IV. The product of two numbers is 3m⁴ if one of them is 1/4m². Find the other?

Solution:

Given,
The product of two numbers is =3m⁴
one of the number=1/4m²
Let the other number be x.
1/4m² × x=3m⁴
x=3m⁴.4m²
x=12m⁶
Therefore, the other number is 12m⁶.

---

V. If P=5x²+2x, Q=2x, R=24. Find the value of (P × R)/Q.

Solution:

Given P=5x²+2x, Q=2x+2, R=24
P × R=24(5x²+2x)
=120x² + 48x
(P × R)/Q= (120x² + 48x)/2x
=60x+24
Hence, the value of (P × R)/Q is 60x+24.

---

VI. If the length and width of the rectangle are (-4a²+7) and (2a + 5) respectively. Find the area of the rectangle?

Solution:

Given,
The length of the rectangle=(-4a²+7)
The breadth of the rectangle=(2a + 5)
Area of the rectangle=length * breadth
=(-4a²+7) * (2a + 5)
=-4a²(2a+5) + 7(2a+5)
=-8a3-20a2+14a+35

---

 

Estimating numbers is an approximation that we use in our daily life, it makes a number simple but keeping its value to what it was. Significant figures or Estimating numbers is the basic concept that your child needs to learn at the primary level itself. The result of an estimate is less accurate and easier to use especially while doing arithmetical operations.

Likewise, rounding a number to the nearest thousand means making the units and tens, hundreds place zeros and either increasing or decreasing the remaining part of the number by 1. Make your calculations easy by changing the given number to the nearest values. In this article, students can learn about Estimate to Nearest Thousand Definition, Rules, how to estimate a number nearest to 1000, Solved Example problems, and so on.

Read More:

• Estimate to Nearest Tens
• Estimate to Nearest Hundreds
• Estimating Sums and Differences

Estimate to Nearest Thousands – Definition

Estimate to Nearest 1000 is a process where you need to convert any given decimal number into the nearest thousandth value. But the obtained number is not the original number, it is an approximate value of the original number. Estimating to the nearest thousand means writing the nearest 1000 of the given number. In the decimal system thousand means (1/1000) or 0.001. Thousand is a place, which is located at the fourth position.

Rules for Estimation of Numbers to Nearest Thousands

There are 2 different rules for estimating the nearest thousand. The following are the 2 rules:

• Rule 1: To Estimate to the nearest 1000, if the digit in the hundreds place is between 0 to 4 or less than 5, then the hundreds place, tens place, and units place digits are replaced by 0.
• Rule 2: If the digit in the hundred’s place is equal to 5 or greater than 5, then the hundreds place digit, tens place digit, and units place digit is replaced by 0, and a thousand places are increased by 1.

How to Estimate a Number to Nearest 1000?

Follow the simple and easy steps provided below for an estimate of the nearest Thousands. They are in the following fashion

• First, we have to identify the number you want to round and mark the digit in the hundredths column.
• To round a digit to nearest hundreds place.
• If the digits in the hundreds place are 0, 1, 2, 3, 4, then replace the value in the hundreds place, tens place, and units place as 1 and keep remaining as it is.
• If the digits in the hundreds place are 5, 6, 7, 8, or 9, then place zero’s in the hundreds place, tens place, units place and increase the thousands place digit by one.
• Now, write the obtained number that is estimated to nearest 1000.

Explore all other Math Concepts similar to Estimation, Rounding Off Numbers all under one roof and practice the activity sheets, practice problems, worksheets, etc. to get a good hold of them.

Round to the Nearest Thousands Examples

Problem 1:
The following are the numbers to estimate nearest to 1000’s,
(i) 6241
(ii) 17692
(iii) 45620

Solution:
The values are given in the question,
Now, we have to round the nearest thousands of the values.
(i)6241
The value is 6241, hundred’s place digit is 2 means that is less than 5. So, we have to replace the hundreds place digit, ten’s place digit, and units place digit with 0.
So, after replacing digits the value is 6000.
Therefore, 6241 is estimated to nearest 1000’s is 6000.
(ii) The number is 17692
We can see that the digit in hundreds place is 6 which is greater than 5. So, we have to replace the hundreds place, tens place, units place digits with 0 and increase the thousands place digit by one.
So, after replacing digits the value is 18000.
Thus, 17692 is estimating nearest to 1000 is 18000.
(iii) The given number is 45720
In this, the tens place digit is 7, which is greater than 5. So, we will place zeros in the hundreds place, tens place, units place, and increase the thousands place digit by one.
So, the obtained value is 46000.
Hence, the estimating nearest 1000 is 46000.

Problem 2:
To estimate the number to the nearest Thousand. The number is 12.8562
Solution: 
As given in the question, the value is 12.8562
Now, we have to round the nearest 1000 of the given number.
First, we have to identify the hundreds place digit, which is 5, which is equal to 5. So, we have to replace zero in hundreds place digit, tens place digit, units place digit, and increase by 1 of the thousands place digit.
So, the number is 12.8562 after replacing and increasing the value is 12.9000
Thus, the rounding-off of 12.8562 to the nearest thousand is 12.9000.

Problem 3: 
What is the value of 4555 Estimating of nearest 1000?

Solution: 
The value 4555 is given in the question.
Now, we have to write the nearest thousand value.
So, we have to choose the two multiplies of 1000 just greater than and just less than 4555.
The nearest thousands of 4555 are 4000, 5000.
Then the value is,
4555 – 4000 = 555
5000 – 4555 = 445
As 5000 has the lowest difference value.
Therefore, 5000 is estimated to nearest 1000 for 4555.

Problem 4:
Find the value 18,675 estimated to nearest thousand?
Solution: 
The given number is 18,675
Now, we have to find the nearest thousand value of that number.
First, we can identify the digit in the hundreds place that is 6, which is greater than 5. So, we have to replace the value in the hundreds place, tens place, units place digit with zero and increase by 1 in the thousands place digit.
So, the value is 18,675
After replacing the value is 19,000
Hence, the value of 18,675 is nearer to thousands is 19,000.

FAQ’s on Estimate to Nearest Thousands

1. What is meant by estimate to nearest thousands?
Estimate to nearest thousand means the estimate of any decimal number to its nearest thousandth value. In decimal numbers, the thousandth means 1/1000 (or) 0.001. It is located in the fourth position.

2. How many rules are there for estimating nearest to thousands?
There are 2 different rules for estimating nearest to thousands.

3. How do you estimate to the nearest thousand?
 To estimate a number to the nearest thousand, first look at hundreds place digit. If the digit is less than equal to 4 then replace zero with the last 3digits. If the hundreds place digit is greater than 5, then replace the digits at hundreds, tens, units place digit and increase by 1 in the thousands place digit.

Do you know what is a cube? Have you find a cube for any number! If not, let us find how to calculate cubes for numbers. Basically, a cube is a number acquired by multiplying the number three times. Thus, the number(x) becomes x³ or x- cube. There is a difference between cube and cube root whereas cube root is the reverse process of the cube of a number and it is denoted by ∛.

Usually, in mathematics, we run mostly with squares and cubes of numbers in arithmetic operations. On this page, 5th Grade Math students will go through more about the cube of numbers, a perfect cube, a cube of negative numbers, and cubes of rational numbers, etc.

Do Check:

• Volume of Cubes and Cuboids
• Worksheet on Volume of a Cube and Cuboid

Cube – Definition

When an integer is multiplied by three times itself and the resultant product of a number is its cube number. If you consider a number n, then the formula of a cube of a number n is n × n × n = n³ because n is a natural number. In other words, we can say a number raised its exponent by 3 is called the cube of a number. For example, to find the cube of a number 5, we write as 5 × 5 × 5 = 125 and says as the cube of number 5 is 125.

We can also write a cube of the number 6 as 6³ in the exponent form and read 6- cubed. For example, to find 7³, first, we calculate 7 × 7 = 49 and next we calculate 49 × 7 = 343. Thus, we say that 343 is the cube of a number 7. It is to be emphasized that the number obtained using a cube formula is the perfect cube number. The cube is also known as the number which is calculated by its square.

Cube of a Number Examples

(i) 2³ = (2 × 2 × 2) = 8, here 8 is the cube of 2.
(ii) 3³ = (3 × 3 × 3) = 27, here 27 is the cube of 3.
(iii) 4 × 4 × 4 = 64, here 64 is the cube of 4.

Perfect Cube

A perfect cube is a number that is similar to the number, multiplied by itself three times or with three same or equal integers. If m is a perfect cube of n, then m = n³. Thus, if we take the cube root of a perfect cube, we get a natural number but not any fraction number. Therefore, ∛m = n. For example, 8 is a perfect cube because ∛8 = 2.

For instance, 125 is a perfect cube because 5³ = 5 × 5 × 5 = 125 where 123 is not a perfect cube because there is no number that comes when the number multiplied three times gives the product 123. The following table shows the perfect cubes of the first 10 natural numbers.

Cubes of Negative Integers

Cube of any negative integer will always be a negative integer, where the cube of any positive number results always a positive number, there a negative integer product will always give a product of negative integer because when a negative number is calculated by the same number three times, it results in a negative number.

Examples of a cube of negative integers when a number is calculated thrice are as such;
(-1)³ = (-1) × (-1) × (-1) = -1,
(-2)³ = (-2) × (-2) × (-2) = -8
(-3)³ = (-3) × (-3) × (-3) = -27, etc.

Cubes of Numbers from 1 to 25 Chart

The below table gives the cube values from 1 to 25 numbers along with their notations. These numbers will help the children in solving the numerical problems accurately.

Number	Multiplying Three Times Itself	Cube of a Number (x3)
1	1× 1× 1	1
2	2× 2× 2	8
3	3× 3× 3	27
4	4× 4× 4	64
5	5× 5× 5	125
6	6× 6× 6	216
7	7× 7× 7	343
8	8× 8× 8	512
9	9× 9× 9	729
10	10× 10× 10	1000
11	11× 11× 11	1331
12	12× 12× 12	1728
13	13× 13× 13	2197
14	14× 14× 14	2744
15	15× 15× 15	3375
16	16× 16× 16	4096
17	17× 17× 17	4913
18	18× 18× 18	5832
19	19× 19× 19	6859
20	20× 20× 20	8000
21	21× 21× 21	9261
22	22× 22× 22	10648
23	23× 23× 23	12167
24	24× 24× 24	13824
25	25× 25× 25	15625

Properties of a Cube

The following are some properties of a cube of numbers where students should remember while doing any cube for an integer.
(i) The cube of even natural numbers is always even.
(ii) The cube of odd natural numbers is always odd.
(iii) The sum of the cubes of first n natural numbers is equal to the square of their sum.
(iv) Cubes of the numbers ending in digits 1, 4, 5, 6, and 9 are the numbers ending in the same digit. For example, 1³=1, 4³=64, 5³=125, 6³=216, and 9³=729.

Cubes of Numbers 1 to 50 PDF Download

The following list helps the children while solving the problems with arithmetic operations. Children can refer to this list of cubes 1 to 50 to solve the problems accurately.

Examples of Cube of Numbers

Example 1: 
Find the cube of each of the following numbers
(i) 55    (ii) -41    (iii) 3.5
Solution: 
(i) Given number is 84
To get the cube of 84, we use the formula
n³ = n × n × n
55 = 55 × 55 × 55 = 1,66,375
Hence, the cube of the number 55 is 1,66,375.

(ii) Given number is -41
The number given is a negative integer and the resultant answer is also a negative integer because it is calculated three times and gives a negative integer.
-41 = -41 × -41 × -41 = -68,921.
Thus, the cube of a number -41 is -68,921.

(iii) 3.5 is a given number
When a decimal number is cubed we convert the decimal number into a fraction number.
(3.5)³ = (35/10)³
= (7/2)³
= 7³/2³
= (7× 7× 7)/(2× 2× 2)
= 343/8.

Example 2: 
What is the value of x if x³ = 64?
Solution: 
The formula to calculate the cube is
x³ = x × x × x
Here, x³ = 64
x = ∛64 = 4
Therefore, x=4.

Example 3: 
Find out the cube numbers 7 and 12. Also, find the sum of the cube numbers?
Solution: 
Firstly, we find the cubed numbers 7 and 12.
The cube number 7 is 7 × 7 × 7 = 343.
The cube number 12 is 12 × 12 × 12 = 1728.
Now, we have to find the sum of the cubed numbers.
i.e., 7³ + 12³ = 343 + 1728 = 2071.
As a result, Sum of the cube numbers is 2,071.

FAQ’s on Cubes

1. What is a Cube?
A cube is a number where it is multiplied thrice by itself. The symbol for the cubed is ³. For example, to get 5³ we multiply 5 three times itself i.e., 5 × 5 × 5 = 125.

2. What are the cube numbers from 1 to 10?
The cubed numbers from 1 to 10 are 1, 8, 27, 64, 125, 216, 343, 512, 729, and 1000.

3. Is 600 is a perfect cube?
To know 600 is a perfect cube or not. We have to find the multiplies of 600.
The multiplies of 600 are

2× 2× 2× 3× 5× 5 are multiplies of 600.
2³ × 3 × 5² = 600.
Therefore, 600 is not a perfect cube because all the factors are not multiple of three.

4. Which are the perfect cubes among the numbers 343, 576, 2197?
Among the given numbers 343 and 2197 are the perfect cubes because 7× 7× 7 = 343 and 13× 13× 13 =2197. Whereas 576 is the perfect square number i.e., 24× 24 = 576.

A quadratic equation has two solutions that may be or may not be distinct. The result may be real numbers or imaginary numbers. Learn the important formulas of quadratic equation, definition here. Let us learn about the introduction to the quadratic equations from this article. You can find examples of quadratic equations with step-by-step explanations.

What is a Quadratic Equation?

In the name, quadratic “quad” means square because the equation is square. A quadratic equation is an algebraic expression of the 2nd degree in variable x. The variable x has two answers real or complex numbers. The answers or solutions of x are called roots of the quadratic equations. They are specified as (α, β). The standard form of the quadratic equation is ax² + bx + c = 0. Where a, b is the coefficient of x² and c is the constant. a,b, c are not fractions nor decimals.

Quadratic Equation Formula

The formula for the quadratic equation is an easy method to find the roots of the equation. Without the formulas, the values are not factorized and can find the roots in the easiest way. The roots of Q.E helps to find the sum of the roots and product of the roots of the quadratic equation.
Quadratic Equation (α, β) = [-b ± √(b² – 4ac)]/2a.

Important Formulas to Solve Quadratic Equations

• The standard form of the quadratic equation is ax² + bx + c = 0.
• The discriminant(D) of quadratic equation is D = b² – 4ac.
• For the case, D = 0 the roots are real and equal.
• For the case, D > 0 the roots are real and distinct.
• For the case, D < 0 the roots do not exist, or the roots are complex.
• The product of the Root of the quadratic equation is αβ = c/a = Constant term/ Coefficient of x²
• The roots of the quadratic equation is x = [-b ± √(b² – 4ac)]/2a.
• The sum of the roots of a Q.E is α + β = -b/a = – Coefficient of x/ Coefficient of x²
• Quadratic equation in the form of roots is x² – (α + β)x + (αβ) = 0
• If α, β, γ are roots of a cubic equation ax³ + bx² + cx + d = 0, then, α + β + γ = -b/a, αβ + βγ + λα = c/a, and αβγ = -d/a
• The roots (α + iβ), (α – iβ) are the conjugate pair of each other.
• For a > 0, the quadratic expression f(x) = ax² + bx + c has a minimum value at x = -b/2a
• For a < 0, the quadratic expression f(x) = ax² + bx + c has a maximum value at x = -b/2a
• For a > 0, the range of the quadratic equation ax² + bx + c = 0 is [b² – 4ac/4a, ∞).
• For a < 0, the range of the quadratic equation ax² + bx + c = 0 is (∞, -(b² – 4ac)/4a]

Methods for Solving Quadratic Equations

There are three methods for solving quadratic equations. They are as follows,
1. Factorization method
2. Completing the square method
3. Quadratic Equation formula

Quadratic Equation Examples

Example 1.
Solve 5x² + 7x + 2 = 0
Solution:
Coefficients are: a = 5, b = 7, c = 2
x = [-b ± √(b² – 4ac)]/2a
x = [-7 ± √(7² – 4.5.2)]/2.5
x = [-7 ± √(49 – 40)]/10
x = [-7 ± √(9)]/10
x = [-7+3]/10 = -4/10 = -2/5
x = [-7 – 3]/10 = -10/10 = -1
Thus x = -2/5 or x = -1

Example 2.
Find the range of k for which 4 lies between the roots of the quadratic equation x² + 2(k – 4)x + 5 = 0.
Solution:
6 will lie between the roots of the quadratic expression f(x) = x² + 2(k – 4)x + 5 if,
f(4) < 0
= 16 + 2(k – 4)4 + 5 < 0
= 16 + (2k – 8)4 + 5 < 0
= 16 + 8k – 32 + 5 < 0
= 8k – 11 < 0
= k < 11/8

Example 3.
Find the factors of the quadratic equation x² + 7x + 12 = 0
Solution:
x² + 7x + 12 = 0
x² + 3x + 4x + 12 = 0
x(x + 3) + 4(x + 3) = 0
(x + 4) (x + 3) = 0
x + 4 = 0 or x + 3 = 0
x = -4 or x = -3

FAQs on Quadratic Equation

1. What is the purpose of quadratic equations?

Quadratic equations are actually used in our daily life, as when calculating areas, determining a product’s profit or formulating the speed of an object.

2. What is the standard form of the quadratic equation?

The standard form of the quadratic equation is ax² + bx + c = 0

3. How many roots does a quadratic equation have?

The quadratic equation has two roots. The Q.E with real or complex coefficients has two solutions that are called roots.

Practice using the Worksheet on Dividing Monomials and improve your algebra basics. You can solve different types of algebraic expressions much easily provided you have a grip on the Division of Monomials Concept. All the Problems in the Dividing Monomials Worksheet are designed with a simple approach so that you will become familiar with the concept step by step. The Free Printable Math Worksheet on Division of Monomials available in PDF Formats can be accessed for free of cost and you can practice regularly.

See More:

• Worksheet on Addition and Subtraction of Polynomials
• Worksheet on Multiplying Monomial and Binomial
• Worksheet on Multiplying Monomial and Polynomial

Dividing Monomials Worksheet with Answers

I. Divide the following monomials and write the answer in simplest form:
(i) (48xy) ÷ (4x)
(ii) (12p⁶q⁴) ÷ (-6p²q²)
(iii) (26m²pn²) ÷ (-2mn)
(iv) (-96x⁴y³) ÷ (-8x²y)
(v) (-56b²x³y⁵) ÷ (4bx²y²)
(vi) (48ab) ÷ (-4)
(vii) (75m²n) ÷ (5n)

Solution:

(i) Given monomials are 48xy,4x
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
48xy/4x=6 × 8 × x × y/2 × 2 × x
=24y/2
=12y
Therefore, By dividing (48xy) with (4x) we get 12y.

(ii) Given (12p⁶q⁴) ÷ (-6p²q²)
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
(12p⁶q⁴) / (-6p²q²)=2 × 6 × p × p ×p ×p × p ×p × q × q × q × q/-6 × p × p × q × q
=-2p⁴q²
Therefore, By dividing 12p⁶q⁴ with -6p²q² we get -2p⁴q².
(iii) Given (26m²pn²) ÷ (-2mn)
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
26m²pn²/-2mn=2 × 13 × m × m × p × n × n/-2 ×m ×n
=-13mpn
Therefore, By dividing 26m²pn² with -2mn we get -13mpn.

(iv) Given (-96x⁴y³) ÷ (-8x²y)
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
-96x⁴y³/8x²y=-12 × 8 × x × x × x × x × y × y × y/8 × x × x ×y
=-12 x²y²
Therefore, By dividing -96x⁴y³ with-8x²y we get -12 x²y².
(v) Given (-56 b²x³y⁵) ÷ (4bx²y²)
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
-56 b²x³y⁵/4bx²y²= -4 × 14 × b × b × x ×  x × x × y × y × y × y × y/4 × b × x ×  x ×  y ×  y
=-14bxy³
Therefore, By dividing -54b²x³y⁵ with 4bx²y² we get -14bxy³.
(vi) Given (48ab) ÷ (-4)
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
48ab/-4=12 × 4 × a × b/-4
=-12ab
Therefore, By dividing 48ab with -4 we get -12ab.
(vii) Given (75m²n) ÷ (5n)
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
75m²n/5n= 15 × 5 × m × m × n/5 × n
=15m²
Therefore, By dividing 75m²n with 5n we get 15m² .

---

II. Divide a monomial by a monomial:
(i) 60a² ÷ 5a
(ii) 63x²y² ÷ 7xy
(iii) 48a⁴b⁶ ÷ 12a²b²
(iv) 108m⁴n³ ÷ (-18m²n²)
(v) (-46a⁴b⁵c²) ÷ (-23a²bc)

Solution:

(i) Given, 60a² ÷ 5a
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
60a²/5a= 12 × 5 × a × a/5 × a
=12a
Hence, By dividing 60a² with 5a we get 12a.
(ii) Given, 63x²y² ÷ 7xy
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
63x²y²/7xy=7 × 9 × x × x × y× y/7 × x × y
=9xy
Therefore, By dividing 63x²y² with 7xy we get 9xy.
(iii) Given, 48a⁴b⁶ ÷ 12a²b²
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
48a⁴b⁶/12a²b²= 12 × 4 × a × a × a × a × b × b × b × b × b × b/4 × 3 × a × a × b × b
=4a2b4
Therefore, By dividing 48a⁴b⁶ with 12a²b² we get 4a2b4.
(iv) Given, 108m⁴n³ ÷ (-18m²n²)
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
108m⁴n³/-18m²n²= 18 × 6 × m × m × m × m  × n × n × n/-6 × 3 × m × m × n × n
=-6m2n
Therefore, By dividing 108m⁴n³  with -18m²n² we get -6m2n.
(v) Given, (-46a⁴b⁵c²) ÷ (-23a²bc)
Now we have to write each term in expanded form and then cancel the terms which are common to both numerator and denominator.
-46a⁴b⁵c²/-23a²bc= -23 × 2 × a × a × a × a × b × b × b × b × b × c × c/-23 × a × a × b × b × c
=2a2b4c
Hence, By dividing -46a⁴b⁵c² with -23a²bc we get 2a2b4c.

---

III. Divide first monomial by the second monomial:
(i) 30xy, 6x
(ii) 75a⁴b, 5b
(iii) 48ab⁴c, 8b²c
(iv) 30a⁵, (-a²)
(v) 22a²b⁵, 2ab

Solution:

(i) Given 30xy, 6x
30xy/6x=5y
Hence, By dividing the first monomial 30xy with the second monomial 6x we get 5y.
(ii) Given 75a⁴, 5b
75a⁴b/5b=15a⁴
Therefore, By dividing the first monomial 75a⁴b with the second monomial 5b we get 15a⁴.
(iii) Given 48ab⁴c, 8b²c
48ab⁴c/8b²c=6ab²
Therefore, By dividing the first monomial 48ab4c with the second monomial 8b2c we get 6ab².
(iv) Given 30a⁵, (-a²)
30a⁵5/-a²=-30a³
Hence, By dividing the first monomial 30a⁵ with the second monomial -a² we get -30a³.
(v) Given 22a²b⁵, 2ab
22a²b⁵/ 2ab=11ab⁴
Therefore, By dividing the first monomial 22a2b5 with the second monomial 2ab we get 11ab⁴.

---

IV. Simplify the division of the monomials
(i) (-18x⁸) ÷ (-9x²)
(ii) (40a⁴b⁸c⁴) ÷ (5a²b²c²)
(iii) (14x⁴y³z²) ÷ (-2x²yz)
(iv) (81a⁷b⁸c³) ÷ (9a⁴b³3c³)
(v) (51x⁶y⁵z⁴) ÷ (-3x⁴y²z)

Solution:

(i) Given (-18×8) ÷ (-9x²)
-18x⁸/-9x²=2x⁶
Hence, By dividing -18x⁸ with -9x² we get 2x⁶.
(ii) Given (40a⁴b⁸c⁴) ÷ (5a²b²c²)
40a⁴b⁸c⁴/5a²b²c²=8a²b⁶c²
Hence, By dividing 40a⁴4b⁸c⁴ with 5a²b²c² we get 8a²b⁶c².
(iii) Given (14x⁴4y³z²) ÷ (-2x²yz)
14x⁴y³z²/-2x²yz=-7x²y²z
Hence, By dividing 14x⁴y³z² with -2x²yz we get -7x²y²z.
(iv) Given (81a⁷b⁸c³) ÷ (9a⁴b3c³)
81a⁷b⁸c³/9a4b3c³=9a³b⁵
Hence, By dividing 81a⁷b⁸c³ with 9a4b3c³ we get 9a³b⁵.
(v) Given (51x⁶y5z4) ÷ (-3x⁴y²z)
51x⁶y5z4/-3x⁴y²z=-17x²2y³z³
Hence, By dividing 51x⁶y5z4 with -3x⁴y²z we get -17x²2y³z³.

---