In this article, you have to learn about how to find the Circumference and Area of a circle. In other words, the circumference of a circle is also known as a Perimeter, it helps to identify the length of the outline of any shape. The circumference and area of a circle are two important parameters of a circle. A circle is made of multiple points arranged equidistant from a single central point and that point is called the center of the circle.
On this page, we will discuss definitions of circumference and area of a circle, formulas, methods to find the circle’s area and circumference with solved example problems, and so on.
Circumference and Area of Circle – Definition
Circumference of Circle: The circumference of a circle is the length of the boundary of the circle. The distance between the center and any point on the circumference is known as the radius of the circle, radius is a constant value. The maximum distance between any two points on the circumference is known as Diameter. It will be twice the radius.
Area of Circle: The area of a circle is defined as the region occupied by the shape in a two-dimensional plane. It means the area of a circle is the total area that is covered by one complete cycle of the radius of the circle on a 2D plane.
Area and Circumference of Circle Formulas
Circumference of a Circle: It is measured in units, centimeters, and etc. The formula for the circumference of a circle is,
Circumference = 2πr = πD
Where r is the radius of the circle and Pi is the constant value i.e. π = 3.14 or 22/7
Area of a Circle: The unit of the area is square units. The area of a circle formula is,
Area of a circle = πr².
Here r is the radius of the circle. π is a constant value that is 22/7 or 3.14
Read More:
- Geometrical Properties of a Square
- Worksheet on Circle
- Worksheet on Circumference and Area of Circle
Circumference and Area of Circle Examples
Example 1:
Find the area and circumference of a circle whose radius is 20 cm. Take the value of π is 3.14?
Solution:
As given in the question, the radius is 20cm.
Now, we will find the area and circumference of a circle.
We know the formulas of the area and circumference of a circle.
Area of a circle = πr2
Substitute the values in above formula, we get
Area = 3.14x(20)2 = 1256 cm2
Next, the Circumference of a circle, C = 2πr
Circumference = 2 × 3.14 × 20 = 125.6 cm
Hence, the area and circumference of a circle are 1256 cm2 and 125.6 cm
Example 2:
Find the area of a circle whose circumference is 14.6 cm?

Solution:
Given in the question, the circumference of a circle is 14.6 cm.
Now, to find out the area of a circle value, we must find the radius.
We all know, Area of a circle is πr2
From the circumference, we will calculate the radius.
Circumference = 2πr = 14.6 cm
(2)(3.14)r = 14.6
r = 14.6/(2)(3.14)
r = 4.4 cm
Therefore, the radius of the circle is 4.451 cm.
Now, substitute the given radius value in the area of a circle formula, we get
A = π(4.4)2
A = 3.14 x 19.36
A = 60.790 cm2
Therefore, the area of a circle is 60.790 cm2.
Example 3:
The ratio of radii of two circles is 5:9. Find the ratio of their circumferences?
Solution:
In the given question, the values are,
The radii ratio of the two circles is 5:9.
Now, we will find out the ratio of their circumference.
Consider the radius of the first circle be r1 and the radius of the second circle be r2.
We know the formula, circumference of a circle is 2πr.
Let, r1 = 5x and r2 = 9x.
The circumference of a 1st circle is 2πr₁
= 2π5x
= 10πx
Next, the circumference of the 2nd circle is 2πr₂
= 2π9x
= 18πx
Now, the ratio of their circumference
= 10πx/18πx
= 10/18
= 5/9
= 5:9
Therefore, the ratio of their circumference is 5:9.
Example 4:
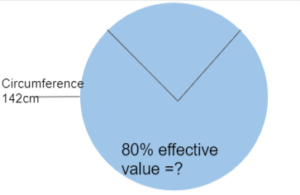
A circular radar screen has a circumference of 142 cm. Only 80% of its area is effective. Find the value of the effective area of the screen?
Solution:
Given in the question, the values are
The circumference of a circular radar screen is 142 cm.
Where r be the radius of the circular radar screen.
We know the formula, circumference = 2πr
2πr = 142
2 x 22/7 x r = 142
r6.28 = 142
radius of a circle = 142/6.28 = 22.6 cm
Next, find the area of a screen.
Area of a circle = πr2
So, the value is 22/7 x (22.6)2
3.14 x 510.76 = 1604 cm2
But, only 80% of the area of the radar screen is effective.
So, the effective area of the 80% of 1604cm2 is, 1604 x 80/100 = 1283.2 cm2
Example 5:
Find the area of a circle of circumference 43 in decimal. Let the π = 3.14?
Solution:
As given in the question, the circumference of a circle is 43.
Now, we will find the area of a circle value.
We know the formula, Area of a circle is πr2. Using circumference, the area of a circle formula is A = C2/4π.
Substitute the given values in the above formula, then the formula is
So, the area is C2/4π.
(43)2 /4(3.14) = 1849 / 12.56 = 147.2 cm2.
Thus, the area of a circle is 147.2 cm2.
FAQ’S on Area and Perimeter of a Circle
1. What are the formulas for calculating the area of a circle?
A. The area of a circle is calculated by using the below formulas:
In terms of radius, the Area of a circle is πr2, where r is a radius.
In terms of diameter, the Area of a circle is (π/4) x d2, where d is the diameter.
In terms of circumference, the Area of a circle is C2/4π, where c is the circumference.
2. What is the perimeter of a circle?
A. The perimeter of a circle means that the circumference of a circle, which is equal to twice the product of pi (π) and radius of the circle, i.e., 2πr.
3. If the radius of a circle is given in meters, then what will be the unit of the area of the circle?
A. The radius of a circle is in meters, then the area of a circle unit is square. meters.