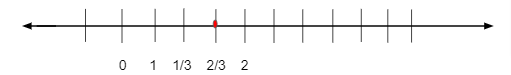
Every Number can be represented on Number Line. The same applies to Rational Numbers too and we can denote them on Number Line. We have mentioned some of the key points to remember while representing rational numbers on a number line. Go through them and get a clear idea of the concept. Practice the different problems on representation of rational numbers on number line and learn how to represent rational numbers on the number line. Solve them on a frequent basis and enhance your problem-solving skills and proficiency in the subject.
Do Refer:
- Representation of Rational Numbers on Number Line
- Problems on Rational Numbers as Decimal Numbers
- Problems Based on Recurring Decimals as Rational Numbers
Rational Numbers on Number Line Questions
Example 1.
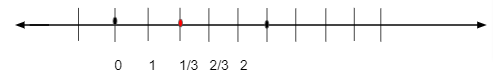
Represent 4/3 on Number Line?
Solution:
Given Rational Number is 4/3
Since the given rational number is positive we will represent it on the right side of the zero on the number line. To denote this improper fraction we will first convert it into a mixed fraction.
4/3 converted to mixed fraction = 1 1/3
Now this fraction will lie between 1 and 2. The Number Line between 1 and 2 is split into three equal parts. The first part of the division is the required representation of the fraction. It can be shown as
Example 2.
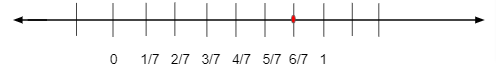
Represent 6/7 on Number Line?
Solution:
Given Rational Number is 6/7
Since the given rational number is positive we will represent it on the right side of the zero on the number line. To denote this proper fraction we will split the number into 7 equal parts between 0 and 1.
The sixth part of the division is the required representation of the fraction. It can be shown as
Example 3.
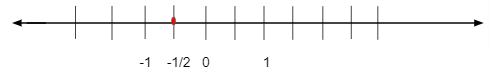
Place -1/2 on Number Line?
Solution:
Given Rational Number is -1/2
Since the given rational number is negative we will represent it on the left side of the zero on the number line. To denote this proper fraction we will split the number into 2 equal parts between 0 and -1.
The second part of the division is the required representation of the fraction. It can be shown as
Example 4.
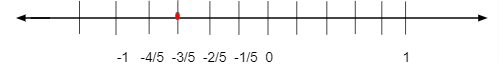
Represent -3/5 on Number Line?
Solution:
Given Rational Number is -3/5
Since the given rational number is negative we will represent it on the left side of the zero on the number line. To denote this proper fraction we will split the number line into 5 equal parts between 0 and -1.
The third part of the division is the required representation of the fraction. It can be shown as
Example 5.
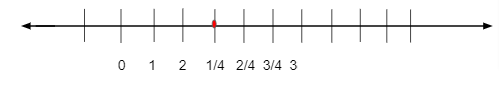
Represent 9/4 on Number Line?
Solution:
Given Rational Number is 9/4
Since the given rational number is positive we will represent it on the right side of the zero on the number line. To denote this improper fraction we will first convert it into a mixed fraction.
9/4 converted to mixed fraction = 2 1/4
Now this fraction will lie between 2 and 3. The Number Line between 2 and 3 is split into four equal parts. The first part of the division is the required representation of the fraction. It can be shown as
Example 6.
Represent 5/3 on Number Line?
Solution:
Given Rational Number is 5/3
Since the given rational number is positive we will represent it on the right side of the zero on the number line. To denote this improper fraction we will first convert it into a mixed fraction.
5/3 converted to mixed fraction = 1 2/3
Now this fraction will lie between 1 and 2. The Number Line between 1 and 2 is split into three equal parts. The second part of the division is the required representation of the fraction. It can be shown as