Problems on Distance Formula are available on this page. Distance between two points in coordinate geometry can be calculated using the distance formula. The distance formula can be found using the Pythagoras theorem. Learn how to find the distance between the points with the help of the distance formula.
Refer Similar Posts:
Distance Formula Problems with Solutions PDF
Example 1.
If the distance between the points (6, – 2) and (2, a) is 5, find the values of a.
Solution:
We know that,
The distance between (x1, y1) and (x2, y2)
is √(x1−x2)² + (y1−y2)²
Here, the distance = 5,
x1 = 6, x2 = 2, y1 = -2 and y2 = a
Therefore, 5 = √(6−2)² + (−2−a)²
25 = √(4)² + (-2-a)²
Squaring on both sides
25 = 16 + (2 + a)²
(2 + a)² = 25 – 16
(2 + a)² = 9
Taking square root, 2 + a = ±3
a = -2 +or- 3
a = (1, -5)
Example 2.
Find the value of a, if the distance between the points P(3, 6) and Q(3, a) is 10 units.
Solution:
Let the given points be:
P(3, 6) = (x1, y1)
Q(3, a) = (x2, y2)
Using distance formula,
Distance between the points P(3, 6) and Q(3, a) is:
[(3 – 3)² + (a – 6)²] = 10 units
Squaring on both sides of the equation,
(0)² + (a – 6)² = 100
(a – 6)² = 100
Taking root on both the sides, we get;
a – 6 = ±10
Case I: Considering +10,
a – 6 = 10,
a = 10 + 6 = 16
Case II: Considering -10
a – 6 = -10
a = -10 + 6
a = – 4
a = (16, -4)
Example 3.
Find a relation between x and y such that the point (x, y) is equidistant from the points (7, 1) and (3, 5).
Solution:
Let P(x, y) be the point which is equidistant from the points A(6, 1) and B(3, 5).
Given,
AP = BP
AP² = BP²
(x – 6)² + (y – 1)² = (x – 3)² + (y – 5)² (by distance formula)
x² – 12x + 36 + y² – 2y + 1 = x² – 6x + 9 + y² – 10y + 25
-12x + 37 – 2y + 6x + 10y – 34 = 0
-6x + 8y = – 3
This is the required relation between x and y
Example 4.
Find a point on the y-axis which is equidistant from points A(6, 5) and B(– 4, 3).
Solution:
We know that a point on the y-axis is of the form (0, y). So, let the point P(0, y) be equidistant from A and B. Then:
AP = BP
AP2 = BP2
(6 – 0)2 + (5 – y)2 = (– 4 – 0)2 + (3 – y)2
36 + 25 + y2– 10y = 16 + 9 + y2 – 6y
61 – 10y = 25 – 6y
10y – 6y = 61 – 25
4y = 36
y = 9
So, the required point is (0, 9).
Verification:
AP = √[(6 – 0)2 + (5 – 9)2]
= √(36+16)
= √52
BP = √[(-4-0)2+(3-9)2]
=√(16+36)
=√52
Hence, we conclude that the point (0, 9) is equidistant from the given two points.
Example 5.
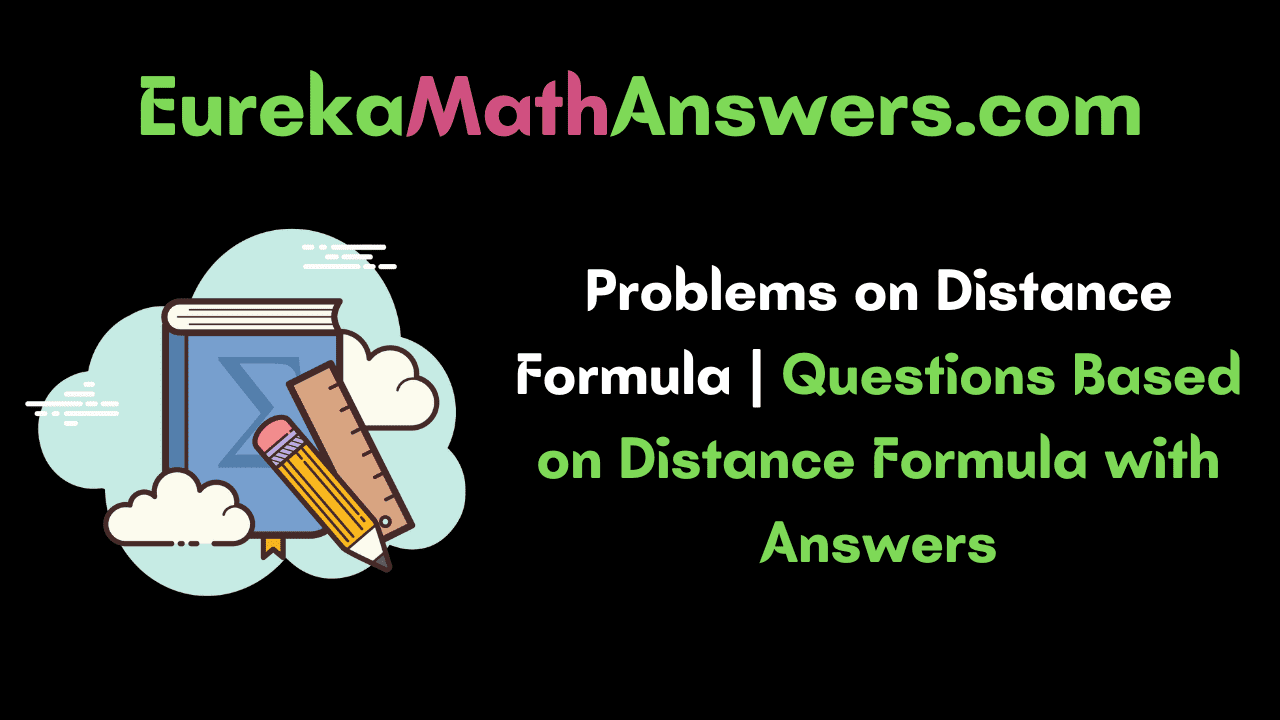
Find the distance between the points (1, 2) and (1, 5).
Solution:
The formula for the distance D between two points (a,b) and (c,d) is given by
D = √(c – a)² + (d – b)²
Apply the formula given above to find distance D between the points (1, 2) and(1, 5) as follows.
D = √(1 – 1)² + (5 – 2)²
√(0)² + (3)²
√0 + 9
√9 = 3
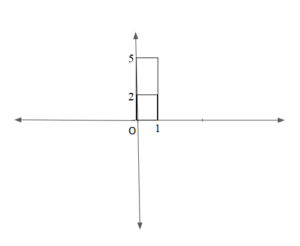
Example 6.
Find a relationship between x and y so that the distance between the points (3, 4) is equal to 12.
Solution:
We apply the distance formula
12 = √(3 – x)² + (4 – y)²
Square both sides
144 = (3 – x)² + (4 – y)²
The above relationship between x and y is the equation of the circle.
144 = 9 – 6x + x² + 16 – 8y + y²
144 = x² + y² – 6x – 8y + 25
x² + y² – 6x – 8y = 144 – 25
x² + y² – 6x – 8y = 119
Example 7.
Find the distance between the points (2,4) and (1,3)
Solution:
The given two points are (x1, y1) = (2,4) and (x2, y2) = (1,3).
Using the distance formula
√(x2 – x1)² + (y2 – y1)²
= √(1 -2)² + (3-4)²
=√(-1)² + (-1)²
= √1 + 1
= √2
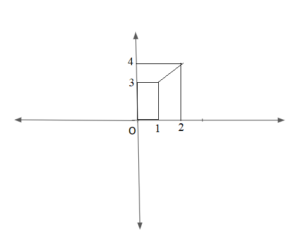
Therefore the distance between the points is √2.
Example 8.
Find the distance between the points (3,6) to the line 3x – 4y = 5
Solution:
The given point is (x1,y1) = (3,6)
The given line can be written has 3x – 4y – 5 = 0
Comparing this with ax + by + c = 0
We get a = 3 and b= -4, c = -5
Using the distance formula to find the distance from a point to aline
d = ax1 + by1 + c/√a² + b²
d = 3(3) – 4(6) -5/√3² + (-4)²
d = 9-24-5/√9 + 16
d = -20/√25
d = -20/5
The distance from the given point to the given line -20/5 units
Example 9.
Find the distance point (3,6) and the midpoint of the line segment joining (1,2) and (1,4).
Solution:
We first find the coordinates of the midpoint M of the segment joining (1,2) and (1,4).
We know that
Midpoint formula = [a1 + a2/2 , b1 + b2/2]
M = [1 + 1/2, 2 + 4/2]
M = [2/2, 6/2]
M = [1, 3]
We now use the distance formula to find the distance between the points (3,6) and (1,3)
D = √(a2 – a1)² + (b2 – b1)²
D = √(1 – 2)² + (6 – 4)²
D = √ (-1)² + (– 2)²
D = √1 + 4
D = √5
Therefore the distance is √5
Example 10.
The town network is mapped on a coordinate grid with the origin being at city hall. Rishi’s house is located at point (2,6) and Lora’s house is located at (1,4). How far is it from Rishi’s house to lora’s house?
Solution:
Using the distance formula
D = √(x2 – x1)² + (y2 – y1)²
D =√ (2 – 3)² + (5 – 4)²
D = √(-1)² + (1)²
D = √1 + 1
D = √2