The angle bisector of the triangle divides the angle opposite side into two segments proportional to the remaining sides of the triangle. The angle bisector divides the angles into two equal parts. Get to know more about the angle bisector theorem and how it divides angles into two equal measures. Check the proof for the angle bisector is equidistant from the arms of the triangle in the following sections.
Angle Bisector Theorem
An angle bisector is a line that divides the angle and triangle into two equal quantities. The main intention of an angle bisector states that any point on the bisector of an angle is equidistant from the sides of the angle and it divides the opposite side in the ratio of the adjacent sides.
The properties of triangle angle bisector are along the lines:
- The angle bisector can be drawn to any type of angle namely acute, obtuse or right angle.
- The angle bisector in a triangle divides the opposite side in the proportion of the other two sides.
- Any point of the angle bisector is equidistant from both angles and arms of the triangle.
Any Point on the Bisector of an Angle Proof
The following is the proof for any point on the bisector of an angle is equidistant from the arms of the angle.
- Let us take a triangle ABC.
- Draw angle bisector BZ that bisects ∠ABC.
- Draw two lines OL ⊥ AB, OM ⊥ BC.
So, we have to prove that OM = OL.
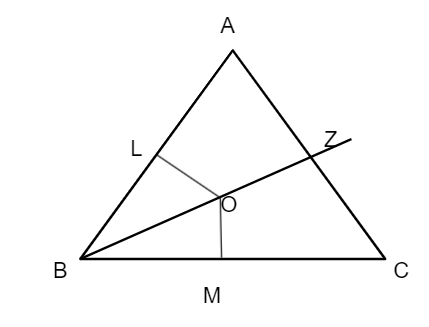
Now we can observe two triangles △BOL, △BOM
BZ bisects ∠ABC. So, ∠LBO = ∠OBM
It is given that, ∠BLO = ∠BMO = 90°
The common vertex for both triangles is OB. So, OB = OB
△BOL ≅ △BOM by the AAS criterion
So, OM = OL
Hence, proved.
More Related Articles:
- Properties of Angles of a Triangle
- Classification of Triangles
- Criteria for Congruency
- Geometry and Measurement
Example Questions on Angle Bisector
Question 1:
A line OB divides dives an angle PQR into two equal parts. If one part is 2x + 5 and the second part is 4x – 7, then what is the value of x?
Solution:
Given that,
OB divides angle PQR into two equal parts. Thus OB is the angle bisector.
Equate the two parts of angles.
2x + 5 = 4x – 7
4x – 2x = 5 + 7
2x = 12
x = 12/2
x = 6
Hence, the value of x is 6.
Question 2:
If an angle bisector divides an acute angle of 50°, then what is the measure of each angle?
Solution:
Given angle is 50°
We already know that the angle bisector divides the angle into two equal parts.
So, 50° is divided into two equal parts. say x
Hence, x + x = 50°
2x = 50°
x = 50°/2
x = 25°
Therefore, the angle bisector divides 50° into two 25°.
FAQ’s on Point on the Bisector of an Angle
1. What are the properties of an angle bisector in a triangle?
Every angle bisector has two propoerties. One is angle bisector divides the opposite side in the ratio of adjacent sides of the triangle. Second is any point on the bisector of an angle is equidistant from the arms of the angle.
2. Does an angle bisector divides an angle into two equal parts?
An angle bisector of a triangle divides the angle into two angles having equal measures.
3. How many angle bisectors do an angle has?
An angle has only one angle bisector.
4. What is meant by angle bisector?
An angle bisector is a ray that divides an angle into two equal measures. Each point on the angle bisector is equidistant from the sides of the nagle.