This article aids the children to gain more knowledge about Midpoint Theorem on Right-angled Triangle. Usually, we know a triangle is cited as a polygon that has three sides of three line segments. In other words, a triangle is just a closed figure where the sum of its angles is equal to 180 degrees. Every shape of a triangle is classified based on the angle made by the two adjacent sides of a particular triangle.
The right-angle triangle is a geometrical shape, it plays a prominent role, and is considered as a basis in trigonometry. On this page, we will be proving that in a right-angled triangle the median drawn to the hypotenuse is half the hypotenuse in length. Let us discuss more on right-angle triangles and prove the statement of the midpoint theorem on the right-angled triangles with a few examples.
Right-angled Triangle – Definition
A right-angled triangle is defined as if one of the angles of a triangle is a 90 degrees right angle, then the triangle is called a right-angled triangle or simply can call a right triangle. In a triangle, the relation between the various sides can be easily realized with the help of a Pythagoras rule. These right-angles triangles are of two types namely, isosceles right-angled triangle and a scalene right-angled triangle.
The side opposite to the right angle is called hypotenuse and it is the largest side of the right-angle triangle. In the following image, triangle ABC is a right triangle, with three sides namely, the base, the altitude, and the hypotenuse.
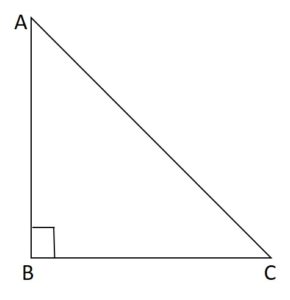
In the above right-angled triangle, AB is the altitude, BC is the base, and the AC is the hypotenuse. AC is the largest side and it is opposite to the right angle within a triangle.
Midpoint of Right Angled Triangle Formula
The formula is represented as the square of the hypotenuse is equal to the sum of the square of the base and the square of the altitude.
Formula: (Hypotenuse)² = (Base)² + (Altitude)².
Midpoint Theorem on Right-Angled Triangle Statement & Proof
Prove that in a right-angled triangle the length of the median drawn to the hypotenuse is half the hypotenuse in length.
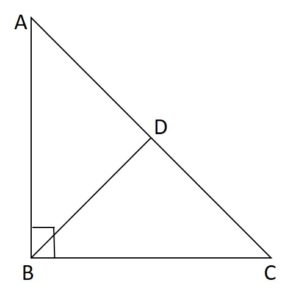
Given:
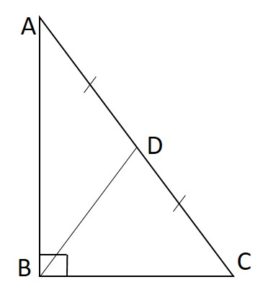
In ∆ABC, ∠B = 90°, and BD is the median drawn to hypotenuse AB.
To Prove:
We have to prove that in a right-angled triangle ABC,
BD = ½ AC.
Construction:
From the above diagram ∆ABC, construct a right-angled triangle to prove the theorem statement.
Draw a line E and DE ∥ BC such that DE cuts AB at E.
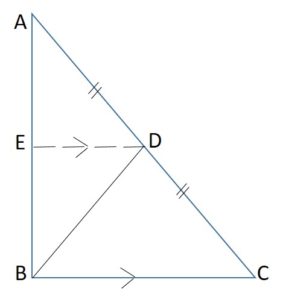
Proof:
In ∆ABC, AD = ½ AC ( D is the midpoint of AC )
In ∆ABC,
(i) D is the midpoint of AC (by given)
(ii) DE ∥ BC (by construction)
Therefore, E is the midpoint of AB (by the converse of the midpoint theorem) —- (1)
ED ⊥ AB (since ED ∥ BC and BC ⊥ AB) —- (2)
In ∆AED and ∆BED,
(i) AE = EB (from (1))
(ii) ED = ED (common side)
(iii) ∠AED = ∠BED = 90° (from (2))
Therefore, ∆AED ≅ ∆BED (by SAS congruency theorem)
AD = BD (by c.p.c.t) —- (3)
Therefore, BD = ½ AC (using (3) in AD = ½ AC).
Hence, the theorem is proved.
Do Check:
- Four Triangles which are Congruent to One Another
- Midpoint Theorem on Trapezium
- Midsegment Theorem on Trapezium
Examples on Midpoint of Hypotenuse of Right-angled Triangles
Example 1:
In ∆PQR, QS is the median of ∆PQR. If the hypotenuse of PR= 12cm. Find the length of the median QS.
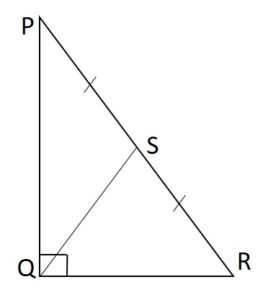
Solution:
We know in a right-angled triangle, the length of the median of the hypotenuse is half the length of the hypotenuse.
Given PR= 12cm
Now, have to find the length of the median QS.
The formula used for a right-angled triangle is QS= ½PR.
QS= ½×12
⇒ QS= 6cm.
Therefore, the length of the median QS of ∆PQR is 6cm.
Example 2:
In ∆ABC, BD is the median of ∆ABC. If the length of the median BD is 14cm. Find the length of the hypotenuse AC.
Solution:
The statement used for a right-angled triangle is the median drawn to the hypotenuse is half the hypotenuse in length.
Given the length of the median BD= 14cm.
Now, find the length of the hypotenuse BD.
The formula we use is BD = ½AC.
14= ½ × AC
⇒ 14 × 2 = AC
⇒ AC= 28cm.
Therefore, the length of the hypotenuse AC of ∆ABC is 28cm.
FAQ’s on Midpoint of Right-Angled Triangle
1. What is the midpoint of the hypotenuse of a right triangle called?
The midpoint of the hypotenuse of a right-angled triangle or a right triangle is known as the circumcenter.
2. Does the median of a triangle form a right angle?
The median of a triangle does not always form a right angle to the side on which it is falling. In case, if we have an equilateral triangle or an isosceles triangle one median falls on the non-equal side of an isosceles triangle.
3. What is the midpoint of a right triangle?
The midpoint of the hypotenuse of a right triangle is the circumcenter of the triangle. Let assume any of the three points on the circle like A(a,0), B(b,0), and C(b,c) thus, the midpoint of the hypotenuse is equal to the center of the circle.
4. Why is the median half the hypotenuse?
Normally, the median of a triangle is drawn from one vertex to the midpoint of the opposite side of the vertex. In a right-angled triangle, the median to the hypotenuse has its property, i.e., the length of the hypotenuse is equal to half the length of the hypotenuse.