A midpoint is known as the average of the coordinate of the section that forms a new coordinate. For example, let us consider a line segment and we want to cut that section into two equal parts, for that we need to know the midpoint of the line segment. So, to find the center we are finding the midpoint. In a simple way, we can say that the midpoint formula is used to know the average of each coordinate in geometry. From this article, the students can find the definition, formula, and examples of the midpoint formula.
Go through:
Midpoint Formula
If you have the ordered pairs or coordinates (x1, y1) and (x2, y2) then the midpoints of these two coordinates can be calculated by using the midpoint formula that forms a new coordinate (x3, y3). It is very simple to calculate the midpoint of the coordinate. The formula to calculate the midpoint is given below.
Mid point = (x₁ + x₂)/2, (y₁ + y₂)/2
How to find Midpoint?
Go through the below steps to know how to find the midpoint using the formula.
1. First identify the coordinates (x₁,y₁) and (x₂,y₂).
2. Substitute the given values in the midpoint formula.
3. Add x-coordinate and y-coordinates and divide the sum of the coordinates by 2.
4. Now you will get the midpoint of the given coordinates.
Do Check: Midpoint Theorem
Midpoint Formula Examples
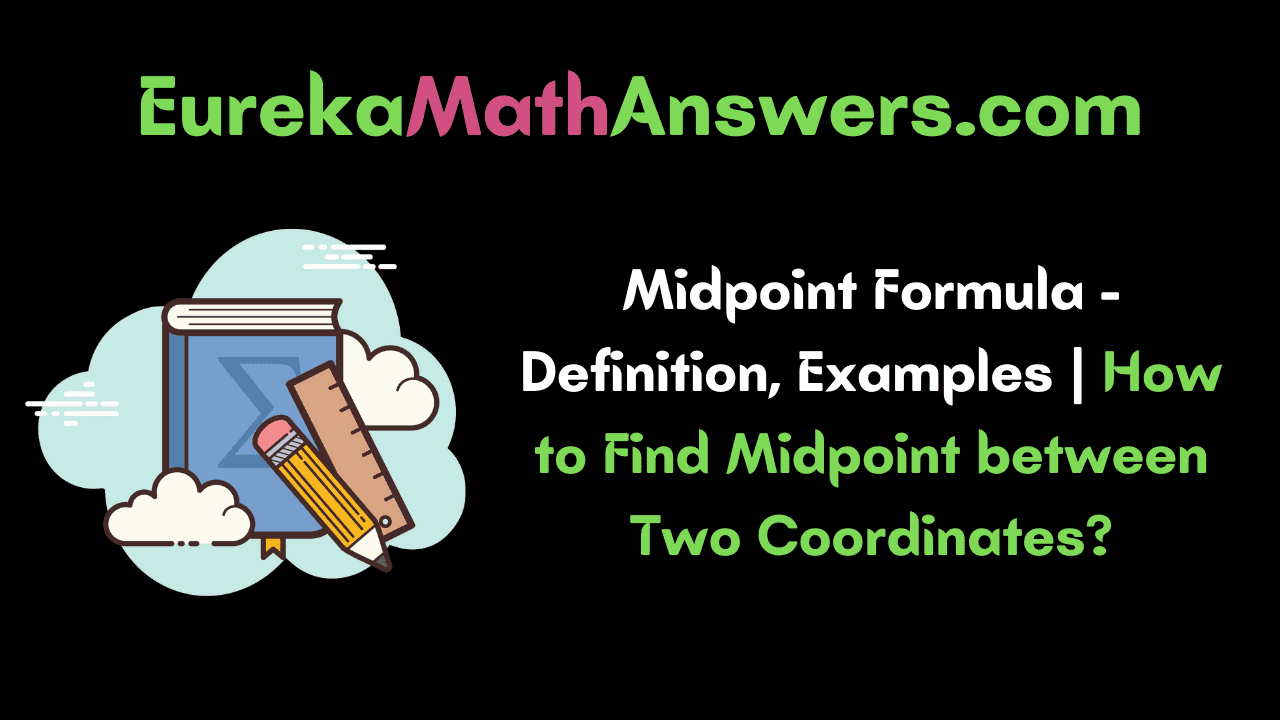
Example 1.
Find the midpoint of a line whose endpoints are (4, 7) and (6, 3).
Solution:
Given that,
(x1, y1) = (4, 7)
(x2, y2) = (6, 3)
According to the formula we can find the midpoint (x, y).
(x, y) = [(x1 + x2)/2, (y1 + y2)/2]
(x, y) = [(4 + 6)/2, (7 + 3)/2]
= (5, 5)
Example 2.
If (1, 0) is the midpoint of the line joining the points A(6, 5) and B, then find the coordinates of B.
Solution:
Given,
(1, 0) is the midpoint of A and B.
A = (6, 5) = (x1, y1)
Let the coordinates of B are (a, b).
(a, b) = (x2, y2)
Using the midpoint formula
(x, y) = [(x1 + x2)/2, (y1 + y2)/2] (1, 0) = [(a + 6)/2, (b + 5)/2]
Now equating the x and y coordinates,
(a + 6)/2 = 1, (b + 5)/2 = 0
a + 6 = 2, b + 5 = 0
a = -4, b = -5
Therefore, the coordinates of B = (-4, -5)
Example 3.
Find the coordinates of the center of the circle whose endpoints of a diameter are (0, 3), (6, 4).
Solution:
Given coordinates of endpoints of a diameter are:
(0, 3) = (x1, y1)
(6, 4) = (x2, y2)
Centre of the circle is the midpoint of diameter.
Coordinates of the centre of a circle = [(x1 + x2)/2, (y1 + y2)/2]
= [(0 + 6)/2, (3 + 4)/2]
= (6/2, 7/2)
= (3, 7/2)
Example 4.
Find the coordinates of the midpoint of the line segment joining the point A (-3, 4) and B (7, -4).
Solution:
Given that
Let M (x, y) be the midpoint of AB.
We know that midpoint formula = (x,y) = (x1 + x2/2 ,y1 + y2/2)
Then, x = (−3)+7/2 = 2 and y = 4+(−4)/2 = 0
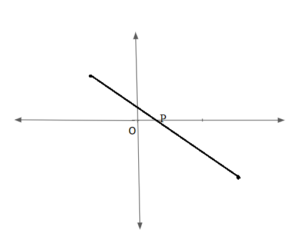
Therefore, the required middle point is M (2, 0).
Example 5.
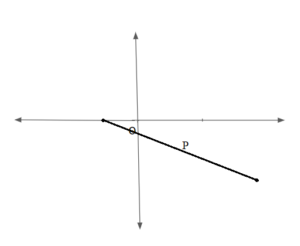
Let P (4, -3) be the middle point of the line segment AB, where A has the coordinates (-2, 0). Find the coordinate of B.
Solution:
Let the coordinates of B be (m, n). The middle point P on AB has the coordinates ((−2)+m/2, 0+n/2).
But P has the coordinates (4, -3).
Therefore, (−2)+m/2 = 4 and 0+n/2 = -3
-2 + m = 8 and n = -6
m = 8 + 2 and n = -6
Therefore, the coordinates of B (10, -6)
FAQs on Midpoint Formula
1. What is the midpoint between two numbers?
The midpoint between two numbers is the number which means in the middle of the two numbers. And it is also called that the midpoint is the same thing as determining the average of two numbers. Therefore, you can estimate the midpoint between any two numbers by adding them and dividing them by two.
2. What is the midpoint in statistics?
The midpoint formula in statistics is the classmark formula, classmark is the midpoint in the class interval. Classmarks can be calculated using the Midpoint = Classmark = (Lower Limit + Upper Limit)/2
3. What is the midpoint formula used for?
The midpoint formula is a mathematical formula used for finding the midpoint of two points. means the point located between two points.