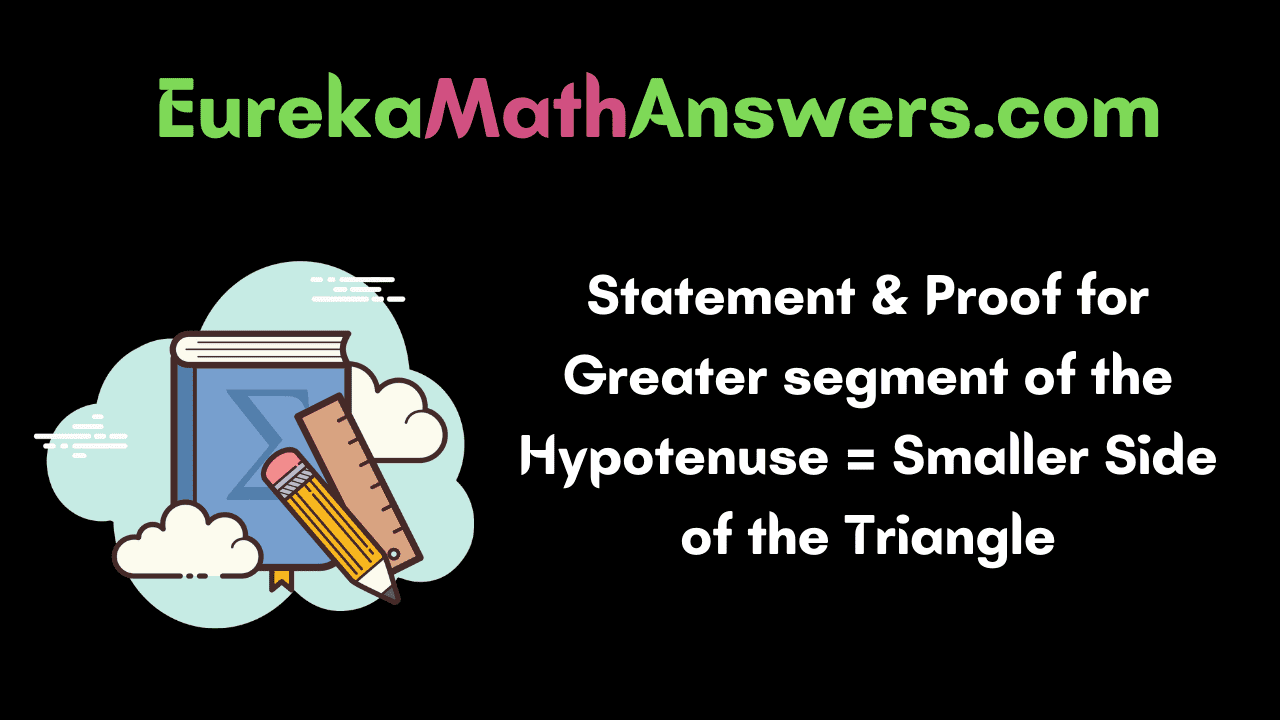A right-angled triangle is a triangle that is having one angle equal to 90 degrees. Here we are giving one statement and proof related to the right-angled triangle statement. Get the example problems with solutions and many more useful details about the greater segment of the hypotenuse is equal to the small side of the right-angled triangle from the following sections.
Greater segment of the Hypotenuse is Equal to the Smaller Side of the Triangle
The statement is if a perpendicular is drawn from the right-angled vertex of a right-angled triangle to the hypotenuse and if the sides of the right-angled triangle are in continued proportion, then the greater segment of the hypotenuse is equal to the smaller side of the triangle.
The proof for the above statement is mentioned here.
Proof:
In ∆ABC, ∠ABC = 90°
BP ⊥ AC
AB < BC and BC < AC
Also, \(\frac { AB }{ BC } \) = \(\frac { BC }{ AC } \)
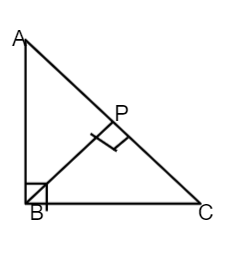
To prove: AB = PC
| Statement | Reason |
|---|---|
| In ∆ABC, ∆BPC ∠ACB = ∠PCB ∠ABC = ∠BPC = 90° |
Common angle Given |
| ∆ABC ∼ ∆BPC | By AA criterion of similarity |
| So, | Corresponding sides of similar triangles are proportional |
| But, | Given |
| Therefore, | From statements 3, 4 |
| Therefore, AB = PC | From statement 5 |
Hence proved.
Do Check:
- Basic Proportionality Theorem
- Converse of Basic Proportionality Theorem
- Application of Basic Proportionality Theorem
Solved Questions
Question 1:
△ABC is a right-angled triangle where B has the right angle. Draw a line from B that is perpendicular to AC. If AB = 3 cm, BC = 6 cm, AC = 12 cm, find the length of the PC.
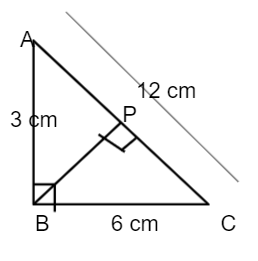
Solution:
Given that,
△ABC is a right-angled triangle with B having a right angle.
AB = 3 cm, BC = 6 cm, AC = 12 cm
BP ⊥ AC, AB < BC and BC < AC
\(\frac { AB }{ BC } \) = \(\frac { BC }{ AC } \)
\(\frac { 3 }{ 6 } \) = \(\frac { 6 }{ 12 } \)
\(\frac { 1 }{ 2 } \) = \(\frac { 1 }{ 2 } \)
The greater segment of the hypotenuse is equal to the smaller side of the triangle
So, AB = PC
Therefore, PC = 3 cm
Question 2:
In ΔABC, D and E are points on the sides AB and AC respectively such that DE ∥ BC. If \(\frac { AD }{ DB } \) = \(\frac { 3 }{ 4 } \) and AC = 15 cm, find AE.
Solution:
According to the basic proportionality theorem,
\(\frac { AD }{ DB } \) = \(\frac { AE }{ EC } \)
AC = 15 cm
Let, AE = x and EC = 15 – x
\(\frac { 3 }{ 4 } \) = \(\frac { x }{ 15 – x } \)
3(15 – x) = 4x
45 – 3x = 4x
4x + 3x = 45
7x = 45
x = 6.43
FAQ’s on Greater segment of the Hypotenuse = Smaller Side of the Triangle
1. What are segments of a hypotenuse?
The hypotenuse of a right triangle is divided into 2 segments by the altitude to the hypotenuse. The sum of the greater segments on the hypotenuse of 2 dissimilar right triangles is equal to the perimeter of the triangle.
2. How are the hypotenuse and legs of the right triangle related?
In a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the remaining legs.
3. What are two segments of the hypotenuse?
In a right angles-triangle, the altitude drawn from the right-angled side to the opposite side divides the hypotenuse into two segments.