Gain some basic knowledge about the Fraction Equivalence and Comparison topics by accessing the free Go Math Grade 4 Answer Key Chapter 6 Fraction Equivalence and Comparison. This resource of Go Math Grade 4 Answer Key aid your preparation for the standard tests. All the lessons covered in chapter 6 Fraction Equivalence and Comparison HMH Go Math Grade 4 Solution Key can be more efficient while your practice sessions. So, get the Homework Help needed by referring to the Go Math Grade 4 Answer Key Chapter 6 Fraction Equivalence and Comparison.
Go Math Grade 4 Chapter 6 Fraction Equivalence and Comparison Answer Key
Download Go Math Grade 4 Solution Key Chapter 6 Fraction Equivalence and Comparison and prepare the concepts whenever you wish. Take the help from the given resource and solve the Grade 4 chapter 6 Fraction Equivalence and Comparison regularly to score high. Refer to the detailed Solutions presented here in Go Math Grade 4 Chapter 6 Fraction Equivalence and Comparison Answer Key and review your answers.
Lesson 1: Investigate • Equivalent Fractions
Lesson 2: Generate Equivalent Fractions
- Generate Equivalent Fractions – Page No. 335
- Generate Equivalent Fractions Lesson Check – Page No. 336
- Generate Equivalent Fractions Lesson Check 1 – Page No. 337
- Generate Equivalent Fractions Lesson Check 2 – Page No. 338
Lesson 3: Simplest Form
- Simplest Form – Page No. 341
- Simplest Form Lesson Check – Page No. 342
- Simplest Form Lesson Check 1 – Page No. 343
- Simplest Form Lesson Check 2 – Page No. 344
Lesson 4: Common Denominators
- Common Denominators – Page No. 347
- Common Denominators Lesson Check – Page No. 348
- Common Denominators Lesson Check 1 – Page No. 349
- Common Denominators Lesson Check 2 – Page No. 350
- Common Denominators Lesson Check 3 – Page No. 353
- Common Denominators Lesson Check 4 – Page No. 354
Lesson 5: Problem Solving • Find Equivalent Fractions
Mid-Chapter Checkpoint
Lesson 6: Compare Fractions Using Benchmarks
- Compare Fractions Using Benchmarks – Page No. 361
- Compare Fractions Using Benchmarks Lesson Check – Page No. 362
- Compare Fractions Using Benchmarks Lesson Check 1 – Page No. 363
- Compare Fractions Using Benchmarks Lesson Check 2 – Page No. 364
Lesson 7: Compare Fractions
- Compare Fractions – Page No. 367
- Compare Fractions Lesson Check – Page No. 368
- Compare Fractions Lesson Check 1 – Page No. 369
- Compare Fractions Lesson Check 2 – Page No. 370
Lesson 8: Compare and Order Fractions
- Compare and Order Fractions – Page No. 373
- Compare and Order Fractions Lesson Check – Page No. 374
- Compare and Order Fractions Lesson Check 1 – Page No. 375
- Compare and Order Fractions Lesson Check 2- Page No. 376
Review/Test
- Review/Test – Page No. 377
- Review/Test – Page No. 378
- Review/Test – Page No. 379
- Review/Test – Page No. 380
- Review/Test – Page No. 381
- Review/Test – Page No. 382
- Review/Test – Page No. 387
- Review/Test – Page No. 388
Common Core – Equivalent Fractions – Page No. 331
Equivalent Fractions
Use the model to write an equivalent fraction.
Question 1.

\(\frac{4}{6}=\frac{2}{3}\)
Answer:
\(\frac{4}{6}=\frac{2}{3}\)
Explanation:
The first image has 4 parts shaded our of 6 parts. Divide \(\frac{8}{10}\) with 2. You will get \(\frac{2}{3}\). That means 2 parts are shaded out of 3 parts.
Question 2.
![]()
\(\frac{3}{4}\) = \(\frac{□}{□}\)
Answer:
\(\frac{3}{4}\) = \(\frac{6}{8}\)
Explanation:
The first image has 3 parts shaded our of 4 parts. Multiply \(\frac{8}{10}\) with 2. You will get \(\frac{6}{8}\). That means 6 parts are shaded out of 8 parts.
Tell whether the fractions are equivalent. Write = or ≠.
Question 3.
\(\frac{8}{10}\) _______ \(\frac{4}{5}\)
Answer:
\(\frac{8}{10}\) = \(\frac{4}{5}\)
Explanation:
Multiply the numerator and denominator of 4 / 5 with 2
8 / 10 = (2 / 2 ) x (4 / 5 )
= 8 / 10
So, 8 / 10 = 4 / 5.
Question 4.
\(\frac{1}{2}\) _______ \(\frac{7}{12}\)
Answer:
\(\frac{1}{2}\) ≠ \(\frac{7}{12}\)
Explanation:
Multiply the numerator and denominator of 1 / 2 with 6
1 / 2 = (6 / 6) x (1 / 2)
= (6 / 12)
So, 1/2 ≠ 7 / 12
Question 5.
\(\frac{3}{4}\) _______ \(\frac{8}{12}\)
Answer:
\(\frac{3}{4}\) ≠ \(\frac{8}{12}\)
Explanation:
Multiply the numerator and denominator of 3 / 4 with 3
3 / 4 = (3 / 3) x (3 / 4)
= (9 / 12)
So, 3 / 4 ≠ 8 / 12
Question 6.
\(\frac{2}{3}\) _______ \(\frac{4}{6}\)
Answer:
\(\frac{2}{3}\) = \(\frac{4}{6}\)
Explanation:
Multiply the numerator and denominator of 2 / 3 with 2
2 / 3 = (2 / 2) x ( 2 / 3 )
= 4 / 6
So, 2 / 3 = 4 / 6.
Question 7.
\(\frac{5}{8}\) _______ \(\frac{4}{10}\)
Answer:
\(\frac{5}{8}\) ≠ \(\frac{4}{10}\)
Explanation:
Multiply the numerator and denominator of 5 / 8 with 2
5 / 8 =(2 / 2) x (5 / 8)
= (10 / 16)
So, 5 / 8 ≠ 4 / 10
Question 8.
\(\frac{2}{6}\) _______ \(\frac{4}{12}\)
Answer:
\(\frac{2}{6}\) = \(\frac{4}{12}\)
Explanation:
Multiply the numerator and denominator of 2 / 6 with 2
2 / 6 = (2 / 2) x (2 / 6)
= (4 / 12)
So, 2 / 6 = 4 / 12.
Question 9.
\(\frac{20}{100}\) _______ \(\frac{1}{5}\)
Answer:
\(\frac{20}{100}\) = \(\frac{1}{5}\)
Explanation:
Cross Multiply the 20 / 100 with 20 / 20
20 / 100 = (20 / 20) x (20 / 100)
= (1 / 5)
So, 20 / 100 = 1 / 5.
Question 10.
\(\frac{5}{8}\) _______ \(\frac{9}{10}\)
Answer:
\(\frac{5}{8}\) ≠ \(\frac{9}{10}\)
Explanation:
Multiply the numerator and denominator of 5 / 8 with 2
5 / 8 = (2 / 2) x (5 / 8)
= 10 / 16
So, 5 / 8 ≠ 9 / 10
Question 11.
Jamal finished \(\frac{5}{6}\) of his homework. Margaret finished \(\frac{3}{4}\) of her homework, and Steve finished \(\frac{10}{12}\) of his homework. Which two students finished the same amount of homework?
_______
Answer:
Jamal and Steve
Explanation:
As per the given data,
Jamal finished work = 5 /6 of his homework
Margaret finished work = 3 / 4th of her homework
Steve finished work = 10 / 12 of his homework
Multiply the numerator and denominator of 5/ 6 with 2
Then, (2 / 2) x (5 / 6) = 10 / 12
Then, Jamal and Steve finished the same amount of homework.
Question 12.
Sophia’s vegetable garden is divided into 12 equal sections. She plants carrots in 8 of the sections. Write two fractions that are equivalent to the part of Sophia’s garden that is planted with carrots.
Type below:
___________
Answer:
\(\frac{2}{3}\) and \(\frac{4}{6}\)
Explanation:
As per the given data,
Sophia’s vegetable garden is divided into 12 equal sections
She plants carrots in 8 of the sections out of 12 sections = 8 / 12
By simplifying the 8 / 12, we will get 4 / 6
Again simplify the 4 /6 by dividing method, you will get 2 /3
2 / 3 = (2 / 2) x (2 / 3)
= 4 / 6
Then, the equivalent fractions are 2 / 3, 4 /6
Common Core – Equivalent Fractions – Page No. 332
Question 1.
A rectangle is divided into 8 equal parts. Two parts are shaded. Which fraction is equivalent to the shaded area of the rectangle?
Options:
a. \(\frac{1}{4}\)
b. \(\frac{1}{3}\)
c. \(\frac{2}{6}\)
d. \(\frac{3}{4}\)
Answer:
a. \(\frac{1}{4}\)
Explanation:
As per the given data,
A rectangle is divided into 8 equal parts
Two parts are shaded
Then, the shaded area of rectangle = 2 / 8
By simplifying the 2/ 8, you will get 1/ 4
So, the shaded area of rectangle = 1 / 4
Question 2.
Jeff uses 3 fifth-size strips to model \(\frac{3}{5}\). He wants to use tenth-size strips to model an equivalent fraction. How many tenth-size strips will he need?
Options:
a. 10
b. 6
c. 5
d. 3
Answer:
b. 6
Explanation:
From the given data,
Jeff uses 3 fifth –size strips to model = 3 / 5 size strips
If he want to use tenth – size strips to an equivalent fraction = 1 / 10 size strips
The number of strips = x
(1 / 10) x = 3 / 5
x = 30/5
then, required number of tenth size trips = 6
Question 3.
Cassidy places 40 stamps on each of 8 album pages. How many stamps does she place in all?
Options:
a. 300
b. 320
c. 360
d. 380
Answer:
b. 320
Explanation:
As per the given data,
Cassidy places 40 stamps on each of 8 album pages = 8 x 40
= 320
So, total placed stamps on album pages by Cassidy = 320 stamps
Question 4.
Maria and 3 friends have 1,200 soccer cards. If they share the soccer cards equally, how many will each person receive?
Options:
a. 30
b. 40
c. 300
d. 400
Answer:
c. 300
Explanation:
As per the given data,
Maria and 3 friends have 1200 soccer cards
If soccer cards shared equally by four members = 1200/4
= 300
Then, each person received soccer cards = 300
Question 5.
Six groups of students sell 162 balloons at the school carnival. There are 3 students in each group. If each student sells the same number of balloons, how many balloons does each student sell?
Options:
a. 9
b. 18
c. 27
d. 54
Answer:
a. 9
Explanation:
As per the given, data,
Six groups of students sell 162 balloons at the school carnival
There are 3 students in each group
Then, total number of students in 6 groups = 6 x 3 = 18
If each student sells the same number of balloons = 162 / 18
= 9
Number of balloons sells by each student = 9
Question 6.
Four students each made a list of prime numbers.
Eric: 5, 7, 17, 23
Maya: 3, 5, 13, 17
Bella: 2, 3, 17, 19
Jordan: 7, 11, 13, 21
Who made an error and included a composite number?
Options:
a. Eric
b. Maya
c. Bella
d. Jordan
Answer:
d. Jordan
Explanation:
As per the given data,
Four students each made a list of prime numbers.
Eric: 5, 7, 17, 23
Maya: 3, 5, 13, 17
Bella: 2, 3, 17, 19
Jordan: 7, 11, 13, 21
21 is not a prime number
So, An error made by Jordan
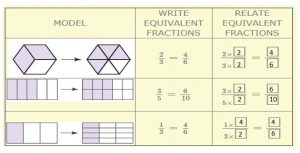
Page No. 335
Question 1.
Complete the table below.
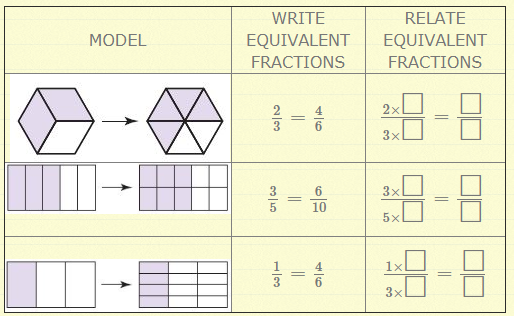
Type below:
___________
Answer:
Write two equivalent fractions.
Question 2.
\(\frac{4}{5}\)
\(\frac{4}{5}\) = \(\frac { 4×□ }{ 5×□ } \) = \(\frac{□}{□}\)
\(\frac{4}{5}\) = \(\frac { 4×□ }{ 5×□ } \) = \(\frac{□}{□}\)
\(\frac{4}{5}\) = \(\frac{□}{□}\) = \(\frac{□}{□}\)
Type below:
___________
Answer:
\(\frac{4}{5}\) = \(\frac{8}{10}\) = \(\frac{80}{100}\)
Explanation:
Two equivalent fractions of 4/5,
(4/5) x (2/2) = 8/10
And
(4/5) x (20/20) = 80/100
8/10 = (8/10) (10/10)
= (80/100)
So, the equivalent fractions of 4/5 = 8/10, 80/100
Question 3.
\(\frac{2}{4}\)
\(\frac{2}{4}\) = \(\frac { 2×□ }{ 4×□ } \) = \(\frac{□}{□}\)
\(\frac{2}{4}\) = \(\frac { 2×□ }{ 4×□ } \) = \(\frac{□}{□}\)
\(\frac{2}{4}\) = \(\frac{□}{□}\) = \(\frac{□}{□}\)
Type below:
___________
Answer:
\(\frac{2}{4}\) = \(\frac{4}{8}\) = \(\frac{8}{16}\)
Explanation:
Two equivalent fractions of 2/4,
(2/4) x (2/2) = 4/8
And
(2/4) x (4/4) = 8/16
4/8 = (4/8) (2/2)
= (8/16)
So, the equivalent fractions of 2/4 = 4/8, 8/16
Write two equivalent fractions.
Question 4.
\(\frac{3}{6}\)
\(\frac{3}{6}\) = \(\frac{□}{□}\) = \(\frac{□}{□}\)
Type below:
___________
Answer:
\(\frac{3}{6}\) = \(\frac{6}{12}\) = \(\frac{12}{24}\)
Explanation:
Two equivalent fractions of 3/6,
(3/ 6) x (2/2) = 6/12
And
(3/6) x (4/ 4) = 12/24
6/12 = (6/12) (2/2)
= (12/24)
So, the equivalent fractions of 3/6 = 6/12, 12/24
Question 5.
\(\frac{3}{10}\)
\(\frac{3}{10}\) = \(\frac{□}{□}\) = \(\frac{□}{□}\)
Type below:
___________
Answer:
\(\frac{3}{10}\) = \(\frac{6}{20}\) = \(\frac{12}{40}\)
Explanation:
Two equivalent fractions of 3/10,
(3/ 10) x (2/2) = 6/20
And
(3/10) x (4/ 4) = 12/40
6/20 = (6/20) (2/2)
= (12/40)
So, the equivalent fractions of 3/10 = 6/20, 12/40
Question 6.
\(\frac{2}{5}\)
\(\frac{2}{5}\) = \(\frac{□}{□}\) = \(\frac{□}{□}\)
Type below:
___________
Answer:
\(\frac{2}{5}\) = \(\frac{4}{10}\) = \(\frac{8}{20}\)
Explanation:
Two equivalent fractions of 2/5,
(2/ 5) x (2/2) = 4/10
And
(2/5) x (4/ 4) = 8/20
4/10 = (4/10) (2/2)
= (8/20)
So, the equivalent fractions of 2/5 = 4/10, 8/20
Tell whether the fractions are equivalent. Write = or ≠.
Question 7.
\(\frac{5}{6}\) ______ \(\frac{10}{18}\)
Answer:
\(\frac{5}{6}\) ≠ \(\frac{10}{18}\)
Explanation:
Multiply the numerator and denominator of 5/6 with 2
5/6 =(2/2) x (5/6)
= (10/12)
So, 5/6 ≠ 10/ 18
Question 8.
\(\frac{4}{5}\) ______ \(\frac{8}{10}\)
Answer:
\(\frac{4}{5}\) = \(\frac{8}{10}\)
Explanation:
Multiply the numerator and denominator of 4/5 with 2
4/5 =(2/2) x (4/5)
= (8/10)
So, 4/5 = 8/10
Question 9.
\(\frac{1}{5}\) ______ \(\frac{4}{10}\)
Answer:
\(\frac{1}{5}\) ≠ \(\frac{4}{10}\)
Explanation:
Multiply the numerator and denominator of 1/5 with 4
1/5 =(4/4) x (1/5)
= (4/20)
So, 1/5 ≠ 4/10
Question 10.
\(\frac{1}{4}\) ______ \(\frac{2}{8}\)
Answer:
\(\frac{1}{4}\) = \(\frac{2}{8}\)
Explanation:
Multiply the numerator and denominator of 1/4 with 2
1/4 =(2/2) x (1/4)
= (2/8)
So, 1/4 = 2/8
Page No. 336
Use the recipe for 11–12.
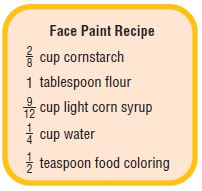
Question 11.
Kim says the amount of flour in the recipe can be expressed as a fraction. Is she correct? Explain.
______
Answer:
As per the given data, Kim says the amount of flour in the recipe can be expressed as a fraction. But in the recipe, 1 tablespoon flour is added. So, Kim says wrong.
Question 12.
How could you use a \(\frac{1}{8}\) – cup measuring cup to measure the light corn syrup?
Type below:
_________
Answer:
As per the given data,
By using the 1/8 cup measure the 9/12 cup light corn syrup
(9/12)/(1/8) = (9 x 8)/12
= (3 x 8)/4
= (3 x 2)
= 6
So, required 6 cups of 1/8 to measure the light corn syrup of 9/12.
Question 13.
Communicate Explain using words how you know a fraction is equivalent to another fraction.
Type below:
_________
Answer:
If you multiply the numerator and denominator of the first fraction by the same number and the products are the numerator and denominator of the second fraction, then the fractions are equivalent
Question 14.
Kyle drank \(\frac{2}{3}\) cup of apple juice. Fill in each box with a number from the list to generate equivalent fractions for \(\frac{2}{3}\). Not all numbers will be used.
Type below:
_________
Answer:
\(\frac{4}{6}\) and \(\frac{12}{18}\)
Explanation:
As per the given data,
Kyle drank 2/3 cup of apple juice
(2/3) x (2/2) = 4/6
(4/6) x (3/3) = 12/18
Equivalent fractions of 2/3 are 4/6 and 12/18
Common Core – Equivalent Fractions – Page No. 337
Write two equivalent fractions for each.
Question 1.
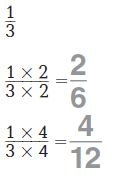
Answer:
\(\frac{2}{6}\) and \(\frac{4}{12}\)
Explanation:
1/3
(1/3) x (2/2) = 2/6
(1/3) x (4/4) = 4/12
So, the equivalent fractions of 1/3 are 2/6 and 4/12
Question 2.
\(\frac{2}{3}\)
Type below:
_________
Answer:
\(\frac{4}{6}\) and \(\frac{8}{12}\)
Explanation:
2/3
(2/3) x (2/2) = 4/6
(2/3) x (4/4) = 8/12
Then, the equivalent fractions of 2/3 = 4/6 and 8/12
Question 3.
\(\frac{1}{2}\)
Type below:
_________
Answer:
\(\frac{2}{4}\) and \(\frac{4}{8}\)
Explanation:
1/2
(1/2) x (2/2) = 2/4
(1/2) x (4/4) = 4/8
Then, the equivalent fractions of 1/2 = 2/4, 4/8
Question 4.
\(\frac{4}{5}\)
Type below:
_________
Answer:
\(\frac{8}{10}\) and \(\frac{80}{100}\)
Explanation:
4/5
(4/5) x (2/2) = 8/10
(4/5) x (20/20) = 80/100
Then, the equivalent fractions of 4/5 = 8/10 and 80/100
Tell whether the fractions are equivalent. Write # or ≠.
Question 5.
\(\frac{1}{4}\) ______ \(\frac{3}{12}\)
Answer:
\(\frac{1}{4}\) = \(\frac{3}{12}\)
Explanation:
1/4
Multiply the numerator and denominator of 1/4 with 3
Then, (1/4) x (3/3) = 3/12
So, 1/4 = 3/12
Question 6.
\(\frac{4}{5}\) ______ \(\frac{5}{10}\)
Answer:
\(\frac{4}{5}\) ≠ \(\frac{5}{10}\)
Explanation:
4/5
Multiply numerator and denominator of 4/5 with 2
(4/5) x (2/2) = 8/10
Then 4/5 ≠ 5/10
Question 7.
\(\frac{3}{8}\) ______ \(\frac{2}{6}\)
Answer:
\(\frac{3}{8}\) ≠ \(\frac{2}{6}\)
Explanation:
3/8 ≠ 2/6
Question 8.
\(\frac{3}{4}\) ______ \(\frac{6}{8}\)
Answer:
\(\frac{3}{4}\) = \(\frac{6}{8}\)
Explanation:
3/4
Multiply the numerator and denominator of 3/4 with 2
Then, (3/4) x (2/2) = 6/8
So, 3/4 = 6/8
Question 9.
\(\frac{5}{6}\) ______ \(\frac{10}{12}\)
Answer:
\(\frac{5}{6}\) = \(\frac{10}{12}\)
Explanation:
5/6
Multiply the numerator and denominator with 2
(5/6) x (2/2) = 10/12
So, 5/6 = 10/12
Question 10.
\(\frac{6}{12}\) ______ \(\frac{5}{8}\)
Answer:
\(\frac{6}{12}\) ≠ \(\frac{5}{8}\)
Explanation:
6/12 ≠ 5/8
Question 11.
\(\frac{2}{5}\) ______ \(\frac{4}{10}\)
Answer:
\(\frac{2}{5}\) = \(\frac{4}{10}\)
Explanation:
2/5
Multiply the numerator and denominator of 2/5 with 2
(2/5) x (2/2) = 4/10
So, 2/5 = 4/10
Question 12.
\(\frac{2}{4}\) ______ \(\frac{3}{12}\)
Answer:
\(\frac{2}{4}\) ≠ \(\frac{3}{12}\)
Explanation:
2/4
Multiply the numerator and denominator of 2/4 with 3
(2/4) x (3/3) = 6/12
So, 2/4 ≠ 3/ 12
Question 13.
Jan has a 12-ounce milkshake. Four ounces in the milkshake are vanilla, and the rest is chocolate. What are two equivalent fractions that represent the fraction of the milkshake that is vanilla?
Type below:
_________
Answer:
\(\frac{1}{3}\) and \(\frac{2}{6}\)
Explanation:
As per the given data,
Jan has a 12-ounce milkshake
Four ounces in the milkshake are vanilla = 4/12 = 1/3
Then, 8-ounces in milkshake are chocolate = 8/12 = 2/3
4/12 = 1/3
By multiplying 1/3 with 2
(1/3) x (2/2) = 2/6
So, the equivalent fractions of vanilla milkshake are 1/3 and 2/6
Question 14.
Kareem lives \(\frac{4}{10}\) of a mile from the mall. Write two equivalent fractions that show what fraction of a mile Kareem lives from the mall.
Type below:
_________
Answer:
\(\frac{2}{5}\) and \(\frac{8}{20}\)
Explanation:
As per the given data,
Kareem lives 4/10 of a mile from the mall
To find the equivalent fractions of 4/10
Simplify the 4/10 = 2/5
Multiply the numerator and denominator of 2/5 with 4
(2/5) x (4/4) = 8/20
Then, the equivalent fraction of a mile Kareem lives from the mall = 2/5 and 8/20
Common Core – Equivalent Fractions – Page No. 338
Question 1.
Jessie colored a poster. She colored \(\frac{2}{5}\) of the poster red. Which fraction is equivalent to \(\frac{2}{5}\)?
Options:
a. \(\frac{4}{10}\)
b. \(\frac{7}{10}\)
c. \(\frac{4}{5}\)
d. \(\frac{2}{2}\)
Answer:
a. \(\frac{4}{10}\)
Explanation:
As per the given data,
Jessie colored a poster
She colored 2/5th of the poster red
Multiply the numerator and denominator of 2/5 with 2
Then, (2/5) x (2/2) = 4 /10
So, the equivalent fraction of 2/5 is 4/10
Question 2.
Marcus makes a punch that is \(\frac{1}{4}\) cranberry juice. Which two fractions are equivalent to \(\frac{1}{4}\)?
Options:
a. \(\frac{2}{5}, \frac{3}{12}\)
b. \(\frac{2}{8}, \frac{4}{12}\)
c. \(\frac{3}{4}, \frac{6}{8}\)
d. \(\frac{2}{8}, \frac{3}{12}\)
Answer:
d. \(\frac{2}{8}, \frac{3}{12}\)
Explanation:
As per the given data,
Marcus makes a punch that is 1/4th of cranberry juice
Multiply the numerator and denominator of 1/4 with 2
Then, (1/4) x (2/2) = 2/8
Multiply the numerator and denominator of 1/4 with 3
Then, (1/4) x (3/3) = 3/12
Equivalent fractions of 1/4 are 2/8 and 3/12
Question 3.
An electronics store sells a large flat screen television for $1,699. Last month, the store sold 8 of these television sets. About how much money did the store make on the television sets?
Options:
a. $160,000
b. $16,000
c. $8,000
d. $1,600
Answer:
b. $16,000
Explanation:
As per the given data,
An electronics store sells a large flat-screen television for $1,699
Last month, the store sold 8 of these television sets = 8 x $1,699 = $13,952. The money is about to $16,000.
Question 4.
Matthew has 18 sets of baseball cards. Each set has 12 cards. About how many baseball cards does Matthew have in all?
Options:
a. 300
b. 200
c. 150
d. 100
Answer:
b. 200
Explanation:
From the given data,
Matthew has 18 sets of basketball cards
Each set has 12 cards = 12 x 18
= 216
Total number of basketball cards with Matthew = 216. So, it is near to 200.
Question 5.
Diana had 41 stickers. She put them in 7 equal groups. She put as many as possible in each group. She gave the leftover stickers to her sister. How many stickers did Diana give to her sister?
Options:
a. 3
b. 4
c. 5
d. 6
Answer:
d. 6
Explanation:
As per the given data,
Diana has 41 stickers
She put them in 7 equal groups = 41/7
= 5 (remaining 6)
She gave the leftover stickers to her sister
The number of stickers Diana give to her sister = 6
Question 6.
Christopher wrote the number pattern below. The first term is 8.
8, 6, 9, 7, 10, …
Which is a rule for the pattern?
Options:
a. Add 2, add 3.
b. Add 6, subtract 3.
c. Subtract 6, add 3.
d. Subtract 2, add 3
Answer:
d. Subtract 2, add 3
Explanation:
From the given data,
Christopher wrote the number pattern = 8, 6, 9, 7, 10, …..
The first number in the pattern = 8
8 – 2 = 6 + 3 = 9 – 2 = 7 +3 = 10 ….
So, the rule for the above pattern is to subtract 2, add 3
Page No. 341
Question 1.
Write \(\frac{8}{10}\) in simplest form.
\(\frac{8}{10}\) = \(\frac { 8÷□ }{ 10÷□ } \) = \(\frac{□}{□}\)
\(\frac{□}{□}\)
Answer:
\(\frac{4}{5}\)
Explanation:
8/10 in simplest form
Divide the 8/10 with 2
(8/2)/(10/2) = 4/5
So, the simplest form of 8/10 is 4/5
Write the fraction in simplest form.
Question 2.
\(\frac{6}{12}\)
\(\frac{□}{□}\)
Answer:
\(\frac{1}{2}\)
Explanation:
6/12 in simplest form
Divide the 6/12 with 6
(6/6)/(12/6) = 1/2
So, the simplest form of 6/12 is 1/2
Question 3.
\(\frac{2}{10}\)
\(\frac{□}{□}\)
Answer:
\(\frac{1}{5}\)
Explanation:
2/10 in simplest form
Divide the 2/10 with 2
(2/2)/(10/2) = 1/5
So, the simplest form of 2/10 is 1/5
Question 4.
\(\frac{6}{8}\)
\(\frac{□}{□}\)
Answer:
\(\frac{3}{4}\)
Explanation:
6/8 in simplest form
Divide the 6/8 with 2
(6/2)/(8/2) = 3/4
So, the simplest form of 6/8 is 3/4
Question 5.
\(\frac{4}{6}\)
\(\frac{□}{□}\)
Answer:
\(\frac{2}{3}\)
Explanation:
4/6 in simplest form
Divide the 4/6 with 2
(4/2)/(6/2) = 2/3
So, the simplest form of 4/6 is 2/3
Write the fraction in simplest form.
Question 6.
\(\frac{9}{12}\)
\(\frac{□}{□}\)
Answer:
\(\frac{3}{4}\)
Explanation:
9/12in simplest form
Divide the 9/12 with 3
(9/3)/(12/3) = 3/4
So, the simplest form of 9/12 is 3/4
Question 7.
\(\frac{4}{8}\)
\(\frac{□}{□}\)
Answer:
\(\frac{1}{2}\)
Explanation:
4/8in simplest form
Divide the 4/8 with 4
(4/4)/(8/4) = 1/2
So, the simplest form of 4/8 is 1/2
Question 8.
\(\frac{10}{12}\)
\(\frac{□}{□}\)
Answer:
\(\frac{5}{6}\)
Explanation:
10/12 in simplest form
Divide the 10/12 with 2
(10/2)/(12/2) = 5/6
So, the simplest form of 10/12 is 5/6
Question 9.
\(\frac{20}{100}\)
\(\frac{□}{□}\)
Answer:
\(\frac{1}{5}\)
Explanation:
20 /100 in simplest form
Divide the 20/100 with 20
(20/20)/(100/20) = 1/5
So, the simplest form of 20/100 is 1/5
Tell whether the fraction is in simplest form. Write yes or no.
Question 10.
\(\frac{2}{8}\)
______
Answer:
No
Explanation:
2/8 in simplest form
Divide the 2/8 with 2
(2/2)/(8/2) = 1/4
The simplest form of 2/8 is 1/4
So, 2/8 is not the simplest form
Question 11.
\(\frac{9}{12}\)
______
Answer:
No
Explanation:
9/12 in simplest form
Divide the 9/12 with 3
(9/3)/(12/3) = 3/4
The simplest form of 9/12 is 3/4
So, 9/12 is not the simplest form
Question 12.
\(\frac{5}{6}\)
______
Answer:
Yes
Explanation:
5/6 is not divided by any number
Yes, 5/6 is the simplest form
Question 13.
\(\frac{4}{10}\)
______
Answer:
No
Explanation:
4/10 in simplest form
Divide the 4/10 with 2
(4/2)/(10/2) = 2/5
So, 4/10 is not the simplest form
Question 14.
There are 18 students in Jacob’s homeroom. Six students bring their lunch to school. The rest eat lunch in the cafeteria. In simplest form, what fraction of students eat lunch in the cafeteria?
\(\frac{□}{□}\) of students
Answer:
\(\frac{2}{3}\) of students
Explanation:
As per the given data,
There are 18 students in Jacob’s homeroom
6 students bring their lunch to school = 6/18 = 1/3
The rest eat lunch in the cafeteria = 18 – 6 = 12/18
Divide the numerator and denominator of 12/18 with 6
(12/6) x (18/6) = 2/3
So, 2/3 of students eat lunch in the cafeteria
Page No. 342
Use the map for 15−16.
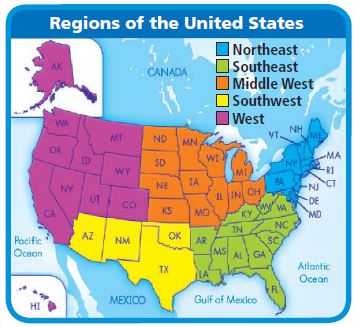
Question 15.
Identify Relationships What fraction of the states in the southwest region share a border with Mexico? Is this fraction in simplest form?
\(\frac{□}{□}\)
Answer:
Yes, \(\frac{3}{4}\)
Explanation:
As per the given data,
Southwest region states = 4
Number of states in the southwest region shares a border with Mexico out of total southwest region states = 3/4
Yes, 3/4 is the simplest form
Question 16.
What’s the Question? \(\frac{1}{3}\) of the states in this region are on the Gulf of Mexico.
Type below:
_________
Answer:
In the simplest form, what fraction of the states in the southeast area on the Gulf of Mexico.
Question 17.
Pete says that to write \(\frac{4}{6}\) as \(\frac{2}{3}\), you combine pieces, but to write \(\frac{4}{6}\) as \(\frac{8}{12}\), you break apart pieces. Does this make sense? Explain.
______
Answer:
As per the given data,
Yes, it makes sense,
To write 4/6 as 2/3 combine sixth size pieces into equal groups of 2
Then (4/2)/(6/2) = 2/3
To write 4/6 as 8/12, break each sixth piece into 2 pieces
Then, 4/6 = (4 x 2)/(6 x 2) = 8/12
Question 18.
In Michelle’s homeroom, \(\frac{9}{15}\) of the students ride the bus to school, \(\frac{4}{12}\) get a car ride, and \(\frac{2}{30}\) walk to school.
For numbers 18a–18c, select True or False for each statement.
a. In simplest form, \(\frac{3}{5}\) of the students ride the bus to school.
i. True
ii. False
Answer:
i. True
Explanation:
9/15 of the students ride the bus to school
By dividing the numerator and denominator of 9/15 with 3
(9/3)/(15/3) =3/5
So, 3/5 of the students ride the bus to school
True
Question 18.
b. In simplest form, \(\frac{1}{4}\) of the students get a car ride to school.
i. True
ii. False
Answer:
ii. False
Explanation:
a. 4/12 of the students get a car ride
The simplest form of 4/12 = 1/3
So, 1/4 of the students get a car ride to school is a False statement
Question 18.
c. In simplest form, \(\frac{1}{15}\) of the students walk to school.
i. True
ii. False
Answer:
i. True
Explanation:
a. 2/30 of the students walk to school
By dividing the 2/30 with 2
(2/2)/(30/2) = 1/15
So, 1/15 of the students walk to school is a true statement
Common Core – Simplest Form – Page No. 343
Write the fraction in simplest form.
Question 1.
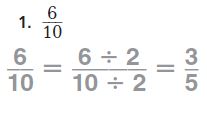
Answer:
\(\frac{3}{5}\)
Explanation:
To write the 6/10 in a simplest form
Divide the numerator and denominator of 6/10 with 2
(6 ÷2)/(10 ÷2) = 3/5
So, the simplest form of 6/10 = 3/5
Question 2.
\(\frac{6}{8}\) = \(\frac{□}{□}\)
Answer:
\(\frac{3}{4}\)
Explanation:
To write the 6/8in a simplest form
Divide the numerator and denominator of 6/8 with 2
(6 ÷2)/(8 ÷2) = 3/4
So, the simplest form of 6/8 = 3/4
Question 3.
\(\frac{5}{5}\) = \(\frac{□}{□}\)
Answer:
\(\frac{1}{1}\) = 1
Explanation:
To write the 5/5in a simplest form
Divide the numerator and denominator of 5/5 with 5
(5 ÷5)/(5 ÷5) = 1/1
So, the simplest form of 5/5 = 1
Question 4.
\(\frac{8}{12}\) = \(\frac{□}{□}\)
Answer:
\(\frac{2}{3}\)
Explanation:
To write the 8/12in a simplest form
Divide the numerator and denominator of 8/12 with 4
(8 ÷4)/(12 ÷4) = 2/3
So, the simplest form of 8/12 = 2/3
Question 5.
\(\frac{100}{100}\) = \(\frac{□}{□}\)
Answer:
\(\frac{1}{1}\) = 1
Explanation:
The simplest form of 100/100 = 1
Question 6.
\(\frac{2}{6}\) = \(\frac{□}{□}\)
Answer:
\(\frac{1}{3}\)
Explanation:
To write the 2/6in a simplest form
Divide the numerator and denominator of 2/6 with 2
(2 ÷2)/(6 ÷2) = 1/3
So, the simplest form of 2/6 = 1/3
Question 7.
\(\frac{2}{8}\) = \(\frac{□}{□}\)
Answer:
\(\frac{1}{4}\)
Explanation:
To write the 2/8in a simplest form
Divide the numerator and denominator of 2/8 with 2
(2 ÷2)/(8 ÷2) = 1/4
So, the simplest form of 2/8 = 1/4
Question 8.
\(\frac{4}{10}\) = \(\frac{□}{□}\)
Answer:
\(\frac{2}{5}\)
Explanation:
To write the 4/10 in a simplest form
Divide the numerator and denominator of 4 /10 with 2
(4 ÷2)/(10 ÷2) = 2/5
So, the simplest form of 4/10 = 2/5
Tell whether the fractions are equivalent. Write = or ≠. (if you dont have ≠on your keybord, copy and paste this one: ≠ )
Question 9.
\(\frac{6}{12}\) _______ \(\frac{1}{12}\)
Answer:
\(\frac{6}{12}\) ≠ \(\frac{1}{12}\)
Explanation:
6/12 ≠ 1/12
Question 10.
\(\frac{3}{4}\) _______ \(\frac{5}{6}\)
Answer:
\(\frac{3}{4}\) ≠ \(\frac{5}{6}\)
Explanation:
3/4 ≠ 5/6
Question 11.
\(\frac{6}{10}\) _______ \(\frac{3}{5}\)
Answer:
\(\frac{6}{10}\) = \(\frac{3}{5}\)
Explanation:
6/10
Divide the numerator and denominator of 6/10 with 2
(6 ÷ 2)/( 10 ÷ 2) = 3/5
So, 6/10 = 3/5
Question 12.
\(\frac{3}{12}\) _______ \(\frac{1}{3}\)
Answer:
\(\frac{3}{12}\) ≠ \(\frac{1}{3}\)
Explanation:
3/12 ≠ 1/3
Question 13.
\(\frac{6}{10}\) _______ \(\frac{60}{100}\)
Answer:
\(\frac{6}{10}\) = \(\frac{60}{100}\)
Explanation:
6/10
Multiply the numerator and denominator of 6/10 with 10
(6 x 10)/(10 x 10) = 60/100
So, 6/10 = 60/100
Question 14.
\(\frac{11}{12}\) _______ \(\frac{9}{10}\)
Answer:
\(\frac{11}{12}\) ≠ \(\frac{9}{10}\)
Explanation:
11/12 ≠ 9/10
Question 15.
\(\frac{2}{5}\) _______ \(\frac{8}{20}\)
Answer:
\(\frac{2}{5}\) = \(\frac{8}{20}\)
Explanation:
2/5
Multiply the numerator and denominator of 2/5 with 4
(2 x 4)/(5 x 4) = 8/20
So, 2/5 = 8/20
Question 16.
\(\frac{4}{8}\) _______ \(\frac{1}{2}\)
Answer:
\(\frac{4}{8}\) = \(\frac{1}{2}\)
Explanation:
4/8
Divide the numerator and denominator of 4/8 with 4
(4 x 4)/(8 x 4) = 1/2
So, 4/8 = 1/2
Question 17.
At Memorial Hospital, 9 of the 12 babies born on Tuesday were boys. In simplest form, what fraction of the babies born on Tuesday were boys?
_______
Answer:
\(\frac{3}{4}\)
Explanation:
As per the given data,
At memorial hospital, 9 of the 12 babies born on Tuesday were boys = 9/12
Divide the numerator and denominator of 9/12 with 3
(9 ÷ 3)/(12 ÷ 3) = 3/4
So, in the simplest form
3/4 of the babies born on Tuesday were boys
Question 18.
Cristina uses a ruler to measure the length of her math textbook. She says that the book is \(\frac{4}{10}\) meter long. Is her measurement in simplest form? If not, what is the length of the book in simplest form?
\(\frac{□}{□}\)
Answer:
\(\frac{2}{5}\)
Explanation:
As per the given data,
Cristiana uses a ruler to measure the length of her math textbook
She says that the book is 4/10meter long
It is not in simplest form
Divide the numerator and denominator of 4/10 with 2
(4÷ 2)/( 10 ÷ 2) = 2/5
The length of the book in the simplest form = 2/5
Common Core – Simplest Form – Page No. 344
Question 1.
Six out of the 12 members of the school choir are boys. In simplest form, what fraction of the choir is boys?
Options:
a. \(\frac{1}{6}\)
b. \(\frac{6}{12}\)
c. \(\frac{1}{2}\)
d. \(\frac{12}{6}\)
Answer:
c. \(\frac{1}{2}\)
Explanation:
As per the given data,
Six out of the 12 members of the school choir are boys = 6/12
To write the simplest form of 6/12, divide the numerator and denominator with 6
Then, (6 ÷ 6)/(12 ÷ 6) = 1/2
In simplest form, 1/2 of the choir is boys
Question 2.
Which of the following fractions is in simplest form?
Options:
a. \(\frac{5}{6}\)
b. \(\frac{6}{8}\)
c. \(\frac{8}{10}\)
d. \(\frac{2}{12}\)
Answer:
a. \(\frac{5}{6}\)
Explanation:
5/6 is in the simplest form
6/8 simplest form = 3/4
8/10 simplest form = 4/5
2/12 simplest form = 1/6
Question 3.
Each of the 23 students in Ms. Evans’ class raised $45 for the school by selling coupon books. How much money did the class raise in all?
Options:
a. $207
b. $225
c. $1,025
d. $1,035
Answer:
d. $1,035
Explanation:
As per the given data,
Each of the 23 students in Ms. Evan’s class raised $45 for the school by selling coupon books
= 23 x $45
= $1,035
Question 4.
Which pair of numbers below have 4 and 6 as common factors?
Options:
a. 12, 18
b. 20, 24
c. 28, 30
d. 36, 48
Answer:
d. 36, 48
Explanation:
36, 48
Here, 36 = 4 x 9
= 2 x 2 x 3 x 3
48 = 6 x 8
= 2 x 3 x 4 x 2
Question 5.
Bart uses \(\frac{3}{12}\) cup milk to make muffins. Which fraction is equivalent to \(\frac{3}{12}\)?
Options:
a. \(\frac{1}{4}\)
b. \(\frac{1}{3}\)
c. \(\frac{1}{2}\)
d. \(\frac{2}{3}\)
Answer:
a. \(\frac{1}{4}\)
Explanation:
As per the given data,
Bart uses 3/12 cup milk to make muffins
Divide the fraction with 3
(3 ÷ 3)/(12 ÷ 3) = 1/4
So, the equivalent fraction for 3/12 = 1/4
Question 6.
Ashley bought 4 packages of juice boxes. There are 6 juice boxes in each package. She gave 2 juice boxes to each of 3 friends. How many juice boxes does Ashley have left?
Options:
a. 24
b. 22
c. 18
d. 12
Answer:
c. 18
Explanation:
As per the given data,
Ashley bought 4 packages of juice boxes
There are 6 juice boxes in each package = 6 x 4 = 24
She gave 2 juice boxes to each of 3 friends = 2 x 3 = 6 juice boxes
So, 24 – 6 = 18
Total number of juice boxes left with Ashley = 18
Page No. 347
Question 1.
Find a common denominator for \(\frac{1}{3}\) and \(\frac{1}{12}\) by dividing each whole into the same number of equal parts. Use the models to help.
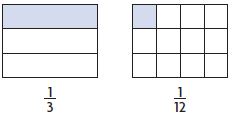
common denominator:
Answer:
common denominator: 12
Explanation:
List the multiples of 3 = 3, 6, 9, 12, 15, 18, 21, ….
List the multiples of 12 = 12, 24, 36, 48, ….
So, common denominators of 1/3 and 1/ 12 is 12
Write the pair of fractions as a pair of fractions with a common denominator.
Question 2.
\(\frac{1}{2}\) and \(\frac{1}{4}\)
Type below:
_________
Answer:
\(\frac{4}{8}\) and \(\frac{2}{8}\)
Explanation:
Common denominator of 1/2 and 1/4
List the multiples of 2 = 2, 4, 6, 8, 10, …
List the multiples of 4 = 4, 8, 12, 16, 20, 24, . . .
Then, the common denominator of 1/2 and 1/4 is 4
For the Common pair of fractions, multiply the common denominator with fractions
That is, (1 x 4) ÷( 2 x 4) and ( 1 x 4 ) ÷ ( 4 x 4)
So, the common pair of fractions = 4/8 and 2/8
Question 3.
\(\frac{3}{4}\) and \(\frac{5}{8}\)
Type below:
_________
Answer:
\(\frac{6}{8}\) and \(\frac{5}{8}\)
Explanation:
Common denominator of 3/4 and 5/8
List the multiples of 4 = 4, 8, 12, 16, 20, 24, . . .
List the multiples of 8 = 8, 16, 24, 32, . . . .
Then, the common denominator of 3/4 and 5/8 is 8
For the Common pair of fractions, multiply the common denominator with fractions
That is, (3 x 8) ÷( 4 x 8) and ( 5 x 8 ) ÷ ( 8 x 8)
So, the common pair of fractions = 6/8 and 5/8
Question 4.
\(\frac{1}{3}\) and \(\frac{1}{4}\)
Type below:
_________
Answer:
\(\frac{4}{12}\) and \(\frac{3}{12}\)
Explanation:
The common denominator of 1/3 and 1/4
List the multiples of 3 = 3, 6, 9, 12, 15, 18, 21, 24, ….
List the multiples of 4 = 4, 8, 12, 16, 20, 24, . . .
Then, the common denominator of 1 /3 and 1/4 is 12
For the Common pair of fractions, multiply the common denominator with fractions
That is, (1 x 12) ÷( 3 x 12) and ( 1 x 12 ) ÷ ( 4 x 12)
So, the common pair of fractions = 4/12 and 3/12
Question 5.
\(\frac{4}{12}\) and \(\frac{5}{8}\)
Type below:
_________
Answer:
\(\frac{8}{24}\) and \(\frac{15}{24}\)
Explanation:
Common denominator of 4/12 and 5/8
List the multiples of 12 = 12, 24, 36, 48, 60, …..
List the multiples of 8 = 8, 16, 24, 32, 40, 48, …
Then, the common denominator of 4/12 and 5/8 is 24
For the Common pair of fractions, multiply the common denominator with fractions
That is, (4 x 24) ÷( 12 x 24) and ( 5 x 24 ) ÷ ( 8 x 24)
So, the common pair of fractions = 8/24 and 15/24
Write the pair of fractions as a pair of fractions with a common denominator.
Question 6.
\(\frac{1}{4}\) and \(\frac{5}{6}\)
Type below:
_________
Answer:
\(\frac{3}{12}\) and \(\frac{10}{12}\)
Explanation:
The common denominator of 1/4 and 5/6
List the multiples of 4 = 4, 8, 12, 16, 20, 24, . . .
List the multiples of 6 = 6, 12, 18, 24, 30, 36, ….
Then, the common denominator of 1/4 and 5/6 is 12
For the Common pair of fractions, multiply the common denominator with fractions
That is, (1 x 12) ÷( 4 x 12) and ( 5 x 12 ) ÷ ( 6 x 12)
So, common pair of fractions = 3/12 and 10/12
Question 7.
\(\frac{3}{5}\) and \(\frac{4}{10}\)
Type below:
_________
Answer:
\(\frac{6}{10}\) and \(\frac{4}{10}\)
Explanation:
Common denominator of 3/5 and 4/10
List the multiples of 5 = 5, 10, 15, 20, 25, 30, …..
List the multiples of 10 = 10, 20, 30, 40, 50 ….
Then, the common denominator of 3/5 and 4/10 is 10
For the Common pair of fractions, multiply the common denominator with fractions
That is, (3 x 10) ÷( 5 x 10) and ( 4 x 10 ) ÷ ( 10 x 10)
So, the common pair of fractions = 6/10 and 4/10
Tell whether the fractions are equivalent. Write = or ≠.
Question 8.
\(\frac{3}{4}\) ______ \(\frac{1}{2}\)
Answer:
\(\frac{3}{4}\) ≠ \(\frac{1}{2}\)
Explanation:
3/4 ≠ 1/2
Question 9.
\(\frac{3}{4}\) ______ \(\frac{6}{8}\)
Answer:
\(\frac{3}{4}\) = \(\frac{6}{8}\)
Explanation:
3/4
Multiply the numerator and denominator of 3/4 with 2
(3 x 2) ÷ ( 4 x 2 ) = 6/8
So, 3/4 = 6/8
Question 10.
\(\frac{1}{2}\) ______ \(\frac{4}{8}\)
Answer:
\(\frac{1}{2}\) = \(\frac{4}{8}\)
Explanation:
1/2
Multiply the numerator and denominator of 1/2 with 4
(1 x 4) ÷ ( 2 x 4 ) = 4/8
So, 1/2 = 4/8
Question 11.
\(\frac{6}{8}\) ______ \(\frac{4}{8}\)
Answer:
\(\frac{6}{8}\) ≠ \(\frac{4}{8}\)
Explanation:
6/8 ≠ 4/8
Question 12.
Jerry has two same-size circles divided into the same number of equal parts. One circle has \(\frac{3}{4}\) of the parts shaded, and the other has \(\frac{2}{3}\) of the parts shaded. His sister says the least number of pieces each circle could be divided into is 7. Is his sister correct? Explain.
______
Answer:
As per the given data,
Jerry has two same size circles divided into the same number of equal parts
One circle has 3/4 of the parts shaded
So, non- shaded parts of one circle = 1 – 3/4 = 1/4
Another circle has 2/3 of the parts shaded
Non – shaded parts = 1 – 2/3 = 1/3
We can’t draw a conclusion that in how many parts or pieces a circle can be divided
So, his sister is incorrect
Page No. 348
Question 13.
Carrie has a red streamer that is \(\frac{3}{4}\) yard long and a blue streamer that is \(\frac{5}{6}\) yard long. She says the streamers are the same length. Does this make sense? Explain.

______
Answer:
Carrie has a red streamer that is 3/4 yard long
The blue streamer that is 5/6 yard long
3/4 ≠ 5/6
She says the streamers are the same length, it doesn’t make any sense.
Question 14.
Leah has two same-size rectangles divided into the same number of equal parts. One rectangle has \(\frac{1}{3}\) of the parts shaded, and the other has \(\frac{2}{5}\) of the parts shaded. What is the least number of parts into which both rectangles could be divided?
______ parts
Answer:
15 parts
Explanation:
As per the given data,
Leah has two same size rectangles divided into the same number of equal parts
One rectangle has 1/3 of the parts shaded
Other rectangle has 2/5 of the parts shaded
15 parts
Question 15.
Julian says a common denominator for \(\frac{3}{4}\) and \(\frac{2}{5}\) is 9. What is Julian’s error? Explain.
Type below:
___________
Answer:
As per the given data,
Julian says a common denominator for 3/4 and 2/5 is 9
To find the common denominator for 3/4 and 2/5
List the multiples of 4 = 4, 8, 12, 16, 20, 24, 28, 32, …..
List the multiples of 5 = 5, 10, 15, 20, 25, 30, ….
So, the common denominator for 3/4 and 2/5 is 20
Julian says 9 in place of 20 and it is wrong.
Question 16.
Miguel has two same-size rectangles divided into the same number of equal parts. One rectangle has \(\frac{3}{4}\) of the parts shaded, and the other has \(\frac{5}{8}\) of the parts shaded.
Into how many parts could each rectangle be divided? Show your work by sketching the rectangles.
______ parts
Answer:

8 parts
Explanation:
As per the given data,
Miguel has two same – size rectangles divided into the same number of equal parts.
One rectangle has 3/4 of the parts shaded.
Another has 5/8 of the parts shaded.
The possible parts are 8.
Common Core – Common Denominators – Page No. 349
Write the pair of fractions as a pair of fractions with a common denominator.
Question 1.
\(\frac{2}{3} \text { and } \frac{3}{4}\)
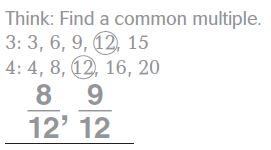
Answer:
\(\frac{8}{12} \text { and } \frac{9}{12}\)
Explanation:
2/3 and 3/4
List the multiples of 3 = 3, 6, 9, 12, 15, 18, 21, 24, …
List the multiples of 4 = 4, 8, 12, 16, 20, …
Common multiple of 3 and 4 is 12
For the Common pair of fractions, multiply the common denominator with fractions
That is, (2 x 12) ÷( 3 x 12) and ( 3 x 12 ) ÷ ( 4 x 12)
So, common pair of fractions = 8/12 and 9/12
Question 2.
\(\frac{1}{4} \text { and } \frac{2}{3}\)
Type below:
_________
Answer:
\(\frac{3}{12} \text { and } \frac{8}{12}\)
Explanation:
1/4 and 2/3
List the multiples of 4 = 4, 8, 12, 16, 20, …
List the multiples of 3 = 3, 6, 9, 12, 15, 18, …
Common multiple of 4 and 3 is 12
For the Common pair of fractions, multiply the common denominator with fractions
That is, (1 x 12) ÷( 4 x 12) and ( 2 x 12 ) ÷ ( 3 x 12)
So, common pair of fractions = 3/12 and 8/12
Question 3.
\(\frac{3}{10} \text { and } \frac{1}{2}\)
Type below:
_________
Answer:
\(\frac{3}{10} \text { and } \frac{5}{10}\)
Explanation:
3/10 and 1/2
List the multiples of 10 = 10, 20, 30, 40, 50, ….
List the multiples of 2 = 2, 4, 6, 8, 10, 12, 14, ….
Common multiple of 10 and 2 is 10
For the Common pair of fractions, multiply the common denominator with fractions
That is, (3 x 10) ÷( 10 x 10) and ( 1 x 10 ) ÷ ( 2 x 10)
So, common pair of fractions = 3/10 and 5/10
Question 4.
\(\frac{3}{5} \text { and } \frac{3}{4}\)
Type below:
_________
Answer:
\(\frac{12}{20} \text { and } \frac{15}{20}\)
Explanation:
3/5 and 3/4
List the multiples of 5 = 5, 10, 15, 20, 25, 30, ….
List the multiples of 4 = 4, 8, 12, 16, 20, 24, …
Common multiple of 5 and 4 is 20
For the Common pair of fractions, multiply the common denominator with fractions
That is, (3 x 20) ÷( 5 x 20) and ( 3 x 20 ) ÷ ( 4 x 20)
So, common pair of fractions = 12/20 and 15/20
Question 5.
\(\frac{2}{4} \text { and } \frac{7}{8}\)
Type below:
_________
Answer:
\(\frac{4}{8} \text { and } \frac{7}{8}\)
Explanation:
2/4 and 7/8
List the multiples of 4 = 4, 8, 12, 16, 20, 24, …
List the multiples of 8 = 8, 16, 24, 32, 40, ….
Common multiple of 4 and 8 is 8
For the Common pair of fractions, multiply the common denominator with fractions
That is, (2 x 8) ÷( 4 x 8) and ( 7 x 8 ) ÷ ( 8 x 8)
So, common pair of fractions = 4/8 and 7/8
Question 6.
\(\frac{2}{3} \text { and } \frac{5}{12}\)
Type below:
_________
Answer:
\(\frac{8}{12} \text { and } \frac{5}{12}\)
Explanation:
2/3 and 5/12
List the multiples of 3 = 3, 6, 9, 12, 15, 18, …
List the multiples of 12 = 12, 24, 36, 48, 60, …
Common multiple of 3 and 12 is 12
For the Common pair of fractions, multiply the common denominator with fractions
That is, (2 x 12) ÷( 3 x 12) and ( 5 x 12 ) ÷ ( 12 x 12)
So, common pair of fractions = 8/12 and 5/12
Question 7.
\(\frac{1}{4} \text { and } \frac{1}{6}\)
Type below:
_________
Answer:
\(\frac{3}{12} \text { and } \frac{2}{12}\)
Explanation:
1/4 and 1/6
List the multiples of 4 = 4, 8, 12, 16, 20, 24, …
List the multiples of 6 = 6, 12, 18, 24, 30, …
Common multiple of 4 and 6 is 12
For the Common pair of fractions, multiply the common denominator with fractions
That is, (1 x 12) ÷( 4 x 12) and ( 1 x 12 ) ÷ ( 6 x 12)
So, common pair of fractions = 3/12 and 2/12
Tell whether the fractions are equivalent. Write = or ≠.
Question 8.
\(\frac{1}{2}\) ______ \(\frac{2}{5}\)
Answer:
\(\frac{1}{2}\) ≠ \(\frac{2}{5}\)
Explanation:
Multiply the numerator and denominator of 1/2 with 2
(1 x 2) ÷ (2 x 2) = 2/4
So, 1/2 ≠ 2/5
Question 9.
\(\frac{1}{2}\) ______ \(\frac{3}{6}\)
Answer:
\(\frac{1}{2}\) = \(\frac{3}{6}\)
Explanation:
1/2
Multiply the numerator and denominator of 1/2 with 3
(1 x 3) ÷ (2 x 3) = 3/6
So, 1/2 = 3/6
Question 10.
\(\frac{3}{4}\) ______ \(\frac{5}{6}\)
Answer:
\(\frac{3}{4}\) ≠ \(\frac{5}{6}\)
Explanation:
3/4 ≠ 5/6
Question 11.
\(\frac{6}{10}\) ______ \(\frac{3}{5}\)
Answer:
\(\frac{6}{10}\) = \(\frac{3}{5}\)
Explanation:
6/10
Divide the numerator and denominator of 6/10 with 2
(6 ÷ 2)/(10 ÷2) = 3/5
So, 6/10 = 3/5
Question 12.
\(\frac{6}{8}\) ______ \(\frac{3}{4}\)
Answer:
\(\frac{6}{8}\) = \(\frac{3}{4}\)
Explanation:
6/8
Divide the numerator and denominator of 6/8 with 2
(6 ÷2)/(8 ÷2) = 3/4
So, 6/8 = 3/4
Question 13.
\(\frac{3}{4}\) ______ \(\frac{2}{3}\)
Answer:
\(\frac{3}{4}\) ≠ \(\frac{2}{3}\)
Explanation:
3/4 ≠ 2/3
Question 14.
\(\frac{2}{10}\) ______ \(\frac{4}{5}\)
Answer:
\(\frac{2}{10}\) ≠ \(\frac{4}{5}\)
Explanation:
2/10
Divide the numerator and denominator of 2/10 with 2
(2 ÷ 2)/(10 ÷ 2) = 1/5
So, 2/10 ≠ 1/5
Question 15.
\(\frac{1}{4}\) ______ \(\frac{3}{12}\)
Answer:
\(\frac{1}{4}\) = \(\frac{3}{12}\)
Explanation:
1/4
Multiply the numerator and denominator of 1/4 with 3
(1 x 3)/(4 x 3) = 3/12
So, 1/4 = 3/12
Question 16.
Adam drew two same size rectangles and divided them into the same number of equal parts. He shaded \(\frac{1}{3}\) of one rectangle and \(\frac{1}{4}\) of other rectangle. What is the least number of parts into which both rectangles could be divided?
_________
Answer:
12 parts
Explanation:
As per the given data,
Adam drew two same size rectangles and divided them into the same number of equal parts
He shaded 1/3 of one rectangle
1/4 of another rectangle
List the multiples of 3 = 3, 6, 9, 12, 15, 18, …
List the multiples of 4 = 4, 8, 12, 16, 20, …
A common multiple of 3 and 4 is 12
So, the least number of parts which rectangles could be divided = 12 parts
Question 17.
Mera painted equal sections of her bedroom wall to make a pattern. She painted \(\frac{2}{5}\) of the wall white and \(\frac{1}{2}\) of the wall lavender. Write an equivalent fraction for each using a common denominator.
Type below:
_________
Answer:
1/2 are 4/10 and 5/10
Explanation:
As per the given data,
Mera painted equal sections of her bedroom wall to make a pattern
She painted 2/5 of the wall white and 1/2 of the wall lavender
List the multiples of 5 = 5, 10, 15, 20, 25, 30, …
List the multiples of 2 = 2 ,4, 6, 8, 10, 12, 14, …
The common denominator of 2/5 and 1/2 = 10
Multiply the 2/5 and 1/2 with 10
(2 x 10)/(5 x 10) and (1 x 10)/(2 x 10)
4/10 and 5/10
So, common fractions of 2/5 and 1/2 are 4/10 and 5/10
Common Core – Common Denominators – Page No. 350
Question 1.
Which of the following is a common denominator of \(\frac{1}{4}\) and \(\frac{5}{6}\)?
Options:
a. 8
b. 9
c. 12
d. 15
Answer:
c. 12
Explanation:
Common denominator of 1/4 and 5/6
List the multiples of 4 = 4, 8, 12, 16, 20, 24, …
List the multiples of 6 = 6, 12, 18, 24, 30, ….
So, the common denominator of 1/4 and 5/6 is 12
Question 2.
Two fractions have a common denominator of 8. Which of the following could be the two fractions?
Options:
a. \(\frac{1}{2} \text { and } \frac{2}{3}\)
b. \(\frac{1}{4} \text { and } \frac{1}{2}\)
c. \(\frac{3}{4} \text { and } \frac{1}{6}\)
d. \(\frac{1}{2} \text { and } \frac{4}{5}\)
Answer:
b. \(\frac{1}{4} \text { and } \frac{1}{2}\)
Explanation:
As per the given data,
Two fractions have a common denominator of 8
a. 1/2 and 2/3
List the multiples of 2 = 2, 4, 6, 8,10, ….
List the multiples of 3 = 3, 6, 9, 12, …
There is no common denominator of 8 for 1/2 and 2/3
b. 1/4 and 1 /2
List the multiples of 2 = 2, 4, 6, 8,10, ….
List the multiples of 4 = 4, 8, 12, 16, …
Here, the common denominator of 1 /4 and 1 /2 is 8
So, the answer is 1/4 and 1/2
Question 3.
Which number is 100,000 more than seven hundred two thousand, eighty-three?
Options:
a. 703,083
b. 712,083
c. 730,083
d. 802,083
Answer:
d. 802,083
Explanation:
802,083
Question 4.
Aiden baked 8 dozen muffins. How many total muffins did he bake?
Options:
a. 64
b. 80
c. 96
d. 104
Answer:
c. 96
Explanation:
As per the given data,
Aiden baked 8 dozen muffins
1 dozen = 12
then, 8 dozens = 12 x 8 = 96
So, Aiden baked totally 96 muffins
Question 5.
On a bulletin board, the principal, Ms. Gomez, put 115 photos of the fourthgrade students in her school. She put the photos in 5 equal rows. How many photos did she put in each row?
Options:
a. 21
b. 23
c. 25
d. 32
Answer:
b. 23
Explanation:
As per the given data,
On a bulletin board, the principal, Ms. Gomez, put 115 photos of the fourth-grade students in her school
She put the photos in 5 equal rows
Then, number of photos in each row = 115/5 = 23
So, Ms. Gomez put photos in each row = 23
Question 6.
Judy uses 12 tiles to make a mosaic. Eight of the tiles are blue. What fraction, in simplest form, represents the tiles that are blue?
Options:
a. \(\frac{2}{3}\)
b. \(\frac{2}{5}\)
c. \(\frac{3}{4}\)
d. \(\frac{12}{18}\)
Answer:
a. \(\frac{2}{3}\)
Explanation:
As per the given data,
Judy uses 12 tiles to make a mosaic
Eight of the tiles are blue = 8/12
Divide the numerator and denominator of 8/12 with 4
(8 ÷ 4)/(12 ÷ 4) = 2/3
The simplest form of 8/12 is 2/3
Page No. 353
Question 1.
Keisha is helping plan a race route for a 10-kilometer charity run. The committee wants to set up the following things along the course.
Viewing areas: At the end of each half of the course
Water stations: At the end of each fifth of the course
Distance markers: At the end of each tenth of the course
Which locations have more than one thing located there?
First, make a table to organize the information.
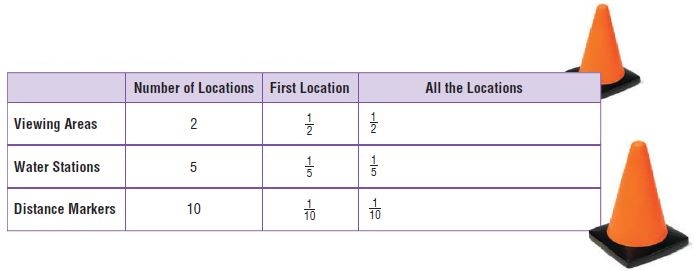
Next, identify a relationship. Use a common denominator, and find equivalent fractions.
Finally, identify the locations at which more than one thing will be set up. Circle the locations.
Type below:
___________
Answer:
Keisha is helping plan a race route for a 10-kilometer charity run.
Question 2.
What if distance markers will also be placed at the end of every fourth of the course? Will any of those markers be set up at the same location as another distance marker, a water station, or a viewing area? Explain.
Type below:
___________
Answer:
It really depends on where you place the other markers.
Question 3.
Fifty-six students signed up to volunteer for the race. There were 4 equal groups of students, and each group had a different task.
How many students were in each group?
_____ students
Answer:
14 students
Explanation:
As per the given data,
Fifty-six students signed up to volunteer for the race
There are four groups of students
Number of students in each group = 56/4 = 14
Total number of students in each group = 14
Page No. 354
Question 4.
A baker cut a pie in half. He cut each half into 3 equal pieces and each piece into 2 equal slices. He sold 6 slices. What fraction of the pie did the baker sell?
\(\frac{□}{□}\)
Answer:
\(\frac{1}{2}\)
Explanation:
A baker cut a pie in half. He cut each half into 3 equal pieces and each piece into 2 equal slices. He sold 6 slices. So, the remaining part is 1/2 of the pie.
Question 5.
Andy cut a tuna sandwich and a chicken sandwich into a total of 15 same-size pieces. He cut the tuna sandwich into 9 more pieces than the chicken sandwich. Andy ate 8 pieces of the tuna sandwich. What fraction of the tuna sandwich did he eat?
\(\frac{□}{□}\)
Answer:
\(\frac{2}{3}\)
Explanation:
Let x be the number of pieces of the chicken sandwich so that x + 9 is the number of pieces of a tuna sandwich.
There is a total of 15 same-size pieces. So, we can write as
x + (x + 9) = 15
2x + 9 = 15
2x = 6
x = 3.
This means that there ate 3 + 9 = 12 pieces of a tuna sandwich. Since Andy ate 8, then this corresponds to a fraction of 8/12 = 2/3.
Question 6.
Luke threw balls into these buckets at a carnival. The number on the bucket gives the number of points for each throw. What is the least number of throws needed to score exactly 100 points? Explain.

_____ throws
Answer:
13 throws
Explanation:
Take the maximum number to get the minimum throws = 9 X 10 = 90.
6 X 1 = 6; 2 X 2 = 4.
Add 90 + 6 + 4 = 100;
So, the least number of throws needed to score exactly 100 points = 10 + 1 + 2 = 13.
Question 7.
Victoria arranges flowers in vases at her restaurant. In each arrangement, \(\frac{2}{3}\) of the flowers are yellow. What other fractions can represent the part of the flowers that are yellow? Shade the models to show your work.
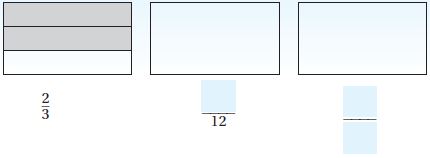
\(\frac{□}{□}\)
Answer:
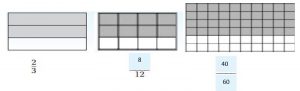
\(\frac{2}{3}\), \(\frac{8}{12}\), \(\frac{40}{60}\)
Explanation:
Basically, any fraction obtained by multiplying both the numerator and denominator by the same value would be an equivalent fraction:
2/3 = 2/3 * 4/4 = 8/12
8/12 = 8/12 * 5/5 = 40/60
etc.
Common Core – Find Equivalent Fractions – Page No. 355
Question 1.
Miranda is braiding her hair. Then she will attach beads to the braid. She wants \(\frac{1}{3}\) of the beads to be red. If the greatest number of beads that will fit on the braid is 12, what other fractions could represent the part of the beads that are red?
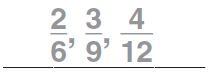
Answer:
\(\frac{2}{6}\), \(\frac{3}{9}\), \(\frac{4}{12}\)
Explanation:
Miranda is braiding her hair. Then she will attach beads to the braid. She wants \(\frac{1}{3}\) of the beads to be red. If the greatest number of beads that will fit on the braid is 12.
\(\frac{1}{3}\) X \(\frac{2}{2}\) = \(\frac{2}{6}\)
\(\frac{1}{3}\) X \(\frac{3}{3}\) = \(\frac{3}{9}\)
\(\frac{1}{3}\) X \(\frac{4}{4}\) = \(\frac{4}{12}\)
Question 2.
Ms. Groves has trays of paints for students in her art class. Each tray has 5 colors. One of the colors is purple. What fraction of the colors in 20 trays is purple?
\(\frac{□}{□}\)
Answer:
\(\frac{20}{100}\) or \(\frac{1}{5}\)
Explanation:
If you have 20 trays that are 100 colors with 20 being purple. 20/ 100 is 1/5
Question 3.
Miguel is making an obstacle course for field day. At the end of every sixth of the course, there is a tire. At the end of every third of the course, there is a cone. At the end of every half of the course, there is a hurdle. At which locations of the course will people need to go through more than one obstacle?
Type below:
_________
Answer:
\(\frac{1}{3}\), \(\frac{1}{2}\), \(\frac{2}{3}\) and final locations
Explanation:
We have three fractions with different denominators: sixths, thirds, and halves.
The first step is to make all the denominators equal for 1/6, 1/3, 1/2.
In this case, we want sixths since LCM(2, 3, 6) = 6
since 1/3 = 2/6, and 1/2 = 3/6. Now we can start solving.
1. There are six tires at the following: 1/6, 2/6, 3/6, 4/6, 5/6, and 6/6.
2. There are three cones at the following (G.C.F.): 2/6 (or 1/3), 4/6 (or 2/3), and 6/6 (or 3/3).
3. There are two hurdles at the following (G.C.F.): 3/6 (or 1/2) and 6/6 (or 2/2).
We look for common numbers.
1. On 2/6, there are two obstacles: a tire and a cone.
2. On 3/6, there are two obstacles: a tire and a hurdle.
3. On 4/6, there are two obstacles: a tire and a cone.
4. At 6/6, there are three obstacles: a tire, cone, and a hurdle.
2/6 = 1/3
3/6 = 1/2
4/6 = 2/3
6/6 = 1
The answers are 1/3, 1/2, 2/3, and 1.
Question 4.
Preston works in a bakery where he puts muffins in boxes. He makes the following table to remind himself how many blueberry muffins should go in each box.

How many blueberry muffins should Preston put in a box with 36 muffins?
_________
Answer:
12 blueberry muffins
Explanation:
Preston works in a bakery where he puts muffins in boxes. He makes the following table to remind himself how many blueberry muffins should go in each box.
So, he had 2 blueberry muffins out of 6 muffins.
2/6 X 2/2 = 4/12. 4 blueberry muffins out of 12 muffins.
2/6 X 4/4 = 8/24. 8 blueberry muffins out of 24 muffins.
2/6 X 6/6 = 12/36. 12 blueberry muffins out of 36 muffins.
Common Core – Find Equivalent Fractions – Page No. 356
Question 1.
A used bookstore will trade 2 of its books for 3 of yours. If Val brings in 18 books to trade, how many books can she get from the store?
Options:
a. 9
b. 12
c. 18
d. 27
Answer:
b. 12
Explanation:
A used bookstore will trade 2 of its books for 3 of yours. If Val brings in 18 books to trade 2/3 X 6/6 = 12/18, she get 12 books
Question 2.
Every \(\frac{1}{2}\) hour Naomi stretches her neck; every \(\frac{1}{3}\) hour she stretches her legs; and every \(\frac{1}{6}\) hour she stretches her arms. Which parts of her body will Naomi stretch when \(\frac{2}{3}\) of an hour has passed?
Options:
a. neck and legs
b. neck and arms
c. legs and arms
d. none
Answer:
c. legs and arms
Explanation:
Summing \(\frac{1}{2}\)‘s only gives integer values giving 1, 2, 3, 4…or
integer values +\(\frac{1}{2}\) and 0 + \(\frac{1}{2}\) = \(\frac{1}{2}\), 1 \(\frac{1}{2}\), 2 \(\frac{1}{2}\)…
So neck is excluded
Every \(\frac{1}{3}\): \(\frac{1}{3}\) + \(\frac{1}{2}\) = \(\frac{2}{3}\)
Legs will be stretched at \(\frac{2}{3}\) hour
Every \(\frac{1}{6}\): \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) = \(\frac{4}{6}\)
Divide top and bottom by 2 giving:
(4 ÷ 2)/(6 ÷ 2) = \(\frac{2}{3}\)
Arms will be stretched at latex]\frac{2}{3}[/latex] hour
Question 3.
At the beginning of the year, the Wong family car had been driven 14,539 miles. At the end of the year, their car had been driven 21,844 miles. How many miles did the Wong family drive their car during that year?
Options:
a. 6,315 miles
b. 7,295 miles
c. 7,305 miles
d. 36,383 miles
Answer:
c. 7,305 miles
Explanation:
If at the beginning of the year, the Wong family’s car had driven 14539 miles and at the end of the year, it had driven 21844 miles, then subtract 14539 from 21844 to determine the difference between the two values, which will tell you how many miles the Wong family drove their car for during the year.
21844 – 14539 = 7305 miles
Question 4.
Widget Company made 3,600 widgets in 4 hours. They made the same number of widgets each hour. How many widgets did the company make in one hour?
Options:
a. 80
b. 90
c. 800
d. 900
Answer:
d. 900
Explanation:
3,600 widgets in 4 hours therefore 3,600 / 4 for one hour = 900 widgets 900 widgets in one hour.
Question 5.
Tyler is thinking of a number that is divisible by 2 and by 3. By which of the following numbers must Tyler’s number also be divisible?
Options:
a. 6
b. 8
c. 9
d. 12
Answer:
a. 6
Explanation:
The number 6 is divisible by 2 and by 3.
Question 6.
Jessica drew a circle divided into 8 equal parts. She shaded 6 of the parts. Which fraction is equivalent to the part of the circle that is shaded?
Options:
a. \(\frac{2}{3}\)
b. \(\frac{3}{4}\)
c. \(\frac{10}{16}\)
d. \(\frac{12}{18}\)
Answer:
b. \(\frac{3}{4}\)
Explanation:
Jessica drew a circle divided into 8 equal parts. She shaded 6 of the parts.
6/8 = 3/4
Page No. 357
Choose the best term from the box.

Question 1.
________ name the same amount.
________
Answer:
Equivalent Fractions
Question 2.
A _________ is a common multiple of two or more denominators
________
Answer:
Common Denominator
Write two equivalent fractions.
Question 3.
\(\frac{2}{5}\)
Type below:
________
Answer:
\(\frac{4}{10}\) and \(\frac{6}{15}\)
Explanation:
Two equivalent fractions of 2/5
Multiply the 2/5 with 2
(2 x 2)/(5 x 2) = 4/10
Multiply the 2/5 with 3
(2 x 3)/(5 x 3) = 6/15
So, the equivalent fractions of 2/5 are 4/10 and 6/15
Question 4.
\(\frac{1}{3}\)
Type below:
________
Answer:
\(\frac{2}{6}\) and \(\frac{3}{9}\)
Explanation:
Two equivalent fractions of 1/3
Multiply the 1/3 with 2
(1 x 2)/(3 x 2) = 2/6
Multiply the 1/3 with 3
(1 x 3)/(3 x 3) = 3/9
So, the equivalent fractions of 1/3 are 2/6 and 3/9
Question 5.
\(\frac{3}{4}\)
Type below:
________
Answer:
\(\frac{6}{8}\) and \(\frac{9}{12}\)
Explanation:
Two equivalent fractions of 3/4
Multiply the 3/4 with 2
(3 x 2)/(4 x 2) = 6/8
Multiply the 3/4 with 3
(3 x 3)/(4 x 3) = 9/12
So, the equivalent fractions of 3/4 are 6/8 and 9/12
Tell whether the fractions are equivalent. Write = or ≠.
Question 6.
\(\frac{2}{3}\) ______ \(\frac{4}{12}\)
Answer:
\(\frac{2}{3}\) ≠ \(\frac{4}{12}\)
Explanation:
2/ 3
Multiply the numerator and denominator of 2/3 with 2
(2 x 2)/(3 x 2) = 4/6
So, 2/3 ≠ 4/12
Question 7.
\(\frac{5}{6}\) ______ \(\frac{10}{12}\)
Answer:
\(\frac{5}{6}\) =_ \(\frac{10}{12}\)
Explanation:
5/6
Multiply the 5/6 with 2
(5 x 2)/(6 x 2) = 10/12
So, 5/6 = 10/12
Question 8.
\(\frac{1}{4}\) ______ \(\frac{4}{8}\)
Answer:
\(\frac{1}{4}\) ≠ \(\frac{4}{8}\)
Explanation:
1/4
Multiply the numerator and denominator of 1/4 with 4
(1 x 4)/(4 x 4) = 4/16
So, 1/4 ≠ 4/8
Write the fraction in simplest form.
Question 9.
\(\frac{6}{8}\)
\(\frac{□}{□}\)
Answer:
\(\frac{3}{4}\)
Explanation:
6/8
Divide the numerator and denominator of 6/8 with 2
(6 ÷ 2)/( 8 ÷ 2) = 3/4
The simplest form of 6/8 is 3/4
Question 10.
\(\frac{25}{100}\)
\(\frac{□}{□}\)
Answer:
\(\frac{1}{4}\)
Explanation:
25/100
Divide the numerator and denominator of 25/100 with 25
(25 ÷ 25)/( 100 ÷ 25) = 1/4
The simplest form of 25/100 is 1/4
Question 11.
\(\frac{8}{10}\)
\(\frac{□}{□}\)
Answer:
\(\frac{4}{5}\)
Explanation:
8/10
Divide the numerator and denominator of 8/10 with 2
(8 ÷ 2)/( 10 ÷ 2) = 4/5
The simplest form of 8/10 is 4/5
Write the pair of fractions as a pair of fractions with a common denominator.
Question 12.
\(\frac{3}{10} \text { and } \frac{2}{5}\)
Type below:
_________
Answer:
\(\frac{3}{10} \text { and } \frac{4}{10}\)
Explanation:
3/ 10 and 2/5
List the multiples of 10 = 10, 20, 30, 40, 50, …
List the multiples of 5 = 5, 10, 15, 20, 25, 30, …
Common denominator of 3/10 and 2/5 = 10
Multiply the 3/10 and 2/5 with 10
(3 x 10)/(10 x 10) and (2 x 10)/(5 x 10)
3/ 10 and 4/10
Pair of fractions of 3/10 and 2/5 are 3/10 and 4/10
Question 13.
\(\frac{1}{3} \text { and } \frac{3}{4}\)
Type below:
_________
Answer:
\(\frac{3}{12} \text { and } \frac{9}{12}\)
Explanation:
1/3 and 3/4
List the multiples of 3 = 3, 6, 9, 12, 15, 18, …
List the multiples of 4 = 4, 8, 12, 16, 20, ….
The common denominator of 1/3 and 3/4 are 12
Multiply the 1/3 and 3/4 with 12
(1 x 12)/(3 x 12) and (3 x 12)/(4 x 12)
3/ 12 and 9/12.
Pair of fractions of 1/3 and 3/4 are 3/12 and 9/12
Page No. 358
Question 14.
Sam needs \(\frac{5}{6}\) cup mashed bananas and \(\frac{3}{4}\) cup mashed strawberries for a recipe. He wants to find whether he needs more bananas or more strawberries. How can he write \(\frac{5}{6}\) and \(\frac{3}{4}\) as a pair of fractions with a common denominator?
Type below:
_________
Answer:
\(\frac{10}{12}\) and \(\frac{9}{12}\)
Explanation:
Sam needs 5/6 cup mashed bananas and 3/4 cup mashed strawberries for a recipe
He wants to find whether he needs more bananas or strawberries
List the multiples of 6 = 6, 12, 18, 24, 30, 36, 42,…..
List the multiples of 4 = 4, 8, 12, 16, 20, 24, ….
The common denominator of 6 and 4 is 12
Multiply the numerator and denominator of 5/6 and 3/4 with 12
(5 x 12)/(6 x 12) and (3 x 12)/(4 x 12)
10/12 and 9/12
Pair of fractions with a common denominator for 5/6 and 3/4 are 10/12 and 9/12
Question 15.
Karen will divide her garden into equal parts. She will plant corn in \(\frac{8}{12}\) of the garden. What is the fewest number of parts she can divide her garden into?
______ parts
Answer:
\(\frac{2}{3}\) parts
Explanation:
As per the given data,
Keren will divide her garden into equal parts
She will plant corn in 8/12 of the garden
To get the least number of parts she can divide her garden, simplify the 8/12
Divide the numerator and denominator of 8/12 with 4
(8 ÷ 4)/(12 ÷ 4) = 2/3
So, Karen can divide her garden into 2/3 of parts
Question 16.
Olivia is making scarves. Each scarf will have 5 rectangles, and \(\frac{2}{5}\) of the rectangles will be purple. How many purple rectangles does she need for 3 scarves?
______ purple rectangles
Answer:
6 purple rectangles
Explanation:
As per the given data,
Olivia is making scarves
Each scarf will have 5 rectangles and 2/5 of the rectangles will be purple = 5 x 2/5 = 2
That means each scarf will have 2 purple rectangles
For 3 scarves = 3 x 2 = 6
So, she needs 6 purple rectangles.
Question 17.
Paul needs to buy \(\frac{5}{8}\) pound of peanuts. The scale at the store measures parts of a pound in sixteenths. What measure is equivalent to \(\frac{5}{8}\) pound?
\(\frac{□}{□}\) pound of peanuts
Answer:
\(\frac{10}{16}\) pound of peanuts
Explanation:
As per the given data,
Paul needs to buy 5/8 pounds of peanuts
The scale at the store measures parts of a pound in sixteenths = 16 x 5/8 = 10
To find Equivalent fraction of 5/8
Multiply the numerator and denominator of 5/8 with 2
(5 x 2)/( 8 x 2) = 10/16
So, the equivalent fraction of 5/8 is 10/16
Page No. 361
Question 1.
Compare \(\frac{2}{5}\) and \(\frac{1}{8}\). Write < or >.
\(\frac{2}{5}\) _____ \(\frac{1}{8}\)
Answer:
\(\frac{2}{5}\) > \(\frac{1}{8}\)
Explanation:
Least common denominator of 5 and 8 = 40
Multiply the numerator and denominator of 2/5 and 1/8 with 40
2/ 5 = (2 x 8)/(5 x 8) = 16/40
1/8 = (1 x 5)/(8 x 5) = 5/40
The denominators are same now
So, compare the numerator to find the greater number
16/40 > 5/40
So, 2/5 > 1/8
Compare. Write < or >.
Question 2.
\(\frac{1}{2}\) _____ \(\frac{4}{6}\)
Answer:
\(\frac{1}{2}\) < \(\frac{4}{6}\)
Explanation:
1/2 and 4/6
Least common denominator of 2 and 6 = 6
Multiply the numerator and denominator of 1/2 and 4/6 with 6
1/ 2 = (1 x 6)/(2 x 6) = 6/12
4/ 6 = (4x 2)/(6 x 2) = 8/12
The denominators are same now
So, compare the numerator to find the greater number.
6/12 < 8/12
So, 1/2 < 4/6
Question 3.
\(\frac{3}{10}\) _____ \(\frac{1}{2}\)
Answer:
\(\frac{3}{10}\) > \(\frac{1}{2}\)
Explanation:
1 / 10 and 1/2
Least common denominator of 10 and 2 = 10
Multiply the numerator and denominator of 3/10 and 1/2 with 10
3/ 10 = (3 x 2)/(10 x 2) = 6/20
1/2 = (1 x 10)/(2 x 10) = 10/20
The denominators are same now
So, compare the numerator to find the greater number.
6/20 < 10/20
So, 3/10 > 1/2
Question 4.
\(\frac{11}{12}\) _____ \(\frac{4}{8}\)
Answer:
\(\frac{11}{12}\) > \(\frac{4}{8}\)
Explanation:
11/12 and 4/8
Least common denominator of 12 and 8 = 24
Multiply the numerator and denominator of 11/12 and 4/8 with 24
11/ 12 = (11 x 8)/(12 x 8) = 88/96
4/8 = (4 x 12)/(8 x 12) = 48/96
The denominators are same now
So, compare the numerator to find the greater number
88/96 > 48/96
So, 11/12 > 4/8
Question 5.
\(\frac{5}{8}\) _____ \(\frac{2}{5}\)
Answer:
\(\frac{5}{8}\) > \(\frac{2}{5}\)
Explanation:
5/ 8 and 2/5
Least common denominator of 5 and 8 = 40
Multiply the numerator and denominator of 5/8 and 2/8 with 40
5/ 8 = (5 x 5)/(8 x 5) = 25/40
2/5 = (2 x 8)/(5 x 8) = 16/40
The denominators are same now
So, compare the numerator to find the greater number
25/ 40 > 16/40
So, 5/8 > 2/5
Question 6.
\(\frac{8}{10}\) _____ \(\frac{3}{8}\)
Answer:
\(\frac{8}{10}\) > \(\frac{3}{8}\)
Explanation:
8/10 and 3/8
Least common denominator of 10 and 8 = 40
Multiply the numerator and denominator of 8/10 and 3/8 with 40
8/ 10 = (8 x 8)/(10 x 8) = 64/80
3/8 = (3 x 10)/(8 x 10) = 30/80
The denominators are same now
So, compare the numerator to find the greater number
64/80 > 30/80
So, 8/10 > 3/8
Question 7.
\(\frac{1}{3}\) _____ \(\frac{7}{12}\)
Answer:
\(\frac{1}{3}\) < \(\frac{7}{12}\)
Explanation:
1/3 and 7/12
Least common denominator of 3 and 12 = 12
Multiply the numerator and denominator of 1/3 and 7/12 with 40.
1/ 3 = (1 x 12)/(3 x 12) = 12/36
7/12 = (7 x 3)/(12 x 3) = 21/36
The denominators are same now
So, compare the numerator to find the greater number
12/36 < 21/36
So, 1/3 < 7/12
Question 8.
\(\frac{2}{6}\) _____ \(\frac{7}{8}\)
Answer:
\(\frac{2}{6}\) < \(\frac{7}{8}\)
Explanation:
2/6 and 7/8
Least common denominator of 6 and 8 = 24
Multiply the numerator and denominator of 2/6 and 7/8 with 40
2/ 6 = (2 x 8)/(6 x 8) = 16/48
7/8 = (7 x 6)/(8 x 6) = 42/48
The denominators are same now
So, compare the numerator to find the greater number
16/48<42/48
So, 2/6 < 7/8
Question 9.
\(\frac{4}{8}\) _____ \(\frac{2}{10}\)
Answer:
\(\frac{4}{8}\) > \(\frac{2}{10}\)
Explanation:
4/8 and 2/10
Least common denominator of 8 and 10 = 40
Multiply the numerator and denominator of 4/8 and 2/10 with 40
4/ 8 = (4 x 10)/(8 x 10) = 40/80
2/10 = (2 x 8)/(10 x 8) = 16/80
The denominators are same now
So, compare the numerator to find the greater number
40/80 > 16/80
So, 4/8 > 2/10
Reason Quantitatively Algebra Find a numerator that makes the statement true.
Question 10.
\(\frac{2}{4}<\frac { □ }{ 6 } \)
□ = _____
Answer:
4
Explanation:
2/4 < x/6
Least common denominator of 4 and 6 = 12
Multiply the numerator and denominator of 2/4 < x/6 with 40
2/4 = (2 x 6)/(4 x 6) = 12/24
x/6 = (x x 4)/(6 x 4) = 4 x/24
The denominators are same now
So, compare the numerator to find the greater number
12/24 < 4 X 4/24
Question 11.
\(\frac{8}{10}>\frac { □ }{ 8 } \)
□ = _____
Answer:
1
Explanation:
8/10 < x/8
Least common denominator of 10 and 8 = 40
8/10 = (8 x 4)/(10 x 4) = 32/40
x/8 = (x X 5)/(8 x 5) = 5x/40
The denominators are same now
So, compare the numerator to find the greater number
8/10 < 5x/40. X will be 1
Question 12.
\(\frac{10}{12}>\frac { □ }{ 4 } \)
□ = _____
Answer:
1
Explanation:
10/12 < x/4
Least common denominator of 12 and 4 = 12
10/12 = (10 x 1)/(12 x 1) = 10/12
x/4 = (x X 3)/(4 x 3) = 3x/12
The denominators are same now
So, compare the numerator to find the greater number
10/12 < 3/12. X will be 1.
Question 13.
\(\frac{2}{5}<\frac { □ }{ 10 } \)
□ = _____
Answer:
5
Explanation:
2/5 < x/10
Least common denominator of 5 and 10 = 10
2/5 = (2x 2)/(5 x 2) = 4/10
x/10 = (x X 1)/(10 x 1) = x/10
The denominators are same now
So, compare the numerator to find the greater number
2/5 < 5/10. X will be 5.
Question 14.
When two fractions are between 0 and \(\frac{1}{2}\), how do you know which fraction is greater? Explain.
Type below:
_______
Answer:
When two fractions are between 0 and \(\frac{1}{2}\). \(\frac{1}{2}\) is greater. As the tenths place of 5 is greater than 0. \(\frac{1}{2}\) is greater.
Question 15.
If you know that \(\frac{2}{6}<\frac{1}{2}\) and \(\frac{3}{4}<\frac{1}{2}\), what do you know about \(\frac{2}{6} \text { and } \frac{3}{4}\)?
Type below:
_______
Answer:
Explanation:
As per the given data,
2/6 < 1/2 and 3/4 < 1/2
Then, 2/6 and 3/4 is
The least common denominator of 6 and 4 is 12
(2 x 4)/(6 x 4) and (3 x 6)/(4 x 6)
8/24 and 18/24
Now, the denominators are same, then compare the numerators
8/24 > 18/24
So, 2/6 > 3/4
Question 16.
Sandra has ribbons that are \(\frac{3}{4}\) yard, \(\frac{2}{6}\) yard, \(\frac{1}{5}\) yard, and \(\frac{4}{7}\) yard long. She needs to use the ribbon longer than \(\frac{2}{3}\) yard to make a bow. Which length of ribbon could she use for the bow?
\(\frac{□}{□}\) yard
Answer:
Explanation:
Page No. 362
Question 17.
Saundra ran \(\frac{7}{12}\) of a mile. Lamar ran \(\frac{3}{4}\) of a mile. Who ran farther? Explain.
_______
Answer:
As per the given data,
Saundra ran 7/12 of a mile
Lamar ran 3/4 of a mile
The least common denominator of 7/12 and 3/4 is 12
(7x 1)/( 12 x 1) and ( 3 x 3 )/( 4 x 3)
7/12 and 9/12
So, 7/12 < 9/12
So, 7/12 < 3/4
Lamar ran greater distance than Saundra
Question 18.
What’s the Question? Selena ran farther than Manny.
Type below:
_______
Answer:
Who ran farther? Selena or Manny
Question 19.
Chloe made a small pan of ziti and a small pan of lasagna. She cut the ziti into 8 equal parts and the lasagna into 9 equal parts. Her family ate \(\frac{2}{3}\) of the lasagna. If her family ate more lasagna than ziti, what fraction of the ziti could have been eaten?
Type below:
_______
Answer:
\(\frac{1}{4}\)
Explanation:
As per the given data,
Chloe made a small pan of ziti and a small pan of lasagna
She cut the ziti into 8 equal parts and the lasagna into 9 equal parts
Her family ate 2/3 of the lasagna = (2/3) x 9 = 6 parts
If her family ate more lasagna than ziti, then that is less than 6 parts
So, 1/4 of the ziti = (1/4) x 8 = 2 parts
So, 1/4 of ziti eaten by Chloe family
Question 20.
James, Ella, and Ryan biked around Eagle Lake. James biked \(\frac{2}{10}\) of the distance in an hour. Ella biked \(\frac{4}{8}\) of the distance in an hour. Ryan biked \(\frac{2}{5}\) of the distance in an hour. Compare the distances biked by each person by matching the statements to the correct symbol. Each symbol may be used more than once or not at all.
Type below:
_______
Answer:
2/10 < 4/8
1 / 8 > 2/5
2/10 < 2/5
Explanation:
As per the given data,
James, Ella, and Ryan biked around eagle lake
James biked 2/10 of the distance in an hour
Ella biked 4/8 of the distance in an hour
Ryan biked 2/5 of the distance in an hour
Least common denominator of 2 /10, 4/8, and 2/5 is 40
(2x 4)/(10 x 4), (4 x 5)/(8 x 5), and (2 x 8)/(5 x 8)
8/40, 20/ 40, and 16/ 40
8/40 < 16/40 < 20/40
2/10 < 2/5 < 4/8
So, 2/10 < 4/8
1 / 8 > 2/5
2/10 < 2/5
Common Core – Compare Fractions Using Benchmarks – Page No. 363
Compare. Write < or > .
Question 1.

Answer:
\(\frac{1}{8}\) < \(\frac{6}{10}\)
Explanation:

Question 2.
\(\frac{4}{12}\) _______ \(\frac{4}{6}\)
Answer:
\(\frac{4}{12}\) < \(\frac{4}{6}\)
Explanation:
4/12 and 4/6
4/12 is less than 1/2
4/6 is greater than 1/2
So, 4/12 < 4/6
Question 3.
\(\frac{2}{8}\) _______ \(\frac{1}{2}\)
Answer:
\(\frac{2}{8}\) < \(\frac{1}{2}\)
Explanation:
2/8 and 1/2
2/8 is less than 1/2
1/2 is equal to 1/2
So, 2/8 < 1/2
Question 4.
\(\frac{3}{5}\) _______ \(\frac{3}{3}\)
Answer:
\(\frac{3}{5}\) < \(\frac{3}{3}\)
Explanation:
3/5 and 3/3
3/5 is greater than 1/2
3/3 is equal to 1
So, 3/5 < 3/3
Question 5.
\(\frac{7}{8}\) _______ \(\frac{5}{10}\)
Answer:
\(\frac{7}{8}\) > \(\frac{5}{10}\)
Explanation:
7/8 and 5/10
7/8 is greater than 1/2
5/10 is equal to 1/2
So, 5/10 < 7/8
Question 6.
\(\frac{9}{12}\) _______ \(\frac{1}{3}\)
Answer:
\(\frac{9}{12}\) > \(\frac{1}{3}\)
Explanation:
9/12 and 1/3
9/ 12 is greater than 1/2
1/3 is less than 1/2
1/3 < 9/12
Question 7.
\(\frac{4}{6}\) _______ \(\frac{7}{8}\)
Answer:
\(\frac{4}{6}\) < \(\frac{7}{8}\)
Explanation:
4/6 and 7/8
4/6 is greater than 1/2
7/8 is closer to 1
So, 4/6 < 7/8
Question 8.
\(\frac{2}{4}\) _______ \(\frac{2}{3}\)
Answer:
\(\frac{2}{4}\) < \(\frac{2}{3}\)
Explanation:
2/4 and 2/3
2/4 is equal to 1/2
2/3 is greater than 1/2
So, 2/4 < 2/3
Question 9.
\(\frac{3}{5}\) _______ \(\frac{1}{4}\)
Answer:
\(\frac{3}{5}\) > \(\frac{1}{4}\)
Explanation:
3/5 and 1/4
3/5 is greater than 1/2
1/4 is less than 1/2
So, 1/4 < 3/5
Question 10.
\(\frac{6}{10}\) _______ \(\frac{2}{5}\)
Answer:
\(\frac{6}{10}\) > \(\frac{2}{5}\)
Explanation:
6/10 and 2/5
6/10 is greater than 1/2
2/5 is less than 1/2
So, 2/5 < 6/10
Question 11.
\(\frac{1}{8}\) _______ \(\frac{2}{10}\)
Answer:
\(\frac{1}{8}\) < \(\frac{2}{10}\)
Explanation:
1/8 and 2/10
1/8 is less than 1/2
2/10 is less than 1/2 but greater than 1/8
So, 1/8 < 2/10
Question 12.
\(\frac{2}{3}\) _______ \(\frac{5}{12}\)
Answer:
\(\frac{2}{3}\) > \(\frac{5}{12}\)
Explanation:
2/3 and 5/12
2/3 is greater than 1/2
5/12 is less than 1/2
So, 5/12 < 2/3
Question 13.
\(\frac{4}{5}\) _______ \(\frac{5}{6}\)
Answer:
\(\frac{4}{5}\)< \(\frac{5}{6}\)
Explanation:
4/5 and 5/6
4/5 is greater than 1/2
5/6 is greater than 1/2
Common denominator is 30
(4×6)/(5×6) and (5×5)/(6×5)
24/30 and 25/30
24/30 < 25/30
So, 4/5 < 5/6
Question 14.
\(\frac{3}{5}\) _______ \(\frac{5}{8}\)
Answer:
\(\frac{3}{5}\) < \(\frac{5}{8}\)
Explanation:
3/5 and 5/8
3/5 is greater than 1/2
5/8 is greater than 1/2
Common denominator is 40
(3×8)/(5×8) and (5×5)/(8×5)
24/40 and 25/ 40
24/40 < 25/40
3/5 < 5/8
Question 15.
\(\frac{8}{8}\) _______ \(\frac{3}{4}\)
Answer:
\(\frac{8}{8}\) > \(\frac{3}{4}\)
Explanation:
8/8 and 3/4
8/8 is equal to 1
3/4 is less than 1
3/4 < 8/8
Question 16.
Erika ran \(\frac{3}{8}\) mile. Maria ran \(\frac{3}{4}\) mile. Who ran farther?
_________
Answer:
Maria
Explanation:
As per the data,
Erika ran 3/8 mile
Maria ran 3/4 mile
Multiply the numerator and denominator of 3/4 with 2
(3×2)/(4×2) = 6/8
3/8 < 6/8
So, 3/8 < 3/4
So, Maria ran faster than Erika
Question 17.
Carlos finished \(\frac{1}{3}\) of his art project on Monday. Tyler finished \(\frac{1}{2}\) of his art project on Monday. Who finished more of his art project on Monday?
_________
Answer:
Tyler
Explanation:
From the given data,
Carlos finished 1/3 of his art project on Monday
Tyler finished ½ of his art project on Monday
1/3 is less than 1/2
1/2 is equal to 1/2
So, 1/3 < 1/2
Then, Tyler finished more of his work on Monday
Common Core – Compare Fractions Using Benchmarks – Page No. 364
Question 1.
Which symbol makes the statement true?
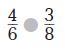
Options:
a. >
b.<
c. =
d. none
Answer:
a. >
Explanation:
4/6 ? 3/8
By comparing 4/6 with 1/2, 4/6 > 1/2
By comparing 3/8 with 1/2, 3/8 < 1/2
So, 4/6 > 3/8
Question 2.
Which of the following fractions is greater than \(\frac{3}{4}\)?
Options:
a. \(\frac{1}{4}\)
b. \(\frac{5}{6}\)
c. \(\frac{3}{8}\)
d. \(\frac{2}{3}\)
Answer:
b. \(\frac{5}{6}\)
Explanation:
From the given data,
By comparing the 3/4 with 1/2, 3/4 > 1/2
Same as above, compare the options with ½
a. 1/4 < 1/2
b. 5/6 > 1/2
c. 3/8 < 1/2
d. 2/3 > 1/2
5/6 and 2/3 are greater than the 1/2
So, compare the 5/6 with 2/3
Then, 5/6 > 2/3
So, 5/6 > 3/4
Question 3.
Abigail is putting tiles on a table top. She needs 48 tiles for each of 8 rows. Each row will have 6 white tiles. The rest of the tiles will be purple. How many purple tiles will she need?
Options:
a. 432
b. 384
c. 336
d. 48
Answer:
c. 336
Explanation:
As per the given data
Abigail is putting tiles on a table top
Number of rows = 8
She needs 48 tiles for each of row = 48×8 = 384
Number of white tiles per row = 6×8 = 48
Rest of the tiles will be purple = 384 – 48 =336
So, the total number of purple color tiles = 336
Question 4.
Each school bus going on the field trip holds 36 students and 4 adults. There are 6 filled buses on the field trip. How many people are going on the field trip?
Options:
a. 216
b. 240
c. 256
d. 360
Answer:
b. 240
Explanation:
From the given data,
Each school bus going on the field trip holds 36 students and 4 adults
There are 6 filled buses on the field trip
6 x (36 + 4) = 6 x 40 = 240
So, the total number of people on the field trip = 240
Question 5.
Noah wants to display his 72 collector’s flags. He is going to put 6 flags in each row. How many rows of flags will he have in his display?
Options:
a. 12
b. 15
c. 18
d. 21
Answer:
a. 12
Explanation:
As mentioned in the data,
Noah wants to display his 72 collector’s flag
He is going to put 6 flags in each row = 6x = 72
X = 12
So, total 12 number of rows of flags will have in his display
Question 6.
Julian wrote this number pattern on the board:
3, 10, 17, 24, 31, 38.
Which of the numbers in Julian’s pattern are composite numbers?
Options:
a. 3, 17, 31
b. 10, 24, 38
c. 10, 17, 38
d. 17, 24, 38
Answer:
b. 10, 24, 38
Explanation:
As per the given information
Julian wrote his number pattern on the board =3, 10, 17, 24, 31, 38
Factors of 3 = 1,3
Factors of 10 = 1,2,5,10
Factors of 17 = 1, 17
Factors of 24 = 1, 2, 3, 4, 6
Factors of 31 = 1, 31
Factors of 38 = 1, 2, 19, 38
So, the composite number is 10, 24, and 38, which numbers have more than 2 factors
Page No. 367
Question 1.
Compare \(\frac{2}{5}\) and \(\frac{1}{10}\).
Think: Use ______ as a common denominator.
\(\frac{2}{5}=\frac { □×□ }{ □×□ } \) = \(\frac{□}{□}\)
\(\frac{1}{10}\)
Think: 4 tenth-size parts ![]() 1 tenth-size part.
1 tenth-size part.
\(\frac{2}{5}\) _____ \(\frac{1}{10}\)
Answer:
\(\frac{2}{5}\) > \(\frac{1}{10}\)
Explanation:
Compare 2/5 and 1/10
Think: 10 as common denominator
Multiply the numerator and denominator of 2/5 with 2
Then, (2×2) ÷ (5×2) = 4/10
Now, compare the 4/10 with 1/10
4/10 > 1/10
So, 2/5 > 1/10
Question 2.
Compare \(\frac{6}{10}\) and \(\frac{3}{4}\).
Think: Use ______ as a common denominator.
\(\frac{6}{10}\)
\(\frac{3}{4}=\frac { □×□ }{ □×□ } \) = \(\frac{□}{□}\)
Think: A tenth-size part ![]() an eighth-size part.
an eighth-size part.
\(\frac{6}{10}\) _____ \(\frac{3}{4}\)
Answer:
\(\frac{6}{10}\) < \(\frac{3}{4}\)
Explanation:
Compare 6/10 and 3/4
Think: Use 40 as a common denominator
So, multiply the denominator and numerator of 3/4 with 10
That is, (3×10) ÷ (4×10) = 30/40
Multiply the numerator and denominator of 6/10 with 4
That is, (6×4) ÷ (10×4) = 24/40
Denominators are same, compare the numerator values of 24/40 and 30/40
So, 24/40 < 30/40
Then, 6/10 < 3/4
Compare. Write <, >, or =.
Question 3.
\(\frac{7}{8}\) _____ \(\frac{2}{8}\)
Answer:
\(\frac{7}{8}\) > \(\frac{2}{8}\)
Explanation:
Compare 7/8 and 2/8
Denominator values are same but numerator values are different
Now, compare the numerator values of 7/8 and 2/8
Then, 7/8 > 2/8
Question 4.
\(\frac{5}{12}\) _____ \(\frac{3}{6}\)
Answer:
\(\frac{5}{12}\) < \(\frac{3}{6}\)
Explanation:
Compare 5/12 and 3/6
Multiply the numerator and denominator of 3/6 with 2
(3×2) ÷ (6×2) = 6/12
So, 5/12 < 6/12
Question 5.
\(\frac{4}{10}\) _____ \(\frac{4}{6}\)
Answer:
\(\frac{4}{10}\) < \(\frac{4}{6}\)
Explanation:
Compare 4/10 and 4/6
Multiply the numerator and denominator of 4/6 with 10
(4×10) ÷ (6×10) = 40/60
Multiply the numerator and denominator of 4/10 with 6
(4×6) ÷ (10×6) = 24/60
So, 24/60 < 40/60
Then, 4/10 < 4/6
Question 6.
\(\frac{6}{12}\) _____ \(\frac{2}{4}\)
Answer:
\(\frac{6}{12}\) = \(\frac{2}{4}\)
Explanation:
Compare 6/12 and 2/4
Multiply the numerator and denominator of 2/4 with 3
(2×3) ÷ (4×3) = 6/12
So, 6/12 = 6/12
Then, 6/12 = 2/4
Question 7.
\(\frac{1}{3}\) _____ \(\frac{1}{4}\)
Answer:
\(\frac{1}{3}\) < \(\frac{1}{4}\)
Explanation:
Compare 1/3 and 1/4
Multiply the numerator and denominator of 1/3 with 4
(1×4) ÷ (3×4) = 4/12
Multiply the numerator and denominator of 1/4 with 3
(1×3) ÷ (4×3) = 3/12
So, 4/12 < 3/12
Then, 1/3 < 1/4
Question 8.
\(\frac{4}{5}\) _____ \(\frac{8}{10}\)
Answer:
\(\frac{4}{5}\) = \(\frac{8}{10}\)
Explanation:
Compare 4/5 and 8/10
Multiply the numerator and denominator of 4/5 with 2
(4×2) ÷ (5×2) = 8/10
So, 8/10 = 8/10
Then, 4/5 = 8/10
Question 9.
\(\frac{3}{4}\) _____ \(\frac{2}{6}\)
Answer:
\(\frac{3}{4}\) < \(\frac{2}{6}\)
Explanation:
Compare 3/4 and 2/6
Multiply the numerator and denominator of 3/4 with 6
(3×6) ÷ (4×6) = 18/24
Multiply the numerator and denominator of 2/6 with 4
(2×4) ÷ (6×4) = 8/24
So, 18/24 < 8/24
Then, 3/4 < 2/6
Question 10.
\(\frac{1}{2}\) _____ \(\frac{5}{8}\)
Answer:
\(\frac{1}{2}\) < \(\frac{5}{8}\)
Explanation:
Compare 1/2 and 5/8
Multiply the numerator and denominator of 1/2 with 4
(1×4) ÷ (2×4) = 4/8
So, 4/8 < 5/8
Then, 1/2 < 5/8
Reason Quantitatively Algebra Find a number that makes the statement true.
Question 11.
\(\frac{1}{2}>\frac { □ }{ 3 } \)
□ = ______
Answer:
1
Explanation:
1/2 > x/3
Multiply the numerator and denominator of 1/2 with 3
(1×3) ÷ (2×3) = 3/6
Multiply the numerator and denominator of x/3 with 2
(Xx2) ÷ (3×2) = 2x/6
3/6 > 2x/6
So, x= 1
Then, 3/6 > 2/6
1/2 > 1/3
Question 12.
\(\frac{3}{10}>\frac { □ }{ 5 } \)
□ = ______
Answer:
1
Explanation:
3/10 > x/5
Multiply the numerator and denominator of x/5 with 2
(Xx2) ÷ (5×2) =2x/10
3/10 > 2x/10
So, x=1
3/10 > 2/10
3/10 > 1/5
Question 13.
\(\frac{5}{12}>\frac { □ }{ 3 } \)
□ = ______
Answer:
1
Explanation:
5/12 > x/3
Multiply numerator and denominator of x/3 with 4
(Xx4) ÷(3×4) = 4x/12
5/12 > 4x/12
So, x = 1
Then, 5/12 > 4/12
5/12 > 1/3
Question 14.
\(\frac{2}{3}>\frac { 4 }{ □ } \)
□ = ______
Answer:
Explanation:
Question 15.
Students cut a pepperoni pizza into 12 equal slices and ate 5 slices. They cut a veggie pizza into 6 equal slices and ate 4 slices. Use fractions to compare the amounts of each pizza that were eaten.
Type below:
_________
Answer:
\(\frac{5}{12}\) < \(\frac{4}{6}\)
Explanation:
As per the given data,
Students cut a pepperoni pizza into 12 equal slices and ate 5 slices
=5/12
They cut veggie pizza into 6 equal slices and ate 4 slices = 4/6
Compare 5/12 and 4/6
Multiply the numerator and denominator of 4/6 with 2
(4×2) ÷ (6×2) = 8/12
So, 5/12 < 8/12
Then, 5/12 < 4/6
Page No. 368
Question 16.
Jerry is making a strawberry smoothie. Which measure is greatest, the amount of milk, cottage cheese, or strawberries?

a. What do you need to find?
Type below:
_________
Answer:
I need to find the greatest measure from milk, cottage cheese, or strawberries
Question 16.
b. How will you find the answer?
Type below:
_________
Answer:
Equal the denominators of 3/4, 2/6, and 8/12
Multiply the numerator and denominator of 3/4 with 3
(3×3) ÷ (4×3) = 9/12
Multiply the numerator and denominator of 2/6 with 2
(2×2) ÷ (6×2) = 4/12
Compare 4/12 < 8/12 < 9/12
So, 2/6 < 8/12 <3/4
Question 16.
c. Show your work.
Type below:
_________
Answer:
2/6 < 8/12 < 3/4
Question 16.
d. Jerry needs more ________ than the other two ingredients.
________
Answer:
Jerry needs more strawberries than the other two ingredients
Question 17.
Angie, Blake, Carlos, and Daisy went running. Angie ran \(\frac{1}{3}\) mile, Blake ran \(\frac{3}{5}\) mile, Carlos ran \(\frac{7}{10}\) mile, and Daisy ran \(\frac{1}{2}\) mile. Which runner ran the shortest distance? Who ran the greatest distance?
The shortest distance: ________
The greatest distance: ________
Answer:
The shortest distance: \(\frac{1}{3}\)
The greatest distance: \(\frac{7}{10}\)
Explanation:
As per the given data,
Angie, Blake, Carlos, and Daisy went running
Angie ran 1/3 mile, Blake ran 3/5 mile, Carlos ran 7/10 mile, and Daisy ran 1/2 mile
Least common denominator of 1/3, 3/5, 7/10, and 1/2 =30
(1x 10)/(3×10), (3×6)/(5×6), (7×3)/(10×3), (1×15)/(2×15)
10/30, 18/30, 21/30, 15/30
10/30 < 15/30 < 18/30 < 21/30
1/3 < 1/2 < 3/5 < 7/10
The shortest distance ran by Angie and that is 1/ 3
The greatest distance ran by Carlos and that is 7/10
Question 18.
Elaine bought \(\frac{5}{8}\) pound of potato salad and \(\frac{4}{6}\) pound of macaroni salad for a picnic. Use the numbers to compare the amounts of potato salad and macaroni salad Elaine bought.

Type below:
_________
Answer:
As per the given data,
Elaine bought 5/8 pound of potato salad and 4/6 pound of macaroni salad for a picnic
Multiply the numerator and denominator of 5/8 with 6
(5×6) / (8×6) = 30/48
Multiply the numerator and denominator of 4/6 with 8
(4×8) / (6×8) = 32/48
30/48 < 32/48
So, 5/8 < 4/6
Elaine bought more macaroni salad than potato salad
Common Core – Compare Fractions – Page No. 369
Compare. Write <, >, or =
Question 1.
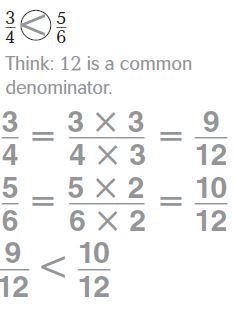
Answer:
\(\frac{1}{5}\) < \(\frac{2}{10}\)
Explanation:
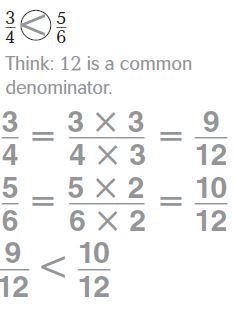
Question 2.
\(\frac{1}{5}\) _____ \(\frac{2}{10}\)
Answer:
\(\frac{1}{5}\) = \(\frac{2}{10}\)
Explanation:
1/5 and 2/10
Think: 10 is a common denominator
1/5 = (1×2) / (5×2) = 2/10
2/10 = 2/10
So, 1/5 = 2/10
Question 3.
\(\frac{2}{4}\) _____ \(\frac{2}{5}\)
Answer:
\(\frac{2}{4}\) > \(\frac{2}{5}\)
Explanation:
2/4 and 2/5
20 is a common denominator
2/4 = (2×5)/(4×5) = 10/20
2/5 = (2×4)/(5×4) = 8/20
10/20 > 8/20
So, 2/4 > 2/5
Question 4.
\(\frac{3}{5}\) _____ \(\frac{7}{10}\)
Answer:
\(\frac{3}{5}\) < \(\frac{7}{10}\)
Explanation:
3/5 and 7/10
10 is a common denominator
3/5 = (3×2)/(5×2) = 6/10
7/10
6/10 < 7/10
So, 3/5 < 7/10
Question 5.
\(\frac{4}{12}\) _____ \(\frac{1}{6}\)
Answer:
\(\frac{4}{12}\) > \(\frac{1}{6}\)
Explanation:
4/12 and 1/6
12 is a common denominator
4/12
1/6 = (1×2)/(6×2) = 2/12
4/12 > 2/12
So, 4/12 > 1/6
Question 6.
\(\frac{2}{6}\) _____ \(\frac{1}{3}\)
Answer:
\(\frac{2}{6}\) = \(\frac{1}{3}\)
Explanation:
2/6 and 1/3
6 is a common denominator
2/6
1/3 = (1×2)/(3×2) = 2/6
So, 2/6 =2/6
So, 2/6 = 1/3
Question 7.
\(\frac{1}{3}\) _____ \(\frac{2}{4}\)
Answer:
\(\frac{1}{3}\) < \(\frac{2}{4}\)
Explanation:
1/3 and 2/4
12 is a common denominator
1/3 = (1×4)/(3×4) = 4/12
2/4 = (2×3)/(4×3) = 6/12
4/12 < 6/12
So, 1/3 < 2/4
Question 8.
\(\frac{2}{5}\) _____ \(\frac{1}{2}\)
Answer:
\(\frac{2}{5}\) < \(\frac{1}{2}\)
Explanation:
2/5 and 1/2
10 is a common denominator
2/5 = (2×2)/(5×2) = 4/10
1/2 = (1×5)/(2×5) = 5/10
4/10 < 5/10
So, 2/5 < 1/2
Question 9.
\(\frac{4}{8}\) _____ \(\frac{2}{4}\)
Answer:
\(\frac{4}{8}\) = \(\frac{2}{4}\)
Explanation:
4/8 and 2/4
8 is a common denominator
4/8
2/4 = (2×2)/(4×2) = 4/8
2/4 = 4/8
So, 4/8 = 2/4
Question 10.
\(\frac{7}{12}\) _____ \(\frac{2}{4}\)
Answer:
\(\frac{7}{12}\) < \(\frac{2}{4}\)
Explanation:
7/12 and 2/4
12 is a common denominator
7/12
2/4 = (2×3)/(4×3) = 6/12
7/12 < 6/12
So, 7/12 < 2/4
Question 11.
\(\frac{1}{8}\) _____ \(\frac{3}{4}\)
Answer:
\(\frac{1}{8}\) < \(\frac{3}{4}\)
Explanation:
1/8 and 3/4
8 is a common denominator
1/8
3/4 = (3×2)/(4×2) = 6/8
1/8 < 6/8
So, 1/8 < 3/4
Question 12.
A recipe uses \(\frac{2}{3}\) of flour and \(\frac{5}{8}\) cup of blueberries. Is there more flour or more blueberries in the recipe?
more _____
Answer:
flour
Explanation:
From the given data,
A recipe uses 2/3 of flour and 5/8 cup of blueberries
Common denominator is 24
2/3 = (2×8)/(3×8) = 16/24
5/8 = (5×3)/(8×3) = 15/24
16/24 > 15/24
So, 2/3 > 5/8
So, flour is more in the recipe
Question 13.
Peggy completed \(\frac{5}{6}\) of the math homework and Al completed \(\frac{4}{5}\) of the math homework. Did Peggy or Al complete more of the math homework?
_________
Answer:
Peggy completed more work than Al
Explanation:
As per the given data,
Peggy completed 5/6 of the math homework
A1 completed 4/5 of the math homework
30 is a common denominator
5/6 = (5×5)/(6×5) = 25/30
4/5 = (4×6)/(5×6) =24/30
25/30 > 24/30
So, 5/6 > 4/5
So, Peggy completed more work than Al
Common Core – Compare Fractions – Page No. 370
Question 1.
Pedro fills a glass \(\frac{2}{4}\) full with orange juice. Which of the following fractions is greater than \(\frac{2}{4}\)?
Options:
a. \(\frac{3}{8}\)
b. \(\frac{4}{6}\)
c. \(\frac{5}{12}\)
d. \(\frac{1}{3}\)
Answer:
b. \(\frac{4}{6}\)
Explanation:
\(\frac{4}{6}\) > \(\frac{2}{4}\)
Question 2.
Today Ian wants to run less than \(\frac{7}{12}\) mile. Which of the following distances is less than \(\frac{7}{12}\) mile?
Options:
a. \(\frac{3}{4}\) mile
b. \(\frac{2}{3}\) mile
c. \(\frac{5}{6}\) mile
d. \(\frac{2}{4}\) mile
Answer:
d. \(\frac{2}{4}\) mile
Explanation:
\(\frac{2}{4}\) is less than \(\frac{7}{12}\)
Question 3.
Ms. Davis traveled 372,645 miles last year on business. What is the value of 6 in 372,645?
Options:
a. 6
b. 60
c. 600
d. 6,000
Answer:
c. 600
Explanation:
Ms. Davis traveled 372, 645 miles last year on business
The value of 6 in 372,645 is 600
Question 4.
One section of an auditorium has 12 rows of seats. Each row has 13 seats. What is the total number of seats in that section?
Options:
a. 25
b. 144
c. 156
d. 169
Answer:
c. 156
Explanation:
From the given information
One section of an auditorium has 12 rows of seats
Each row has 13 seats = 13×12 = 156 seats
So, the total number of seats in the auditorium = 156 seats
Question 5.
Sam has 12 black-and-white photos and 18 color photos. He wants to put the photos in equal rows so each row has either black-and-white photos only or color photos only. In how many rows can Sam arrange the photos?
Options:
a. 1, 2, 3, or 6 rows
b. 1, 3, 6, or 9 rows
c. 1, 2, or 4 rows
d. 1, 2, 3, 4, 6, or 9 rows
Answer:
a. 1, 2, 3, or 6 rows
Explanation:
As per the given information
Sam has 12 black and white photos 18 color photos
He wants to put the photos in equal rows
So each row has either black and white photos only or color photos only
H.C.F of 12 and 18 is 6
Rows of 6.
2 rows of black equal 12.
3 rows of white equals 18.
Question 6.
The teacher writes \(\frac{10}{12}\) on the board. He asks students to write the fraction in simplest form. Who writes the correct answer?
Options:
a. JoAnn writes \(\frac{10}{12}\)
b. Karen writes \(\frac{5}{12}\)
c. Lynn writes \(\frac{6}{5}\)
d. Mark writes \(\frac{5}{6}\)
Answer:
d. Mark writes \(\frac{5}{6}\)
Explanation:
As per the given data,
The teacher writes 10/12 on the board
He asks students to write the fraction in simplest form
For the simplest form of 10/12, divide the 10/12 with 2
(10÷2)/(12÷2) = 5/6
5/6 is the simplest form of 10/12
So, Mark writes the correct answer
Page No. 373
Question 1.
Locate and label points on the number line to help you write \(\frac{3}{10}, \frac{11}{12}, \text { and } \frac{5}{8}\) in order from least to greatest.

Type below:
___________
Answer:
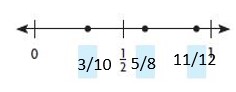
Explanation:
3/10, 11/12, 5/8
3/10 is closer to 0
11/12 is closer to 1
5/8 is closer to 1/2
So, 3/10 < 5/8 < 11/12
Write the fraction with the greatest value.
Question 2.
\(\frac{7}{10}, \frac{1}{5}, \frac{9}{10}\)
\(\frac{□}{□}\)
Answer:
\(\frac{9}{10}\)
Explanation:
7/10, 1/5, and 9/10
7/10 is closer to 1/2
1/5 is closer to 0
9/10 is closer to 1
So, 9/10 > 7/10 > 1/5
Greatest value is 9/10
Question 3.
\(\frac{5}{6}, \frac{7}{12}, \frac{7}{10}\)
\(\frac{□}{□}\)
Answer:
\(\frac{5}{6}\)
Explanation:
7/12 is less than 1/2
7/10 and 5/6 are greater than 1/2
Compare 5/6 and 7/12
Multiply the numerator and denominator of 5/6 with 2
(5×2)/(6×2) = 10/12 > 7/12
So, 5/6 > 7/12
Compare 5/6 and 7/10
Multiply the 5/6 with 10
(5×10)/(6×10) = 50/60
Multiply the 7/10 with 6
(7×6)/(10×6) = 42/60
So, 5/6> 7/10
So, 7/12 <7/10<5/6
Question 4.
\(\frac{2}{8}, \frac{1}{8}, \frac{2}{4}, \frac{2}{6}\)
\(\frac{□}{□}\)
Answer:
\(\frac{2}{4}\)
Explanation:
2/8, 1/8, 2/4, 2/6
Common denominator of 4,6,8 = 24
(2×3)/(8×3), (1×3)/(8×3), (2×6)/(4×6), (2×4)/(6×4)
6/24, 3/24, 12/24, 8/24
Compare the numerator values
12/24 > 8/24 > 6/24 > 3/24
So, 2/4 > 2/6 > 2/8 >1/8
Write the fractions in order from least to greatest.
Question 5.
\(\frac{1}{4}, \frac{3}{6}, \frac{1}{8}\)
\(\frac{□}{□}\)
Type below:
________
Answer:
\(\frac{1}{8}, \frac{3}{6}, \frac{1}{4}\)
Explanation:
1/4, 3/6, 1/8
1/ 4 is closer to 1/2
3/6 is equal to 1/2
1/8 is closer to 0
So, 1/8 < 3/6 < 1/4
Question 6.
\(\frac{3}{5}, \frac{2}{3}, \frac{3}{10}, \frac{4}{5}\)
\(\frac{□}{□}\)
Type below:
________
Answer:
\(\frac{4}{5}, \frac{3}{10}, \frac{3}{5}, \frac{2}{3}\)
Explanation:
3/5, 2/3, 3/10, 4/5
3/5 is closer to 1/2
2/3 is greater than 1/2
3/10 is less than 1/2
4/5 is closer to 0
So, 4/5 < 3/10 < 3/5 < 2/3
Question 7.
\(\frac{3}{4}, \frac{7}{12}, \frac{5}{12}\)
\(\frac{□}{□}\)
Type below:
________
Answer:
\(\frac{5}{12}, \frac{7}{12}, \frac{3}{4}\)
Explanation:
3/4, 7/12, 5/12
3/ 4 is closer to 1
7/12 is greater than 1/2
5/ 12 is closer to 1/2
So, 5/12 < 7/12 < 3/4
Write the fractions in order from least to greatest.
Question 8.
\(\frac{2}{5}, \frac{1}{3}, \frac{5}{6}\)
\(\frac{□}{□}\)
Type below:
________
Answer:
\(\frac{1}{3}, \frac{2}{5}, \frac{5}{6}\)
Explanation:
2/5, 1/3, 5/6
2/5 is closer to 1/2
1/3 is closer to 0
5/6 is closer to 1
So, 1/3 < 2/5 < 5/6
Question 9.
\(\frac{4}{8}, \frac{5}{12}, \frac{1}{6}\)
\(\frac{□}{□}\)
Type below:
________
Answer:
\(\frac{1}{6}, \frac{5}{12}, \frac{4}{8}\)
Explanation:
4/8, 5/12, 1/6
4/8 is equal to1/2
5/12 is closer to 1/2
1/6 is closer to 0
So, 1/6 < 5/12 < 4/ 8
Question 10.
\(\frac{7}{100}, \frac{9}{10}, \frac{4}{5}\)
\(\frac{□}{□}\)
Type below:
________
Answer:
\(\frac{7}{100}, \frac{4}{5}, \frac{9}{10}\)
Explanation:
7/100, 9/10, 4/5
7/100 is closer to 0
9/10 is closer to 1
4/5 is greater than 1/2
So, 7/100 < 4/5 < 9/10
Reason Quantitatively Algebra Write a numerator that makes the statement true.
Question 11.
\(\frac{1}{2}<\frac { □ }{ 10 } <\frac{4}{5}\)
□ = _____
Answer:
6 or 7
Explanation:
1/2 < x/10 < 4/5
Common denominator is 10
(1×5)/(2×5) < x/10 < (4×2)/(5×2)
5/10 < x/10 < 8/10
Then, x = 6 or 7
Question 12.
\(\frac{1}{4}<\frac{5}{12}<\frac { □ }{ 6 } \)
□ = _____
Answer:
6
Explanation:
1/4 < 5/12 < x/6
Common denominator is 24
(1×6)/(4×6) < (5×2)/(12×2) < 4x/(6×4)
6/24 < 10/24 < 4x/24
If x = 6, then 4x = 24
So, 6/24 < 10/24 < 24/24
Question 13.
\(\frac { □ }{ 8 } <\frac{3}{4}<\frac{7}{8}\)
□ = _____
Answer:
1,2,3,4,5
Explanation:
x/8 < 3/4 < 7/8
Common denominator is 8
x/8 < (3×2)/(4×2) < 7/8
x/8 < 6/8 < 7/8
so x = 1,2,3,4,5
Page No. 374
Question 14.
Nancy, Lionel, and Mavis ran in a 5-kilometer race. The table shows their finish times. In what order did Nancy, Lionel, and Mavis finish the race?

a. What do you need to find?
Answer:
In which Nancy, Lionel, and Mavis finished the race?
Question 14.
b. What information do you need to solve the problem?
Type below:
_________
Answer:
the amount of time it took each runner to finish the race
Question 14.
c. What information is not necessary?
Type below:
_________
Answer:
the distance of the race
Question 14.
d. How will you solve the problem?
Type below:
_________
Answer:
By using the running race time of Nancy, Lionel, and Mavis
Question 14.
e. Show the steps to solve the problem.
Type below:
_________
Answer:
Common denominator of 2/3, 7/12, 3/4 is 12
(2×4)/(3×4), (7/12), (3×3)/(4×3)
8/12, 7/12, 9/12
7/12 < 8/12 < 9/12
7/12 < 2/3 < 3/4
Lionel < Nancy < Mavis
Question 14.
f. Complete the sentences.
The runner who finished first is _______.
The runner who finished second is _______.
The runner who finished third is _______.
The first: _______
The second: _______
The third: _______
Answer:
Lionel finished the race first
Nancy finished the race second
Mavis finished the race third
Lionel
Nancy
Mavis
Common Core – Compare and Order Fractions – Page No. 375
Write the fractions in order from least to greatest.
Question 1.
\(\frac{5}{8}, \frac{2}{12}, \frac{8}{10}\)
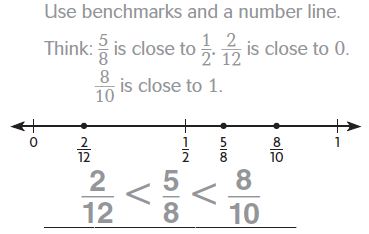
Answer:
\(\frac{2}{12}, \frac{5}{8}, \frac{8}{10}\)
Explanation:

Question 2.
\(\frac{1}{5}, \frac{2}{3}, \frac{5}{8}\)
Type below:
_________
Answer:
\(\frac{1}{5}, \frac{5}{8}, \frac{2}{3}\)
Explanation:
1/5, 2/3, 5/8
1/5 is closer to 0
2/3 is greater than 1/2
5/8 greater than 1/2
1/5 < 5/8 < 2/3
Question 3.
\(\frac{1}{2}, \frac{2}{5}, \frac{6}{10}\)
Type below:
_________
Answer:
\(\frac{2}{5}, \frac{1}{2}, \frac{6}{10}\)
Explanation:
1/2, 2/5, 6/10
1/2 is equal to 1/2
2/5 is less than 1/2
6/10 is greater than 1/2
Question 4.
\(\frac{4}{6}, \frac{7}{12}, \frac{5}{10}\)
Type below:
_________
Answer:
\(\frac{5}{10}\) < \(\frac{7}{12}\) < \(\frac{4}{6}\)
Explanation:
4/6, 7/12, 5/10
4/6 is closer to 1
7/12 is greater than 1/2
5/10 is equal to 1/2
Question 5.
\(\frac{1}{4}, \frac{3}{6}, \frac{1}{8}\)
Type below:
_________
Answer:
\(\frac{1}{8}\) < \(\frac{1}{4}\) < \(\frac{3}{6}\)
Explanation:
1/4, 3/6, 1/8
1/4 is less than 1/2
3/6 is equal to 1/2
1/8 is closer to 0
Question 6.
\(\frac{1}{8}, \frac{3}{6}, \frac{7}{12}\)
Type below:
_________
Answer:
\(\frac{1}{8}\) < \(\frac{7}{12}\) < \(\frac{3}{6}\)
Explanation:
1/8, 3/6, 7/12
1/8 is closer to 0
3/6 is equal to 1/2
7/12 is greater than 1/2
Question 7.
\(\frac{8}{100}, \frac{3}{5}, \frac{7}{10}\)
Type below:
_________
Answer:
\(\frac{8}{100}\) < \(\frac{3}{5}\) < \(\frac{7}{10}\)
Explanation:
8/100, 3/5, 7/10
8/100 is closer to 0
3/5 is greater than 1/2
7/10 is closer to 1
Question 8.
\(\frac{3}{4}, \frac{7}{8}, \frac{1}{5}\)
Type below:
_________
Answer:
\(\frac{1}{5}\) < \(\frac{3}{4}\) < \(\frac{7}{8}\)
Explanation:
3/4, 7/8, 1/5
3/4 is greater than 1/2
7/8 is closer to 1
1/5 is closer to 0
Question 9.
Amy’s math notebook weighs \(\frac{1}{2}\) pound, her science notebook weighs \(\frac{7}{8}\) pound, and her history notebook weighs \(\frac{3}{4}\) pound. What are the weights in order from lightest to heaviest?
Type below:
_________
Answer:
\(\frac{1}{2}\) pound, \(\frac{3}{4}\) pound, \(\frac{7}{8}\) pound
Explanation:
From the given data,
Amy’s math notebook weighs 1/2 pound
Science notebook weighs 7/8 pound
History notebook weighs 3/4 pound
7/8 is closer to 1
3/4 is greater than 1/2
1/2 < 3/4 < 7/8
So, Amy’s math notebook weight < history notebook weight < science notebook
Question 10.
Carl has three picture frames. The thicknesses of the frames are \(\frac{4}{5}\) inch, \(\frac{3}{12}\) inch, and \(\frac{5}{6}\) inch. What are the thicknesses in order from least to greatest?
Type below:
_________
Answer:
\(\frac{3}{12}\) inch, \(\frac{4}{5}\) inch, \(\frac{5}{6}\) inch
Explanation:
As per the given data,
Carl has three picture frames
The thickness of the frames are 4/5 inch, 3/12 inch, 5/6 inch
4/5 is greater than 1/2
3/12 is less than 1/2
5/6 is closer to 1
3/12 < 4/5 < 5/6
Common Core – Compare and Order Fractions – Page No. 376
Question 1.
Juan’s three math quizzes this week took him \(\frac{1}{3}\) hour, \(\frac{4}{6}\) hour, and \(\frac{1}{5}\) hour to complete. Which list shows the lengths of time in order from least to greatest?
Options:
a. \(\frac{1}{3}\) hour, \(\frac{4}{6}\) hour, \(\frac{1}{5}\) hour
b. \(\frac{1}{5}\) hour, \(\frac{1}{3}\) hour, \(\frac{4}{6}\) hour
c. \(\frac{1}{3}\) hour, \(\frac{1}{5}\) hour, \(\frac{4}{6}\) hour
d. \(\frac{4}{6}\) hour, \(\frac{1}{3}\) hour, \(\frac{1}{5}\) hour
Answer:
b. \(\frac{1}{5}\) hour, \(\frac{1}{3}\) hour, \(\frac{4}{6}\) hour
Explanation:
From the given information
Juan’s three math quizzes this week took him 1/3 hour, 4/6 hour, and 1/5 hour
Compare 1/3 and 1/2
1/3 is less than 1/2
4/6 is greater than 1/2
1/5 is closer to 0
1/5 < 1/3 < 4/6
So, Juan’s math quizzes times from least to greatest is 1/5, 1/3, 4/6
Question 2.
On three days last week, Maria ran \(\frac{3}{4}\) mile, \(\frac{7}{8}\) mile, and \(\frac{3}{5}\) mile. What are the distances in order from least to greatest?
Options:
a. \(\frac{3}{4}\) mile, \(\frac{7}{8}\) mile, \(\frac{3}{5}\) mile
b. \(\frac{3}{5}\) mile, \(\frac{3}{4}\) mile, \(\frac{7}{8}\) mile
c. \(\frac{7}{8}\) mile, \(\frac{3}{4}\) mile, \(\frac{3}{5}\) mile
d. \(\frac{7}{8}\) mile, \(\frac{3}{5}\) mile, \(\frac{3}{4}\) mile
Answer:
b. \(\frac{3}{5}\) mile, \(\frac{3}{4}\) mile, \(\frac{7}{8}\) mile
Explanation:
As per the information
On three days last week, Maria ran 3/4 mile, 7/8 mile, and 3/5 mile
3/4 is greater than 1/2
7/8 is closer to 1
3/5 is greater than 1/2
Compare 3/5 and 3/4
3/4 is greater than 3/5
So, 3/5 < 3/4 < 7/8
Distance from least to greatest is 3/5, 3/4 , 7/8
Question 3.
Santiago collects 435 cents in nickels. How many nickels does he collect?
Options:
a. 58
b. 78
c. 85
d. 87
Answer:
d. 87
Explanation:
As per the given data,
Santiago collects 435 cents in nickels
1 nickel worth is 5 cents
Then, nickels per 435 cents = 435/5 = 87
So, Santiago collects 87 nickels
Question 4.
Lisa has three classes that each last 50 minutes. What is the total number of minutes the three classes last?
Options:
a. 15 minutes
b. 150 minutes
c. 153 minutes
d. 156 minutes
Answer:
b. 150 minutes
Explanation:
From the given data,
Lisa has three classes that each last 50 minutes
The total number of minutes the three classes last = 3×50 =150 minutes
Question 5.
Some students were asked to write a composite number. Which student did NOT write a composite number?
Options:
a. Alicia wrote 2.
b. Bob wrote 9.
c. Arianna wrote 15.
d. Daniel wrote 21.
Answer:
a. Alicia wrote 2.
Explanation:
As per the information
Some students were asked to write a composite number
a. Alicia wrote 2
Factors of 2 is 1 and 2
b. Bob wrote 9
Factors of 9 is 1, 3, 9
c. Arianna wrote 15
Factors of 15 is 1, 3, 5, 15
d. Daniel wrote 21
Factors of 21 is 1,3,7,21
So, Alicia did not write a composite number
Question 6.
Mrs. Carmel serves \(\frac{6}{8}\) of a loaf of bread with dinner. Which fraction is equivalent to \(\frac{6}{8}\)?
Options:
a. \(\frac{2}{4}\)
b. \(\frac{9}{16}\)
c. \(\frac{2}{3}\)
d. \(\frac{3}{4}\)
Answer:
d. \(\frac{3}{4}\)
Explanation:
As per the given information
Mrs. Carmel serves 6/8 of a loaf of bread with dinner
To find the equivalent fraction of 6/8, simplify the 6/8 by dividing with the 2
(6÷2)/(8÷2) = ¾
So, the equivalent fraction of 6/8 is 3/4
Page No. 377
Question 1.
For numbers 1a–1d, tell whether the fractions are equivalent by selecting the correct symbol.
a. \(\frac{4}{16}\) _____ \(\frac{1}{4}\)
Answer:
\(\frac{4}{16}\) = \(\frac{1}{4}\)
Explanation:
4/16 and 1/4
Divide the numerator and denominator of 4/16 with 4
(4÷4)/(16÷4) = 1/4
So, 4/16 = 1/4
Question 1.
b. \(\frac{3}{5}\) _____ \(\frac{12}{15}\)
Answer:
\(\frac{3}{5}\) ≠ \(\frac{12}{15}\)
Explanation:
3/5 and 12/15
Multiply the numerator and denominator of 3/5 with 3
(3×3)/(5×3) = 9/15
So, 3/5 ≠ 12/15
Question 1.
c. \(\frac{5}{6}\) _____ \(\frac{25}{30}\)
Answer:
\(\frac{5}{6}\) = \(\frac{25}{30}\)
Explanation:
c. 5/6 and 25/30
Multiply the numerator and denominator of 5/6 with 5
(5×5)/(6×5) = 25/30
So, 5/6 = 25/30
Question 1.
d. \(\frac{6}{10}\) _____ \(\frac{5}{8}\)
Answer:
\(\frac{6}{10}\) ≠ \(\frac{5}{8}\)
Explanation:
6/10 and 5/8
Divide the numerator and denominator of 6/10 with 2
(6÷2)/(10÷2) = 3/5
6/10 ≠5/8
Question 2.
Juan’s mother gave him a recipe for trail mix.
\(\frac{3}{4}\) cup cereal \(\frac{2}{3}\) cup almonds
\(\frac{1}{4}\) cup peanuts \(\frac{1}{2}\) cup raisins
Order the ingredients used in the recipe from least to greatest.
Type below:
_________
Answer:
As per the given data,
Juan’s mother gave him a recipe for trail mix
3/4 cup cereal and 2/3 cup almonds
1/4 cup peanuts and 1/2 cup raisins
3/4 is closer to 1
2/3 is greater than 1/2
1/4 is less than 1/2
1/2 is equal to 1/2
So, 1/4 < 1/2 <2/3 < 3/4
So, Jaun’s mother gave him a recipe for trail mix in order
1/4 cup of peanuts < 1/2 cup of raisins < 2/3 cup almonds < 3/4 cup of cereals
Question 3.
Taylor cuts \(\frac{1}{5}\) sheet of construction paper for an arts and crafts project. Write \(\frac{1}{5}\) as an equivalent fraction with the denominators shown.
Type below:
_________
Answer:
From the given data,
Taylor cuts 1/5 sheet of construction paper for an arts and crafts project
So, the equivalent fractions of 1/5
Multiply the numerator and denominator of 1/5 with 2
(1×2)/(5×2) = 2/10
Multiply the numerator and denominator of 1/5 with 3
(1×3)/(5×3) = 3/15
Multiply the numerator and denominator of 1/5 with 5
(1×5)/(5×5) = 5/25
Multiply the numerator and denominator of 1/5 with 8
(1×8)/(5×8) = 8/40
So, the equivalent fractions of 1/5 are 2/10, 3/15, 5/25, 8/40
Question 4.
A mechanic has sockets with the sizes shown below. Write each fraction in the correct box.
\(\frac{7}{8} in. \frac{3}{16} in. \frac{1}{4} in. \frac{3}{8} in. \frac{4}{8} in. \frac{11}{16} in.\)
Type below:
_________
Answer:

Explanation:
As per the given data,
A mechanic has sockets with the sizes 7/8 inch, 3/16 inch, 1/4 inch, 3/8 inch, 4/8 inch, 11/16 inch
7/8 is greater than 1/2
3/16 is less than 1/2
1/4 is less than 1/2
3/8 is less than 1/2
4/8 is equal to 1/2
11/16 is greater than 1/2
Page No. 378
Question 5.
Darcy bought \(\frac{1}{2}\) pound of cheese and \(\frac{3}{4}\) pound of hamburger for a barbecue. Use the numbers to compare the amounts of cheese and hamburger Darcy bought.
Answer:
Explanation:
From the given data,
Darcy bought 1/2 pound of cheese and 3/4 pound of hamburger for a barbecue
3/4 is greater than 1/2
Question 6.
Brad is practicing the piano. He spends \(\frac{1}{4}\) hour practicing scales and \(\frac{1}{3}\) hour practicing the song for his recital. For numbers 6a–6c, select Yes or No to tell whether each of the following is a true statement.
a. 12 is a common denominator of \(\frac{1}{4}\) and \(\frac{1}{3}\).
i. yes
ii. no
Answer:
i. yes
Explanation:
12 is a common denominator of 1/3 and 1/4
Question 6.
b. The amount of time spent practicing scales can be rewritten as \(\frac{3}{12}\).
i. yes
ii. no
Answer:
i. yes
Explanation:
b. The amount of time spent practicing scales can be rewritten as 3/12
Multiply the numerator and denominator of 1/4 with 3
(1×3)/(4×3) = 3/12
Yes, amount of time spent practicing scales can be rewritten as 3/12
Question 6.
c. The amount of time spent practicing the song for the recital can be rewritten as \(\frac{6}{12}\).
i. yes
ii. no
Answer:
ii. no
Explanation:
c. The amount of time spent practicing the song for the recital can be rewritten as 6/12
The amount of time spent practicing for the song for his recital = 1/3
Multiply the numerator and denominator of 1/3 with 4
(1×4)/(3×4) = 4/12
No, time spent practicing the song for the recital can not be written as 6/12
Question 7.
In the school chorus, \(\frac{4}{24}\) of the students are fourth graders. In simplest form, what fraction of the students in the school chorus are fourth graders?
\(\frac{□}{□}\)
Answer:
\(\frac{1}{6}\)
Explanation:
As per the given information,
In the school chorus,
4/24 of the students are fourth graders
For the simplest form of 4/24
Divide the numerator and denominator of 4/24 with 4
(4÷4)/(24÷4) =1/6
The simplest form of 4/24 is 1/6
Question 8.
Which pairs of fractions are equivalent? Mark all that apply.
a. \(\frac{8}{12} \text { and } \frac{2}{3}\)
b. \(\frac{3}{4} \text { and } \frac{20}{24}\)
c. \(\frac{4}{5} \text { and } \frac{12}{16}\)
d. \(\frac{7}{10} \text { and } \frac{21}{30}\)
Answer:
a. \(\frac{8}{12} \text { and } \frac{2}{3}\)
Explanation:
a. 8/12 and 2/3
Multiply the numerator and denominator of 2/3 with 4
(2×4)/(3×4) = 8/12
So, 8/12 = 2/3
b. 3/4 and 20/24
Multiply the numerator and denominator of 3/4 with 6
(3×6)/(4×6) = 18/24
c. 4/5 and 12/16
4/5 ≠ 12/16
d. 7/10 and 21/30
Multiply the numerator and denominator of 7/10 with 3
(7×3)/(10×3) =21/30
So, 7/10 = 21/30
Question 9.
Sam worked on his science fair project for \(\frac{1}{4}\) hour on Friday and \(\frac{1}{2}\) hour on Saturday. What are four common denominators for the fractions? Explain your reasoning.
Answer:
From the given data,
Sam worked on his science fair project for 1/4 hour on Friday and 1/2 hour on Saturday
4,8,12,16 are all common denominators because they all multiples of 2 and 4
Page No. 379
Question 10.
Morita works in a florist shop and makes flower arrangements. She puts 10 flowers in each vase, and \(\frac{2}{10}\) of the flowers are daisies.
Part A
If Morita makes 4 arrangements, how many daisies does she need? Show how you can check your answer.
_____ daisies
Answer:
8 daisies
Explanation:
If Morita makes 4 arrangements, 4 X 2 = 8.
Question 10.
Part B
Last weekend, Morita used 10 daisies to make flower arrangements. How many flowers other than daisies did she use to make the arrangements? Explain your reasoning.
_____ other flowers
Answer:
40 other flowers
Explanation:
If she used 10 daises, she must have made 5 arrangements. In each vase, she put \(\frac{2}{10}\) of the flowers are daisies. So, remaining flowers for each vase = 10 – 2 = 8. If she made 5 arrangements, 8 X 5 = 40 other flowers.
Question 11.
In Mary’s homeroom, \(\frac{10}{28}\) of the students have a cat, \(\frac{6}{12}\) have a dog, and \(\frac{2}{14}\) have a pet bird. For numbers 11a–11c, select True or False for each statement.
a. In simplest form, \(\frac{5}{14}\) of the students have a cat.
i. True
ii. False
Answer:
i. True
Explanation:
In simplest form 5/14 of the students have a cat
From the above, 10/28 of the students have a cat
Divide the numerator and denominator of 10/28 with 2
(10÷2)/(28÷2) = 5/14
True
Question 11.
b. In simplest form, \(\frac{2}{4}\) of the students have a dog.
i. True
ii. False
Answer:
i. True
Explanation:
In simplest form, 2/4 of the students have a dog
From the above, 6/12 of the students have a dog
Divide the 6/12 with 3
(6 = 2/4
True
Question 11.
c. In simplest form, \(\frac{1}{7}\) of the students have a pet bird.
i. True
ii. False
Answer:
i. True
Explanation:
In the simplest form, 1/7 of the students have a pet bird
From the data, 2/14 of the students have a pet bird
Divide the numerator and denominator of 2/14 with 2
(2÷2)/(14÷2) = 1/7
True
Page No. 380
Question 12.
Regina, Courtney, and Ellen hiked around Bear Pond. Regina hiked \(\frac{7}{10}\) of the distance in an hour. Courtney hiked \(\frac{3}{6}\) of the distance in an hour. Ellen hiked 38 of the distance in an hour. Compare the distances hiked by each person by matching the statements to the correct symbol. Each symbol may be used more
than once or not at all.

Type below:
_________
Answer:

Explanation:
From the given information
Regina, Courtney, and Ellen hiked around Bear Pond
Regina hiked 7/10 of the distance in an hour
Courtney hiked 3/6 of the distance in an hour
Ellen hiked 3 /8 of the distance in an hour
Compare 7/10 and 3/6
The common denominator of 7/10 and 3/6 is 30
(7×3)/(10×3) and (3×5)/(6×5)
21/30 and 15/30
So, 21/30 > 15/30
So, 7/10 > 15/30
Compare 3/8 and 3/6
The common denominator of 3/8 and 3/6 is 24
(3×3)/(8×3) and (3×4)/(6×4)
9/24 and 12/24 = 9/24 < 12/24 = 3/8 < 3/6
Compare 7/10 and 3/8
The common denominator of 7/10 and 3/8 is 40
(7×4)/(10×4) and (3×5)/(8×5)
28/40 >15/40 = 7/10 > 3/8
Question 13.
Ramon is having some friends over after a baseball game. Ramon’s job is to make a vegetable dip. The ingredients for the recipe are given.
Part A
Which ingredient does Ramon use the greater amount of, buttermilk or cream cheese? Explain how you found your answer.
Type below:
_________
Answer:
Ramon use 5/8 cup of buttermilk and 1/2 cup cream cheese
By comparing these two ingredients
The common denominator of 5/8 and 1/2 are 8
(1×4)/(2×4) =4/8
So, 5/8 > 4/8
So, 5/8 cup buttermilk is > ½ cup cream cheese
Question 13.
Part B
Ramon says that he needs the same amount of two different ingredients. Is he correct? Support your answer with information from the problem.
______
Answer:
Ramon says that he needs the same amount of two ingredients
Yes, Ramon uses 3/4 cup parsley and 6/8 cup scallions
Multiply the 3/4 with 2
(3×2)/(4×2) = 6/8
So, Ramon uses the same amount that is 3/4 cup for parsley and scallions
Page No. 381
Question 14.
Sandy is ordering bread rolls for her party. She wants \(\frac{3}{5}\) of the rolls to be whole wheat. What other fractions can represent the part of the rolls that will be whole wheat? Shade the models to show your work.
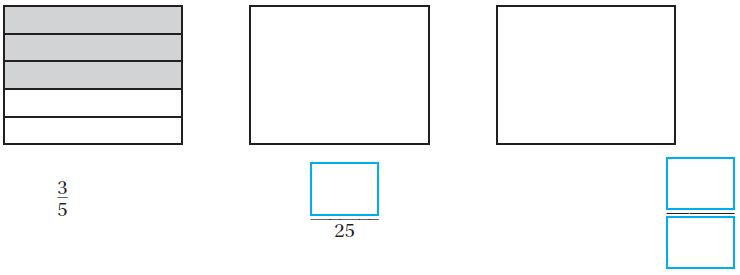
Type below:
_________
Answer:
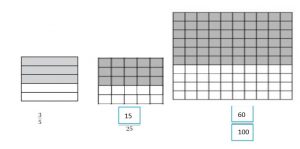
Explanation:
As per the information,
Sandy is ordering bread rolls for her party
She wants 3/5 of the rolls to be whole wheat
For an equivalent fraction of 3/5, multiply with 5
(3×5)/(5×5) = 15/25
Again multiply the 15/25 with 4
(15×4)/(25×4) = 60/100
Question 15.
Angel has \(\frac{4}{8}\) yard of ribbon and Lynn has \(\frac{3}{4}\) yard of ribbon. Do Angel and Lynn have the same amount of ribbon? Shade the model to show how you found your answer. Explain your reasoning.
Type below:
_________
Answer:
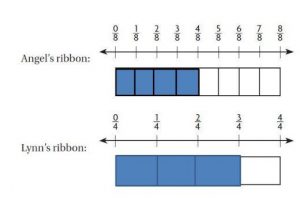
Angel and Lynn didn’t have the same amount of ribbon. 4/8 is a greater fraction compared to 3/4. So, Angel’s ribbon is long compared to Lynn’s ribbon.
Question 16.
Ella used \(\frac{1}{4}\) yard of red ribbon. Fill in each box with a number from the list to show equivalent fractions for \(\frac{1}{4}\). Not all numbers will be used.
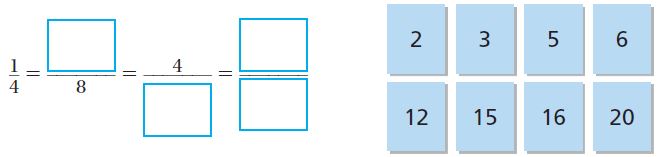
Type below:
_________
Answer:
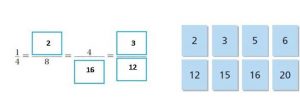
Explanation:
1/4 = 2/8 = 4/16 = 3/12
Page No. 382
Question 17.
Frank has two same-size rectangles divided into the same number of equal parts. One rectangle has \(\frac{3}{4}\) of the parts shaded, and the other has \(\frac{1}{3}\) of the parts shaded.
Part A
Into how many parts could each rectangle be divided? Show your work by drawing the parts of each rectangle.
_____ parts
Answer:
12 parts
Question 17.
Part B
Is there more than one possible answer to Part A? If so, did you find the least number of parts into which both rectangles could be divided? Explain your reasoning.
Type below:
_________
Answer:
Yes, as long it is a multiple of 12.
And yes,12 is the least in order to have 1 rectangle have 3/4 shaded and the other 1/3 shaded.
Question 18.
Suki rode her bike \(\frac{4}{5}\) mile. Claire rode her bike \(\frac{1}{3}\) mile. They want to compare how far they each rode their bikes using the benchmark \(\frac{1}{2}\). For numbers 18a–18c, select the correct answers to describe how to solve the problem.
a. Compare Suki’s distance to the benchmark:
\(\frac{4}{5}\) _____ \(\frac{1}{2}\)
Answer:
\(\frac{4}{5}\) ≠ \(\frac{1}{2}\)
Explanation:
The fraction \(\frac{4}{5}\) is not equal to \(\frac{1}{2}\).
Question 18.
b. Compare Claire’s distance to the benchmark:
\(\frac{1}{3}\) _____ \(\frac{1}{2}\)
Answer:
\(\frac{1}{3}\) ≠ \(\frac{1}{2}\)
Explanation:
The fraction \(\frac{1}{3}\) is not equal to \(\frac{1}{2}\)
Question 18.
c. Suki rode her bike _____ Claire.
Answer:
Suki rode her bike faster than Claire.
Page No. 387
Use the model to write an equation.
Question 1.

Type below:
_________
Answer:
\(\frac{3}{5}\) + \(\frac{1}{5}\) = \(\frac{4}{5}\)
Question 2.
Type below:
_________
Answer:
\(\frac{2}{3}\) – \(\frac{1}{3}\) = \(\frac{1}{3}\)
Question 3.

Type below:
_________
Answer:
\(\frac{1}{4}\) + \(\frac{1}{4}\) = \(\frac{2}{4}\)
Question 4.
Type below:
_________
Answer:
1 – \(\frac{5}{8}\) = \(\frac{8}{8}\) – \(\frac{5}{8}\) = \(\frac{3}{8}\)
Use the model to solve the equation.
Question 5.
\(\frac{3}{4}-\frac{1}{4}\) = \(\frac{□}{□}\)
Answer:
\(\frac{2}{4}\)
Question 6.
\(\frac{5}{6}+\frac{1}{6}\) = \(\frac{□}{□}\)
Answer:
\(\frac{6}{6}\) = 1
Question 7.
Reason Abstractly Sean has \(\frac{1}{5}\) of a cupcake and \(\frac{1}{5}\) of a large cake.
a. Are the wholes the same? Explain.
______
Answer:
Yes; From the given information, the fraction of the cupcake and large cake are the same.
Explanation:
Question 7.
Does the sum \(\frac{1}{5}+\frac{1}{5}=\frac{2}{5}\) make sense in this situation? Explain.
______
Answer:
Yes; it makes sense. From the given data, 1 part is out of 5 parts. So, adding two fractions (1 part is out of 5 parts), the complete fraction becomes 2/5.
Question 8.
Carrie’s dance class learned \(\frac{1}{5}\) of a new dance on Monday, and \(\frac{2}{5}\) of the dance on Tuesday. What fraction of the dance is left for the class to learn on Wednesday?
\(\frac{□}{□}\)
Answer:
\(\frac{3}{5}\)
Explanation:
The fraction of left for the class to learn on Wednesday is \(\frac{3}{5}\).
Page No. 388
Question 9.
Samantha and Kim used different models to help find \(\frac{1}{3}+\frac{1}{6}\). Whose model makes sense? Whose model is nonsense? Explain your reasoning below each model.
Answer:
Both Samantha and Kim’s statements make sense. Because both models have an equal number of fractions for each diagram.
Question 10.
Draw a model you could use to add \(\frac{1}{4}+\frac{1}{2}\).
Type below:
___________
Answer:
Question 11.
Cindy has two jars of paint. One jar is \(\frac{3}{8}\) full. The other jar is \(\frac{2}{8}\) full. Use the fractions to write an equation that shows the amount of paint Cindy has.
Type below:
___________
Answer:
\(\frac{3}{8}+\frac{2}{8}\) = \(\frac{5}{8}\)
Conclusion:
By downloading the Go Math Grade 4 Answer Key Chapter 6 Fraction Equivalence and Comparison PDF, students of grade 4 will aid you to understand different topics in Chapter 6 easily. Prepare well with the help of Go Math Grade 4 Answer Key PDFand solve each and every question properly. For more help utilize this Go Math Grade 4 Solution Key Chapter 6 Fraction Equivalence and Comparison PDF and gain what you require.