Eureka Math Precalculus Module 1 Lesson 22 Answer Key

Engage NY Eureka Math Precalculus Module 1 Lesson 22 Answer Key

Eureka Math Precalculus Module 1 Lesson 22 Exercise Answer Key

Opening Exercise
Let D\(\left(\begin{array}{l}
x \\
y
\end{array}\right)\)=\(\left(\begin{array}{ll}
3 & 0 \\
0 & 3
\end{array}\right)\left(\begin{array}{l}
x \\
y
\end{array}\right)\).

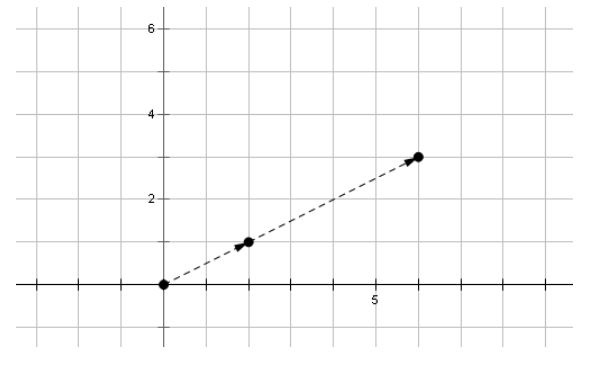
a. Plot the point \(\left(\begin{array}{l}
2 \\
1
\end{array}\right)\).

b. Find D\(\left(\begin{array}{l}
2 \\
1
\end{array}\right)\) and plot it.
Answer:

c. Describe the geometric effect of performing the transformation \(\left(\begin{array}{l}
x \\
y
\end{array}\right) \rightarrow D\left(\begin{array}{l}
x \\
y
\end{array}\right)\).
Answer:
Each point in the plane gets dilated by a factor of 3. In other words, a point P gets moved to a new location that is on the line through P and the origin, but its distance from the origin increases by a factor of 3.

Exercises 1–2

Exercise 1.
Let f(t)=\(\left(\begin{array}{ll}
t & 0 \\
0 & t
\end{array}\right)\left(\begin{array}{l}
2 \\
4
\end{array}\right)\), where t represents time, measured in seconds. P=f(t) represents the position of a moving object at time t. If the object starts at the origin, how long would it take to reach (12,24)?
Answer:
\(\left(\begin{array}{ll}
t & 0 \\
0 & t
\end{array}\right)\left(\begin{array}{l}
2 \\
4
\end{array}\right)\),t×2+0×4=12,2t=12,t=6, or
\(\left(\begin{array}{ll}
t & 0 \\
0 & t
\end{array}\right)\left(\begin{array}{l}
2 \\
4
\end{array}\right)\)=\(\left(\begin{array}{l}
12 \\
24
\end{array}\right)\),0×2+t×4=24,4t=24,t=6

Exercise 2.
Let g(t)=\(\).
a. Find the value of k that moves an object from the origin to (12,24) in just 2 seconds.
t=2,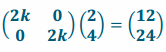,2k×2+0×4=12,k=\(\frac{12}{4}\)=3, or
t=2,,0×2k+2k×4=24,k=\(\frac{24}{8}\)=3

b. Find the value of k that moves an object from the origin to (12,24) in 30 seconds.
Answer:
t=30,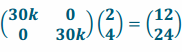,30k×2+0×4=12,k=\(\frac{12}{60}\)=\(\frac{1}{5}\), or
t=30, ,0×30k+30k×4=24,k=\(\frac{24}{120}\)=\(\frac{1}{5}\)

Exercise 3.
Let f(t)=, where t represents time, measured in seconds, and f(t) represents the position of a moving object at time t.
a. Find the position of the object at t=0,t=1, and t=2.

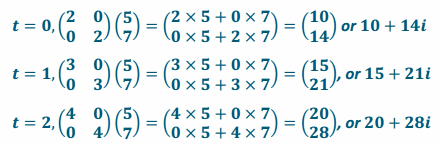
b. Write f(t) in the form \(\left(\begin{array}{l}
x(t) \\
y(t)
\end{array}\right)\).
Answer:

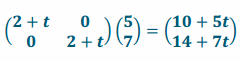
Exercise 4.
Write the transformation g(t)=\(\left(\begin{array}{c}
15+5 t \\
-6-2 t
\end{array}\right)\) as a matrix transformation.
Answer:
Answers vary based on factoring of factors. However, they start at different points that are all from the line, and they all end up having the same result: g(t)=\(\left(\begin{array}{c}
15+5 t \\
-6-2 t
\end{array}\right)\).

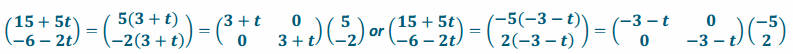
Exercise 5.
An object is moving in a straight line from (18,12) to the origin over a 6-second period of time. Find a function f(t) that gives the position of the object after t seconds. Write your answer in the form f(t)=\(\left(\begin{array}{l}
x(t) \\
y(t)
\end{array}\right)\), and then express f(t) as a matrix transformation.
Answer:
For the x-coordinates, we have 18-6k=0, k=3. The x-coordinate of the point is decreasing at 3 units per second. Thus, x(t)=18-3t.
For the y-coordinates, we have 12-6m=0, m=2. The y-coordinate of the point is decreasing at 2 units per second. Thus, x(t)=12-2t.

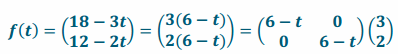
Exercise 6.
Write a rule for the function that shifts every point in the plane 6 units to the left.
Answer:

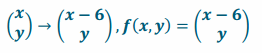
Exercise 7.
Write a rule for the function that shifts every point in the plane 9 units upward.
Answer:

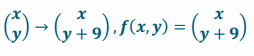
Exercise 8.
Write a rule for the function that shifts every point in the plane 10 units down and 4 units to the right.
Answer:

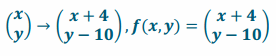
Exercise 9.
Consider the rule . Describe the effect this transformation has on the plane.
Answer:
Every point in the plane is shifted 7 units to the left and 2 units upward.

Eureka Math Precalculus Module 1 Lesson 22 Problem Set Answer Key

Question 1.
Let . Find and plot the following.
a. Plot the point \(\left(\begin{array}{c}
-1 \\
2
\end{array}\right)\), and find \(\left(\begin{array}{c}
-1 \\
2
\end{array}\right)\) and plot it.
Answer:

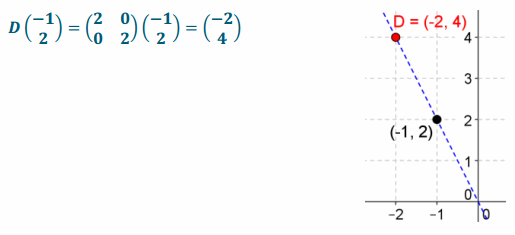
b. Plot the point \(\left(\begin{array}{l}
3 \\
4
\end{array}\right)\), and find D\(\left(\begin{array}{l}
3 \\
4
\end{array}\right)\) and plot it.
Answer:

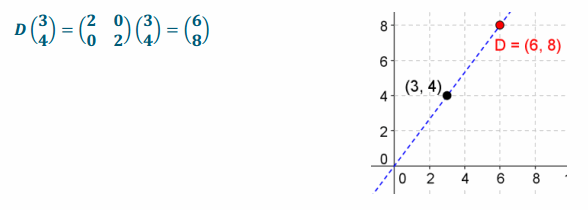
c. Plot the point \(\left(\begin{array}{l}
5 \\
2
\end{array}\right)\), and find D\(\left(\begin{array}{l}
5 \\
2
\end{array}\right)\) and plot it.
Answer:

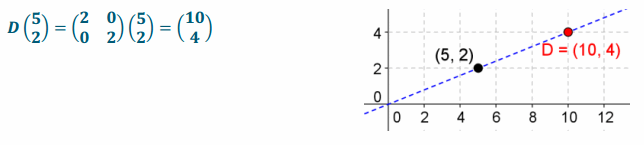
Question 2.
Let f(t)=. Find f(0),f(1),f(2),f(3), and plot them on the same graph.
Answer:

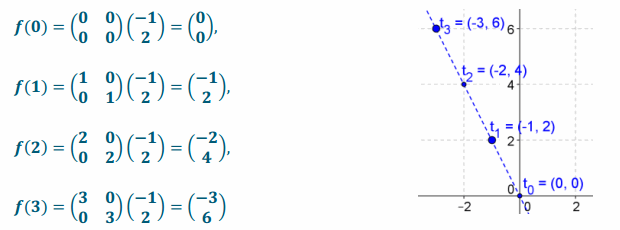
Question 3.
Let f(t)= represent the location of an object at time t that is measured in seconds.
a. How long does it take the object to travel from the origin to the point \(\left(\begin{array}{c}
12 \\
8
\end{array}\right)\)?
Answer:
3t+0×2=12,t=4 or 0×3+2t=8,t=4

b. Find the speed of the object in the horizontal direction and in the vertical direction.
Answer:
f(t)=\(\left(\begin{array}{l}
3 t \\
2 t
\end{array}\right)\) The object is moving 2 units upward per second and 3 units to the right per second.

Question 4.
Let f(t)=,h(t)=. Which one will reach the point \(\left(\begin{array}{c}
12 \\
8
\end{array}\right)\) first? The time t is measured in seconds.
Answer:
For f(t),0.2t×3+0×2=12,t=\(\frac{12}{0.6}\)=20 seconds.
For h(t),2t×3+0×2=12,t=\(\frac{12}{6}\)=2 seconds; therefore, h(t) will reach the point \(\left(\begin{array}{c}
12 \\
8
\end{array}\right)\) first.

Question 5.
Let f(t)=. Find the value of k that moves the object from the origin to \(\left(\begin{array}{l}
-45 \\
-30
\end{array}\right)\) in 5 seconds.
Answer:

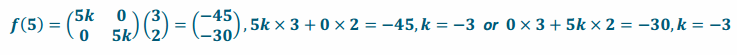
Question 6.
Write f(t) in the form \(\left(\begin{array}{l}
x(t) \\
y(t)
\end{array}\right)\) if

a. f(t)=\(\left(\begin{array}{ll}
\boldsymbol{t} & \mathbf{0} \\
\mathbf{0} & \boldsymbol{t}
\end{array}\right)\left(\begin{array}{l}
2 \\
\mathbf{5}
\end{array}\right)\)
f(t)=\(\left(\begin{array}{c}
2 t \\
5 t
\end{array}\right)\)

b. f(t)=\(\left(\begin{array}{cc}
2 t+1 & 0 \\
0 & 2 t+1
\end{array}\right)\left(\begin{array}{l}
3 \\
2
\end{array}\right)\)
Answer:
f(t)=\(\left(\begin{array}{l}
6 t+3 \\
4 t+2
\end{array}\right)\)

c. f(t)=\(\left(\begin{array}{cc}
\frac{t}{2}-3 & 0 \\
0 & \frac{t}{2}-3
\end{array}\right)\left(\begin{array}{c}
4 \\
-6
\end{array}\right)\)
Answer:
f(t)=\(\left(\begin{array}{l}
2 t-12 \\
3 t-18
\end{array}\right)\)

Question 7.
Let f(t)=\(\left(\begin{array}{ll}
\boldsymbol{t} & \mathbf{0} \\
\mathbf{0} & \boldsymbol{t}
\end{array}\right)\left(\begin{array}{l}
2 \\
5
\end{array}\right)\) represent the location of an object after t seconds.
a. If the object starts at \(\left(\begin{array}{c}
6 \\
15
\end{array}\right)\), how long would it take to reach \(\left(\begin{array}{l}
34 \\
85
\end{array}\right)\)?
Answer:
f(t)=\(\left(\begin{array}{l}
2 t \\
5 t
\end{array}\right)\); it starts at \(\left(\begin{array}{c}
6 \\
15
\end{array}\right)\); therefore, f(t)=\(\left(\begin{array}{c}
2 t+6 \\
5 t+15
\end{array}\right)\).
2t+6=34,t=14 or 5t+15=85,t=14

b. Write the new function f(t) that gives the position of the object after t seconds.
Answer:

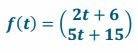
c. Write f(t) as a matrix transformation.
Answer:

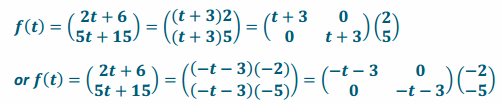
The answers vary; it depends on how the factoring is applied.

Question 8.
Write the following functions as a matrix transformation.
a.
Answer:

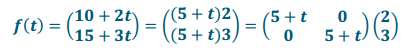
b.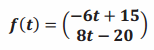
Answer:

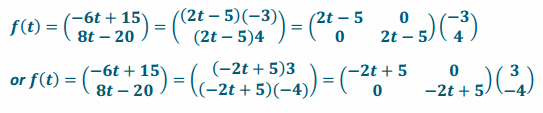
Question 9.
Write a function rule that represents the change in position of the point \(\left(\begin{array}{l}
x \\
y
\end{array}\right)\) for the following.

a. 5 units to the right and 3 units downward
Answer:

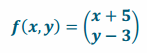
b. 2 units downward and 3 units to the left
Answer:

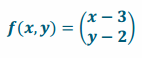
c. 3 units upward, 5 units to the left, and then it dilates by 2.
Answer:

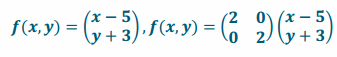
d. 3 units upward, 5 units to the left, and then it rotates by π/2 counterclockwise.
Answer:

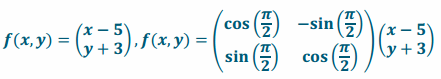
Question 10.
Annie is designing a video game and wants her main character to be able to move from any given point \(\left(\begin{array}{l}
x \\
y
\end{array}\right)\) in the following ways: right 1 unit, jump up 1 unit, and both jump up and move right 1 unit each.
a. What function rules can she use to represent each time the character moves?
Answer:

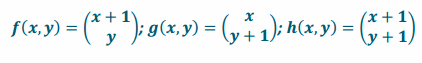
b. Annie is also developing a ski slope stage for her game and wants to model her character’s position using matrix transformations. Annie wants the player to start at \(\left(\begin{array}{c}
-20 \\
10
\end{array}\right)\) and eventually pass through the origin moving 5 units per second down. How fast does the player need to move to the right in order to pass through the origin? What matrix transformation can Annie use to describe the movement of the character? If the far right of the screen is at x=20, how long until the player moves off the screen traveling this path?
Answer:
If the player is moving 5 units per second down, then she will reach y=0 in t=2 seconds. Thus, the player needs to move 10 units per second to the right.

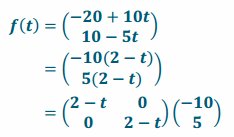
The player will leave the screen in 4 seconds.

Question 11.
Remy thinks that he has developed matrix transformations to model the movements of Annie’s characters in Problem 10 from any given point \(\left(\begin{array}{l}
x \\
y
\end{array}\right)\), and he has tested them on the point \(\left(\begin{array}{l}
1 \\
1
\end{array}\right)\). This is the work Remy did on the transformations:

Do these matrix transformations accomplish the movements that Annie wants to program into the game? Explain why or why not.
Answer:
These do not accomplish the movements. If we apply the transformations to any other point in the plane, then they will not produce the same results of moving one unit to the right, one unit up, and one unit up and right.
As a counterexample, any of the three matrix transformations applied to the origin do nothing.

Question 12.
Nolan has been working on how to know when the path of a point can be described with matrix transformations and how to know when it requires translations and cannot be described with matrix transformations. So far, he has been focusing on the following two functions, which both pass through the point (2,5):

a. If we simplify these functions algebraically, how does the rule for f differ from the rule for g? What does this say about which function can be expressed with matrix transformations?
Answer:
Thus, there is a common factor in both the x- and y-coordinate. Because there is a common factor, we can pull the factor out as a scalar and rewrite the scalar as a matrix multiplication. g(t) does not have a common factor (other than 1) between the x- and y-coordinate.

b. Nolan has noticed that functions that can be expressed with matrix transformations always pass through the origin; does either f or g pass through the origin, and does this support or contradict Nolan’s reasoning?
Answer:
At t=-3, the graph of f passes through the origin. On the other hand, the graph of g crosses the x-axis at t=-2 and the y-axis at t=-5, so it does not pass through the origin. This seems to support Nolan’s reasoning. This agrees with our response to part (a), since the common factor has the same zero and causes the function to cross the origin.

c. Summarize the results of parts (a) and (b) to describe how we can tell from the equation for a function or from the graph of a function that it can be expressed with matrix transformations.
Answer:
If a function has a common factor involving t that can be pulled out of both the x- and y-coordinates, then the function can be represented as a matrix transformation. If the graph of the function passes through the origin, then the function can be represented as a matrix transformation.

Eureka Math Precalculus Module 1 Lesson 22 Exit Ticket Answer Key

Question 1.
Consider the function h(t)=\(\left(\begin{array}{c}
t+5 \\
t-3
\end{array}\right)\). Draw the path that the point P=h(t) traces out as t varies within the interval 0≤t≤4.
Answer:

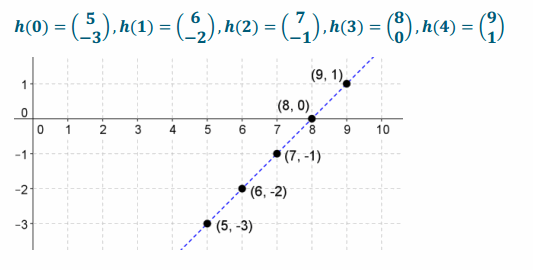
Question 2.
The position of an object is given by the function f(t)=, where t is measured in seconds.
a. Write f(t) in the form .
Answer:

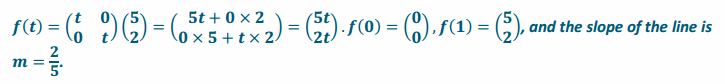
b. Find how fast the object is moving in the horizontal direction and in the vertical direction.
Answer:
The object is moving 2 units upward vertically per second and 5 units to the right horizontally per second.

Question 3.
Write a function f(x,y), which will translate all points in the plane 2 units to the left and 5 units downward.
Answer:
f(x,y)=