Engage NY Eureka Math 8th Grade Module 7 Lesson 17 Answer Key
Eureka Math Grade 8 Module 7 Lesson 17 Example Answer Key
Example 1.
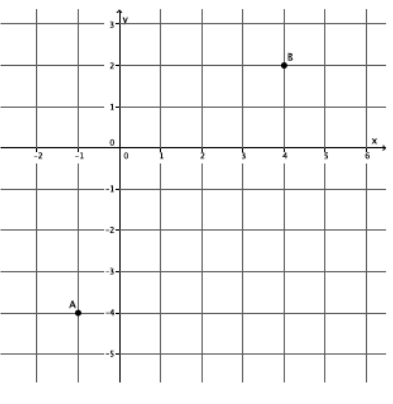
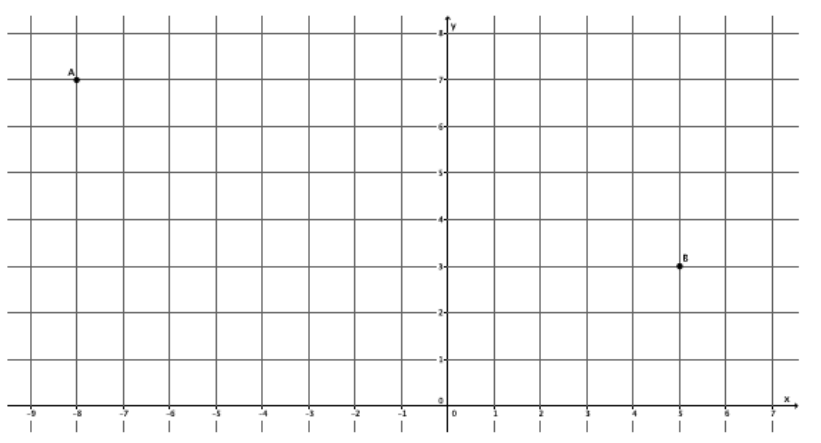
What is the distance between the two points A and B on the coordinate plane?
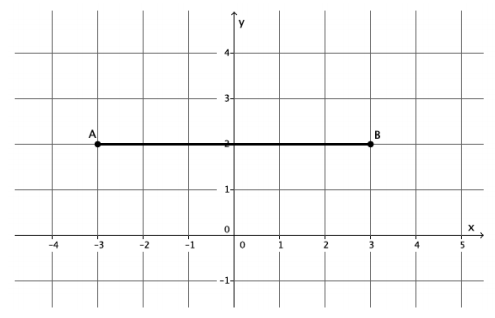
Answer:
What is the distance between the two points A and B on the coordinate plane?
The distance between points A and B is 6 units.
What is the distance between the two points A and B on the coordinate plane?
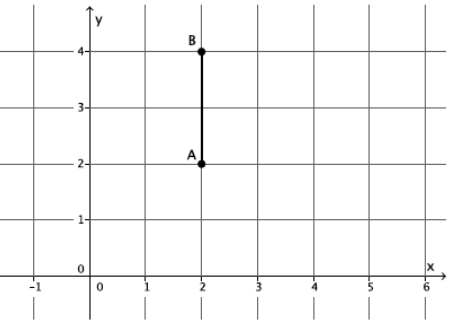
Answer:
What is the distance between the two points A and B on the coordinate plane?
The distance between points A and B is 2 units.
What is the distance between the two points A and B on the coordinate plane? Round your answer to the tenths place.
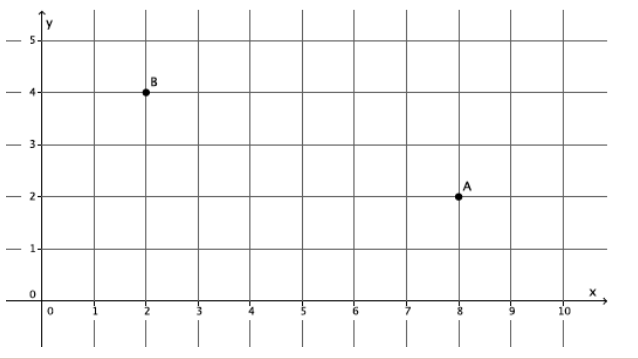
Answer:
What is the distance between the two points A and B on the coordinate plane? Round your answer to the tenths place.
Provide students time to solve the problem. Have students share their work and estimations of the distance between the points. The questions that follow can be used to guide students’ thinking.
We cannot simply count units between the points because the line that connects A to B is not horizontal or vertical. What have we done recently that allowed us to find the length of an unknown segment?
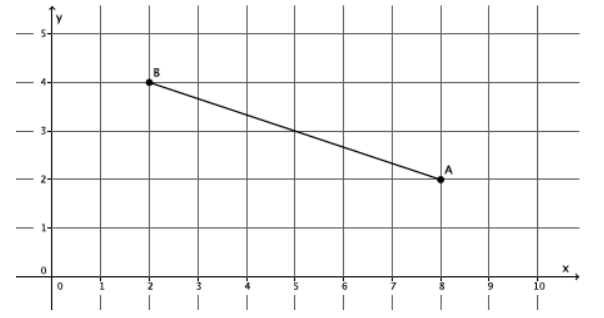
→ The Pythagorean theorem allows us to determine the length of an unknown side of a right triangle.
→ Use what you know about the Pythagorean theorem to determine the distance between points A and B.
Provide students time to solve the problem now that they know that the Pythagorean theorem can help them. If necessary, the questions below can guide students’ thinking.
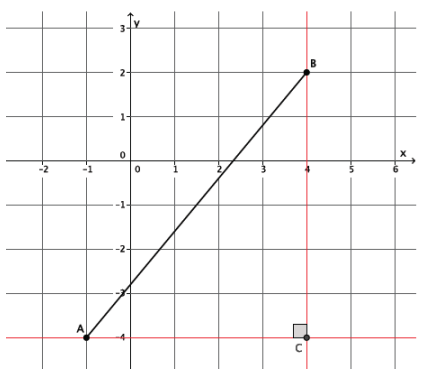
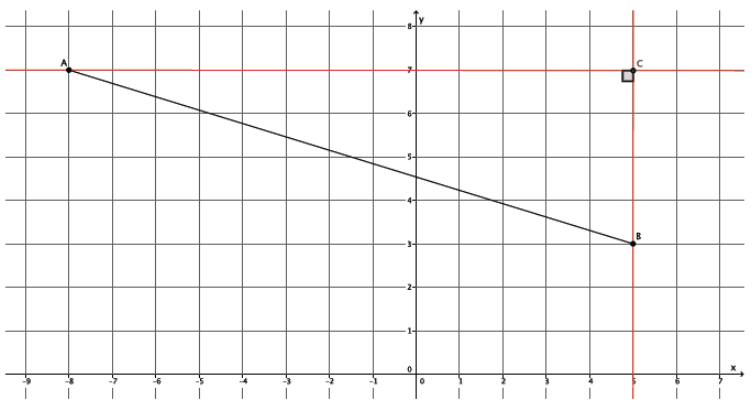
We must draw a right triangle so that |AB| is the hypotenuse. How can we construct the right triangle that we need?
→ Draw a vertical line through B and a horizontal line through A. Or, draw a vertical line through A and a horizontal line through B.
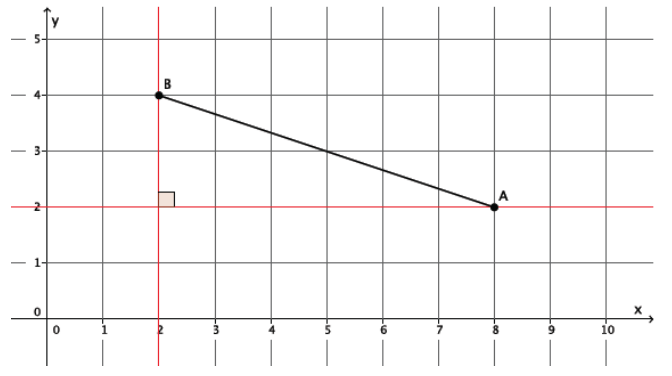
Let’s mark the point of intersection of the horizontal and vertical lines we drew as point C. What is the length of \(\overline{A C}\)? \(\overline{B C}\)?
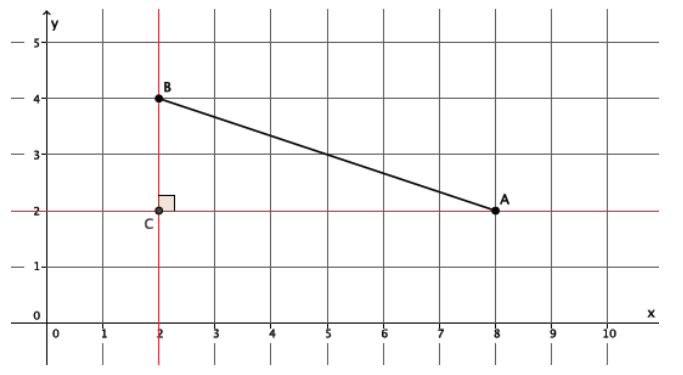
|AC| = 6 units, and |BC| = 2 units
Now that we know the lengths of the legs of the right triangle, we can determine the length of \(\overline{A B}\).
Remind students that because we are finding a length, we need only consider the positive value of the square root because a negative length does not make sense. If necessary, remind students of this fact throughout their work in this lesson.
Let c be the length of \(\overline{A B}\).
22 + 62 = c2
4 + 36 = c2
40 = c2
\(\sqrt{40}\) = c
6.3 ≈ c The distance between points A and B is approximately 6.3 units.
Example 2.
Given two points A and B on the coordinate plane, determine the distance between them. First, make an estimate; then, try to find a more precise answer. Round your answer to the tenths place.
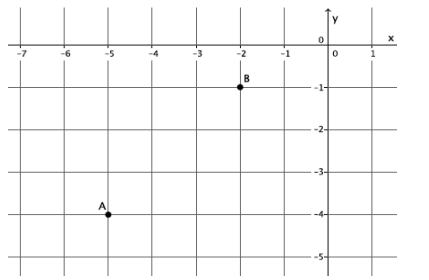
Answer:
Provide students time to solve the problem. Have students share their work and estimations of the distance between the points. The questions below can be used to guide students’ thinking.
We know that we need a right triangle. How can we draw one?
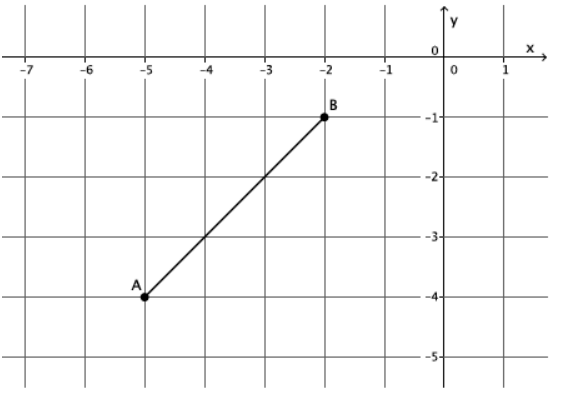
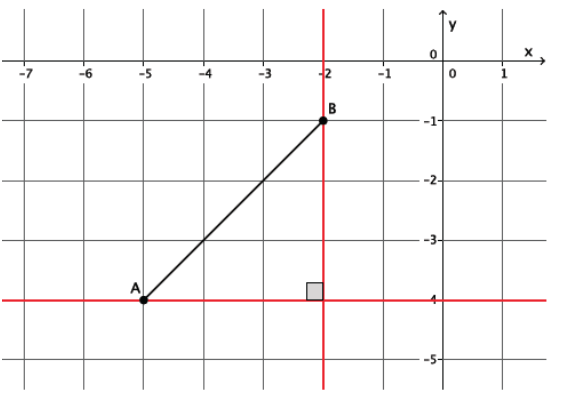
→ Draw a vertical line through B and a horizontal line through A. Or draw a vertical line through A and a horizontal line through B.
Mark the point C at the intersection of the horizontal and vertical lines. What do we do next?
→ Count units to determine the lengths of the legs of the right triangle, and then use the Pythagorean theorem to find |AB|.
Show the last diagram, and ask a student to explain the answer.
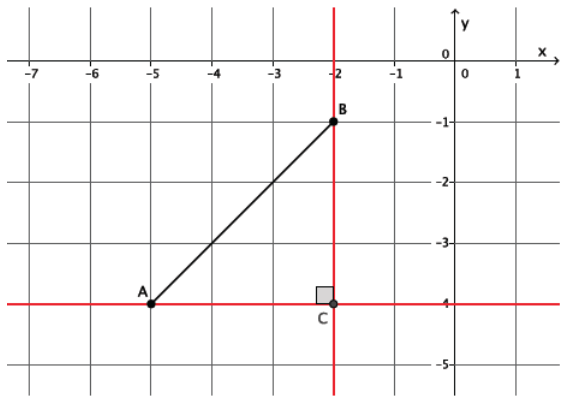
|AC| = 3 units, and |BC| = 3 units. Let c be |AB|.
32 + 32 = c2
9 + 9 = c2
18 = c2
\(\sqrt{18}\) = c
4.2 ≈ c
The distance between points A and B is approximately 4.2 units.
Example 3.
Is the triangle formed by the points A, B, C a right triangle?
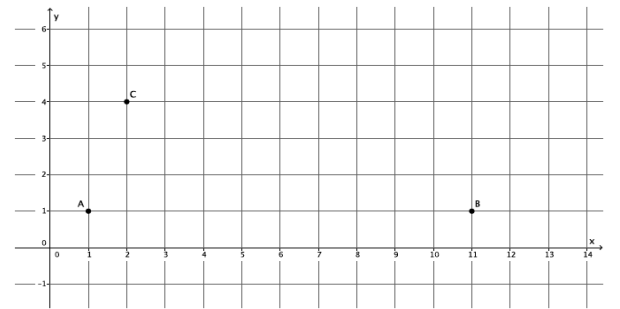
Answer:
→How can we verify if a triangle is a right triangle?
Use the converse of the Pythagorean theorem.
→ What information do we need about the triangle in order to use the converse of the Pythagorean theorem, and how would we use it?
We need to know the lengths of all three sides; then, we can check to see if the side lengths satisfy the Pythagorean theorem.
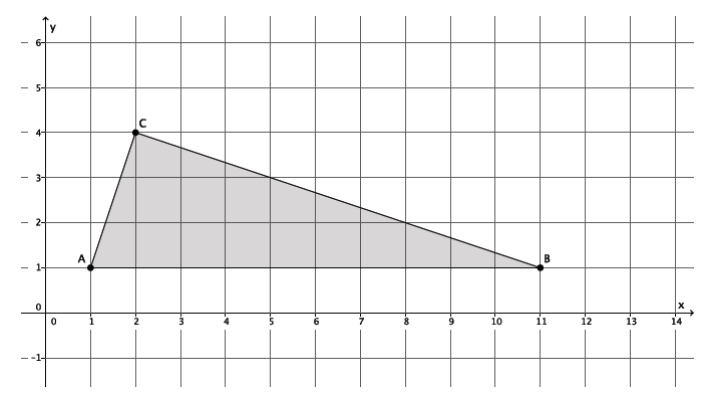
→ Clearly, |AB| = 10 units. How can we determine |AC|?
To find |AC|, follow the same steps used in the previous problem. Draw horizontal and vertical lines to form a right triangle, and use the Pythagorean theorem to determine the length.
→ Determine |AC|. Leave your answer in square root form unless it is a perfect square.
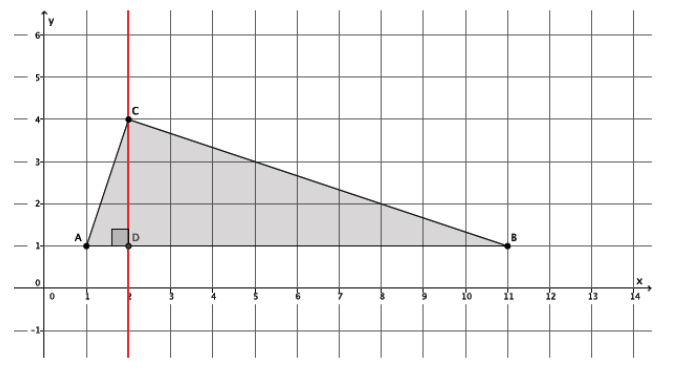
Let c represent |AC|.
12 + 32 = c2
1 + 9 = c2
10 = c2
\(\sqrt{10}\) = c
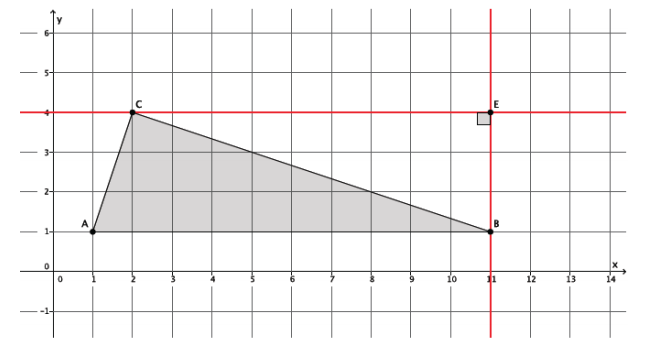
Now, determine |BC|. Again, leave your answer in square root form unless it is a perfect square.
Let c represent |BC|.
92 + 32 = c2
81 + 9 = c2
90 = c2
\(\sqrt{90}\) = c
→ The lengths of the three sides of the triangle are 10 units, \(\sqrt{10}\) units, and \(\sqrt{90}\) units. Which number represents the hypotenuse of the triangle? Explain.
The side \(\overline{A B}\) must be the hypotenuse because it is the longest side. When estimating the lengths of the other two sides, I know that \(\sqrt{10}\) is between 3 and 4, and \(\sqrt{90}\) is between 9 and 10. Therefore, the side that is 10 units in length is the hypotenuse.
→ Use the lengths 10, \(\sqrt{10}\), and \(\sqrt{90}\) to determine if the triangle is a right triangle.
Sample response:
(\(\sqrt{10}\))2 + (\(\sqrt{90}\))2 = 102
10 + 90 = 100
100 = 100
Therefore, the points A, B, C form a right triangle.
Eureka Math Grade 8 Module 7 Lesson 17 Exercise Answer Key
Exercises 1–4
For each of the Exercises 1–4, determine the distance between points A and B on the coordinate plane. Round your answer to the tenths place.
Exercise 1.
Answer:
Let c represent |AB|.
52 + 62 = c2
25 + 36 = c2
61 = c2
\(\sqrt{61}\) = c
7.8 ≈ c
The distance between points A and B is about 7.8 units.
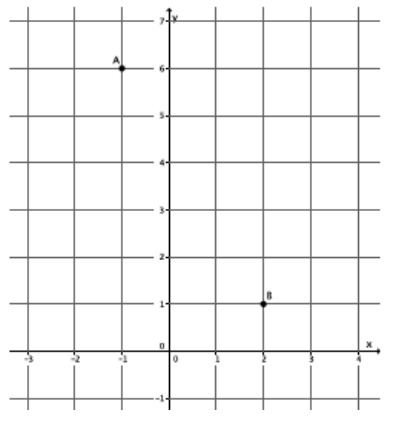
Exercise 2.
Answer:
Let c represent |AB|.
132 + 42 = c2
169 + 16 = c2
185 = c2
\(\sqrt{185}\) = c
13.6 ≈ c
The distance between points A and B is about 13.6 units.
Exercise 3.
Answer:
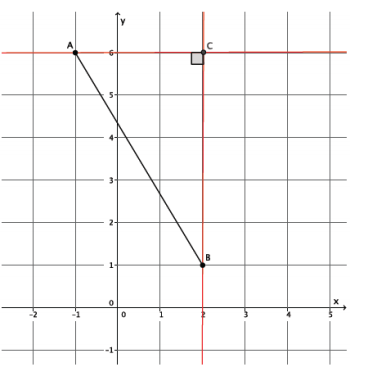
Let c represent |AB|.
32 + 52 = c2
9 + 25 = c2
34 = c2
\(\sqrt{34}\) = c
5.8 ≈ c
The distance between points A and B is about 5.8 units.
Exercise 4.
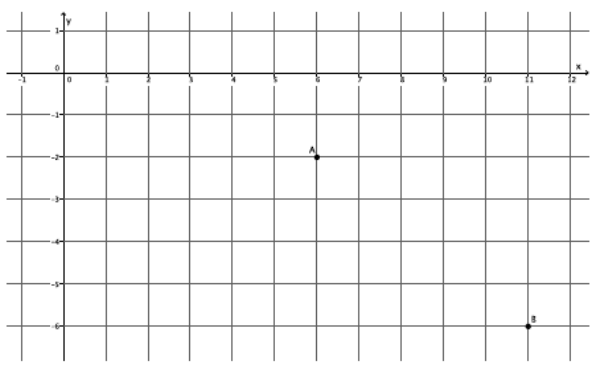
Answer:
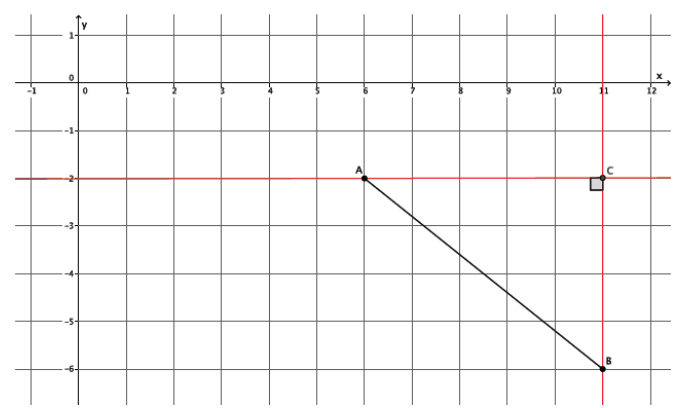
Let c represent |AB|.
52 + 42 = c2
25 + 16 = c2
41 = c2
\(\sqrt{41}\) = c
6.4 ≈ c
The distance between points A and B is about 6.4 units.
Eureka Math Grade 8 Module 7 Lesson 17 Problem Set Answer Key
For each of the Problems 1–4, determine the distance between points A and B on the coordinate plane. Round your answer to the tenths place.
Question 1.
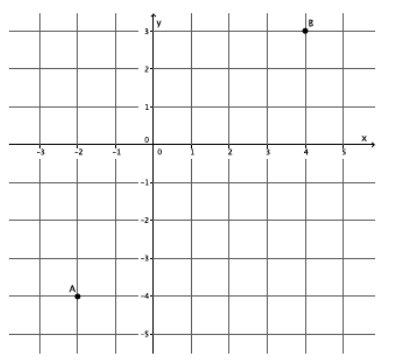
Answer:
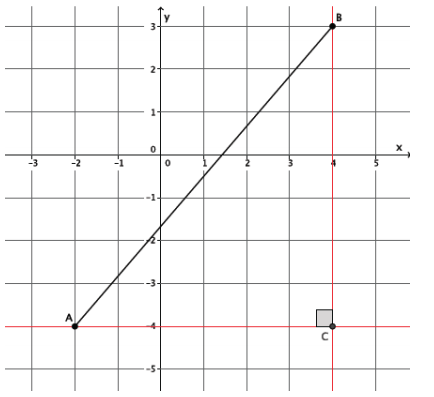
Let c represent |AB|.
62 + 72 = c2
36 + 49 = c2
85 = c2
\(\sqrt{85}\) = c
9.2 ≈ c
The distance between points A and B is about 9.2 units.
Question 2.
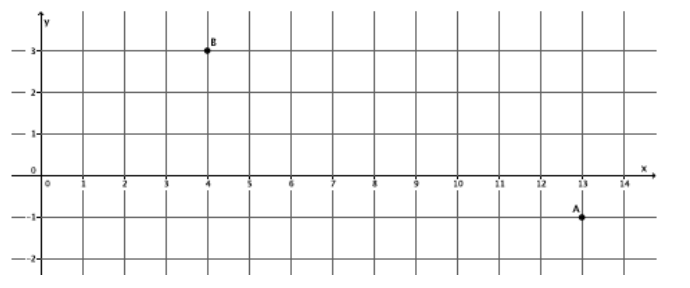
Answer:
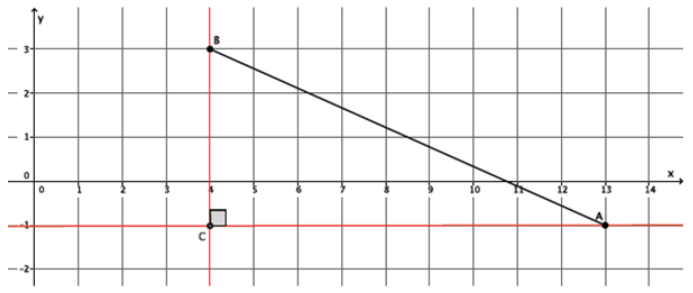
Let c represent |AB|.
92 + 42 = c2
81 + 16 = c2
97 = c2
\(\sqrt{97}\) = c
9.8 ≈ c
The distance between points A and B is about 9.8 units.
Question 3.
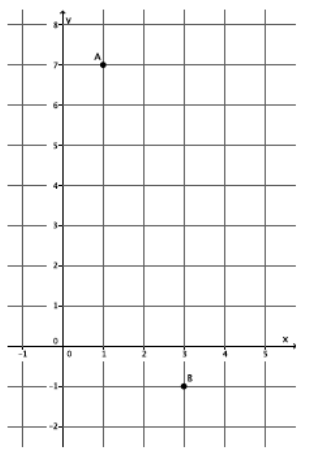
Answer:
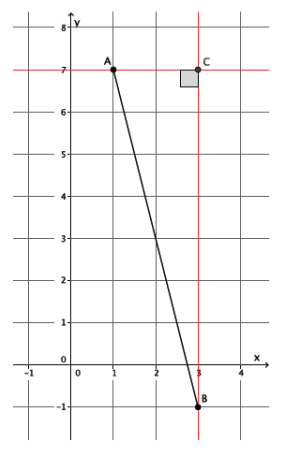
Let c represent |AB|.
22 + 82 = c2
4 + 64 = c2
68 = c2
\(\sqrt{68}\) = c
8.2 ≈ c
The distance between points A and B is about 8.2 units.
Question 4.
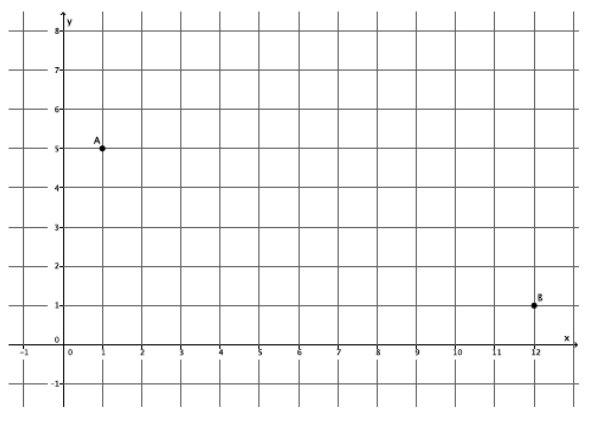
Answer:
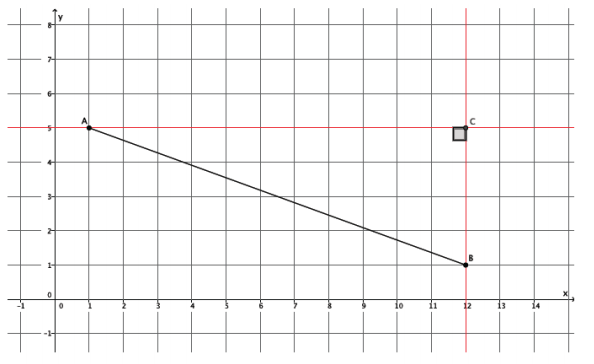
Let c represent |AB|.
112 + 42 = c2
121 + 16 = c2
137 = c2
\(\sqrt{137}\) = c
11.7 ≈ c
The distance between points A and B is about 11.7 units.
Question 5.
Is the triangle formed by points A, B, C a right triangle?
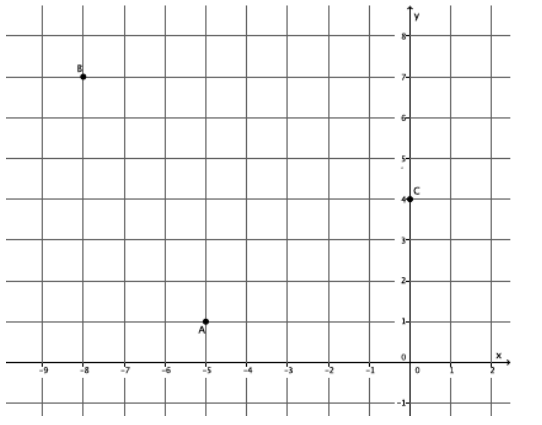
Answer:
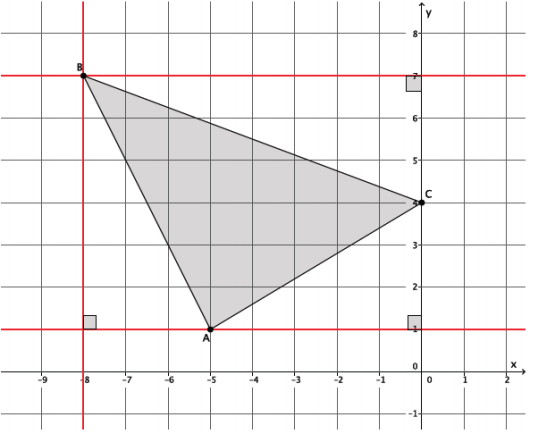
Let c represent |AB|.
32 + 62 = c2
9 + 36 = c2
45 = c2
\(\sqrt{45}\) = c
Let c represent |AC|.
32 + 52 = c2
9 + 25 = c2
34 = c2
\(\sqrt{34}\) = c
Let c represent |BC|.
32 + 82 = c2
9 + 64 = c2
73 = c2
\(\sqrt{73}\) = c
(\(\sqrt{45}\))2 + (\(\sqrt{34}\))2 = (\(\sqrt{73}\))2
45 + 34 = 73
79 ≠ 73
No, the points do not form a right triangle.
Eureka Math Grade 8 Module 7 Lesson 17 Exit Ticket Answer Key
Use the following diagram to answer the questions below.
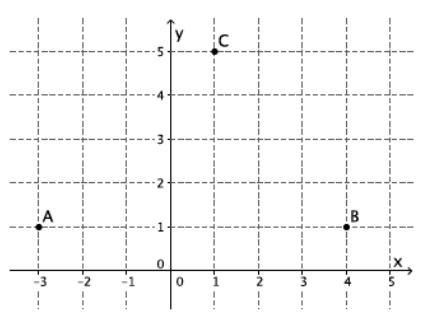
Question 1.
Determine |AC|. Leave your answer in square root form unless it is a perfect square.
Answer:
Let c represent |AC|.
42 + 42 = c2
16 + 16 = c2
32 = c2
\(\sqrt{32}\) = c
Question 2.
Determine |CB|. Leave your answer in square root form unless it is a perfect square.
Answer:
Let d represent |CB|.
32 + 42 = d2
9 + 16 = d2
25 = d2
\(\sqrt{25}\) = d
5 = d
Question 3.
Is the triangle formed by the points A, B, C a right triangle? Explain why or why not.
Answer:
Using the lengths 5,\(\sqrt{32}\), and |AB| = 7 to determine if the triangle is a right triangle, I have to check to see if
52 + (\(\sqrt{32}\))2 = 72
25 + 32 ≠ 49
Therefore, the triangle formed by the points A, B, C is not a right triangle because the lengths of the triangle do not satisfy the Pythagorean theorem.