Engage NY Eureka Math 7th Grade Module 3 Lesson 25 Answer Key
Eureka Math Grade 7 Module 3 Lesson 25 Example Answer Key
Example 1: Volume of a Fish Tank
Jay has a small fish tank. It is the same shape and size as the right rectangular prism shown in the Opening Exercise.
a. The box it came in says that it is a 3-gallon tank. Is this claim true? Explain your reasoning. Recall that 1 gal = 231 in3.
Answer:
The volume of the tank is 715 in3. To convert cubic inches to gallons, divide by 231.
715 in3 ∙ \(\frac{1 \text { gallon }}{231 \mathrm{in}^{3}}\) = 3.09 gallons
The claim is true if you round to the nearest whole gallon.
b. The pet store recommends filling the tank to within 1.5 in of the top. How many gallons of water will the tank hold if it is filled to the recommended level?
Answer:
Use 8.5 in. instead of 10 in. to calculate the volume. V = 11 in ∙ 6.5 in ∙ 8.5 in = 607.75 in3.
607.75 in3 ∙ \(\frac{1 \text { gallon }}{231 \mathrm{in}^{3}}\) = 2.63 gallons
c. Jay wants to cover the back, left, and right sides of the tank with a background picture. How many square inches will be covered by the picture?
Answer:
Back side area = 10 in ∙ 11 in = 110 in2
Left and right side area = 2(6.5 in)(10 in) = 130 in2
The total area to be covered with the background picture is 240 in2.
d. Water in the tank evaporates each day, causing the water level to drop. How many gallons of water have evaporated by the time the water in the tank is four inches deep? Assume the tank was filled to within
Answer:
1.5 in. of the top to start.
Volume when water is 4 in. deep:
11 in. ∙ 6.5 in. ∙ 4 in = 286in3
Difference in the two volumes:
607.75 in3-286 in3 = 321.75 in3
When the water is filled to within 1.5 in of the top, the volume is 607.75 in3. When the water is 4 in deep, the volume is 286 in3. The difference in the two volumes is 321.75 in3. Converting cubic inches to gallons by dividing by 231 gives a difference of 1.39 gal., which means 1.39 gal. of water have evaporated.
Eureka Math Grade 7 Module 3 Lesson 25 Exercise Answer Key
Opening Exercise
What is the surface area and volume of the right rectangular prism?
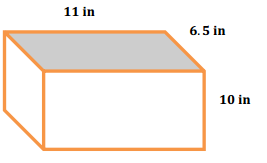
Answer:
Surface Area = 2(11 in)(6.5 in) + 2(10 in)(6.5 in) + 2(11 in)(10 in) = 493 in2
Volume = 11 in ∙ 6.5 in ∙ 10 in = 715 in3
Exercise 1: Fish Tank Designs
Two fish tanks are shown below, one in the shape of a right rectangular prism (R) and one in the shape of a right trapezoidal prism (T).
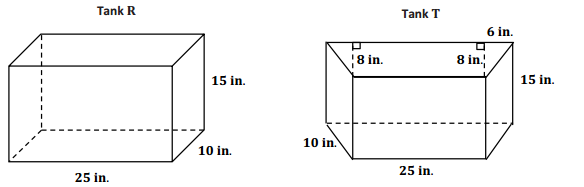
a. Which tank holds the most water? Let Vol(R) represent the volume of the right rectangular prism and Vol(T) represent the volume of the right trapezoidal prism. Use your answer to fill in the blanks with Vol(R) and Vol(T).
__________________ < __________________
Answer:
Volume of the right rectangular prism: (25 in × 10 in) × 15 in = 3,750 in3
Volume of the right trapezoidal prism: (31 in × 8 in) × 15 in = 3,720 in3
The right rectangular prism holds the most water.
Vol(T) < Vol(R)
b. Which tank has the most surface area? Let SA(R) represent the surface area of the right rectangular prism and SA(T) represent the surface area of the right trapezoidal prism. Use your answer to fill in the blanks with SA(R) and SA(T).
__________________ < __________________
Answer:
The surface area of the right rectangular prism:
2(25 in × 10 in) + 2(25 in × 15 in) + 2(10 in × 15 in) = 500 in2 + 750 in2 + 300 in2 = 1,550 in2
The surface area of the right trapezoidal prism:
2(31 in × 8 in) + 2(10 in × 15 in) + (25 in × 15 in) + (31 in × 15 in) = 496 in2 + 300 in2 + 375 in2 + 555 in2 = 1726 in2
The right trapezoidal prism has the most surface area.
SA(R) < SA(T)
c. Water evaporates from each aquarium. After the water level has dropped \(\frac{1}{2}\) inch in each aquarium, how many cubic inches of water are required to fill up each aquarium? Show work to support your answers.
Answer:
The right rectangular prism will need 125 in3 of water. The right trapezoidal prism will need 124 in3 of water. First, decrease the height of each prism by a half inch and recalculate the volumes. Then, subtract each answer from the original volume of each prism.
NewVol(R) = (25 in)(10 in)(14.5 in) = 3,625 in3
NewVol(T) = (31 in)(8 in)(14.5 in) = 3,596 in3
3,750 in3-3,625 in3 = 125 in3
3,720 in3-3,596 in3 = 124 in3
Exercise 2: Design Your Own Fish Tank
Design at least three fish tanks that will hold approximately 10 gallons of water. All of the tanks should be shaped like right prisms. Make at least one tank have a base that is not a rectangle. For each tank, make a sketch, and calculate the volume in gallons to the nearest hundredth.
Answer:
Three possible designs are shown below.

10 gal. is 2,310 in3
Rectangular Base: Volume = 2,304 in3 or 9.97 gal.
Triangular Base: Volume = 2,240 in3 or 9.70 gal.
Hexagonal Base: Volume = 2,325 in3 or 10.06 gal.
Challenge: Each tank is to be constructed from glass that is \(\frac{1}{4}\) in. thick. Select one tank that you designed, and determine the difference between the volume of the total tank (including the glass) and the volume inside the tank. Do not include a glass top on your tank.
Answer:
Height = 12 in-\(\frac{1}{4}\) in = 11.75 in
Length = 24 in-\(\frac{1}{2}\) in = 23.5 in
Width = 8 in-\(\frac{1}{2}\) in = 7.5 in
Inside Volume = 2,070.9 in3
The difference between the two volumes is 233.1 in3, which is approximately 1 gal.
Eureka Math Grade 7 Module 3 Lesson 25 Problem Set Answer Key
Question 1.
The dimensions of several right rectangular fish tanks are listed below. Find the volume in cubic centimeters, the capacity in liters (1 L = 1000 cm3), and the surface area in square centimeters for each tank. What do you observe about the change in volume compared with the change in surface area between the small tank and the extra-large tank?
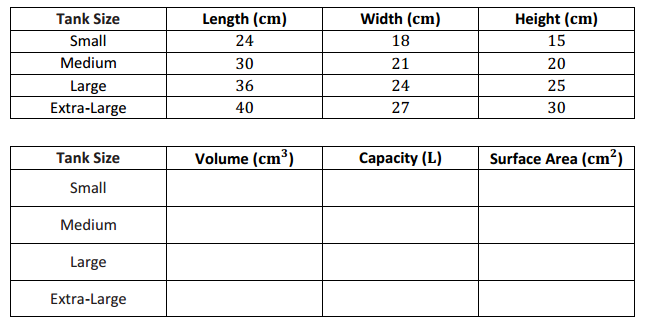
Answer:
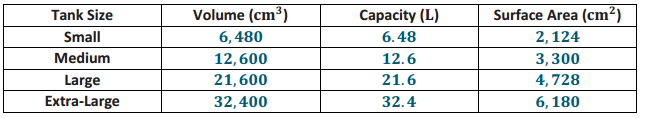
While the volume of the extra-large tank is about five times the volume of the small tank, its surface area is less than three times that of the small tank.
Question 2.
A rectangular container 15 cm long by 25 cm wide contains 2.5 L of water.
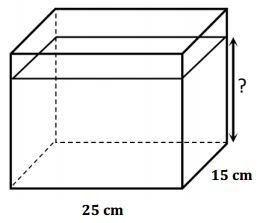
a. Find the height of the water level in the container. (1 L = 1000 cm3)
2.5 L = 2,500 cm3
To find the height of the water level, divide the volume in cubic centimeters by the area of the base.
\(\frac{2,500 \mathrm{~cm}^{3}}{25 \mathrm{~cm} \cdot 15 \mathrm{~cm}}\) = 6 \(\frac{2}{3}\) cm
b. If the height of the container is 18 cm, how many more liters of water would it take to completely fill the container?
Answer:
Volume of tank: (25 cm × 15 cm) × 18 cm = 6,750 cm3
Capacity of tank: 6.75 L
Difference: 6.75 L- 2.5 L = 4.25 L
c. What percentage of the tank is filled when it contains 2.5 L of water?
Answer:
\(\frac{2.5 L}{6.75 L}\) = 0.37 = 37%
Question 3.
A rectangular container measuring 20 cm by 14.5 cm by 10.5 cm is filled with water to its brim. If 300 cm3 are drained out of the container, what will be the height of the water level? If necessary, round to the nearest tenth.
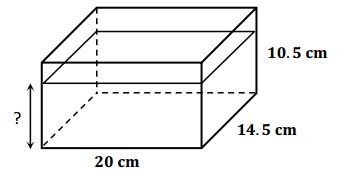
Answer:
Volume: (20 cm × 14.5 cm) × 10.5 cm = 3,045 cm3
Volume after draining: 2,745 cm3
Height (divide the volume by the area of the base):
\(\frac{2745 \mathrm{~cm}^{3}}{20 \mathrm{~cm} \times 14.5 \mathrm{~cm}}\) ≈ 9.5 cm
Question 4.
Two tanks are shown below. Both are filled to capacity, but the owner decides to drain them. Tank 1 is draining at a rate of 8 liters per minute. Tank 2 is draining at a rate of 10 liters per minute. Which tank empties first?
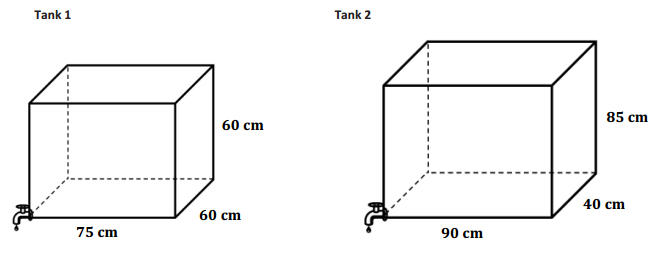
Answer:
Tank 1 Volume: 75 cm × 60 cm × 60 cm = 270,000 cm3
Tank 2 Volume: 90 cm × 40 cm × 85 cm = 306,000 cm3
Tank 1 Capacity: 270 L
Tank 2 Capacity: 306 L
To find the time to drain each tank, divide the capacity by the rate (liters per minute).
Time to drain tank 1: \(\frac{270 \mathrm{~L}}{8 \frac{\mathrm{L}}{\mathrm{min}}}\) = 33.75 min.
Time to drain tank 2: \(\frac{360 \mathrm{~L}}{10 \frac{\mathrm{L}}{\mathrm{min}}}\) = 30.6 min.
Tank 2 empties first.
Question 5.
Two tanks are shown below. One tank is draining at a rate of 8 liters per minute into the other one, which is empty. After 10 minutes, what will be the height of the water level in the second tank? If necessary, round to the nearest minute.
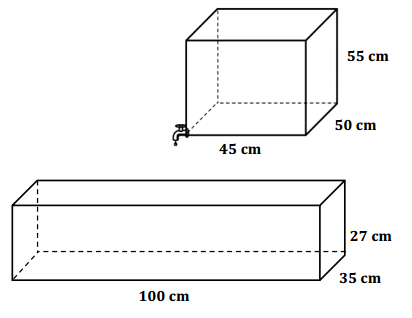
Answer:
Volume of the top tank: 45 cm × 50 cm × 55 cm = 123,750 cm3
Capacity of the top tank: 123.75 L
At 8 L/min for 10 minutes, 80 L will have drained into the bottom tank after 10 minutes.
That is 80,000 cm3. To find the height, divide the volume by the area of the base.
\(\frac{80,000 \mathrm{~cm}^{3}}{100 \mathrm{~cm} \cdot 35 \mathrm{~cm}}\) ≈ 22.9 cm
After 10 minutes, the height of the water in the bottom tank will be about 23 cm.
Question 6.
Two tanks with equal volumes are shown below. The tops are open. The owner wants to cover one tank with a glass top. The cost of glass is $0.05 per square inch. Which tank would be less expensive to cover? How much less?
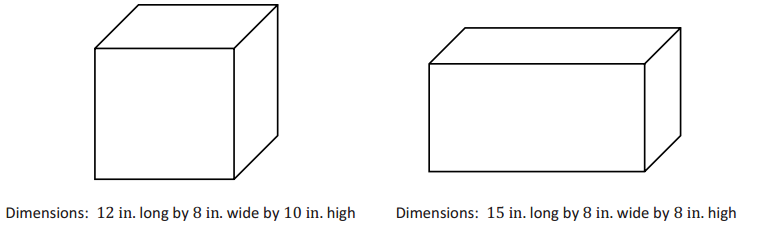
Answer:
Dimensions: 12 in. long by 8 in. wide by 10 in. high
Surface area: 96 in2
Cost: \(\frac{\$ 0.05}{\mathrm{in}^{2}}\) ∙ 96 in2 = $4.80
Dimensions: 15 in. long by 8 in. wide by 8 in. high
Surface area: 120 in2
Cost: \(\frac{\$ 0.05}{\mathrm{in}^{2}}\) ∙ 120 in2 = $6.00
The first tank is less expensive. It is $1.20 cheaper.
Question 7.
Each prism below is a gift box sold at the craft store.
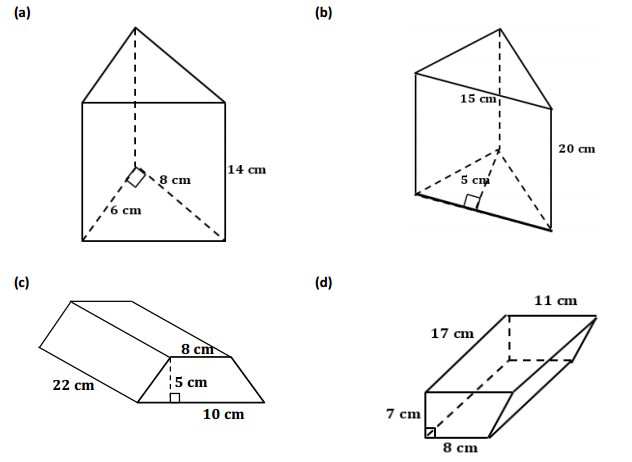
a. What is the volume of each prism?
Answer:
(a) Volume = 336 cm3
(b) Volume = 750 cm3
(c) Volume = 990 cm3
(d) Volume = 1130.5 cm3
b. Jenny wants to fill each box with jelly beans. If one ounce of jelly beans is approximately 30 cm^3, estimate how many ounces of jelly beans Jenny will need to fill all four boxes? Explain your estimates.
Answer:
Divide each volume in cubic centimeters by 30.
(a) 11.2 ounces
(b) 25 ounces
(c) 33 ounces
(d) 37.7 ounces
Jenny would need a total of 106.9 ounces.
Question 8.
Two rectangular tanks are filled at a rate of 0.5 cubic inches per minute. How long will it take each tank to be half-full?
a. Tank 1 Dimensions: 15 in. by 10 in. by 12.5 in.
Answer:
Volume: 1,875 in3
Half of the volume is 937.5 in3.
To find the number of minutes, divide the volume by the rate in cubic inches per minute.
Time: 1,875 minutes.
b. Tank 2 Dimensions: 2 \(\frac{1}{2}\) in. by 3 \(\frac{3}{4}\) in. by 4 \(\frac{3}{8}\) in.
Answer:
Volume: \(\frac{2625}{64}\) in3
Half of the volume is \(\frac{2625}{128}\) in3.
To find the number of minutes, divide the volume by the rate in cubic inches per minute.
Time: 41 minutes
Eureka Math Grade 7 Module 3 Lesson 25 Exit Ticket Answer Key
Melody is planning a raised bed for her vegetable garden.
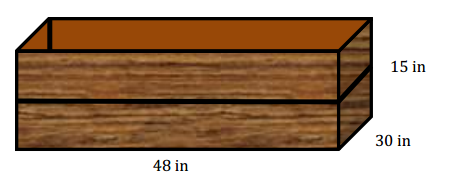
a. How many square feet of wood does she need to create the bed?
Answer:
2(4 ft)(1.25 ft) + 2(2.5 ft)(1.25 ft) = 16.25 ft2
The dimensions in feet are 4 ft. by 1.25 ft. by 2.5 ft. The lateral area is 16.25 ft2.
b. She needs to add soil. Each bag contains 1.5 cubic feet. How many bags will she need to fill the vegetable garden?
Answer:
V = 4 ft ∙ 1.25 ft ∙ 2.5 ft = 12.5 ft3
The volume is 12.5 ft3. Divide the total cubic feet by 1.5 ft3 to determine the number of bags.
12.5 ft3 ÷ 1.5 ft3 = 8 \(\frac{1}{3}\)
Melody will need to purchase 9 bags of soil to fill the garden bed.