Engage NY Eureka Math 7th Grade Module 3 Lesson 1 Answer Key
Eureka Math Grade 7 Module 3 Lesson 1 Example Answer Key
Example 1.
Any Order, Any Grouping Property with Addition
a. Rewrite 5x+3x and 5x-3x by combining like terms.
Write the original expressions and expand each term using addition. What are the new expressions equivalent to?
Answer:
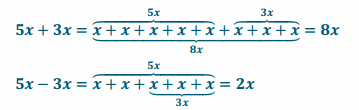
Because both terms have the common factor of x, we can use the distributive property to create an equivalent expression.
5x+3x=(5+3)x=8x
5x-3x=(5-3)x=2x
Ask students to try to find an example (a value for x) where 5x+3x≠8x or where
5x-3x≠2x. Encourage them to use a variety of positive and negative rational numbers. Their failure to find a counterexample helps students realize what equivalence means.
In Example 1, part (b), students see that the commutative and associative properties of addition are regularly used in consecutive steps to reorder and regroup like terms so that they can be combined. Because the use of these properties does not change the value of an expression or any of the terms within the expression, the commutative and associative properties of addition can be used simultaneously. The simultaneous use of these properties is referred to as the any order, any grouping property.
b. Find the sum of 2x+1 and 5x.
Answer:
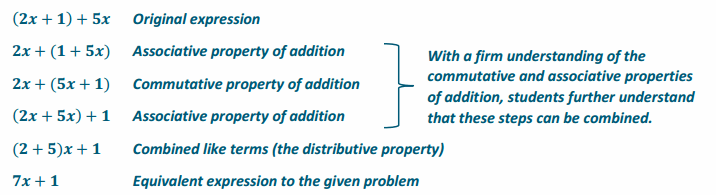
7x+1 Equivalent expression to the given problem
→ Why did we use the associative and commutative properties of addition?
→ We reordered the terms in the expression to group together like terms so that they could be combined.
→ Did the use of these properties change the value of the expression? How do you know?
→ The properties did not change the value of the expression because each equivalent expression includes the same terms as the original expression, just in a different order and grouping.
→ If a sequence of terms is being added, the any order, any grouping property allows us to add those terms in any order by grouping them together in any way.
→ How can we confirm that the expressions (2x+1)+5x and 7x+1 are equivalent expressions?
→ When a number is substituted for the x in both expressions, they both should yield equal results.
The teacher and student should choose a number, such as 3, to substitute for the value of x and together check to see if both expressions evaluate to the same result.
Given Expression
((2x+1)+5x
(2∙3+1)+5∙3
(6+1)+15
(7)+15
22
Equivalent Expression?
7x+1
7∙3+1
21+1
22
→ The expressions both evaluate to 22; however, this is only one possible value of x. Challenge students to find a value for x for which the expressions do not yield the same number. Students find that the expressions evaluate to equal results no matter what value is chosen for x.
→ What prevents us from using any order, any grouping in part (c), and what can we do about it?
→ The second expression, (5a-3), involves subtraction, which is not commutative or associative; however, subtracting a number x can be written as adding the opposite of that number. So, by changing subtraction to addition, we can use any order and any grouping.
c. Find the sum of -3a+2 and 5a-3.
Answer:
(-3a+2)+(5a-3) Original expression
-3a+2+5a+(-3) Add the opposite (additive inverse)
-3a+5a+2+(-3) Any order, any grouping
2a+(-1) Combined like terms (Stress to students that the expression is not yet simplified.)
2a-1 Adding the inverse is subtracting.
→ What was the only difference between this problem and those involving all addition?
→ We first had to rewrite subtraction as addition; then, this problem was just like the others.
Example 2.
Any Order, Any Grouping with Multiplication
Find the product of 2x and 3.
Answer:

→ Why did we use the associative and commutative properties of multiplication?
→ We reordered the factors to group together the numbers so that they could be multiplied.
→ Did the use of these properties change the value of the expression? How do you know?
→ The properties did not change the value of the expression because each equivalent expression includes the same factors as the original expression, just in a different order or grouping.
→ If a product of factors is being multiplied, the any order, any grouping property allows us to multiply those factors in any order by grouping them together in any way.
Example 3.
Any Order, Any Grouping in Expressions with Addition and Multiplication
Use any order, any grouping to write equivalent expressions.
a. 3(2x)
Answer:
(3∙2)x
6x
b. 4y(5)
Answer:
(4∙5)y
20y
c. 4∙2∙z
Answer:
(4∙2)z
8z
d. 3(2x)+4y(5)
Answer:
![]()
(3∙2)x+(4∙5)y
6x+20y
e. 3(2x)+4y(5)+4∙2∙z
Answer:
![]()
(3∙2)x+(4∙5)y+(4∙2)z
6x+20y+8z
f. Alexander says that 3x+4y is equivalent to (3)(4)+xy because of any order, any grouping. Is he correct? Why or why not?
Encourage students to substitute a variety of positive and negative rational numbers for x and y because in order for the expressions to be equivalent, the expressions must evaluate to equal numbers for every substitution of numbers into all the letters in both expressions.
Alexander is incorrect; the expressions are not equivalent because if we, for example, let x=-2 and let
y=-3, then we get the following:
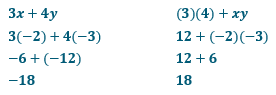
-18≠18, so the expressions cannot be equivalent.
→ What can be concluded as a result of part (f)?
→ Any order, any grouping cannot be used to mix multiplication with addition. Numbers and letters that are factors within a given term must remain factors within that term.
Eureka Math Grade 7 Module 3 Lesson 1 Problem Set Answer Key
For Problems 1–9, write equivalent expressions by combining like terms. Verify the equivalence of your expression and the given expression by evaluating each for the given values: a=2, b=5, and c=-3.
Question 1.
3a+5a
Answer:
8a
8(2)
16
3(2)+5(2)
6+10
16
Question 2.
8b – 4b
Answer:
4b
4(5)
20
8(5)-4(5)
40-20
20
Question 3.
5c+4c+c
10c
10(-3)
-30
5(-3)+4(-3)+(-3)
-15+(-12)+(-3)
-27+(-3)
-30
Question 4.
3a+6+5a
8a+6
8(2)+6
16+6
22
3(2)+6+5(2)
6+6+10
12+10
22
Question 5.
8b + 8 – 4b
4b+8
4(5)+8
20+8
28
8(5)+8-4(5)
40+8-20
48-20
28
Question 6.
2c
2(-3)
-6
5(-3)-4(-3)+(-3)
-15+(-4(-3))+(-3)
-15+(12)+(-3)
-3+(-3)
-6
Eureka Math Grade 7 Module 3 Lesson 1 Exit Ticket Answer Key
Question 1.
Write an equivalent expression to 2x+3+5x+6 by combining like terms.
Answer:
2x+3+5x+6
2x+5x+3+6
7x+9
Question 2.
Find the sum of (8a+2b-4) and (3b-5).
Answer:
(8a+2b-4)+(3b-5)
8a+2b+(-4)+3b+(-5)
8a+2b+3b+(-4)+(-5)
8a+(5b)+(-9)
8a+5b-9
Question 3.
Write the expression in standard form: 4(2a)+7(-4b)+(3∙c∙5).
Answer:
(4∙2)a+(7∙(-4))b+(3∙5)c
8a+(-28)b+15c
8a-28b+15c
Eureka Math Grade 7 Module 3 Lesson 1 Opening Exercise Answer Key
Each envelope contains a number of triangles and a number of quadrilaterals. For this exercise, let t represent the number of triangles, and let q represent the number of quadrilaterals.
a. Write an expression using t and q that represents the total number of sides in your envelope. Explain what the terms in your expression represent.
Answer:
3t+4q. Triangles have 3 sides, so there will be 3 sides for each triangle in the envelope. This is represented by 3t. Quadrilaterals have 4 sides, so there will be 4 sides for each quadrilateral in the envelope. This is represented by 4q. The total number of sides will be the number of triangle sides and the number of quadrilateral sides together.
b. You and your partner have the same number of triangles and quadrilaterals in your envelopes. Write an expression that represents the total number of sides that you and your partner have. If possible, write more than one expression to represent this total.
Answer:
3t+4q+3t+4q; 2(3t+4q); 6t+8q
c. Each envelope in the class contains the same number of triangles and quadrilaterals. Write an expression that represents the total number of sides in the room.
Answer:
Answer depends on the number of students in the classroom. For example, if there are 12 students in the classroom, the expression would be 12(3t+4q), or an equivalent expression
d. Use the given values of t and q and your expression from part (a) to determine the number of sides that should be found in your envelope.
Answer:
3t+4q
3(4)+4(2)
12+8
20
There should be 20 sides contained in my envelope.
e. Use the same values for t and q and your expression from part (b) to determine the number of sides that should be contained in your envelope and your partner’s envelope combined.
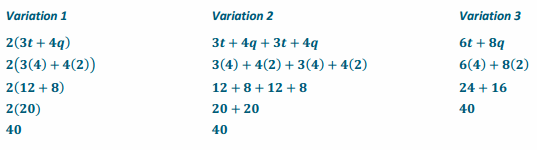
My partner and I have a combined total of 40 sides.
f. Use the same values for t and q and your expression from part (c) to determine the number of sides that should be contained in all of the envelopes combined.
Answer:
Answer will depend on the seat size of your classroom. Sample responses for a class size of 12:
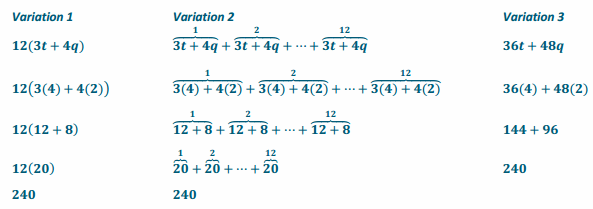
For a class size of 12 students, there should be 240 sides in all of the envelopes combined.
g. What do you notice about the various expressions in parts (e) and (f)?
Answer:
The expressions in part (e) are all equivalent because they evaluate to the same number: 40. The expressions in part (f) are all equivalent because they evaluate to the same number: 240. The expressions themselves all involve the expression 3t+4q in different ways. In part (e), 3t+3t is equivalent to 6t, and 4q+4q is equivalent to 8q. There appear to be several relationships among the representations involving the commutative, associative, and distributive properties.