Eureka Math Grade 6 Module 5 Lesson 9 Answer Key

Engage NY Eureka Math Grade 6 Module 5 Lesson 9 Answer Key

Eureka Math Grade 6 Module 5 Lesson 9 Example Answer Key

Example 1
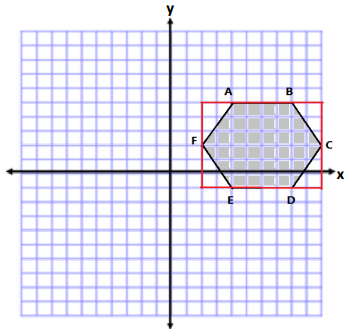
Jasjeet has made a scale drawing of a vegetable garden she plans to make in her backyard. She needs to determine the perimeter and area to know how much fencing and dirt to purchase. Determine both the perimeter and area.

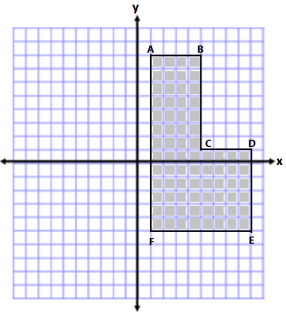
Answer:

AB = 4 units BC = 7 units CD = 4 units
DE = 6 units EF = 8 units AF = 13 units

Perimeter = 4 units + 7 units + 4 units + 6 units + 8 units + 13 units
Perimeter = 42 units

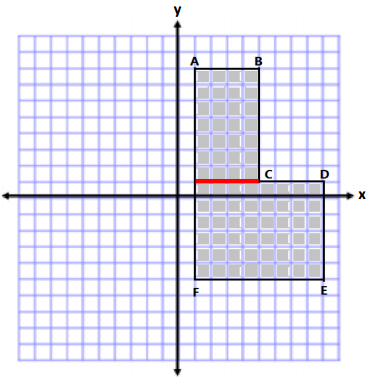
The area is determined by making a horizontal cut from (1, 1) to point C.
Area of Top
A = lw
A = (4 units)(7 units)
A = 28 units²

Area of Bottom
A = lw
A = (8 units) (6 units)
A = 48 units²

Total Area = 28 units² + 48 units²
Total Area = 76 units²

Example 2:

Calculate the area of the polygon using two different methods. Write two expressions to represent the two methods, and compare the structure of the expressions.

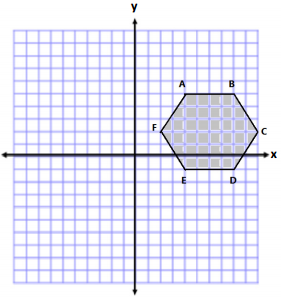
Answer:
Answers will vary. The following are two possible methods. However, students could also break the shape into two
triangles and a rectangle or use another correct method.

Method one:

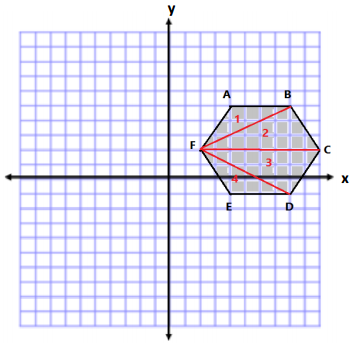
Method Two:

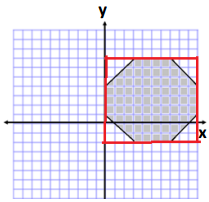
Area of Triangle 1 and 4
A = \(\frac{1}{2}\) bh
A = \(\frac{1}{2}\) (4 units)(3 units)
A = \(\frac{1}{2}\) (12 units²)
A = 6 units2
Since there are 2, we have a total area of 12 units².

Area of Triangle 2 and 3
A = \(\frac{1}{2}\) bh
A = \(\frac{1}{2}\) (8 units)(3 units)
A = \(\frac{1}{2}\) (24 units²)
A = 12 units2
Since there are 2, we have a total area of 24 units².

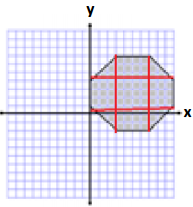
A = lw
A = (8 units) (6 units)
A = 48 units²

A = \(\frac{1}{2}\) bh
A = \(\frac{1}{2}\) (2 units)(3 units)
A = 3 units²

There are 4 triangles of equivalent base and height.
4(3 units2) = 12 units²

Total Area = 48 units² – 12 units²
Total area = 36 units²

Total Area = 12 units² + 24 units² = 36 units²
Total area = 36 units²

Expressions:
2(\(\frac{1}{2}\)(4)(3)) + 2(\(\frac{1}{2}\)(8)(3)) or (8)(6) – 4(\(\frac{1}{2}\)(2)(3))

The first expression shows terms being added together because I separated the hexagon into smaller pieces and had to add their areas back together.
The second expression shows terms being subtracted because I made a larger outside shape and then had to take away the extra pieces.

Eureka Math Grade 6 Module 5 Lesson 9 Exercise Answer Key

Question 1.
Determine the area of the following shapes.

a.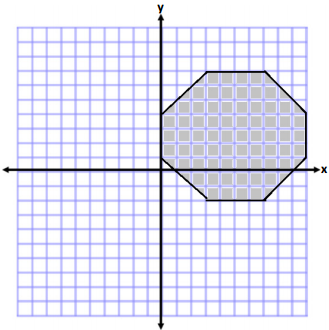
Answer:

Area of rectangle = lw
A = (10 units) (9 units)
A = 90 units ²

Area of Triangle
A = \(\frac{1}{2}\) bh
A = \(\frac{1}{2}\) (3 units) (3 units)
A = 4.5 units ²

4 triangles with equivalent base and height
4 (4.5 units ²) = 18 units²

Area = 90 units² – 18 units²
Area = 72 units²

Please note the students may also choose to solve by decomposing. Here is another option.

b.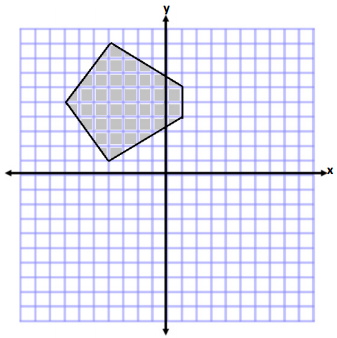
Answer:

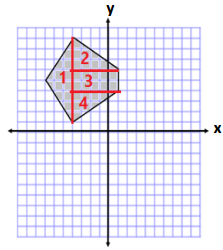
Area of Triangle 1
A = \(\frac{1}{2}\) bh
A = \(\frac{1}{2}\) (8 units)(3 units)
A = (4 units)(3 units)
A = 12 units²

Area of Triangles 2 and 4
A = \(\frac{1}{2}\) bh
A = \(\frac{1}{2}\) (5 units)(3 units)
A = \(\frac{1}{2}\) (15 units²)
A = 7.5 units²

Since triangles 2 and 4 have the same base and height measurements, the combined area is 15 units.

Area of Rectangle 3
A = bh
A = (5 units)(2 units)
A = 10 units²

Total Area = 12 units² + 15 units² + 10 units²
Total Area = 37 units²

Another correct solution might start with the following diagram:

Question 2.
Determine the area and perimeter of the following shapes.

a.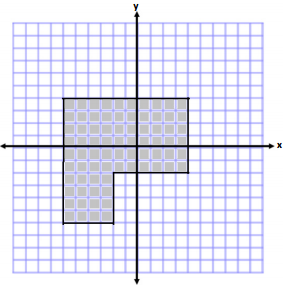
Answer:

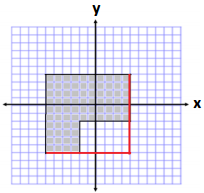
Area of Large Square

A = s²
A = (10 units)²
A = 100units²

Removed Piece
A = bh
A = (6 units)(4 units)
A = 24 units²

Area = 100 units² – 24 units²
Area = 76 units²

Perimeter = 10 units + 6 units + 6 units + 4 units + 4 units + 10 units
Perimeter = 40 units

Other correct solutions might start with the following diagrams:

b.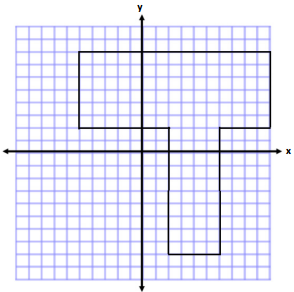
Answer:

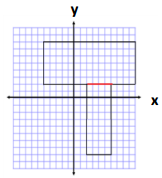
Area:

Horizontal Area
A = bh
A = (15 units) (6 units)
A = 90 units²

Vertical Area
A = bh
A = (10 units) (4 units)
A = 40 units²

Total Area = 90 units² + 40 units²
Total Area = 130 units²

Perimeter = 15 units + 6 units + 4 units + 10 units + 4 units + 10 units + 7 units + 6 units
Perimeter = 62 units

Other correct solutions might start with the following diagrams:

Eureka Math Grade 6 Module 5 Lesson 9 Problem Set Answer Key

Question 1.
Determine the area of polygon.

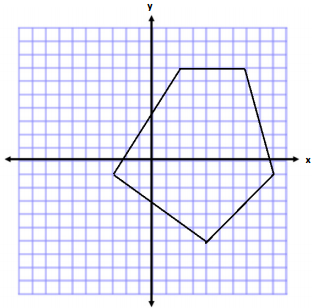
Answer:

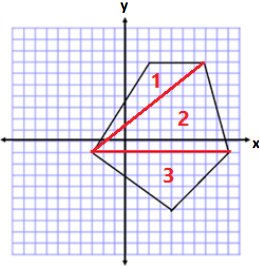
Area of Triange 1
A = \(\frac{1}{2}\) bh
A = \(\frac{1}{2}\) (5 units)(8 units)
A = \(\frac{1}{2}\) (40 units²)
A = 20 units²

Area of Triangle 2
A = \(\frac{1}{2}\) bh
A = \(\frac{1}{2}\) (12 units)(8 units)
A = \(\frac{1}{2}\) (96 units²)
A = 48 units²

Area of Tnangle 3
A = \(\frac{1}{2}\) bh
A = \(\frac{1}{2}\) (12 units)( 5 units)
A = \(\frac{1}{2}\) (60 units²)
A = 30 units²

Total Area = 20 units² + 48 units² + 30 units²
Total Area = 98 units²

Question 2.
Determine the area and perimeter of the polygon.

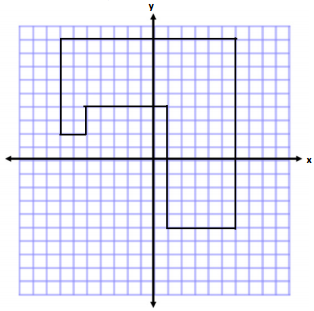
Answer:

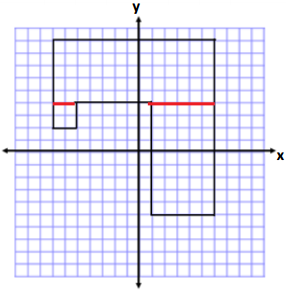
Area:

Horizontal Rectangle
A = bh
A = (13 units) (5 units)
A = 65 units²

Vertical Rectangle
A = bh
A = (5 units) (9 units)
A = 45 units²

Square
A = S²
A = (2 units)²
A = 4 units²

Total Area = 65 units² + 45 units² +4 units²
Total Area = 114 units²

Perimeter:

Perimeter = 2 units + 2 units + 7 units + 13 units + 14 units + 5 units + 9 units + 6 units
Perimeter = 58 units

Question 3.
Determine the area of the polygon. Then, write an expression that could be used to determine the area.

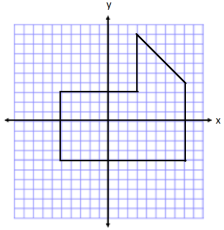
Answer:

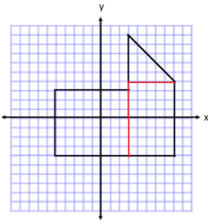
Area of Rectangle on Left
A = lw
A = (8 units) (7 units)
A = 56 units²

Area of Rectangle on Right
A = lw
A = (5 units) (8 units)
A = 40 units²

Area of Triangle on Top
A = \(\frac{1}{2}\) bh
A = \(\frac{1}{2}\) (5 units)(5 units)
A = 12.5 units²

Total Area = 56 units² + 40 units² + 12.5 units² = 108.5 units²
Expression: (8)(7) + (5)(8) + \(\frac{1}{2}\) (5)(5)

Question 4.
If the length of each square was worth 2 instead of 1, how would the area in Problem 3 change? How would your expression change to represent this area?
Answer:
If each length is twice as long, when they are multiplied, 2l × 2w = 41w. Therefore, the area will be four times larger when the side lengths are doubled.

I could multiply my entire expression by 4 to make it 4 times as big. 4 [(8)(7) + (5)(8) + \(\frac{1}{2}\) (5)(5)]

Question 5.
Determine the area of the polygon. Then, write an expression that represents the area.

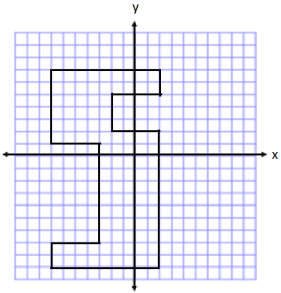
Answer:

Area of Outside Rectangle
A = lw
A = (9 units) (16 units)
A = 144 units²

Area of Rectangle on Left
A = lw
A = (4 units) (8 units)
A = 32 units²

Area of Rectangle on Right
A = lw
A = (4 units) (3 units)
A = 12 units²

Total Area = 144 units² – 32 units² – 12 units²
Total Area = 100 units²
Expression: (9)(16) – (4)(8) – (4)(3)

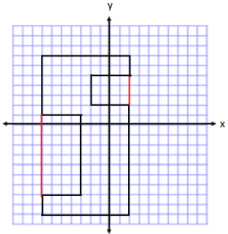
Question 6.
Describe another method you could use to find the area of the polygon in Problem 5. Then, state how the expression for the area would be different than the expression you wrote.
Answer:
I could have broken up the large shape into many smaller rectangles. Then I would need to add all the areas of these rectangles together to determine the total area.

My expression showed subtraction because I created o rectangle that was larger than the original polygon, and then I had to subtract the extra oreos. If I break the shape into pieces, I would need to add the terms together instead of subtracting them to get the total area.

Question 7.
Write one of the letters from your name using rectangles on the coordinate plane. Then, determine the area and perimeter. (For help see Exercise 2(b). This irregular polygon looks sort of like a T.)
Answer:

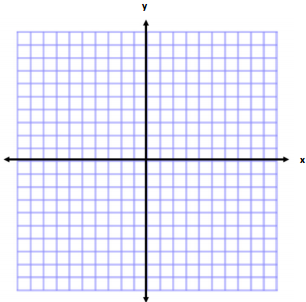
Answers will vary.

Eureka Math Grade 6 Module 5 Lesson 9 Exit Ticket Answer Key

Question 1.
Determine the area and perimeter of the figure below. Note that each square unit is 1 unit in length.

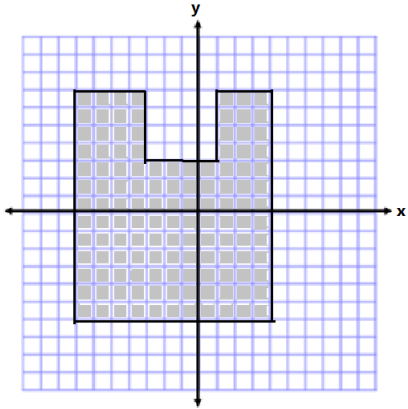
Answer:

Area:

Area of Large Rectangle
A = bh
A = (11 units)(13 units)
A = 143 units²

Area of Small Square
A = s²
A = (4units)²
A = 16 units²

Area of Irregular Shape
A = 143 units² – 16 units²
A = 127 units²

Perimeter = 13 units + 4 units + 4 units + 4 units + 4 units + 3 units + 13 units + 11 units
Perimeter = 56 units

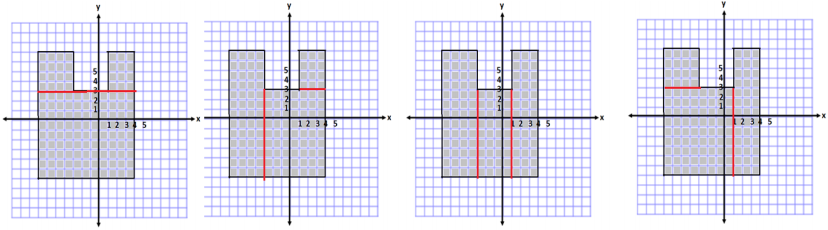
Other correct solutions might start with the following diagrams:

Eureka Math Grade 6 Module 5 Lesson 9 Addition and Subtraction Equations Answer Key

Addition and Subtraction Equations – Round 1:

Directions: Find the value of m in each equation.

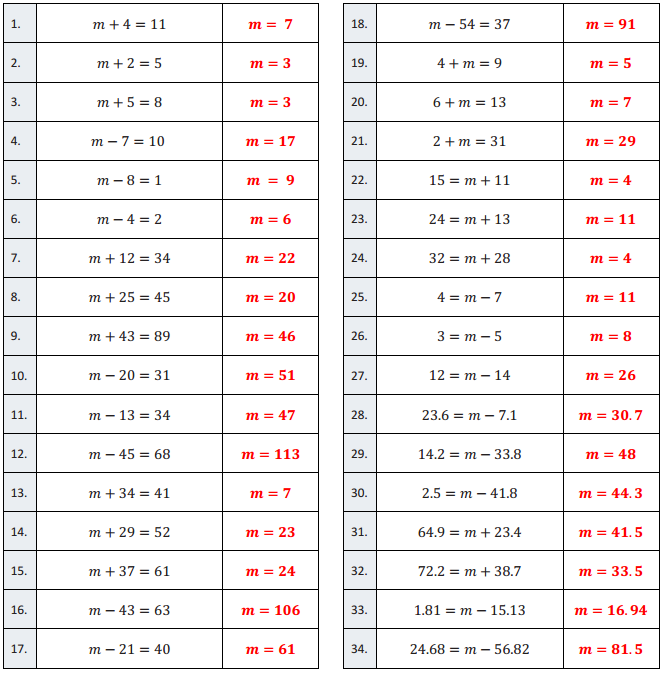
Question 1.
m + 4 = 11
Answer:
m = 7

Question 2.
m + 2 = 5
Answer:
m = 3

Question 3.
m + 5 = 8
Answer:
m = 3

Question 4.
m – 7 = 10
Answer:
m = 17

Question 5.
m – 8 = 1
Answer:
m = 9

Question 6.
m – 4 = 2
Answer:
m = 6

Question 7.
m + 12 = 34
Answer:
m = 22

Question 8.
m + 25 = 45
Answer:
m = 20

Question 9.
m + 43 = 89
Answer:
m = 46

Question 10.
m – 20 = 31
Answer:
m = 51

Question 11.
m – 13 = 34
Answer:
m = 47

Question 12.
m – 45 = 68
Answer:
m = 113

Question 13.
m + 34 = 41
Answer:
m = 7

Question 14.
m + 29 = 52
Answer:
m = 23

Question 15.
m + 37 = 61
Answer:
m = 24

Question 16.
m – 43 = 63
Answer:
m = 106

Question 17.
m – 21 = 40
Answer:
m = 61

Question 18.
m – 54 = 37
Answer:
m = 91

Question 19.
4 + m = 9
Answer:
m = 5

Question 20.
6 + m = 13
Answer:
m = 7

Question 21.
2 + m = 31
Answer:
m = 29

Question 22.
15 = m + 11
Answer:
m = 4

Question 23.
24 = m + 13
Answer:
m = 11

Question 24.
32 = m + 28
Answer:
m = 4

Question 25.
4 = m – 7
Answer:
m = 11

Question 26.
3 = m – 5
Answer:
m = 8

Question 27.
12 = m – 14
Answer:
m = 26

Question 28.
23.6 = m – 7.1
Answer:
m = 30.7

Question 29.
14.2 = m – 33.8
Answer:
m = 48

Question 30.
2.5 = m – 41.8
Answer:
m = 44.3

Question 31.
64.9 = m + 23.4
Answer:
m = 41.5

Question 32.
72.2 = m + 38.7
Answer:
m = 33.5

Question 33.
1.81 = m – 15.13
Answer:
m = 16.94

Question 34.
24.68 = m – 56.82
Answer:
m = 81.5

Addition and Subtraction Equations – Round 1:

Directions: Find the value of m in each equation.

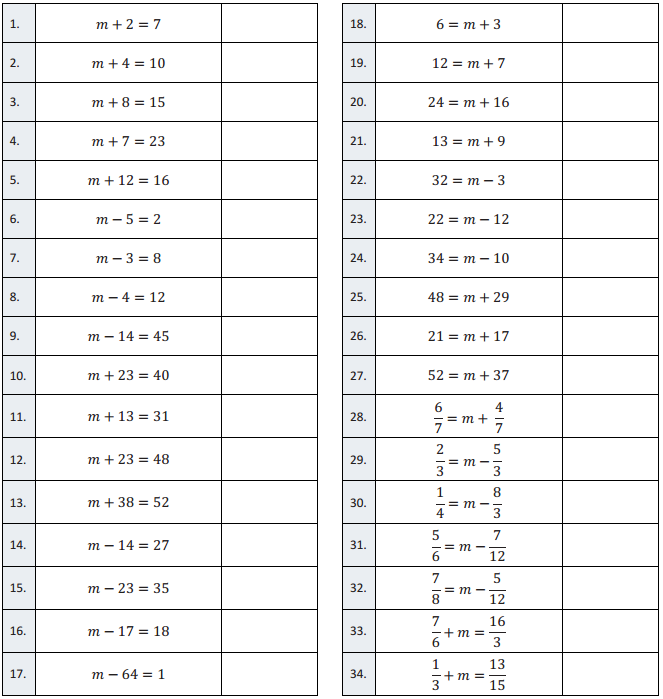
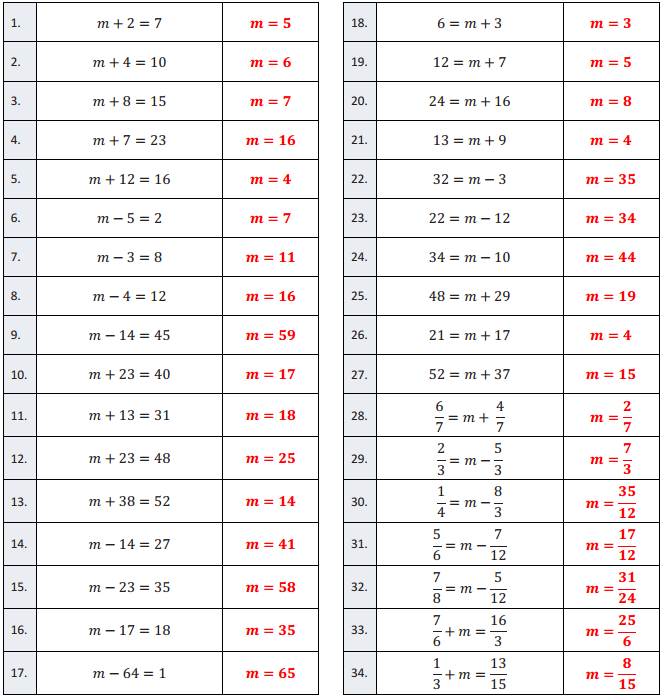
Question 1.
m + 2 = 7
Answer:
m = 5

Question 2.
m + 4 = 10
Answer:
m = 6

Question 3.
m + 8 = 15
Answer:
m = 7

Question 4.
m + 7 = 23
Answer:
m = 16

Question 5.
m + 12 = 16
Answer:
m = 4

Question 6.
m – 5 = 2
Answer:
m = 7

Question 7.
m – 3 = 8
Answer:
m = 11

Question 8.
m – 4 = 12
Answer:
m = 16

Question 9.
m – 14 = 45
Answer:
m = 59

Question 10.
m + 23 = 40
Answer:
m = 17

Question 11.
m + 13 = 31
Answer:
m = 18

Question 12.
m + 23 = 48
Answer: 25
m =

Question 13.
m + 38 = 52
Answer:
m = 14

Question 14.
m – 14 = 27
Answer:
m = 13

Question 15.
m – 23 = 35
Answer:
m = 12

Question 16.
m – 17 = 18
Answer:
m = 35

Question 17.
m – 64 = 1
Answer:
m = 65

Question 18.
6 = m + 3
Answer:
m = 3

Question 19.
12 = m + 7
Answer:
m = 5

Question 20.
24 = m + 16
Answer:
m = 8

Question 21.
13 = m + 9
Answer:
m = 4

Question 21.
32 = m – 3
Answer:
m = 35

Question 23.
22 = m – 12
Answer:
m = 34

Question 24.
34 = m – 10
Answer:
m = 44

Question 25.
48 = m + 29
Answer: 19

Question 26.
21 = m + 17
Answer:
m = 4

Question 27.
52 = m + 37
Answer:
m = 15

Question 28.
\(\frac{6}{7}\) = m + \(\frac{4}{7}\)
Answer:
m = \(\frac{2}{7}\)

Question 29.
\(\frac{2}{3}\) = m – \(\frac{5}{3}\)
Answer:
m = \(\frac{7}{3}\)

Question 30.
\(\frac{1}{4}\) = m – \(\frac{8}{3}\)
Answer:
m = \(\frac{35}{12}\)

Question 31.
\(\frac{5}{6}\) = m – \(\frac{7}{12}\)
Answer:
m = \(\frac{17}{12}\)

Question 32.
\(\frac{7}{8}\) = m – \(\frac{5}{12}\)
Answer:
m = \(\frac{31}{24}\)

Question 33.
\(\frac{7}{6}\) + m = \(\frac{16}{3}\)
Answer:
m = \(\frac{25}{6}\)

Question 34.
\(\frac{1}{3}\) + m = \(\frac{13}{15}\)
Answer:
m = \(\frac{8}{15}\)