Engage NY Eureka Math Grade 6 Module 5 Lesson 6 Answer Key
Eureka Math Grade 6 Module 5 Lesson 6 Exploratory Challenge Answer Key
Exploratory Challenge 1: Classroom Wall Paint
Question 1.
The custodians are considering painting our classroom next summer. In order to know how much paint they must buy, the custodians need to know the total surface area of the walls. Why do you think they need to know this, and how can we find the information?
Answer:
All classroom walls are different. Taking overall measurements and then subtracting windows, doors, or other areas will give a good approximation.
Question 2.
Make a prediction of how many square feet of painted surface there are on one wall in the room. If the floor has square tiles, these can be used as a guide.
Estimate the dimensions and the area. Predict the area before you measure. My prediction: _____ ft2.
a. Measure and sketch one classroom wall. Include measurements of windows, doors, or anything else that would not be painted.
Answer:
Student responses will depend on the teacher’s choice of wall.
b. Work with your partners and your sketch of the wall to determine the area that needs paint. Show your sketch and calculations below; clearly mark your measurements and area calculations.
Answer:
c. A gallon of paint covers about 350 ft2. Write an expression that shows the total area of the wall. Evaluate it to find how much paint is needed to paint the wall.
Answer:
Answers will vary based on the size of the wall. Fractional answers are to be expected.
d. How many gallons of paint would need to be purchased to paint the wall?
Answer:
Answers will vary based on the size of the wall. The answer from part (d) should be on exact quantity because gallons of paint are discrete units. Fractional answers from part (c) must be rounded up to the nearest whole gallon.
Exploratory Challenge 2:
Answer:
Eureka Math Grade 6 Module 5 Lesson 6 Problem Set Answer Key
Question 1.
Below is a drawing of a wall that is to be covered with either wallpaper or paint. The wall is 8 ft. high and 16 ft. wide. The window, mirror, and fireplace are not to be painted or papered. The window measures 18 in. wide and 14 ft. high. The fireplace is 5 ft. wide and 3 ft. high, while the mirror above the fireplace is 4 ft. wide and 2 ft. high. (Note: this drawing is not to scale.)
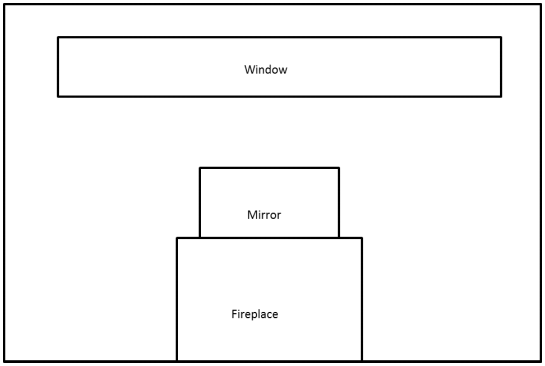
a. How many square feet of wallpaper are needed to cover the wall?
Answer:
Total wall area = 8 ft.× 16 ft. = 128 ft2
Window area = 14 ft.× 1.5 ft. = 21 ft2
Fireplace area= 3 ft.× 51t.= 15 ft2
Mirrorarea= 4 ft.× 2 ft.= 8 ft2
Net wall area to be covered 128 ft2 – (21 ft2 + 15 ft2 + 8 ft2) = 84 ft2
b. The wallpaper is sold in rolls that are 18 in. wide and 33 ft. long. Rolls of solid color wallpaper will be used, so patterns do not have to match up.
i. What is the area of one roll of wallpaper?
Answer:
Area of one roll of wallpaper: 33 ft. × 1.5 ft. = 49.5 ft2
ii. How many rolls would be needed to cover the wall?
Answer:
84 ft2 ÷ 49.5 ft2 ≈ 1.7; therefore, 2 rolls would need to be purchased.
c. This week, the rolls of wallpaper are on sale for $11. 99/roll. Find the cost of covering the wall with wallpaper.
Answer:
We need two rolls of wallpaper to cover the wall, which will cost $11.99 × 2 = $23.98.
d. A gallon of special textured paint covers 200 ft2 and is on sale for $22.99/gallon. The wall needs to be painted twice (the wall needs two coats of paint). Find the cost of using paint to cover the wall.
Answer:
Total wall area = 8 ft. × 16 ft. = 128 ft2
Windowarea = 14 ft. × 1.5 ft.= 21 ft2
Fireplace area = 3 ft.× 5 ft. = 15 ft2
Mirror area = 4 ft. × 2 ft. = 8 ft2
Net wall area to be covered 128 ft2 – (21 ft2 + 15 ft2 + 8 ft2) = 84 ft2
If the wall needs to be painted twice, we need to point a total area of 84 ft2 × 2 = 168 ft2. One gallon is enough paint for this wall, so the cost will be $22. 99.
Question 2.
A classroom has a length of 30 ft. and a width of 20 ft. The flooring is to be replaced by tiles. If each tile has a length of 36 in. and a width of 24 in., how many tiles are needed to cover the classroom floor?
Answer:
Area of the classroom: 30 ft. × 20 ft. = 600 ft2
Area of each tile 3 ft. × 2 ft. = 6 ft2
Area of the classroom \(\frac{\text { Area of the classroom }}{\text { Area of each tile }}=\frac{600 \mathrm{ft}^{2}}{6 \mathrm{ft}^{2}}\) = 100
100 tiles are needed to cover the classroom floor. Allow for students who say that if the tiles are 3 ft. × 2 ft., and they orient them in a way that corresponds to the 30 ft. × 20 ft. room, then they will have ten rows of ten tiles giving them 100 tiles.
Using this method, the students do not need to calculate the areas and divide. Orienting the
tiles the other way, students could say that they will need 105 tiles as they will need 6\(\frac{2}{3}\) rows of 15 tiles, and since \(\frac{2}{3}\) of a tile cannot be purchased, they will need 7 rows of 15 tiles.
Question 3.
Challenge: Assume that the tiles from Problem 2 are unavailable. Another design is available, but the tiles are square, 18 in. on a side. If these are to be installed, how many must be ordered?
Answer:
Solutions will vary. An even number of tiles fit on the 30 foot length of the room (20 tiles), but the width requires 13\(\frac{1}{3}\) tiles. This accounts for a 20 tile by 13 tile array. 20 × 13 = 260. 260 tiles need to be ordered.
The remaining area is 30 ft. × 0.5 ft. (20 × \(\frac{1}{3}\)) Since 20 of the \(\frac{1}{3}\) tiles are needed, 7 additional tiles must be cut to form \(\frac{21}{3}\). 20 of these will be used with \(\frac{1 {3}\) of 1 tile left over. Using the same logic as above, some students may correctly say they will need 280 tiles.
Question 4.
A rectangular flower bed measures 10 m by 6 m. It has a path 2 m wide around it. Find the area of the path.
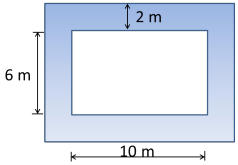
Answer:
Total Area = 14 m × 10 m = 140 m2
Flower bed area = 10 m × 6 m = 60 m2
Area of path: 140 m2 – 60 m2 = 80 m2
Question 5.
A diagram of Tracy’s deck is shown below, shaded blue. He wants to cover the missing portion of his deck with soil in order to grow a garden.
a. Find the area of the missing portion of the deck. Write the expression and evaluate it.
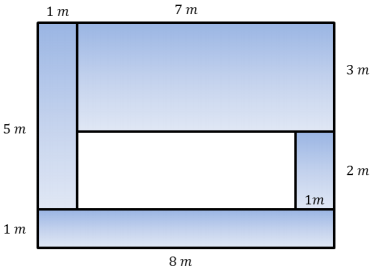
Answer:
Students should use one of two methods to find the area: finding the dimensions of the garden area (interior rectangle, 6 m𝐠× 2 m or finding the total area minus the sum of the four wooden areas, shown below.
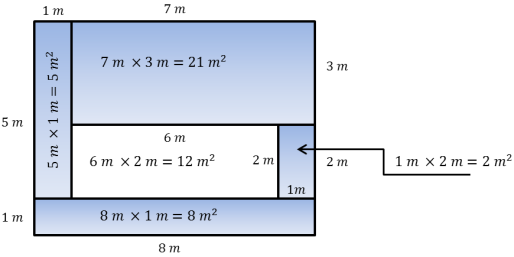
6 m × 2 m = 12 m2
8 × 6 – 7 × 3 – 5 × 1 – 8 × 1 – 2 × 1 = 12 (All linear units are in meters; area is in square metres.)
b. Find the area of the missing portion of the deck using a different method. Write the expression and evaluate it.
Answer:
Students should choose whichever method was not used in part (a).
c. Write two equivalent expressions that could be used to determine the area of the missing portion of the deck.
Answer:
8 × 6 – 7 × 3 – 5 × 1 – 8 × 1 – 2 × 1
6 × 2
d. Explain how each expression demonstrates a different understanding of the diagram.
Answer:
One expression shows the dimensions of the garden area (interior rectangle, 6 m × 2 m), and one shows finding the total area minus the sum of the four wooden areas.
Question 6.
The entire large rectangle below has an area of 3\(\frac{1}{2}\) ft2. If the dimensions of the white rectangle are as shown below, write and solve an equation to find the area, A, of the shaded region.
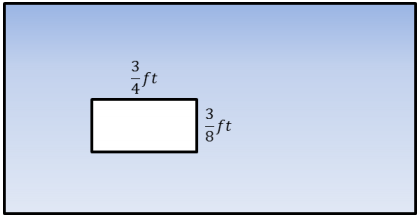
Answer:
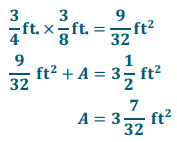
Eureka Math Grade 6 Module 5 Lesson 6 Exit Ticket Answer Key
Question 1.
Find the area of the deck around this pool. The deck is the white area in the diagram.
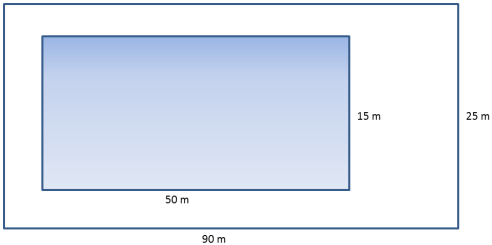
Answer:
Area of Walkway and Pool:
A = bh
A = 90 m × 25 m
A = 2,250 m2
Area of Pool:
A = bh
A = 50 m × 15 m
A = 750 m2
Area of Walkway:
2,250 m2 – 750 m2 = 1,500 m2