Engage NY Eureka Math Grade 6 Module 5 Lesson 18 Answer Key
Eureka Math Grade 6 Module 5 Lesson 18 Opening Exercise Answer Key
Opening Exercise:
a. What three-dimensional figure does the net create?
Answer:
Rectangular Prism
b. Measure (in inches) and label each side of the figure.
Answer:
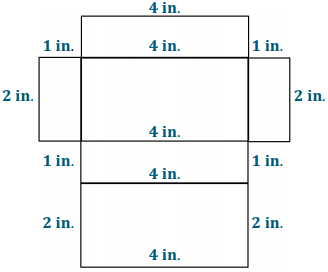
c. Calculate the area of each face, and record this value inside the corresponding rectangle.
Answer:
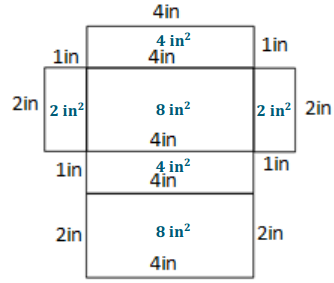
d. How did we compute the surface area of solid figures in previous lessons?
Answer:
To determine surface area, we found the area of each of the faces and then added those areas.
e. Write an expression to show how we can calculate the surface area of the figure above.
Answer:
(4 in. × 1 in.) + (4 in. × 2 in.) + (4 in. × 1 in.) + (4 in. × 2 in.) + (2 in. × 1 in.) + (2 in. × 1 in.) OR
2(4 in. × 1 in.) + 2(4 in. × 2 in.) + 2(2 in. × 1 in.)
f. What does each part of the expression represent?
Answer:
Each part of the expression represents an area of one face of the given figure. We were able to write a more compacted form because there are three pairs of two faces that are identical.
g. What is the surface area of the figure?
Answer:
(4in.× 1 in) + (4 in. × 2 in.) + (4 in. × 1 in.) + (4 in. × 2 in.) + (2 in. × 1 in.) + (2 in. × 1 in.)
2(4 in. × 1 in.) + 2(4 in. × 2 in.) + 2(2 in. × 1 in.)
28 in2
Eureka Math Grade 6 Module 5 Lesson 18 Example Answer Key
Example 1:
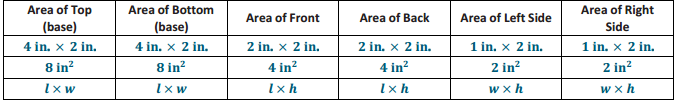
Fold the net used in the Opening Exercise to make a rectangular prism. Have the two faces with the largest area be the bases of the prism. Fill in the first row of the table below.
Answer:

→ What do you notice about the areas of the faces?
Pairs of faces have equal areas.
→ What is the relationship between the faces having equal area?
The faces that have the same area are across from each other. The bottom and top have the same area, the front and the back have the same area, and the two sides have the same area.
→ How do we calculate the area of the two bases of the prism?
length × width
→ How do we calculate the area of the front and back faces of the prism?
length × height
→ How do we calculate the area of the right and left faces of the prism?
width × height
→ Using the name of the dimensions, fill in the third row of the table.
Examine the rectangular prism below. Complete the table.
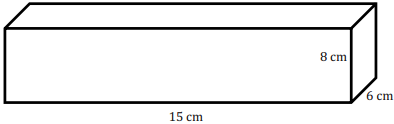
Answer:
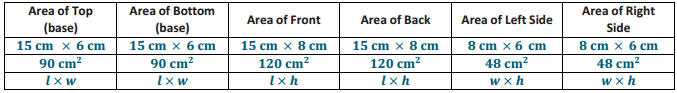
→ When comparing the methods to finding surface area of the two rectangular prisms, can you develop a general formula?
SA = l × w + l × w + l × h + l × h + w × h + w × h
→ Since we use the same expression to calculate the area of pairs of faces, we can use the distributive property to write an equivalent expression for the surface area of the figure that uses half as many terms.
→ We have determined that there are two l × w dimensions. Let’s record that as 2 times l times w, or simply 2(l × w). How can we use this knowledge to alter other parts of the formula?
We also have two l × h, so we can write that as 2(l × h), and we can write the two w × h as 2(w × h).
→ Writing each pair in a simpler way, what is the formula to calculate the surface area of a rectangular prism?
SA = 2(l × w) + 2(l × h) + 2(w × h)
→ Knowing the formula to calculate surface area makes it possible to calculate the surface area without a net.
Example 2:
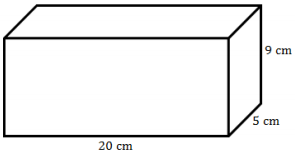
Answer:
→ What are the dimensions of the rectangular prism?
The length is 20 cm, the width is 5 cm, and the height is 9 cm.
→ We use substitution in order to calculate the area. Substitute the given dimensions into the surface area formula.
SA = 2(20 cm)(5 cm) + 2(20 cm)(9 cm) + 2(5 cm)(9 cm)
→ Solve the equation. Remember to use order of operations.
SA = 200cm2 + 360 cm2 + 90 cm2
SA = 650 cm2
Eureka Math Grade 6 Module 5 Lesson 18 Exercise Answer Key
Exercises 1 – 3:
Exercise 1.
Calculate the surface area of each of the rectangular prisms below.
a. 
Answer:
SA = 2(12 in.)(2 in.) + 2(12 in.)(3 in.) + 2(2 in.)(3 in.)
SA = 48 in2 + 72 in2 + 12 in2
SA = 132 in2
b. 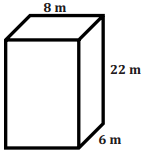
Answer:
SA = 2(8 m)(6 m) + 2(8 m)(22 m) + 2(6 m)(22 m)
SA = 96 m2 + 352 m2 + 264 m2
SA = 712 m2
c. 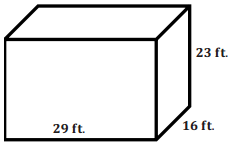
Answer:
SA = 2(29 ft. ) (16 ft.) + 2(29 ft.) (23 ft.) + 2(16 ft.) (23 ft.)
SA = 928 ft2 + 1,334 ft2 + 736 ft2
SA = 2,998 ft2
d. 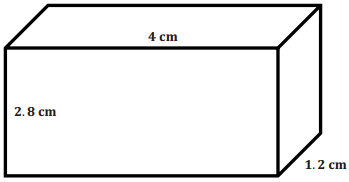
Answer:
SA = 2(4 cm) (1.2 cm) + 2(4 cm) (2.8 cm) + 2(1.2 cm) (2.8 cm)
SA = 9.6 cm2 + 22.4 cm2 + 6.72 cm2
SA = 38.72 cm2
Exercise 2.
Calculate the surface area of the cube.
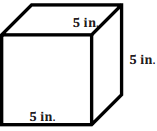
Answer:
SA = 2(5 in.) (5 in.) + 2(5 in.) (5 in.) + 2(5 in.) (5 in.)
SA = 50 in2 + 50 in2 + 50 in2
SA = 150 in2
Exercise 3.
All the edges of a cube have the same length. Tony claims that the formula SA = 6s2, where s is the length of each side of the cube, can be used to calculate the surface area of a cube.
a. Use the dimensions from the cube in Problem 2 to determine if Tony’s formula is correct.
Answer:
Tony’s formula is correct because SA = 6(5 km)2 = 150 km2, which is the same surface area when we use the surface area formula for rectangular prisms.
b. Why does this formula work for cubes?
Answer:
Each face is a square, and to find the area of a square, you multiply the side lengths together. However, since the side lengths are the same, you can just square the side length. Also, a cube has 6 identical faces, so after calculating the area of one face, we can just multiply this area by 6 to determine the total surface area of the cube.
c. Becca does not want to try to remember two formulas for surface area, so she is only going to remember the formula for a cube. Is this a good idea? Why or why not?
Answer:
Becca’s idea is not a good idea. The surface area formula for cubes only works for cubes because rectangular prisms do not have 6 identical faces. Therefore, Becca also needs to know the surface area formula for rectangular prisms.
Eureka Math Grade 6 Module 5 Lesson 18 Problem Set Answer Key
Calculate the surface area of each figure below. Figures are not drawn to scale.
Question 1.
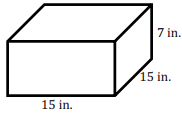
Answer:
SA = 2(15 in.)(15 in.) + 2(15 in.)(7 in.) + 2(15 in.)(7 in.)
SA = 450 in2 + 210 in2 + 210 in2
SA = 870 in2
Question 2.
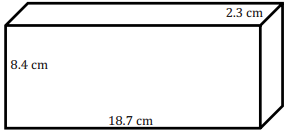
Answer:
SA = 2(18.7 cm) (2. 3 cm) + 2(18.7 cm) (8. 4cm) + 2(2.3 cm) (8. 4 cm)
SA = 86.02 cm2 + 314. 16 cm2 + 38.64 cm2
SA = 438.82 cm2
Question 3.
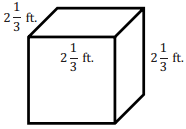
Answer:
SA = 6(2\(\frac{1}{3}\) ft.)2
SA = 6(\(\frac{7}{3}\) ft.)2
SA = 6(\(\frac{49}{9}\) ft.)2
SA = \(\frac{294}{9}\) ft2 = 32\(\frac{2}{3}\) ft2
Question 4.
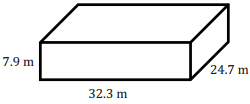
Answer:
SA = 2(32.3 m) (24.7 m) + 2(32.3 m) (7.9 m) + 2(24.7 m) (7.9 m)
SA= 1,595.62 m2 + 510.34 m2 + 390.26 m2
SA = 2,496.22 m2
Question 5.
Write a numerical expression to show how to calculate the surface area of the rectangular prism. Explain each part of the expression.
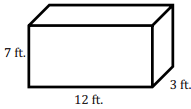
Answer:
2(12 ft. × 3 ft.) + 2(12 ft. × 7 ft.) + 2(7 ft. × 3 ft.)
The first part of the expression shows the area of the top and bottom of the rectangular prism. The second part of the expression shows the area of the front and back of the 7 ft. rectangular prism. The third part of the expressin shows the area of the two sides of the rectangular prism. 3 ft. The surface area of the figure is 282 ft2.
Question 6.
When Louie was calculating the surface area for Problem 4, he identified the following:
length = 24. 7 m, width = 32.3 m, and height = 7.9 m.
However, when Rocko was calculating the surface area for the same problem, he identified the following:
length = 32.3 m, width = 24.7 m, and height = 7.9 m.
Would Louie and Rocko get the same answer? Why or why not?
Answer:
Louie and Rocko would get the same answer because they are still finding the correct area of all six faces of the rectangular prism.
Eureka Math Grade 6 Module 5 Lesson 18 Exit Ticket Answer Key
Calculate the surface area of each figure below. Figures are not drawn to scale.
Question 1.
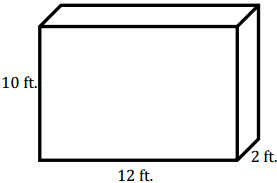
Answer:
SA = 2lw + 2lh + 2wh
SA = 2(12 ft.) (2 ft.) + 2(12 ft ) (10 ft.) + 2(2 ft) (10 ft.)
SA = 48 ft2 + 240 ft2 + 40 ft2
SA = 328 ft2
Question 2.
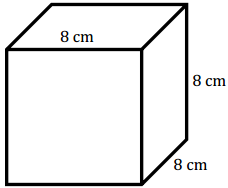
Answer:
SA = 6S2
SA = 6(8 cm)2
SA = 6(64 cm2)
SA = 384 cm2