Engage NY Eureka Math Grade 6 Module 4 Lesson 29 Answer Key
Eureka Math Grade 6 Module 4 Lesson 29 Example Answer Key
Example:
The school librarian, Mr. Marker, knows the library has 1,400 books but wants to reorganize how the books are displayed on the shelves. Mr. Marker needs to know how many fiction, nonfiction, and resource books are in the library. He knows that the library has four times as many fiction books as resource books and half as many nonfiction books as fiction books. If these are the only types of books in the library, how many of each type of boo are in the library?
Draw a tape diagram to represent the total number of books in the library.
Answer:
![]()
Draw two more tape diagrams, one to represent the number of fiction books in the library and one to represent the number of resource books in the library.
Answer:
What variable should we use throughout the problem?
Answer:
We should user to represent the number of resource books in the library because it represents the fewest amount of books. Choosing the variable to represent a different type of book would create fractions throughout the problem.
Write the relationship between resource books and fiction books algebraically.
Answer:
If we let r represent the number resource books, then 4r represents the number of fiction books.
Draw a tape diagram to represent the number of nonfiction books.
Answer:
![]()
How did you decide how many sections this tape diagram would have?
Answer:
There are half as many nonfiction books as fiction books. Since the fiction book tape diagram has four sections, the nonfiction book tape diagram should have two sections.
Represent the number of nonfiction books in the library algebraically.
Answer:
2r because that is half as many as fiction books.
Use the tape diagrams we drew to solve the problem.
Answer:
We know that combining the tape diagrams for each type of book will leave us with 1,400 total books.

Write an equation that represents the tape diagram.
Answer:
4r + 2r + r = 1,400
Determine the value of r.
Answer:
We can gather like terms and then solve the equation.
7r = 1,400
7r + 7 = 1,400 + 7
r = 200
→ What does this 200 mean?
There are 200 resource books in the library because r represented the number of resource books.
How many fiction books are in the library?
Answer:
There are 800 fiction books in the library because 4(200) = 800.
How many nonfiction books are in the library?
Answer:
There are 400 nonfiction books in the library because 2(200) = 400.
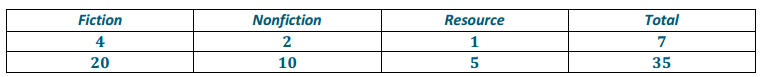
→ We can use a different math tool to solve the problem as well. If we were to make a table, how many columns would we need?
4
→ Why do we need four columns?
We need to keep track of the number of fiction, nonfiction, and resource books that are in the library, but we also need to keep track of the total number of books.
Set up a table with four columns, and label each column.
Answer:
![]()
→ Highlight the important information from the word problem that will help us fill out the second row in our table.
The school librarian, Mr. Marker, knows the library has 1,400 books but wants to reorganize how the books are displayed on the shelves. Mr. Marker needs to know how many fiction, nonfiction, and resource books are in the library. He knows that the library has four times as many fiction books as resource books and half as many nonfiction books as fiction books. If these are the only types of books in the library, how many of each type of book are in the library?
→ Fill out the second row of the table using the algebraic representations.

→ If r = 1, how many of each type of book would be in the library?

→ How can we fill out another row of the table?
Substitute different values in for r.
→ Substitute 5 in for r. How many of each type of book would be in the library then?
→ Does the library have four times as many fiction books as resource books?
Yes, because 5 . 4 = 20.
→ Does the library have half as many nonfiction books as fiction books?
Yes, because half of 20 is 10.
→ How do we determine how many of each type of book is in the library when there are 1,400 books in the library?
Continue to multiply the rows by the same value, until the total column has 1400 books.
At this point, allow students to work individually to determine how many fiction, nonfiction, and resource books are in the library if there are 1,400 total books. Each table may look different because students may choose different values to multiply by. A sample answer is shown below.
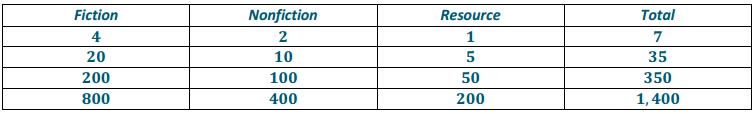
How many fiction books are in the library?
Answer:
800
How many nonfiction books are in the library?
Answer:
400
How many resource books are in the library?
Answer:
200
Does the library have four times as many fiction books as resource books?
Answer:
Yes, because 200 . 4 = 800.
Does the library have half as many nonfiction books as fiction books?
Answer:
Yes, because half of 800 is 400.
Does the library have 1,400 books?
Answer:
Yes, because 800 + 400 + 200 = 1400.
Eureka Math Grade 6 Module 4 Lesson 29 Exercise Answer Key
Exercises:
Solve each problem below using tables and algebraic methods. Then, check your answers with the word problems.
Exercise 1.
Indiana Ridge Middle School wanted to add a new school sport, so they surveyed the students to determine which sport is most popular. Students were able to choose among soccer, football, lacrosse, or swimming. The same number of students chose lacrosse and swimming. The number of students who chose soccer was double the number of students who chose lacrosse. The number of students who chose football was triple the number of students who chose swimming. If 434 students completed the survey, how many students chose each sport?
Answer:

The rest of the table will vary.

124 students chose soccer, 186 students chose football, 62 students chose lacrosse, and 62 students chose swimming.
We can confirm that these numbers satisfy the conditions of the word problem because lacrosse and swimming were chosen by the same number of students. 124 is double 62, so soccer was chosen by double the number of students as lacrosse, and 186 is triple 62, so football was chosen by 3 times as many students as swimming. Also,
124 + 186 + 62 + 62 = 434.
Algebraically: Let s represent the number of students who chose swimming. Then, 2s is the number of students who chose soccer, 3s is the number of students who chose football, and s is the number of students who chose lacrosse.
2s + 3s + s + s = 434
7s = 434
7s ÷ 7 = 434 ÷ 7
s = 62
Therefore, 62 students chose swimming, and 62 students chose lacrosse. 124 students chose soccer because 2(62) = 124, and 186 students chose football because 3(62) = 186.
Exercise 2.
At Prairie Elementary School, students are asked to pick their lunch ahead of time so the kitchen staff will know what to prepare. On Monday, 6 times as many students chose hamburgers as chose salads. The number of students who chose lasagna was one third the number of students who chose hamburgers. If 225 students ordered lunch, how many students chose each option if hamburger, salad, and lasagna were the only three options?
Answer:

The rest of the table will vary.

150 students chose a hamburger for lunch, 25 students chose a salad, and 50 students chose lasagna.
We can confirm that these numbers satisfy the conditions of the word problem because 25 . 6 = 150, so hamburgers were chosen by 6 times more students than salads. Also, \(\frac{1}{3}\) . 150 = 50, which means lasagna was chosen by one third of the number of students who chose hamburgers. Finally, 150 + 25 + 50 = 225, which means 225 students completed the survey.
Algebraically: Let s represent the number of students who chose a salad. Then, 6s represents the number of students who chose hamburgers, and 2s represents the number of students who chose lasagna.
6s + s + 2s = 225
9s = 225
9s ÷ 9 = 225 ÷ 9s
s = 25
This means that 25 students chose salad, 150 students chose hamburgers because 6(25) = 150, and 50 students chose lasagna because 2(25) = 50.
Exercise 3.
The art teacher, Mr. Gonzalez, is preparing for a project. In order for students to have the correct supplies, Mr. Gonzalez needs 10 times more markers than pieces of construction paper. He needs the same number of bottles of glue as pieces of construction paper. The number of scissors required for the project is half the number of pieces of construction paper. If Mr. Gonzalez collected 400 items for the project, how many of each supp’y did he collect?
Answer:
![]()
The rest of the table will vary.

Mr. Gonzalez collected 320 markers, 32 pieces of construction paper, 32 glue bottles, and 16 scissors for the project.
We can confirm that these numbers satisfy the conditions of the word problem because Mr. Gonzalez collected the same number of pieces of construction paper and glue bottles. Also, 32 . 10 = 320, so Mr. Gonzalez collected 10 times more markers than pieces of construction paper and glue bottles. Mr. Gonzalez only collected 16 pairs of scissors, which is half of the number of pieces of construction paper. The supplies collected add up to 400 supplies, which is the number of supplies indicated in the word problem.
Algebraically: Let s represent the number of scissors needed for the project, which means 20s represents the number of markers needed, 2s represents the number of pieces of construction paper needed, and 2s represents the number of glue bottles needed.
20s + 2s + 2s + s = 400
25s = 400
\(\frac{25 s}{25}=\frac{400}{25}\)
s = 16
This means that 16 pairs of scissors, 320 markers, 32 pieces of construction paper, and 32 glue bottles are required
for the project.
Exercise 4.
The math teacher, Ms. Zentz, is buying appropriate math tools to use throughout the year. She is planning on buying twice as many rulers as protractors. The number of calculators Ms. Zentz Is planning on buying is one quarter of the number of protractors. If Ms. Zentz buys 65 Items, how many protractors does Ms. Zentz buy?
Answer:

The rest of the table will vary.

Ms. Zentz will buy 20 protractors.
We can confirm that this number satisfies the conditions of the word problem because the number of protractors is half of the number of rulers, and the number of calculators is one fourth of the number of protractors. Also, 40 + 20 + 5 = 65, so the total matches the total supplies that Ms. Zentz bought.
Algebraically: Let c represent the number of calculators Ms. Zentz needs for the year. Then, 8c represents the number of rulers, and 4c represents the number of protractors Ms. Zentz will need throughout the year.
8c + 4c + c = 65
13c = 65
\(\frac{13 c}{13}=\frac{65}{13}\)
c = 5
Therefore, Ms. Zentz will need 5 calculators, 40 rulers, and 20 protractors throughout the year.
Eureka Math Grade 6 Module 4 Lesson 29 Problem Set Answer Key
Create tables to solve the problems, and then check your answers with the word problems.
Question 1.
On average, a baby uses three times the number of large diapers as small diapers and double the number of medium diapers as small diapers.
a. If the average baby uses 2,940 diapers, size large and small, how many of each size would be used?
Answer:
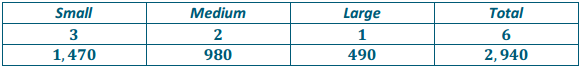
An average baby would use 490 small diapers, 980 medium diapers, and 1,470 large diapers.
The answer makes sense because the number of large diapers is 3 times more than small diapers. The number of medium diapers is double the number of small diapers, and the total number of diapers is 2, 940.
b. Support your answer with equations.
Answer:
Let s represent the number of small diapers a baby needs. Therefore, 2s represents the number of medium diapers, and 3s represents the amount of large diapers a baby needs.
s + 2s + 3s = 2,940
6s = 2,940
\(\frac{6 s}{6}=\frac{2,940}{6}\)
s = 490
Therefore, a baby requires 490 small diapers, 980 medium diapers (because 2(490) = 980), and 1,470 large diapers (because 3(490) = 1470), which matches the answer in part (a).
Question 2.
Tom has three times as many pencils as pens but has a total of 100 writing utensils.
a. How many pencils does Tom have?
Answer:
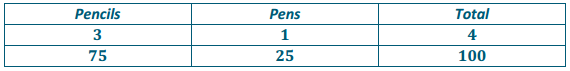
b. How many more pencils than pens does Tom have?
Answer:
75 – 25 = 50. Tom has 50 more pencils than pens.
Question 3.
Serena’s mom is planning her birthday party. She bought balloons, plates, and cups. Serena’s mom bought twice as many plates as cups. The number of balloons Serena’s mom bought was half the number of cups.
a. If Serena’s mom bought 84 items, how many of each item did she buy?
Answer:
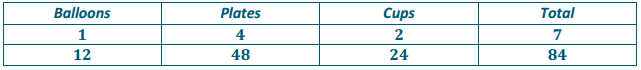
Serena’s mom bought 12 balloons, 48 plates, and 24 cups.
b. Tammy brought 12 balloons to the party. How many total balloons were at Serena’s birthday party?
Answer:
12 + 12 = 24. There were 24 total balloons at the party.
c. If half the plates and all but four cups were used during the party, how many plates and cups were used?
Answer:
\(\frac{1}{2}\) . 48 = 24. Twenty-four plates were used during the party.
24 – 4 = 20. Twenty cups were used during the party.
Question 4.
Elizabeth has a lot of jewelry. She has four times as many earrings as watches but half the number of necklaces as earrings. Elizabeth has the same number of necklaces as bracelets.
a. If Elizabeth has 117 pieces of jewelry, how many earrings does she have?
Answer:
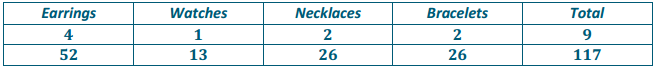
Elizabeth has 52 earrings, 13 watches, 26 necklaces, and 26 bracelets.
b. Support your answer with an equation.
Answer:
Let w represent the number of watches Elizabeth has. Therefore, 4w represents the number of earrings Elizabeth has, and 2w represents both the number of necklaces and bracelets she has.
4w + w + 2w + 2w = 117
9w = 117
\(\frac{9 w}{9}=\frac{117}{9}\)
w = 13
Therefore, Elizabeth has 13 watches, 52 earrings because 4(13) = 52, and 26 necklaces and bracelets each because 2(13) = 26.
Question 5.
Claudia was cooking breakfast for her entire family. She made double the amount of chocolate chip pancakes as she did regular pancakes. She only made half as many blueberry pancakes as she did regular pancakes. Claudia also knows her family loves sausage, so she made triple the amount of sausage as blueberry pancakes.
a. How many of each breakfast item did Claudia make If she cooked 90 Items in total?
Answer:
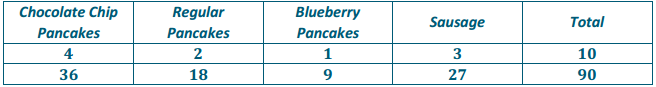
Claudia cooked 36 chocolate chip pancakes, 18 regular pancakes, 9 blueberry pancakes, and 27 pieces of sausage.
b. After everyone ate breakfast, there were 4 chocolate chip pancakes, 5 regular pancakes, 1 blueberry pancake, and no sausage left. How many of each item did the family eat?
Answer:
The family ate 32 chocolate chip pancakes, 13 regular pancakes, 8 blueberry pancakes, and 27 pieces of sausage during breakfast.
Question 6.
During a basketball game, Jeremy scored triple the number of points as Donovan. Kolby scored double the number of points as Donovan.

a. If the three boys scored 36 points, how many points did each boy score?
Answer:
Jeremy scored 18 points, Donovan scored 6 points, and Kolby scored 12 points.
b. Support your answer with an equation.
Answer:
Let d represent the number of points Donovan scored, which means 3d represents the number of points Jeremy scored, and 2d represents the number of points Kolby scored.
3d + d + 2d = 36
6d = 36
\(\frac{6 d}{6}=\frac{36}{6}\)
d = 6
Therefore, Donovan scored 6 points, Jeremy scored 18 points because 3(6) = 18, and Kolby scored 12 points because 2(6) = 12
Eureka Math Grade 6 Module 4 Lesson 29 Exit Ticket Answer Key
Solve the problem using tables and equations, and then check your answer with the word problem. Try to find the answer only using two rows of numbers on your table.
Question 1.
A pet store owner, Byron, needs to determine how much food he needs to feed the animals. Byron knows that he needs to order the same amount of bird food as hamster food. He needs four times as much dog food as bird food and needs half the amount of cat food as dog food. If Byron orders 600 packages of animal food, how much dog food does he buy? Let b represent the number of packages of bird food Byron purchased for the pet store.
Answer:

The rest of the table will vary (unless they follow suggestions from the Closing).

Byron would need to order 300 packages of dog food.
The answer makes sense because Byron ordered the same amount of bird food and hamster food. The table also shows that Byron ordered four times as much dog food as bird food, and the amount of cat food he ordered Is half the amount of dog food. The total amount of pet food Byron ordered was 600 packages, which matches the word problem.
Algebraically: Let b represent the number of packages of bird food Byron purchased for the pet store. Therefore, b also represents the amount of hamster food, 4b represents the amount of dog food, and 2b represents the amount of cat food required by the pet store.
b + b + 4b + 2b = 600
8b = 600
8b ÷ 8 = 600 ÷ 8
b = 75
Therefore, Byron will order 75 pounds of bird food, which results in 300 pounds of dog food because 4(75) = 300.