Engage NY Eureka Math 6th Grade Module 2 Lesson 19 Answer Key
Eureka Math Grade 6 Module 2 Lesson 19 Opening Exercise Answer Key
Euclid’s algorithm is used to find the greatest common factor (GCF) of two whole numbers.
1. Divide the larger of the two numbers by the smaller one.
2. If there is a remainder, divide it into the divisor.
3. Continue dividing the last divisor by the last remainder until the remainder is zero.
4. The final divisor is the GCF of the original pair of numbers.
383 ÷ 4 =
Answer:
95.75
432 ÷ 12 =
Answer:
36
403 ÷ 13 =
Answer:
31
Eureka Math Grade 6 Module 2 Lesson 19 Example Answer Key
Example 1:
Euclid’s Algorithm Conceptualized
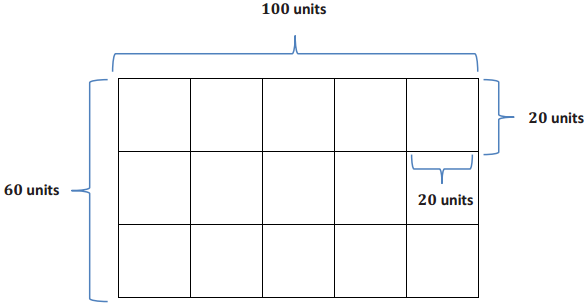
Answer:
→ Notice that we can use the GCF of 20 to create the largest square tile that covers the rectangle without any overlap or gaps. We used a 20 × 20 tile.
→ But, what if we did not know that? We could start by guessing. What is the biggest square tile that we can guess?
60 × 60
Display the following diagram:
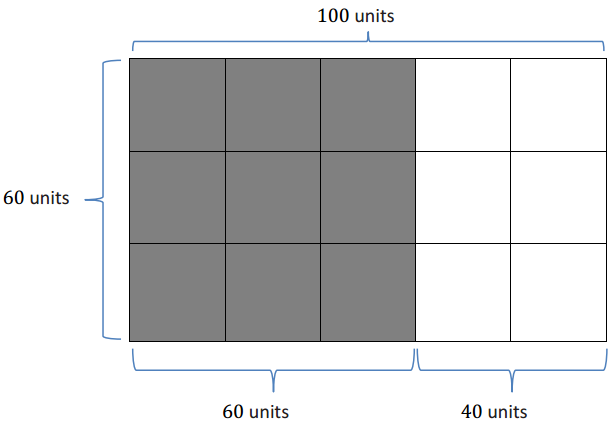
→ It fits, but there are 40 units left over. Do the division problem to prove this.
→ What is the leftover area?
60 × 40
→ What is the largest square tile that we can fit in the leftover area?
40 × 40
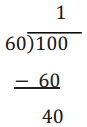
Display the following diagram:
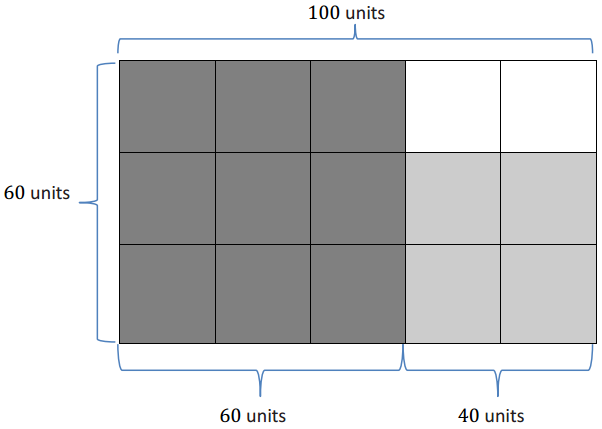
→ What is the leftover area?
20 × 40
→ What is the largest square tile that we can fit in the leftover area? 20)40
20 × 20
→ When we divide 40 by 20, there is no remainder. So, we have tiled the entire rectangle.
→ If we had started tiling the whole rectangle with squares, the largest square we could have used would be 20 by 20.
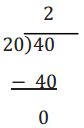
Example 2.
a. Let’s apply Euclid’s algorithm to some of the problems from our last lesson.
i. What is the GCF of 30 and 50?
Answer:
10
ii. Using Euclid’s algorithm, we follow the steps that are listed In the Opening Exercise.
Answer:
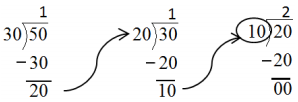
When the remainder is zero, the final divisor is the GCF.
b. Apply Euclid’s algorithm to find the GCF (30, 45).
Answer:
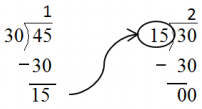
15
Example 3.
Larger Numbers
GCF (96, 144)
Answer:
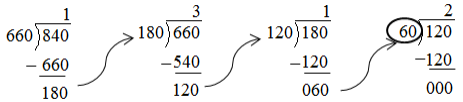
Example 4:
Area Problems
The greatest common factor has many uses. Among them, the GCF lets us find out the maximum size of squares that cover a rectangle. When we solve problems like this, we cannot have any gaps or any overlapping squares. Of course, the maximum size squares is the minimum number of squares needed.
A rectangular computer table measures 30 inches by 50 inches. We need to cover it with square tiles. What is the side length of the largest square tile we can use to completely cover the table without overlap or gaps?
Answer:

a. If we use squares that are 10 by 10, how many do we need?
Answer:
3 . 5, or 15 squares
b. If this were a giant chunk of cheese ¡n a factory, would ¡t change the thinking or the calculations we just did?
Answer:
No
c. How many 10 inch × 10 inch squares of cheese could be cut from the giant 30 inch × 50 inch slab?
Answer:
15
Eureka Math Grade 6 Module 2 Lesson 19 Problem Set Answer Key
Question 1.
Use Euclid’s algorithm to find the greatest common factor of the following pairs of numbers:
a. GCF(12, 78)
Answer:
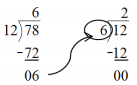
GCF (12, 78) = 6
b. GCF(18, 176)
Answer:
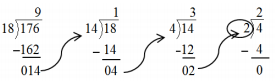
GCF (18, 176) = 2
Question 2.
Juanita and Samuel are planning a pizza party. They order a rectangular sheet pizza that measures 21 inches by 36 inches. They tell the pizza maker not to cut it because they want to cut it themselves.
a. All pieces of pizza must be square with none left over. What is the side length of the largest square pieces into which Juanita and Samuel can cut the pizza?
Answer:
GCF (21, 36) = 3 They can cut the pizza into 3 inch by 3 inch squares.
b. How many pieces of this size can be cut?
Answer:
7. 12 = 84 Juanita and Samuel can cut 84 pieces.
Question 3.
Shelly and Mickelle are making a quilt. They have a piece of fabric that measures 48 inches by 168 inches.
a. All pieces of fabric must be square with none left over. What is the side length of the largest square pieces into which Shelly and Mickelle can cut the fabric?
Answer:
GCF (48, 168) = 24
b. How many pieces of this size can Shelly and Mickelle cut?
Answer:
2 . 7 = 14 They can cut 14 pieces.
Eureka Math Grade 6 Module 2 Lesson 19 Exit Ticket Answer Key
Question 1.
Use Euclid’s algorithm to find the greatest common factor of 45 and 75.
Answer:
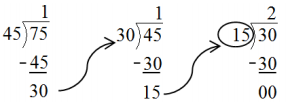
GCF (45, 75) = 15