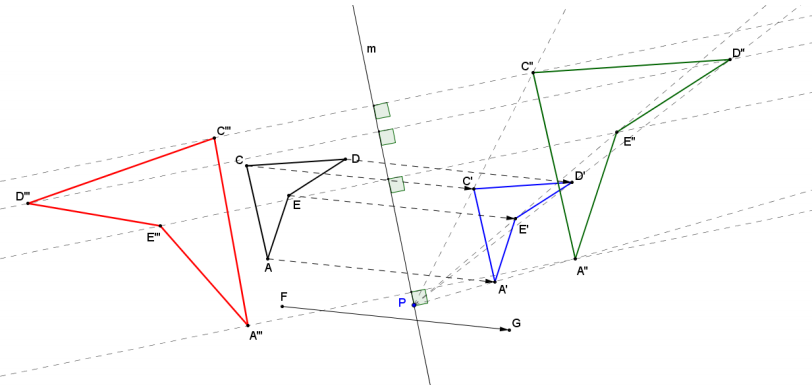
Engage NY Eureka Math Geometry Module 2 Lesson 13 Answer Key
Eureka Math Geometry Module 2 Lesson 13 Example Answer Key
Example 1.
Similarity transformation G consists of a rotation about the point P by 90°, followed by a dilation centered at P with scale factor of r = 2, and then followed by a reflection across line f. Find the image of the triangle.
Answer:
→ Before getting to the actual construction process, draw a predictive sketch of the image of the triangle after transformation. You should have three sketches.
Drawing a predictive sketch helps illuminate the path ahead before getting into the details of each construction. The sketches also provide a time to reflect on how the properties are true for each transformation. For example, ask students to select a property from their lists and describe where in their sketches they see it in the transformation. For instance, a student might select property (2), which states that each point has a pre-image P and can point to each pre-image and image with each passing transformation.
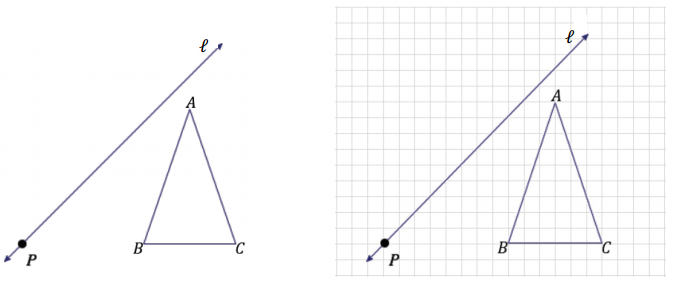
Complete this example with students. Remind them that the rotation requires all three geometry tools.
Note to the teacher: As an alternative strategy, consider using coordinate geometry by placing the image over an appropriately scaled grid. Students gained familiarity with coordinate geometry as used with transformations of the plane in Grade 8. The image on the right above has been provided for this alternative. Placement of the x- and y-axes can be determined where convenient for this example. Given this flexibility, most students likely choose point P to be the origin of the coordinate plane since two of the given transformations are centered at P.
→ How will we rotate the triangle 90°?
To locate the vertices of the triangle’s image, draw rays through each vertex of the triangle originating from P. Use each ray to form three 90° angles, and then use the compass to locate each new vertex on the corresponding ray.
Point out to students that in their constructions, the ray through B’ and C’ coincides and appears to be one ray, giving an appearance of only two rays, not three.
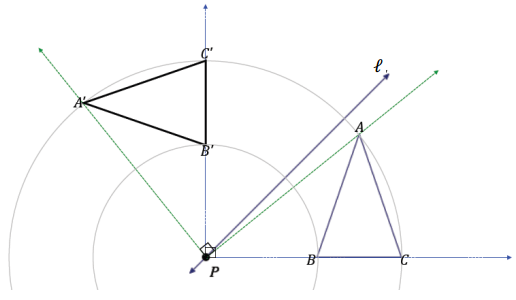
Next, allow students time to dilate ∆ A’B’C’. If necessary, review the steps to create a dilation.
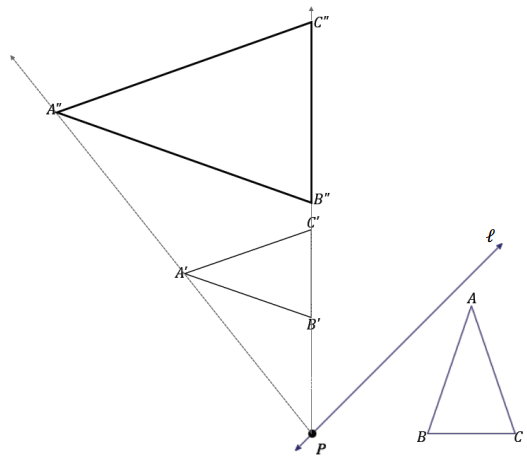
→ How will we reflect the triangle over the line?
Create the construction marks that determine the image of each vertex so that the line of reflection is the perpendicular bisector of the segment that joins each vertex with its image. In other words, we must create the construction marks so that the images of the vertices are located so that the line of reflection is the perpendicular bisector of \(\overline{A^{\prime \prime} A^{\prime \prime \prime}}, \overline{B^{\prime \prime} B^{\prime \prime \prime}}, \overline{C^{\prime \prime} C^{\prime \prime \prime}}\).
The steps to determine B” are shown below.
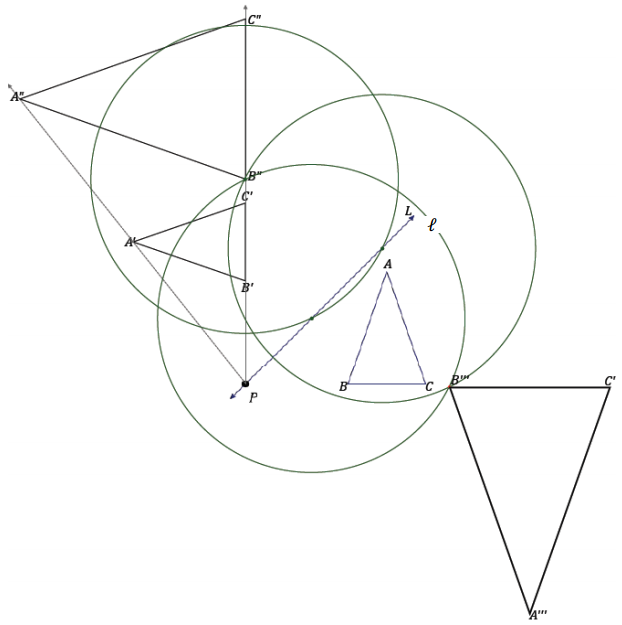
→ We have applied the outlined similarity transformation to ∆ ABC and found its image, that is, the similar figure ∆ A”’B”’C”’
→ Since G comprises three individual transformations and each of the transformations satisfies the known properties, we know that G also satisfies the properties.
Example 2.
A similarity transformation G applied to trapezoid ABCD consists of a translation by vector \(\overrightarrow{X Y}\), followed by a reflection across line m, and then followed by a dilation centered at P with a scale factor of r = 2. Recall that we can describe the same sequence using the following notation: Dp,2 (rm(Txy(ABCD))). Find the image of ABCD.
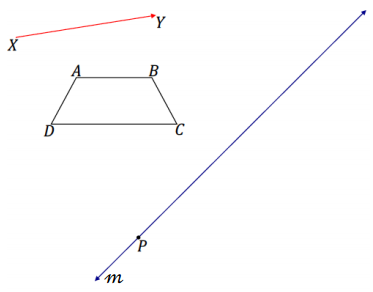
Answer:
Encourage students to draw a predictive sketch for each stage of the transformation before beginning the construction.
→ Describe the steps to apply the translation by vector XY to one point of the figure.
To apply the translation to A, construct C1: center A, radius XY. Then, construct C2: center Y, radius XA.
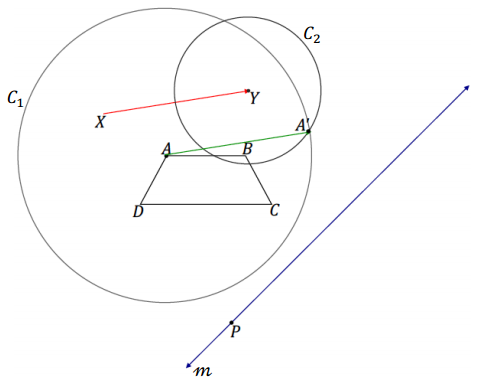
The process for locating the image of B under the translation is shown below:
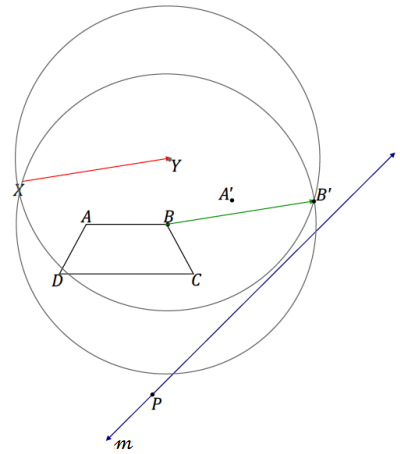
Allow students time to complete the rest of the example before reviewing it.
The following image shows the reflection of vertex C’:
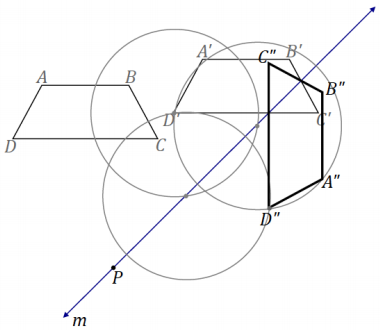
The following image shows the dilation of A”B”C”D”:
Eureka Math Geometry Module 2 Lesson 13 Exercise Answer Key
Exercise 1.
A similarity transformation for triangle DEF is described by rn(DA,\(\frac{1}{2}\) (RA,90°(DEF))). Locate and label the image of triangle DEF under the similarity.
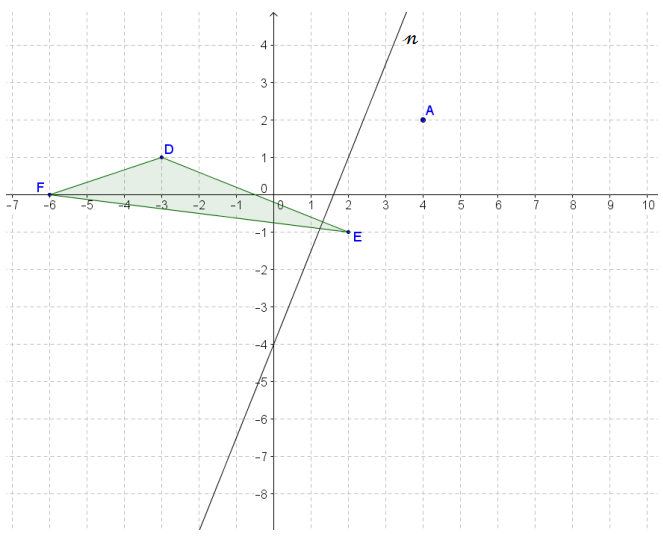
Answer:
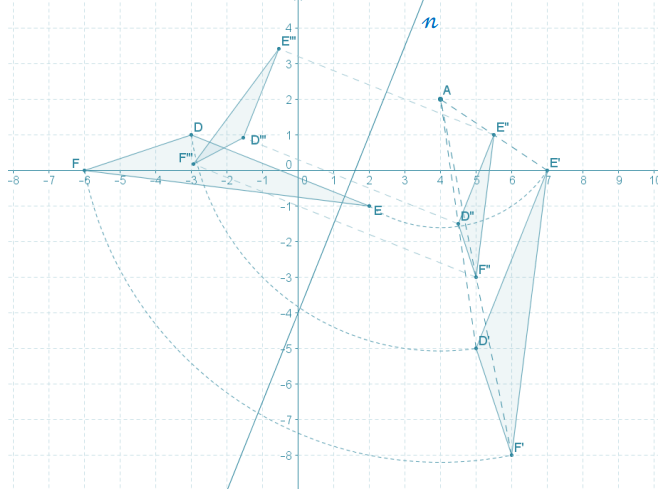
Eureka Math Geometry Module 2 Lesson 13 Problem Set Answer Key
Question 1.
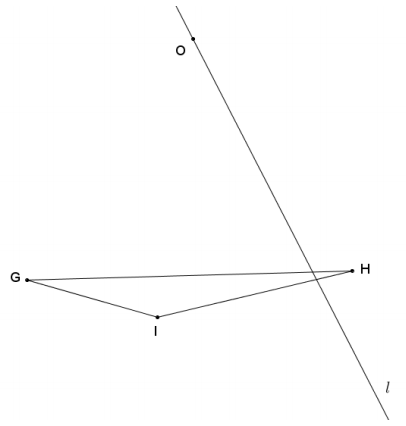
A similarity transformation consists of a reflection over line t’, followed by a dilation from O with a scale factor of r = \(\frac{3}{4}\). Use construction tools to find ∆ G”H”I”.
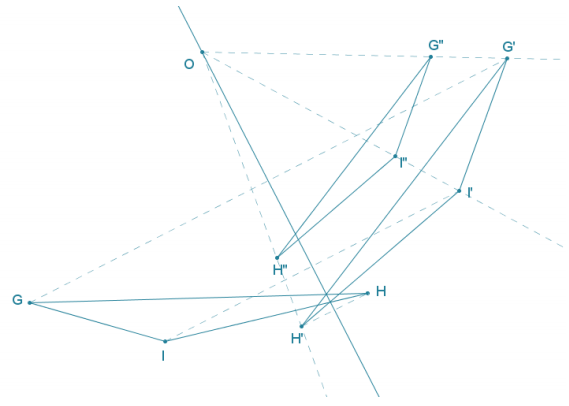
Answer:
Question 2.
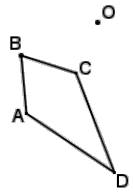
A similarity transformation consists of a dilation from point O with a scale factor of r = 2\(\frac{1}{2}\), followed by a rotation about O of – 90°. Use construction tools to find kite A”B”C”D”.
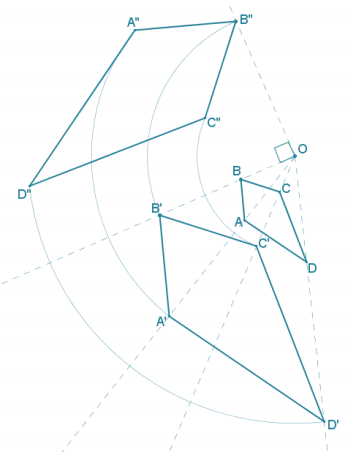
Answer:
Question 3.
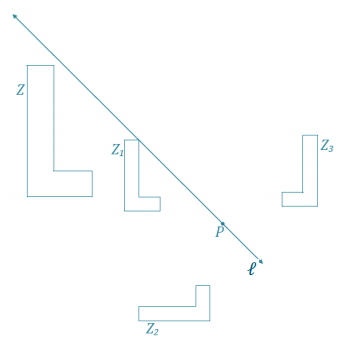
For the Figure Z, find the image of rl(Rp,90° (Dp,\(\frac{1}{2}\)(z)).
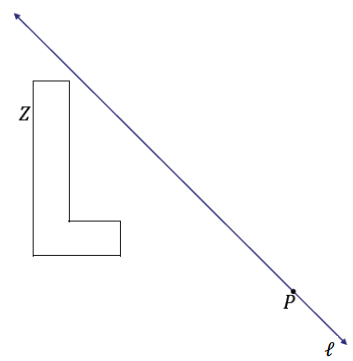
Answer:
Question 4.
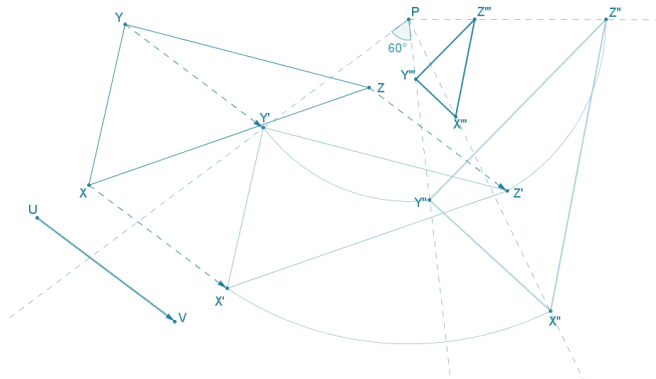
A similarity transformation consists of a translation along vector \(\overrightarrow{U V}\), followed by a rotation of 60° about P, then followed by a dilation from P with a scale factor of r = \(\frac{1}{3}\). Use construction tools to find ∆ X”’Y”’Z”’.
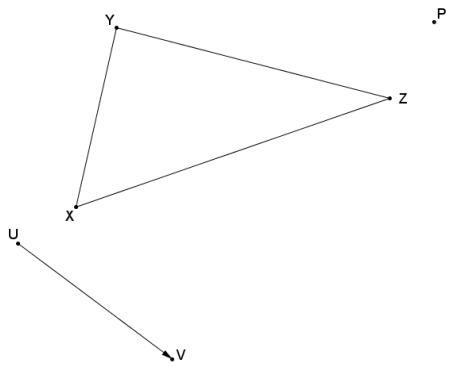
Answer:
Question 5.
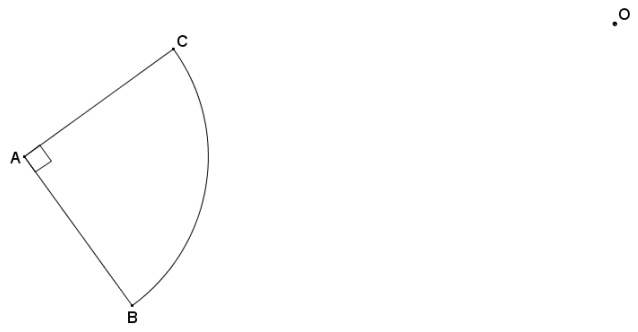
Given the quarter-circular figure determined by points A, B, and C, a similarity transformation consists of a – 65° rotation about point B, followed by a dilation from point O with a scale factor of r = \(\frac{1}{2}\). Find the image of the figure determined by points A”, B”, and C”.
Answer:
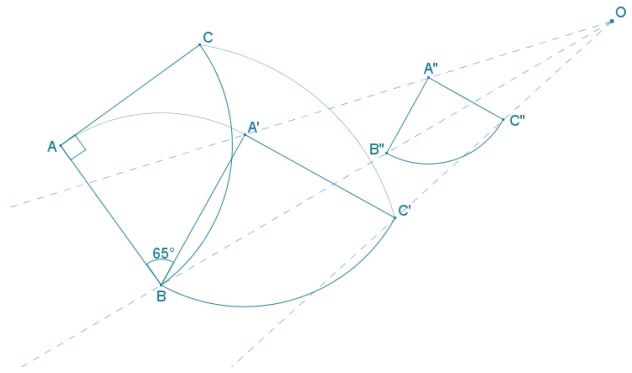
Describe a different similarity transformation that would map quarter-circle ABC to quarter-circle A”B”C”.
Answer:
The quarter-circular region could have first been dilated from point O using o scale factor of \(\frac{1}{2}\), followed by a rotation of 65° about point B’.
Question 6.
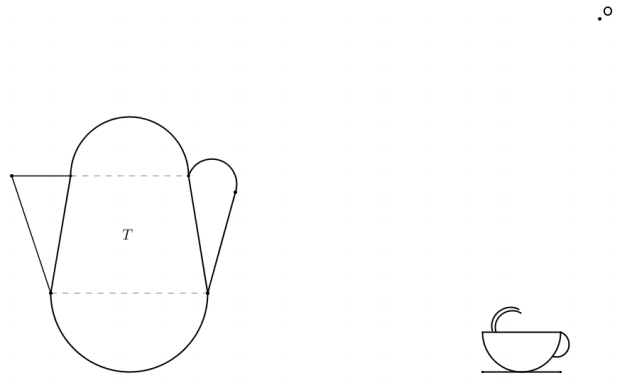
A similarity transformation consists of a dilation from center O with a scale factor of \(\frac{1}{2}\) followed by a rotation of 60° about point O. Complete the similarity transformation on Figure T to complete the drawing of Figure T”.
Answer:
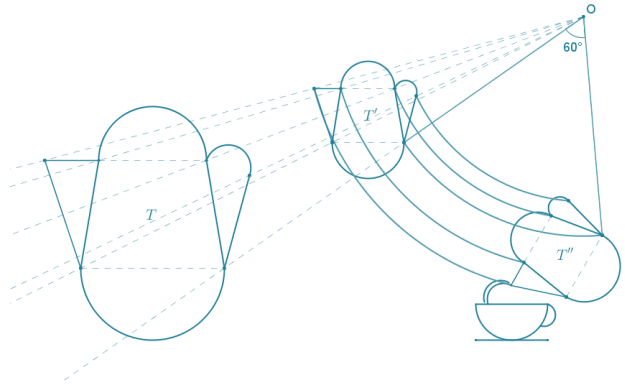
Question 7.
Given Figure R on the coordinate plane shown below, a similarity transformation consists of a dilation from (0, 6) with a scale factor of \(\frac{1}{4}\), followed by a reflection over line x = – 1, and then followed by a vertical translation of 5 units down. Find the image of Figure R.
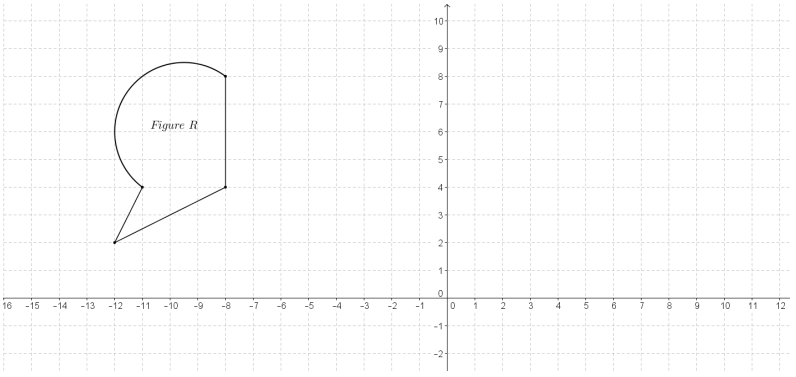
Answer:
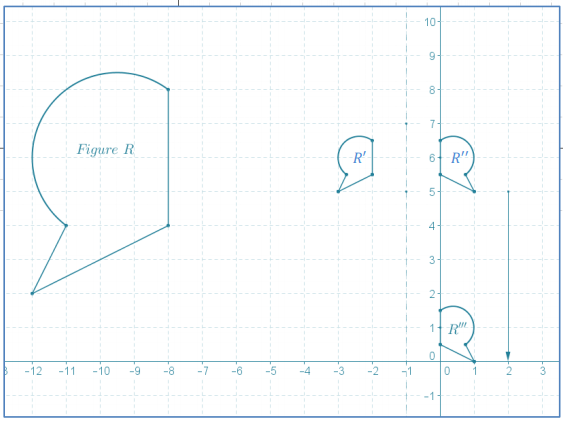
Question 8.
Given ∆ ABC, with vertices A(2, – 7), B(- 2, – 1), and C(3, – 4), locate and label the image of the triangle under the similarity transformation DB’,\(\frac{1}{2}\) (RA,120°.(rx=2 (ABC))).
Answer:
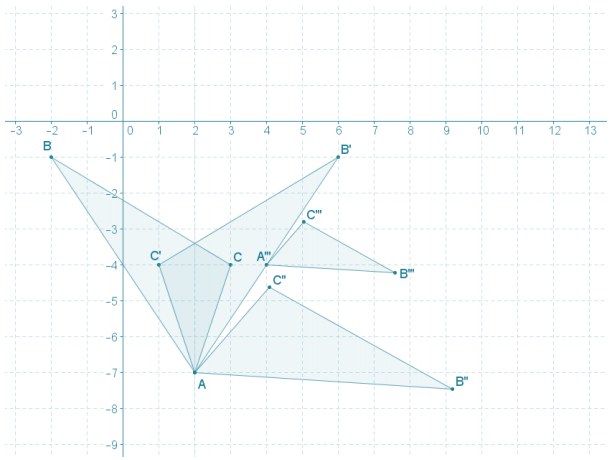
Question 9.
In Problem 8, describe the relationship of A”’ to \(\overline{A B^{\prime}}\), and explain your reasoning.
Answer:
A” is the midpoint of \(\overline{A B^{\prime}}\). I know this because A = A’ = A”, and since the dilation at the end of the similarity transformation was centered at B’, the image of A” must lie on the ray joining it to the center B’, which means that A” lies on \(\overline{A B^{\prime}}\). Furthermore, by the definition of dilation, A”’B’ = r(A”B’), and since the given scale factor was r = \(\frac{1}{2}\), it follows that A”’B’ = \(\frac{1}{2}\)(A”B’), so A” must, therefore, be the midpoint of \(\overline{A B^{\prime}}\).
Question 10.
Given O(-8, 3) and quadrilateral BCDE, with B(-5, 1), C(-6, -1), D(-4, -1), and E(-4, 2), what are the coordinates of the vertices of the image of BCDE under the similarity transformation rx-axis (D0,3 (BCDE))?
Answer:
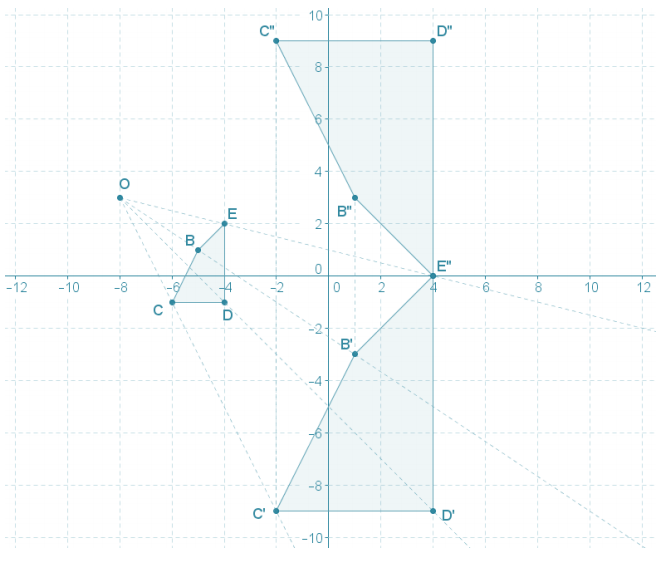
The coordinates of the image of BCDE are B”(1, 3), C”(-2, 9), D”(4, 9), and E”(4, 0).
Question 11.
Given triangle ABC as shown on the diagram of the coordinate plane:
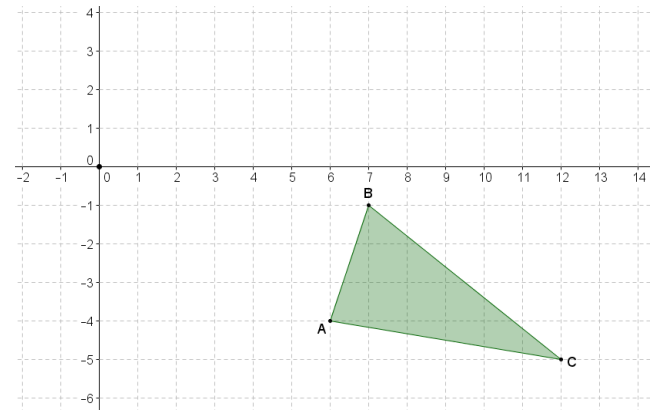
a. Perform a translation so that vertex A maps to the origin.
Answer:
See diagram.
b. Next, dilate the image A’B’C’ from the origin using a scale factor of \(\frac{1}{3}\).
Answer:
See diagram.
c. Finally, translate the image A”B”C” so that the vertex A” maps to the original point A.
Answer:
See diagram.
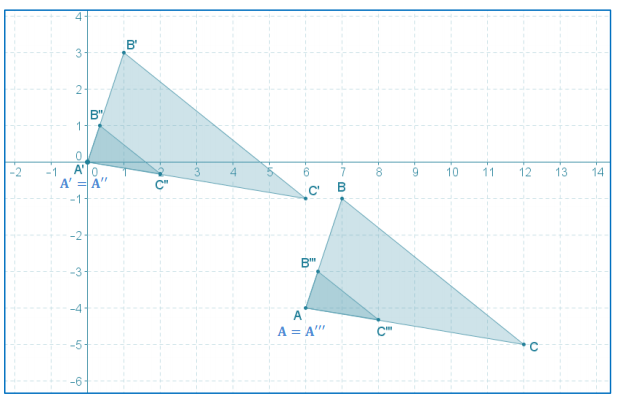
d. Using transformations, describe how the resulting image A”B”C” relates to the original figure ABC.
Answer:
The image A”B”C” is a dilation of figure ABC from point A with a scale factor of \(\frac{1}{3}\).
Question 12.
a. In the coordinate plane, name the single transformation resulting from the composition of the two dilations shown below:
D(0,0),2 followed by D(0,4)\(\frac{1}{2}\)
(Hint: Try it!)
Answer:
The image can be obtained by a translation two units to the right (a vector that has half the distance as the distance between the centers of dilation).
b. In the coordinate plane, name the single transformation resulting from the composition of the two dilations shown below:
D(0,0),2 followed by D(4,4)\(\frac{1}{2}\)
(Hint: Try it!)
Answer:
The Image can be obtained by a translation two units to the right (a vector that has half the distance as the distance between the centers of dilation).
c. Using the results from parts (a) and (b), describe what happens to the origin under both similarity transformations.
Answer:
The origin maps to the midpoint of a segment joining the centers used for each dilation.
Eureka Math Geometry Module 2 Lesson 13 Exit Ticket Answer Key
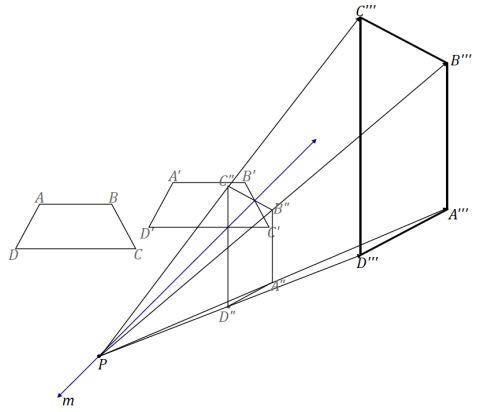
A similarity transformation consists of a translation along the vector \(\overline{F G}\), followed by a dilation from point P with a scale factor of r = 2, and finally a reflection over line m. Use construction tools to find A”’C”’D”’E”’.
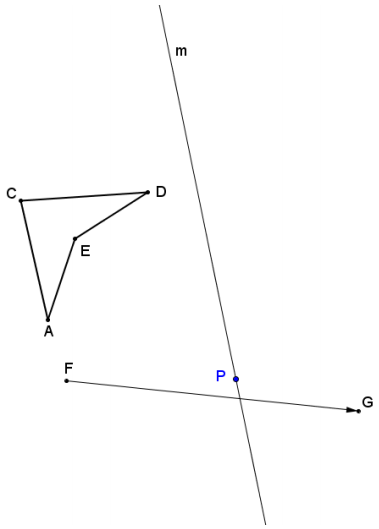
Answer: