Engage NY Eureka Math Algebra 2 Module 4 Lesson 2 Answer Key
Eureka Math Algebra 2 Module 4 Lesson 2 Example Answer Key
Example 1: Building a New High School
The school board of Waldo, a rural town in the Midwest, is considering building a new high school primarily funded by local taxes. They decided to interview eligible voters to determine if the school board should build a new high school facility to replace the current high school building. There is only one high school in the town. Every registered voter in Waldo was interviewed. In addition to asking about support for a new high school, data on gender and age group were also recorded. The data from these interviews are summarized below.
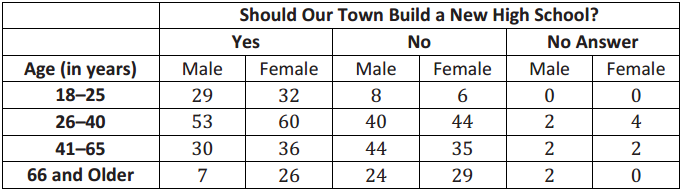
Exercises 1 – 8: Building a New High School
Exercise 1.
Based on this survey, do you think the school board should recommend building a new high school? Explain your answer.
Answer:
273 out of the 515 eligible voters (approximately 53%) indicated “yes.” As a result, I think the voters will recommend building a new high school.
Exercise 2.
An eligible voter is picked at random. If this person is 21 years old, do you think he would indicate that the town should build a high school? Why or why not?
Answer:
Most of the eligible voter’s ages 18 – 25 indicated “yes. 61 of the 75 eligible voters in this age group indicated “yes.” I would predict o 21-year-old in this age group to have answered “yes.”
Exercise 3.
An eligible voter ¡s picked at random. If this person is 55 years old, do you think she would indicate that the town should build a high school? Why or why not?
Answer:
Most of the eligible voters ages 41 – 65 indicated “no” to the question about building a high school. I would predict that a person in this age group would indicate that the town should not build a high school.
Exercise 4.
The school board wondered if the probability of recommending a new high school was different for different age categories. Why do you think the survey classified voters using the age categories 18 – 25 years old, 26 – 40 years old, 41 – 65 years old, and 66 years old and older?
Answer:
The age groups used in the table represent people with different interests or opinions regarding the building of a new high school. For example, people ages 26 – 40 are more likely to have children in school than people in the other age groups. The probability that o person in each of these age categories would recommend building a new high school might vary.
Exercise 5.
It might be helpful to organize the data in a two-way frequency table. Use the given data to complete the following two-way frequency table. Note that the age categories are represented as rows, and the possible responses are represented as columns.
| Yes | No | No Answer | Total | |
| 18–25 Years Old | ||||
| 26–40 Years Old | ||||
| 41–65 Years Old | ||||
| 66 Years Old and Older | ||||
| Total |
Answer:
| Yes | No | No Answer | Total | |
| 18–25 Years Old | 61 | 14 | 0 | 75 |
| 26–40 Years Old | 113 | 84 | 6 | 203 |
| 41–65 Years Old | 66 | 79 | 4 | 149 |
| 66 Years Old and Older | 33 | 53 | 2 | 88 |
| Total | 273 | 230 | 12 | 515 |
Headline 2: Older Voters Less Likely to Support Building a New High School
Exercise 6.
A local news service plans to write an article summarizing the survey results. Three possible headlines for this article are provided below. Is each headline accurate or inaccurate? Support your answering probabilities calculated using the table above.
Answer:
Headline 1: Waldo Voters Likely to Support Building a New High School
Yes, this is accurate. We see that 273 out of 515, which is approximately 53.0%, support building a new school. The probability that an eligible voter would vote “yes” is greater than 0.50, so you would think it is likely that voters will support building a new high school.
Headline 1: Older Voters Likely to Support Building a New High School
Yes, this headline is accurate. If you define older voters as 41 or older, then 132 out of 237 voters 41 or older, which is approximately 55. 7%, indicated they would not support building a new high school.
Headline 3: Younger Voters Not Interested in Building o New High School
This headline is not accurate. We see that 61 out of 75 eligible voters, which is approximately 81.3% representing the youngest eligible voters, indicated “yes” to building a new high school.
Exercise 7.
The school board decided to put the decision on whether or not to build the high school up for a referendum in the next election. At the last referendum regarding this issue, only 25 of the eligible voters ages 18 – 25 voted, 110 of the eligible voters ages 26 – 40 voted, 130 of the eligible voters ages 41 – 65 voted, and 80 of the eligible voters ages 66 and older voted. If the voters in the next election turn out in similar numbers, do you think this referendum will pass? Justify your answer.
Answer:
Use the probabilities that a voter from an age group would vote “yes”. Multiply the probabilities that o person would vote “yes” by the number of people estimated to vote in each age category.
25(0.813) + 110(0.557) + 130(0. 443) + 80(0. 375) ≈ 169
169 out of the estimated 345 voters will vote “yes.” Since this is less than half, we would predict that the vote will indicate the high school should not be built.
Exercise 8.
Is it possible that your prediction of the election outcome might be incorrect? Explain.
Answer:
Yes. The above is only a prediction. The actual results could be different. lt depends on the actual voter turnout and whether people actually vote as they indicated in the survey.
Example 2: Smoking and Asthma
Health officials in Milwaukee, Wisconsin, were concerned about teenagers with asthma. People with asthma often have difficulty with normal breathing. In a local research study, researchers collected data on the incidence of asthma among students enrolled in a Milwaukee public high school.
Students in the high school completed a survey that was used to begin this research. Based on this survey, the probability of a randomly selected student at this high school having asthma was found to be 0.193. Students were also asked if they had at least one family member living in their house who smoked. The probability of a randomly selected student having at least one member in his (or her) household who smoked was reported to be 0.421.
Exercises 9 – 14:
It would be easy to calculate probabilities if the data for the students had been organized into a two-way table like the one used in Exercise 5. But there is no table here, only probability information. One way around this is to think about what the table might have been if there had been 1,000 students at the school when the survey was given. This table is called a hypothetical 1000 two-way table.
What if the population of students at this high school was 1,000? The population was probably not exactly 1,000 students, but using an estimate of 1,000 students provides an easier way to understand the given probabilities. Connecting these estimates to the actual population is completed in a later exercise. Place the value of 1,000 in the cell representing the total population. Based on a hypothetical 1000 population, consider the following table:
| No Household Member Smokes | At Least One Household Member Smokes | Total | |
| Student Has Asthma | Cell 1 | Cell 2 | Cell 3 |
| Student Does Not Have Asthma | Cell 4 | Cell 5 | Cell 6 |
| Total | Cell 7 | Cell 8 |
Exercise 9.
The probability that a randomly selected student at this high school has asthma is 0. 193. This probability can be used to calculate the value of one of the cells in the table above. Which cell is connected to this probability? Use this probability to calculate the value of that cell.
Answer:
Cell 3
The value for this cell would be 193 students.
Exercise 10.
The probability that a randomly selected student has at least 1 household member who smokes is 0.421. Which cell is connected to this probability? Use this probability to calculate the value of that cell.
Answer:
Cell 8
The value for this cell would be 421 students.
Exercise 11.
In addition to the previously given probabilities, the probability that a randomly selected student has at least one household member who smokes and has asthma ¡s 0. 120. Which cell is connected to this probability? Use this probability to calculate the value of that cell.
Answer:
Cell 2
The value for this cell would be 120 students.
Exercise 12.
Complete the two-way frequency table by calculating the values of the other cells in the table.
| No Household Member Smokes | At Least One Household Member Smokes | Total | |
| Student Has Asthma | |||
| Student Does Not Have Asthma | |||
| Total | 1,000 |
Answer:
| No Household Member Smokes | At Least One Household Member Smokes | Total | |
| Student Has Asthma | 73 | 120 | 193 |
| Student Does Not Have Asthma | 506 | 301 | 807 |
| Total | 579 | 421 | 1,000 |
Exercise 13.
Based on your completed two-way table, estimate the following probabilities as a fraction and also as a decimal (rounded to three decimal places):
a. A randomly selected student has asthma. What is the probability this student has at least 1 household member who smokes?
Answer:
120
The probability is \(\frac{120}{193^{\prime}}\) which approximately 0.622.
b. A randomly selected student does not have asthma. What is the probability this student has at least one household member who smokes?
Answer:
301
The probability is \(\frac{301}{807^{\prime}}\) which approximately 0. 373.
c. A randomly selected student has at least one household member who smokes. What is the probability this student has asthma?
Answer:
120
The probability is \(\frac{120}{421^{\prime}}\) which approximately 0.285.
Exercise 14.
Do you think that whether or not a student has asthma is related to whether or not this student has at least one family member who smokes? Explain your answer.
Answer:
Yes, the probability a student with asthma has a household member who smokes is noticeably greater than the probability a student who does not have asthma has o household member who smokes.
Eureka Math Algebra 2 Module 4 Lesson 2 Problem Set Answer Key
Question 1.
The Waldo School Board asked eligible voters to evaluate the town’s library service. Data are summarized in the following table:
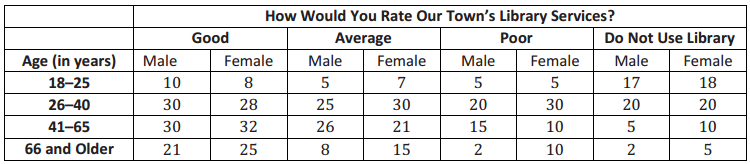
a. What is the probability that a randomly selected person who completed the survey rated the library as good?
Answer:
\(\frac{184}{515}\) ≈ 0. 357
b. Imagine talking to a randomly selected male voter who had completed the survey. How do you think this person rated the library services? Explain your answer.
Answer:
Answers will vary. A general look at the table indicates that most mole voters rated the library, as good. As a result, I would predict that this person would rate the library as good.
c. Use the given data to construct a two-way table that summarizes the responses on gender and rating of the library services. Use the following template as your guide:
| Good | Average | Poor | Do not use | Total | |
| Male | |||||
| Female | |||||
| Total |
Answer:
| Good | Average | Poor | Do Not Use | Total | |
| Male | 91 | 64 | 42 | 44 | 241 |
| Female | 93 | 73 | 55 | 53 | 274 |
| Total | 184 | 137 | 97 | 97 | 515 |
d. Based on your table, answer the following:
i. A randomly selected person who completed the survey is male. What ¡s the probability he rates the library services as good?
Answer:
\(\frac{91}{241}\) ≈ 0. 378
ii. A randomly selected person who completed the survey is female. What is the probability she rates the
library services as good?
Answer:
\(\frac{93}{274}\) ≈ 0.339
e. Based on your table, answer the following:
i. A randomly selected person who completed the survey rated the library services as good. What is the probability this person is male?
Answer:
\(\frac{91}{184}\) ≈ 0.495
ii. A randomly selected person who completed the survey rated the library services as good. What ¡s the
probability this person is female?
Answer:
\(\frac{93}{274}\) ≈ 0. 339
f. Do you think there Is a difference in how male and female voters rated library services? Explain your answer.
Answer:
Answers will vary. Yes, I think there is a difference. For example, 37.9% of the male voters rated the library services as good, but only 33.9% of the female voters rated the services this way. There are also differences between the ratings from male and female voters for the other categories.
Question 2.
Obedience School for Dogs is a small franchise that offers obedience classes for dogs. Some people think that larger dogs are easier to train and, therefore, should not be charged as much for the classes. To investigate this claim, dogs enrolled in the classes were classified as large (30 pounds or more) or small (under 30 pounds). The dogs were also classified by whether or not they passed the obedience class offered by the franchise. 45% of the dogs involved in the classes were large. 60% of the dogs passed the class. Records indicate that 40% of the dogs in the classes were small and passed the course.
a. Complete the following hypothetical 1000 two-way table:
| Passed the course | Did not pass the course | Total | |
| Lage dogs | |||
| Small dogs | |||
| Total |
Answer:
| Passed the Course | Did Not Pass the Course | Total | |
| Large Dogs | 200 | 250 | 450 |
| Small Dogs | 400 | 150 | 550 |
| Total | 600 | 400 | 1,000 |
b. Estimate the probability that a dog selected at random from those enrolled in the classes passed the course.
Answer:
\(\frac{600}{1000}\) = 0. 600, meaning 60% passed the course.
c. A dog was randomly selected from the dogs that completed the class. If the selected dog was a large dog, what is the probability this dog passed the course?
Answer:
\(\frac{200}{450}\) = 0.444, meaning that approximately 44.4% of large dogs passed the course.
d. A dog was randomly selected from the dogs that completed the class. If the selected dog is a small dog, what is the probability this dog passed the course?
Answer:
\(\frac{400}{550}\) = 0. 727, meaning that approximately 72.7% of small dogs passed the course.
e. Do you think dog size and whether or not a dog passes the course are related?
Answer:
Answers will vary. Yes, there is a noticeably greater probability that a dog passed the obedience class if a dog is small than if the dog is large.
f. Do you think large dogs should get a discount? Explain your answer.
Answer:
Answers will vary. No, large dogs should not get a discount. Large dogs are not as likely to have passed the obedience class as small dogs.
Eureka Math Algebra 2 Module 4 Lesson 2 Exit Ticket Answer Key
Did male and female voters respond similarly to the survey question about building a new high school? Recall the original summary of the data.
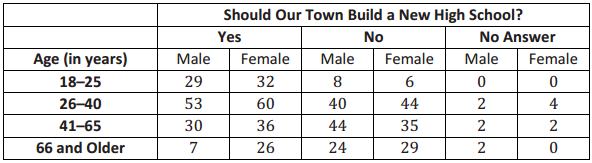
Question 1.
complete the following two – way frequency table:
| Yes | No | No Answer | Total | |
| Male | 119 | 6 | ||
| Female | ||||
| Total | 230 | 12 | 515 |
Answer:
| Yes | No | No Answer | Total | |
| Male | 119 | 116 | 6 | 241 |
| Female | 154 | 114 | 6 | 274 |
| Total | 273 | 230 | 12 | 515 |
Question 2.
Use the above two-way frequency table to answer the following questions:
a. If a randomly selected eligible voter is female, what is the probability she will vote to build a new high school?
Answer:
\(\frac{154}{274}\) ≈ 0.562
b. If a randomly selected eligible voter is male, what Is the probability he will vote to build a new high school?
Answer:
\(\frac{119}{241}\) ≈ 0.494
Question 3.
An automobile company has two factories assembling its luxury cars. The company Is interested in whether consumers rate cars produced at one factory more highly than cars produced at the other factory. Factory A assembles 60% of the cars. A recent survey indicated that 70% of the cars made by this company (both factories combined) were highly rated. This same survey indicated that 10% of all cars made by this company were both made at Factory B and were not highly rated.
a. Create a hypothetical 1000 two-way table based on the results of this survey by filling in the table below.
| Car Was Highly Rated by Consumers | Car Was Not Highly Rated by Consumers | Total | |
| Factory A | |||
| Factory B | |||
| Total |
Answer:
| Car Was Highly Rated by Consumers | Car Was Not Highly Rated by Consumers | Total | |
| Factory A | 400 | 200 | 600 |
| Factory B | 300 | 100 | 400 |
| Total | 700 | 300 | 1,000 |
b. A randomly selected car was assembled in Factory B. What is the probability this car is highly rated?
Answer:
The probability a car from Factory B is highly rated is \(\frac{300}{400^{\prime}}\) which is 0.750.