Engage NY Eureka Math Algebra 1 Module 1 Lesson 12 Answer Key
Eureka Math Algebra 1 Module 1 Lesson 12 Opening Exercise Answer Key
Answer the following questions.
a. Why should the equations (x-1)(x+3)=17+x and (x-1)(x+3)=x+17 have the same solution set?
Answer:
The commutative property
b. Why should the equations (x-1)(x+3)=17+x and (x+3)(x-1)=17+x have the same solution set?
Answer:
The commutative property
c. Do you think the equations (x-1)(x+3)=17+x and (x-1)(x+3)+500=517+x should have the same solution set? Why?
Answer:
Yes. 500 was added to both sides.
d. Do you think the equations (x-1)(x+3)=17+x and 3(x-1)(x+3)=51+3x should have the same solution set? Explain why.
Answer:
Yes. Both sides were multiplied by 3.
Eureka Math Algebra 1 Module 1 Lesson 12 Exercise Answer Key
Exercise 1.
a. Use the commutative property to write an equation that has the same solution set as
x2-3x+4=(x+7)(x-12)(5).
Answer:
-3x+x2+4=(x+7)(5)(x-12)
b. Use the associative property to write an equation that has the same solution set as
x2-3x+4=(x+7)(x-12)(5).
Answer:
(x2-3x)+4=((x+7)(x-12))(5)
c. Does this reasoning apply to the distributive property as well?
Answer:
Yes, it does apply to the distributive property.
Exercise 2.
Consider the equation x2+1=7-x.
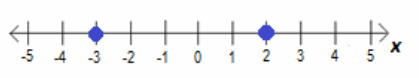
a. Verify that this has the solution set {-3,2}. Draw this solution set as a graph on the number line. We will later learn how to show that these happen to be the ONLY solutions to this equation.
Answer:
22+1=7-2 True. (-3)2+1=7-(-3) “True.”
b. Let’s add 4 to both sides of the equation and consider the new equation x2+5=11-x. Verify 2 and -3 are still solutions.
Answer:
22+5=11-2 True. (-3)2+5=11-(-3) True. They are still solutions.
c. Let’s now add x to both sides of the equation and consider the new equation x2+5+x=11. Are 2 and -3 still solutions?
Answer:
22+5+2=11 True. (-3)2+5+-3=11 True. 2 and -3 are still solutions.
d. Let’s add -5 to both sides of the equation and consider the new equation x2+x=6. Are 2 and -3 still solutions?
Answer:
22+2=6 True. (-3)2+-3=6 True. 2 and -3 are still solutions.
e. Let’s multiply both sides by \(\frac{1}{6}\) to get \(\frac{x^{2}+x}{6}\)=1. Are 2 and -3 still solutions?
Answer:
\(\frac{2^{2}+2}{6}\)=1 True. \(\frac{(-3)^{2}+(-3)}{6}\)=1 True. 2 and -3 are still solutions.
f. Let’s go back to part (d) and add 3x3 to both sides of the equation and consider the new equation
x2+x+3x3=6+3x3. Are 2 and -3 still solutions?
Answer:
22+2+3(2)3=6+3(2)3
4+2+24=6+24
(-3)2+-3+3(-3)3=6+3(-3)3
True.
9-3-81=6-81
True.
2 and -3 are still solutions.
Exercise 3.
Solve for r: \(\frac{3}{2r}\)=\(\frac{1}{4}\)
Answer:
r=6
b. Solve for s: s2+5=30
Answer:
s=5, s=-5
c. Solve for y: 4y-3=5y-8
Answer:
y=5
Exercise 4.
Consider the equation 3x+4=8x-16. Solve for x using the given starting point.
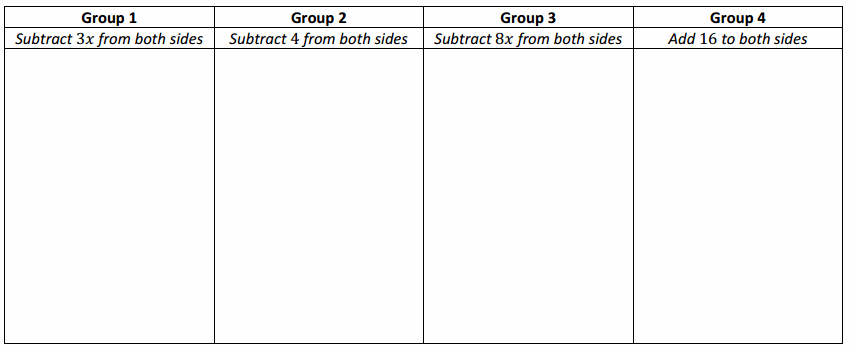
Answer:
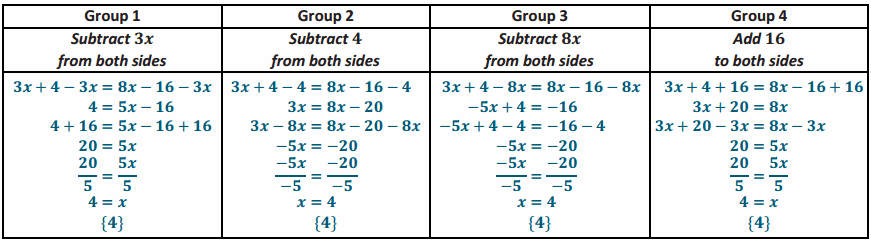
→ Therefore, according to this exercise, does it matter which step happens first? Explain why or why not.
→ No, because the properties of equality produce equivalent expressions, no matter the order in which they happen.
→ How does one know “how much” to add/subtract/multiply/divide? What is the goal of using the properties, and how do they allow equations to be solved?
Encourage students to verbalize their strategies to the class and to question each other’s reasoning and question the precision of each other’s description of their reasoning. From middle school, students recall that the goal is to isolate the variable by making 0s and 1s. Add/subtract numbers to make the zeros, and multiply/divide numbers to make the 1s. The properties say any numbers will work, which is true, but with the 0s and 1s goal in mind, equations can be solved very efficiently.
→ The ability to pick the most efficient solution method comes with practice.
Closing
Consider the equation 3x2+x=(x-2)(x+5)x.
a. Use the commutative property to create an equation with the same solution set.
Answer:
x+3x2=(x+5)(x-2)x
b. Using the result from part (a), use the associative property to create an equation with the same solution set.
Answer:
(x+3x2 )=((x+5)(x-2))x
c. Using the result from part (b), use the distributive property to create an equation with the same solution set.
Answer:
x+3x2=x3+3x2-10x
d. Using the result from part (c), add a number to both sides of the equation.
Answer:
x+3x2+5=x3+3x3-10x+5
e. Using the result from part (d), subtract a number from both sides of the equation.
Answer:
x+3x2+5-3=x3+3x2-10x+5-3
f. Using the result from part (e), multiply both sides of the equation by a number.
Answer:
4(x+3x2+2)=4(x3+3x2-10x+2)
g. Using the result from part (f), divide both sides of the equation by a number.
Answer:
x+3x2+2=x3+3x2-10x+2
h. What do all seven equations have in common? Justify your answer.
Answer:
They will all have the same solution set.
Eureka Math Algebra 1 Module 1 Lesson 12 Problem Set Answer Key
Question 1.
Which of the following equations have the same solution set? Give reasons for your answers that do not depend on solving the equations.
I. x-5=3x+7
II. 3x-6=7x+8
III. 15x-9=6x+24
IV. 6x-16=14x+12
V. 9x+21=3x-15
VI. -0.05+\(\frac{x}{100}\)=3\(\frac{x}{100}\)+0.07
Answer:
I, V, and VI all have the same solution set; V is the same as I after multiplying both sides by 3 and switching the left side with the right side; VI is the same as I after dividing both sides by 100 and using the commutative property to rearrange the terms on the left side of the equation.
II and IV have the same solution set. IV is the same as II after multiplying both sides by 2 and subtracting 4 from both sides.
III does not have the same solution set as any of the others.
Solve the following equations, check your solutions, and then graph the solution sets.
Question 2.
-16-6v=-2(8v-7)
Answer:
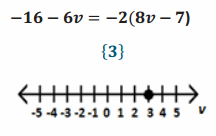
Question 3.
2(6b+8)=4+6b
Answer:
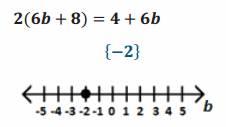
Question 4.
x2 -4x + 4 = 0
Answer:
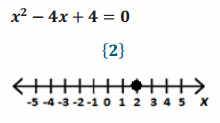
Question 5.
7-8x=7(1+7x)
Answer:

Question 6.
39-8n=-8(3+4n)+3n
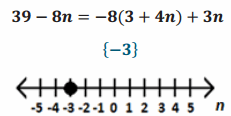
Question 7.
(x-1)(x+5)=x2+4x-2
Answer:
no solution
Question 8.
x2-7=x2-6x-7
Answer:
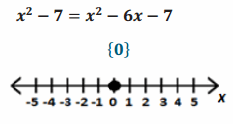
Question 9.
-7-6a+5a=3a-5a
Answer:
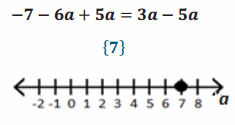
Question 10.
7-2x=1-5x+2x
Answer:
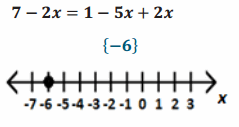
Question 11.
4(x-2)=8(x-3)-12
Answer:
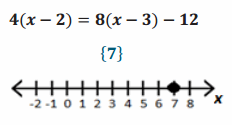
Question 12.
-3(1-n)=-6-6n
Answer:
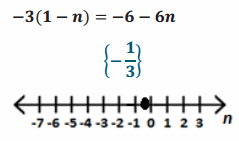
Question 13.
-21-8a=-5(a+6)
Answer:
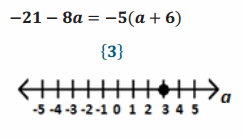
Question 14.
-11-2p=6p+5(p+3)
Answer:

Question 15.
\(\frac{x}{x+2}\)=4
Answer:
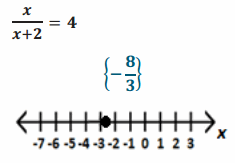
Question 16.
2+\(\frac{x}{9}\)=\(\frac{x}{3}\)-3
Answer:
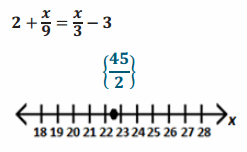
Question 17.
-5(-5x-6)=-22-x
Answer:
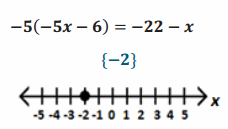
Question 18.
\(\frac{x+4}{3}\) = \(\frac{x+2}{5}\)
Answer:
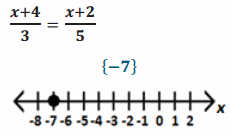
Question 19.
-5(2r-0.3)+0.5(4r+3)=-64
Answer:
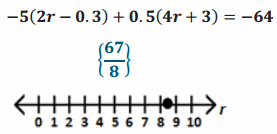
Eureka Math Algebra 1 Module 1 Lesson 12 Exit Ticket Answer Key
Determine which of the following equations have the same solution set by recognizing properties rather than solving.
a. 2x+3=13-5x
b. 6+4x=-10x+26
c. 6x+9=\(\frac{13}{5}\)-x
d. 0.6+0.4x=-x+2.6
e. 3(2x+3)=\(\frac{13}{5}\)-x
f. 4x=-10x+20
g. 15(2x+3)=13-5x
h. 15(2x+3)+97=110-5x
Answer:
(a), (b), (d), and (f) have the same solution set. (c), (e), (g), and (h) have the same solution set.