Are you looking for any material to know the relation between all Trigonometrical Ratios of 90 Degree Minus Theta? Then, you can relax now. On this page, we have enclosed the detailed information on the relation between Trigonometric Ratios of (90° – θ) along with the proofs. Get the example questions and step-by-step solutions in the following sections of this article. Check out the simple formula to memorize the Trigonometric Functions.
How to Determine the Trigonometric Ratios of 90 Degree Minus Theta?
Here, you can see Trigonometrical Functions of 90 Degree Minus Theta can be determined. As per the ASTC “All Silver Tea Cups” or “All Students Take Calculus”
A means All, S means “Sinθ, Cosecθ”, T means “Tanθ, Cotθ”, C means Cosθ, Secθ.
The pictorial representation of the ASTC formula is as follows:
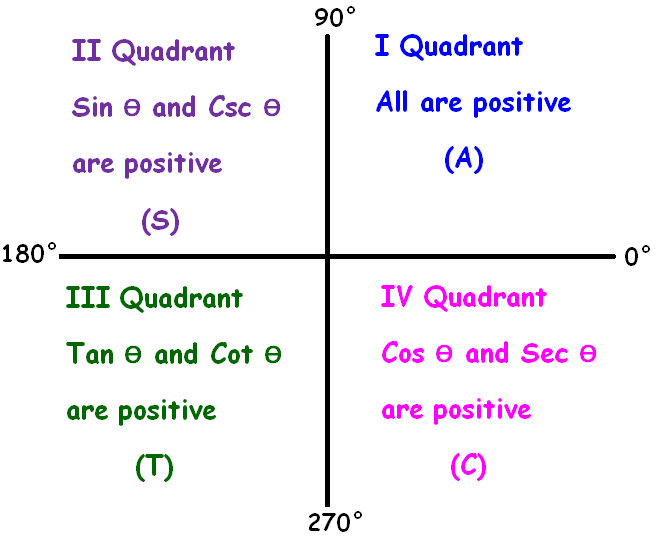
From the above picture, (90° – θ) falls in the first quadrant.
sin (90° – θ) = cos θ
cos (90° – θ) = sin θ
tan (90° – θ) = cot θ
cosec (90° – θ) = sec θ
sec (90° – θ) = cosec θ
cot (90° – θ) = tan θ
Evaluate Trigonometrical Ratios of 90 Degree Minus Theta
1. Evaluate Sin(90° – θ)?
To evaluate sin (90° – θ), we have to consider the following important points.
- (90° – θ) will fall in the 1st quadrant.
- When we have 90°, “sin” will become “cos”.
- In the 1st quadrant, the sign of “sin” is positive.
Considering the above points, we have
Sin (90° – θ) = Cos θ
2. Evaluate Cos(90° – θ)?
To evaluate cos (90° – θ), we have to consider the following important points.
- (90° – θ) will fall in the 1st quadrant.
- When we have 90°, “cos” will become “sin”.
- In the 1st quadrant, the sign of “cos” is positive.
Considering the above points, we have
Cos (90° – θ) = Sin θ
3. Evaluate Tan(90° – θ)?
To evaluate tan (90° – θ), we have to consider the following important points.
- (90° – θ) will fall in the 1st quadrant.
- When we have 90°, “tan” will become “cot”.
- In the 1st quadrant, the sign of “tan” is positive.
Considering the above points, we have
Tan (90° – θ) = Cot θ
4. Evaluate Cot(90° – θ)?
To evaluate cot (90° – θ), we have to consider the following important points.
- (90° – θ) will fall in the 1st quadrant.
- When we have 90°, “cot” will become “tan”
- In the 1st quadrant, the sign of “cot” is positive.
Considering the above points, we have
Cot (90° – θ) = Tan θ
5. Evaluate Cosec(90° – θ)?
To evaluate Cosec (90° – θ), we have to consider the following important points.
- (90° – θ) will fall in the 1st quadrant.
- When we have 90°, “Cosec” will become “sec”.
- In the 1st quadrant, the sign of “Cosec” is positive.
Considering the above points, we have
Cosec (90° – θ) = Sec θ
6. Evaluate Sec(90° – θ)?
To evaluate sec (90° – θ), we have to consider the following important points.
- (90° – θ) will fall in the 1st quadrant.
- When we have 90°, “sec” will become “cosec”.
- In the 1st quadrant, the sign of “sec” is positive.
Considering the above points, we have
Sec (90° – θ) = Cosec θ
More Related Articles:
- Trigonometrical Ratios of 90 Degree Plus Theta
- Trigonometric Functions of 180 Minus Theta
- Trigonometrical Ratios Table
- Worksheet on Trigonometric Identities
Solved Examples on Trigonometric Ratios of 90° – θ
Example 1:
Find the value of Tan 45°?
Solution:
Tan 45° = Tan (90° – 45°)
We know that Tan (90° – θ) = Cot θ
So, Tan 45° = Cot 45°
= 1 [Cot 45° = 1]
Therefore, Tan 45° = 1.
Example 2:
Find the value of Sin 65°?
Solution:
Sin 65° = Sin (90° – 25°)
We know that Sin (90° – θ) = Cos θ
So, Sin 65° = Cos 25°
= 0.906308 [ Cos 25° = 0.906308]
Therefore, Sin 65° = 0.906308
Example 3:
Find the value of Cot 80°?
Solution:
Cot 80° = Cot (90° – 10°)
We know that Cot (90° – θ) = Tan θ
So, Cot 80° = Tan 10°
= 0.17633 [ Tan 10° = 0.17633]
Therefore, Cot 80° = 0.17633
Example 4:
Find the value of Cos 50°?
Solution:
Cos 50° = Cos (90° – 40°)
We know that Cos (90° – θ) = Sin θ
So, Cos 50° = Sin(40°)
= 0.64278760968 [ Sin(40°) = 0.64278760968]
Therefore, Cos 50° = 0.64278760968.