LCM or Least Common Multiple which is used to add or subtract any two fractions when the denominators are unlike. It is used while performing arithmetic operations like addition, subtraction, multiplication, and division. This L.C.M will help to make the denominators common. There are different methods to find the L.C.M of two or more numbers such as listing multiples method, prime factors method, division method, etc. The Methods of Least Common Multiple are shown in the below sections.
Do Refer:
Methods of L.C.M
There are three methods in finding the LCM and we have explained each one of them in detail by considering few examples. Check out all the methods and choose the one that you are comfortable and find the Least Common Multiple easily. They are as follows
1. LCM by Listing Multiples Method
Follow the below Steps to Find LCM by Listing Multiples and they are as such
1. First write the numbers.
2. Then write the several multiples of the first number.
3. Next, write the several multiples of the second number.
4. Now find the smallest multiple from the given numbers.
Example: Find the LCM of 8 and 16.
Solution:
The multiples of 8 are 8, 16, 24
The factors of 16 are 16, 32, 48
Thus the Least Common Multiple of 8 and 16 is 16.
2. Prime Factorization Method of LCM
The prime factors are another method to find the least common multiples of two or more numbers. Steps to find the LCM using Prime Factors Method is as under
1. Find the prime factorization of each number.
2. Write each number as a product of primes, matching primes vertically when possible.
3. Bring down the primes in each column.
4. Multiply the factors to get the LCM.
Example: Find the LCM of 12 and 16.
Solution:
First, find the factors of 12 and 16.
Factors of 12 = 2 × 2 × 3
Factors of 16 = 2 × 2 × 2 × 2
Now write the prime factors of two numbers
12 × 16 = 2 × 2 × 3 × 2 × 2 × 2 × 2
= 3 × 2 × 2 × 2 × 2 = 48
Thus the LCM of 12 and 16 is 48.
L.C.M by Division Method
Finding LCM using the Division method is very easy. Follow the below steps to find the LCM of two or more numbers.
1. First the write the numbers by putting commas.
2. Now divide the numbers with the prime numbers like 2, 3, 5…
3. If any number is not divisible, then write down that and continue the procedure.
4. Keep on dividing the numbers with the least prime numbers until you get the remainder for the given numbers.
5. Now LCM of the numbers must be equal to the product of all the prime numbers.
Example: Find the LCM of 10 and 20.
Solution:
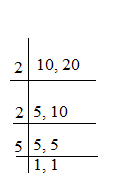
LCM = 2 × 2 × 5 = 20
Thus the LCM of 10 and 20 is 20.
Relation Between HCF and LCM
HCF means highest common factor and LCM means Least Common Multiple. These are the two methods that are used to find multiples and factors in arithmetic operations like addition, subtraction, multiplication, and division.
LCM(a, b) = a × b/HCF(a, b)
HCF(a, b) = a × b/LCM(a, b)
LCM Method Examples
Example 1.
Find the LCM of 25 and 50 by using the division method.
Solution:
Dividing the numbers with the least prime numbers until you get the remainder for the given numbers.
Let us start with 2.
50 = 2 × 25
Now divide two numbers by 5 until you get the remainder as 1.
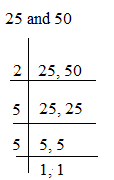
LCM = 2 × 5 × 5 = 50
Thus the Least Common Multiple of 25 and 50 is 50.
Example 2.
Find the LCM of 6, 12, and 18 by listing multiples.
Solution:
We have to find the LCM of 6, 12 and 18 by listing multiples.
Multiples of 6 are 6, 12, 18, 24, 30, 36, 42
Multiples of 12 are 12, 24, 36, 48, 60
Multiples of 18 are 18, 36, 54, 72, 90
Thus the LCM of 6, 12 and 18 is 36.
Example 3.
Find the LCM of two numbers 42 and 54 by using the prime factorization method.
Solution:
The prime factors of 42 = 2 × 3 × 7
The prime factors of 54 = 2 × 3 × 3 × 3
The common prime factors are 2 × 3 × 3 × 3 × 7 = 378.
Example 4.
Find the LCM of 24 and 36
Solution:
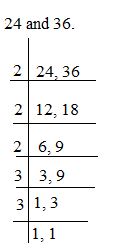
LCM = 2 × 2 × 2 × 3 × 3 = 72
Thus the LCM of 24 and 36 is 72.
Example 5.
Find the LCM of 30 and 40 by using the prime factorization method.
Solution:
The prime factors of 30 are 2 × 3 × 5
The prime factors of 40 are 2 × 2 × 2 × 5
LCM = 2 × 2 × 2 × 3 × 5 = 120
Thus the LCM of 30 and 40 is 120.
FAQs on Method of finding L.C.M
1. What is the LCM of 4 and 12.
We can find the LCM of 4 and 12 using the listing multiples method.
Multiples of 4 – 4, 8, 12, 16, 20
Multiples of 12 – 12, 24, 36
Thus the LCM of 4 and 12 is 12.
2. What are the methods to find the LCM?
There are 3 ways to find the LCM of the given numbers.
1. Prime Factorization Method or Factor Tree Method
2. Division Method
3. Listing Multiples
3. What is L.C.M?
LCM stands for Least common multiple. LCM is the method to find the smallest possible multiple of two or more numbers.