Engage NY Eureka Math 5th Grade Module 5 Lesson 14 Answer Key
Eureka Math Grade 5 Module 5 Lesson 14 Problem Set Answer Key
Question 1.
George decided to paint a wall with two windows. Both windows are 3\(\frac{1}{2}\) -ft by 4\(\frac{1}{2}\)-ft rectangles. Find the area the paint needs to cover.
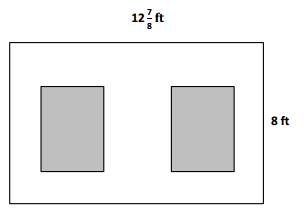
Answer:
Given, the measurements of the window = 3 1/2 feet by 4 1/2 feet
Area = length x width
A = 3 1/2 x 4 1/2
= 7/2 x 9/2
= 63/4
= 15 3/4
Number of windows = 2
So, 2 x 15 3/4
= 30 6/4 = 31 1/2 square feet
Area of the wall = 12 7/8 x 8
= 103 square feet
Area to paint = 103 – 31 1/2
= 71 1/2 square feet
Therefore, the area need to paint = 71 1/2 square feet.
Question 2.
Joe uses square tiles, some of which he cuts in half, to make the figure below. If each square tile has a side length of 2\(\frac{1}{2}\) inches, what is the total area of the figure?

Answer:
Total number of full tiles = 10
Number of half tiles = 6 or 3 full tiles
Total = 10 + 3 = 13 tiles
Area of the tiles = 2 1/2 x 21/2
A =25/4 = 6 1/4 square inches
Now, 13 x 6 1/4 = 78 13/4
= 81 1/4 square inches
Therefore, the area of the figure = 81 1/4 square inches.
Question 3.
All-In-One Carpets is installing carpeting in three rooms. How many square feet of carpet are needed to carpet all three rooms?
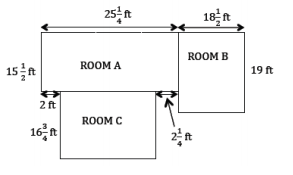
Answer:
Area of the room A =
25 1/4 ft x 15 1/2 ft
= 25 1/4 x 15 1/2
= 101/4 x 31/2
= 3131/8
= 391 3/8 square feet
Area of the room B =
19 x 18 1/2
= 352 19/2
= 351 1/2 square feet
Area of room C =
25 1/4 – 4 1/4
= 21
21 x 16 3/4
= 336 63/4
= 351 3/4 square feet
Total area of three rooms =
391 3/8 + 351 1/2 + 351 3/4
= 1093 5/8
Therefore, 1094 5/8 square feet of carpet is needed to cover all the three rooms.
Question 4.
Mr. Johnson needs to buy sod for his front lawn.
a. If the lawn measures 36\(\frac{2}{3}\) ft by 45\(\frac{1}{6}\) ft, how many square feet of sod will he need?
b. If sod is only sold in whole square feet, how much will Mr. Johnson have to pay?
Sod Price
| Area |
Price per Square Foot |
| First 1,000 sq ft | $0.27 |
| Next 500 sq ft | $0.22 |
| Additional square feet | $0.19 |
Answer:
a.
Area = length x width
A= 36 2/3 x 45 1/6
= ( 36 x 45 ) + ( 36 x 1/6) +(45 x 2/3 ) + ( 2/3 x 1/6)
= 1620 + 6 +30 +1/9
= 1656 1/9 square feet
Therefore, he need 1656 1/9 square feet of sod.
b.
According to given table,
1000 x $0.27 = $270.00
500 x $ 0.22 = $ 110.00
157 x $ 0.19 = $ 29.83
Total = $270 + $110 + $29.83 = $409.83
Therefore, he will have to pay $409.83
Question 5.
Jennifer’s class decides to make a quilt. Each of the 24 students will make a quilt square that is 8 inches on each side. When they sew the quilt together, every edge of each quilt square will lose \(\frac{3}{4}\) of an inch.
a. Draw one way the squares could be arranged to make a rectangular quilt. Then, find the perimeter of your arrangement.
b. Find the area of the quilt.
Answer:
a.
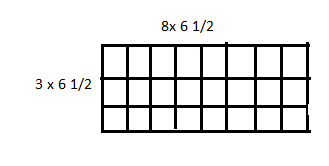
Perimeter =
8 x 6 1/2
= 8 x 13/2 = 52
3 x 6 1/2
= 3 x 13 /2 = 19 1/2
Perimeter = 52 + 52 + 19 1/2 + 19 1/2
= 104 + 39
= 143 inches
Therefore, perimeter of the arrangement = 143 inches’
b.
The area of the quilt =
Each square area = 8 – 1 1/2
= 6 1/2
All the squares = 42 1/4 x 24
= 169/4 x 24
= 1014 square inches
Therefore, the area of the quilt = 1014 square inches
Eureka Math Grade 5 Module 5 Lesson 14 Exit Ticket Answer Key
Mr. Klimek made his wife a rectangular vegetable garden. The width is 5\(\frac{3}{4}\) ft, and the length is 9\(\frac{4}{5}\) ft. What is the area of the garden?
Answer:
Area = length x width
= 5 3/4 x 9 4/5
= ( 5 x 9 ) + ( 5 x 4/5 ) + ( 9 x 3/4 ) + ( 3/4 x 4/5 )
= 45 + 27/4 + 20/5 + 12/20
= 900 + 135 + 80 + 12/ 20
= 1127/20
= 56 7/20 square feet
Therefore, the area of the garden = 56 7/20 square feet.
Eureka Math Grade 5 Module 5 Lesson 14 Homework Answer Key
Question 1.
Mr. Albano wants to paint menus on the wall of his café in chalkboard paint. The gray area below shows where the rectangular menus will be. Each menu will measure 6-ft wide and 7\(\frac{1}{2}\)-ft tall.
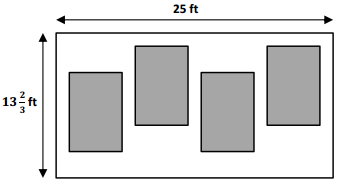
• How many square feet of menu space will Mr. Albano have?
• What is the area of wall space that is not covered by chalkboard paint?
Answer:
a.
The measurement of menus = 6 feet wide by 7 1/2 feet tall
Area = 6 x 7 1/2
= ( 6 x 7 ) + ( 6 x 1/2 )
= 42 + 3
= 45 square feet
The number of menus = 4
Total = 4 x 45 = 180
Therefore, the total space for menus = 180 square feet.
b.
The area of big board =
= 25 x 13 2/3
= ( 25 x 2/3 ) + ( 25 x 2/3 )
= 325 + 50/3
= 341 2/3 square feet
Now,
The area not covered = 341 2/3 – 180
= 161 2/3 square feet
Question 2.
Mr. Albano wants to put tiles in the shape of a dinosaur at the front entrance. He will need to cut some tiles in half to make the figure. If each square tile is 4\(\frac{1}{4}\) inches on each side, what is the total area of the dinosaur?
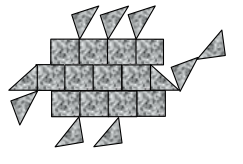
Answer:
The number of full tiles = 12
The number of half tiles = 5
Total : 12 + 5 = 17
Given, the measurement each square = 4 1/4
Area = 4 1/4 x 4 1/4
= 18 1/16 square inches
Now,
17 x 18 1/16
= ( 17 x 18 ) + ( 17 x 1/16 )
= 306 + 17/16
= 306 + 1 1/16
= 307 1/16
Therefore, the total area of the figure = 307 1/16 square inches.
Question 3.
A-Plus Glass is making windows for a new house that is being built. The box shows the list of sizes they must make.
15 windows 4\(\frac{3}{4}\) -ft long and 3\(\frac{3}{5}\)-ft wide
7 windows 2\(\frac{4}{5}\) -ft wide and 6\(\frac{1}{2}\) -ft long
How many square feet of glass will they need?
Answer:
The area of 15 windows =
4 3/4 x 3 3/5
= ( 4 x 3 ) + ( 4 x 3/5 ) + ( 3 x 3/4 ) + ( 3/4 x 3/5 )
= 12 + 12/5 + 9/4 + 9/20
= 16 + 8/20 + 5/20 +9/20
= 16 22/20
= 17 1/10 square feet
Now,
15 x 17 1/10
= 255 + 15/10
= 256 1/2 square feet.
The area of 7 windows =
2 4/5 x 6 1/2
= 17 + 6/5
= 18 1/5 square feet
Total :
17 1/10 + 18 1/5
= 35 + 1/10 + 2/10
= 35 3/10
Therefore, they will need 35 3/10 square feet of glass
Question 4.
Mr. Johnson needs to buy seed for his backyard lawn.
• If the lawn measures 40\(\frac{4}{5}\) ft by 50\(\frac{7}{8}\) ft, how many square feet of seed will he need to cover the entire area?
• One bag of seed will cover 500 square feet if he sets his seed spreader to its highest setting and 300 square feet if he sets the spreader to its lowest setting. How many bags of seed will he need if he uses the highest setting? The lowest setting?
Answer:
a.
40 4/5 x 50 7/8
= ( 40 x 50 ) + ( 40 x 7/8) + ( 50 x 4/5) + ( 4/5 x 7/8)
= 200 +280/8 +200/5 + 28/40
=2075 7/10 square feet
Therefore, he will need 2075 7/10 square feet of seed.
b.
For highest setting:
2075 7/10 square feet / 500 = 4 76/500
= 5 bags
For lowest selling :
2075 7/10 / 300
= 6 276/300
= 7 bags
Therefore, he need 5 bags for highest selling and 7 bags for the lowest selling.