There are different methods to find the Least Common Multiples. To find the L.C.M by division method, we write two numbers in a row and divide them by the prime numbers. Divide the numbers until you get 1 in the last row. Generally, the least common multiples are used to find the arithmetic operations of the fractions. Let us discuss the topic with Examples to find the least common multiple of two numbers by using the division method from here.
Practice Math Online with Unlimited Questions provided in 5th Grade Math Activity Sheets and become a blossoming mathematician in no time.
Do refer:
- To find Least Common Multiple by using Prime Factorization Method
- Examples to find Least Common Multiple by using Prime Factorization Method
LCM of Two Numbers Examples using Division Method
Some of the examples to find the L.C.M of two numbers by using the Division Method are shown below. Check out the problems and understand the concept of LCM by Division method.
Question 1.
Find least common multiple (L.C.M) of 12 and 24 by division method.
Solution:
First, write the given numbers in the rows.
Divide your numbers by prime numbers as long as at least one of your numbers is evenly divisible by a prime number.
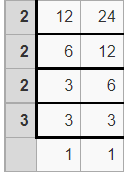
Find the product of the prime numbers in the first column to get the LCM of 12 and 24.
LCM = 2 × 2 × 2 × 3
LCM(12, 24) = 24
Question 2.
Find least common multiple (L.C.M) of 15 and 30 by division method.
Solution:
First, write the given numbers in the rows.
Divide your numbers by prime numbers as long as at least one of your numbers is evenly divisible by a prime number.
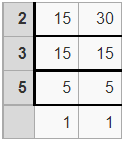
Find the product of the prime numbers in the first column to get the LCM of 15 and 30.
LCM = 2 x 3 x 5
LCM = 30
Thus the least common multiples of 15 and 30 are 30.
Question 3.
Find least common multiple (L.C.M) of 16 and 48 by division method.
Solution:
First, write the given numbers in the rows.
Divide your numbers by prime numbers as long as at least one of your numbers is evenly divisible by a prime number.
Find the product of the prime numbers in the first column to get the LCM of 16 and 48.
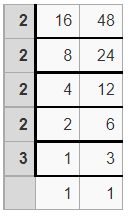
LCM = 2 x 2 x 2 x 2 x 3
The Least Common Multiple of 16 and 48 = 48
Therefore, LCM(16, 48) = 48
Question 4.
Find least common multiple (L.C.M) of 3 and 18 by division method.
Solution:
First, write the given numbers in the rows.
Divide your numbers by prime numbers as long as at least one of your numbers is evenly divisible by a prime number.
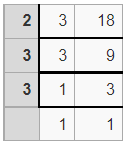
Find the product of the prime numbers in the first column to get the LCM of 3 and 18.
LCM = 2 x 3 x 3
The Least Common Multiple of 3 and 18 = 18
Therefore, LCM(3, 18) = 18
Question 5.
Find least common multiple (L.C.M) of 9 and 27 by division method.
Solution:
First, write the given numbers in the rows.
Divide your numbers by prime numbers as long as at least one of your numbers is evenly divisible by a prime number.
Find the product of the prime numbers in the first column to get the LCM of 9 and 27.
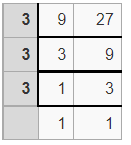
LCM = 3 x 3 x 3
The Least Common Multiple of 9 and 27 = 27
Therefore, LCM(9, 27) = 27
Question 6.
Find least common multiple (L.C.M) of 27 and 81 by division method.
Solution:
First, write the given numbers in the rows.
Divide your numbers by prime numbers as long as at least one of your numbers is evenly divisible by a prime number.
Find the product of the prime numbers in the first column to get the LCM of 27 and 81.
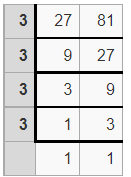
LCM = 3 x 3 x 3 x 3
The Least Common Multiple of 27 and 81 = 81
Therefore, LCM(27, 81) = 81
Question 7.
Find least common multiple (L.C.M) of 14 and 52 by division method.
Solution:
First, write the given numbers in the rows.
Divide your numbers by prime numbers as long as at least one of your numbers is evenly divisible by a prime number.
Find the product of the prime numbers in the first column to get the LCM of 14 and 52.
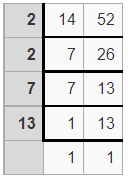
LCM = 2 x 2 x 7 x 13
The Least Common Multiple of 14 and 52 = 364
Therefore, LCM(14, 52) = 364
Question 8.
Find least common multiple (L.C.M) of 30 and 50 by division method.
Solution:
First, write the given numbers in the rows.
Divide your numbers by prime numbers as long as at least one of your numbers is evenly divisible by a prime number.
Find the product of the prime numbers in the first column to get the LCM of 30 and 50.
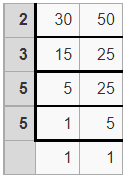
LCM = 2 x 3 x 5 x 5
The Least Common Multiple of 30 and 50 = 150
Therefore, LCM(30, 50) = 150
Question 9.
Find least common multiple (L.C.M) of 11 and 121 by division method.
Solution:
First, write the given numbers in the rows.
Divide your numbers by prime numbers as long as at least one of your numbers is evenly divisible by a prime number.
Find the product of the prime numbers in the first column to get the LCM of 11 and 121.
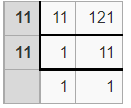
LCM = 11 x 11
The Least Common Multiple of 11 and 121 = 121
Therefore, LCM(11, 121) = 121
Question 10.
Find least common multiple (L.C.M) of 21 and 42 by division method.
Solution:
First, write the given numbers in the rows.
Divide your numbers by prime numbers as long as at least one of your numbers is evenly divisible by a prime number.
Find the product of the prime numbers in the first column to get the LCM of 21 and 42.
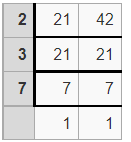
LCM = 2 x 3 x 7
The Least Common Multiple of 21 and 42 is = 42
Therefore, LCM(21, 42) = 42