Solid shapes or figures are solids having 3 dimensions, namely length, breadth, and height. Solid figures are classified into different categories. The characteristics and properties of solid shapes, the number of faces, edges, and also the number of vertices are explained below. Also, we have given examples for a better understanding of solid shapes. Also, we have given the solid shapes with clear explanations. Check out the complete concept to learn about solid shapes.
Attributes of Solid Figures
Face: The flat surface present on the solid figure is known as the Face of the solid figure.
Edge: The edge of the solid figure is defined as the line where two faces meet.
Corner: The corner is a point where three or more edges join together is known as a corner.
Different Types of Solid Figures
There are different types of solid figures available in geometry. Check out the detailed explanation of some of the examples of solid figures below.
Cube
The first and important solid figure everyone discusses Cube. A cube is defined as a slid box-shaped that has six identical square faces. One solid figure that called a cube must consist of 6 equal and plane surfaces they appear as a square in shape.
A cube consists of 6 plane surfaces, 8 vertices and, 12 edges. There are two adjoining planes available in a cube which are called surfaces that meet at an edge. Also, it consists of 12 edges that are equal in length. These edges are straight edges. Furthermore, the joining point of two corners called a vertex. In a cube, there are 8 such vertices available.
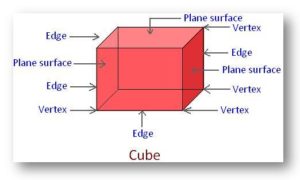
Parts of a Cube
(i) Face: The sides of a cube are known as the Face of the cube. A cube consists of six faces. All the faces of a cube are square in shapes. Each face of a cube has four equal sides.
(ii) Edge: When two edges join each other with a line segment, then that corner is called the edge. The cube has 12 edges. All the 12 edges are equal in length as all faces are squares. These edges are straight edges.
(iii) Vertex: A point formed with the joint of the three edges is known as a vertex of a cube. There are 8 vertices in a cube.
(iv) Face Diagonals: Face Diagonals of a cube is the line segment that joins the opposite vertices of a face. There are 12 diagonals in the cube that are formed with 2 diagonals in each face altogether.
(v) Space Diagonals: Space diagonals of a cube are the line segment that joins the opposite vertices of a cube and also cutting through its interior. There are 4 space diagonals in a cube.
Properties of a Cube
Volume: The volume of a cube is shown by s³ where s is the length of one edge.
Surface Area: The surface area of a cube is 6s², where s is the length of one edge.
Also, Read:
Cuboid
The cuboid consists of 6 rectangular faces which form a convex polyhedron. The opposite rectangular plane surfaces are equal. It has 8 vertices and 12 edges.
A cuboid consists of 6 rectangular plane surfaces. There are 8 vertices and 12 edges. All the faces of a cuboid are equal and square. Therefore, a cube has all the six faces equal, whereas a cuboid has the opposite faces equal.
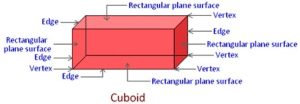
Properties of a Cuboid
Volume: The volume of a cuboid is lwh, where l is the length, h is the height, and w is the width.
Lateral Surface Area: The lateral surface area of a cuboid is 2lh + 2wh, where l is the length, w is the width, and h is the height.
Surface Area: The surface area of a cuboid is 2lw + 2lh + 2wh, where l is the length, w is the width and h is the height.
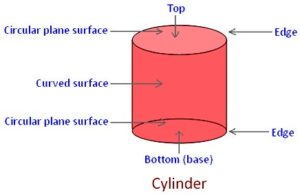
Cylinder
The cylinder is one of the basic 3d shapes that stands on a circular plane surface consisting of circular plane surfaces on its top and bottom. A cylinder has two circular plane surfaces. One surface presents at its base and the other one presents at its top. Also, it has a curved surface in the middle. Two edges at which the two plane surfaces meet with the curved surface present on a cylinder. The edges are curved in a shape.
A cylinder has 2 plane surfaces and 1 curved surface. There are 2 edges and no vertices. Furthermore, the top and bottom of the cylinder are of the same shape as well as in size. They both are equal.
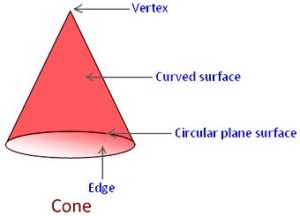
Cone
A cone is a distinctive three-dimensional geometric figure that has one plane circular surface. It consists of a base and only one curved surface. There are 1 edge and 1 vertex present in the cone. The edge of the cone is a curved edge. It is formed by the circular plane surface meeting with the curved surface.
Sphere
A sphere is a geometrical figure that has a ball-like shape. There is only one curve surface present in the sphere and no edge and no vertex present in it.